Difference between revisions of "Aufgaben:Exercise 5.2Z: Two-Way Channel"
| (13 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Stochastic_System_Theory |
}} | }} | ||
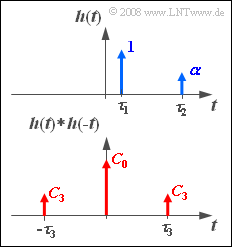
| − | [[File:P_ID517__Sto_Z_5_2.png|right| | + | [[File:P_ID517__Sto_Z_5_2.png|right|frame|Two–way channel impulse responses $h(t), h(t) * h( { - t} )$]] |
| − | + | From a transmission system it is known that following relationship exists between the input signal x(t) and the output signal y(t) : | |
:y(t)=x(t−τ1)+α⋅x(t−τ2). | :y(t)=x(t−τ1)+α⋅x(t−τ2). | ||
| − | + | The corresponding impulse response h(t) is sketched above. | |
| + | In the sketch below, the function | ||
| + | :h(t)∗h(−t)∘−−−∙|H(f)|2 | ||
| − | + | is shown, where the parameters $C_0, C_3$ and $\tau_3 depend on \alpha$, $\tau_1 and \tau_2$ ⇒ see subtask '''(4)'''. | |
| − | |||
| − | + | Let the input signal $x(t)$ be band-limited white noise | |
| + | *with power density $N_0 = 10^{-6} \hspace{0.08cm} \rm W/Hz$ | ||
| + | *and bandwidth $B = 10 \hspace{0.08cm} \rm kHz$, | ||
| − | |||
| + | from which the power Px=10mW can be calculated. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | Notes: |
| + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Stochastic_System_Theory| Stochastic System Theory]]. | ||
| + | *Use always the value $\alpha = 0.5$ for numerical calculations. | ||
| + | *For the subtasks '''(1)''' and '''(2)''', let $\tau_1 = 0 and \tau_2 = 4\hspace{0.08cm}\rm ms$ be valid. | ||
| + | *For later tasks, assume τ1=1ms and τ2=5ms. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the frequency response H(f) for τ1=0 and $\tau_2 = 4\hspace{0.08cm}\rm ms$. Show that H(f) is a periodic function with f0. What is the value of f0? |
|type="{}"} | |type="{}"} | ||
| − | f0 = { 0.25 3% } kHz | + | $f_0 \ = \ { 0.25 3% }\ \rm kHz$ |
| − | { | + | {What is the size of |H(f)|2 with τ1=0, $\tau_2 = 4\hspace{0.08cm}\rm ms$ and α=0.5? Enter the value at f=0. |
|type="{}"} | |type="{}"} | ||
| − | |H(f=0)|2 = { 2.25 3% } | + | $|H(f = 0)|^2 \ = \ $ { 2.25 3% } |
| − | { | + | {How does |H(f)|2 change with $\tau_1 = 1\hspace{0.08cm}\rm ms$ and $\tau_2 = 5\hspace{0.08cm}\rm ms$? Let the attenuation constant still be α=0.5. Enter the value at f=0. |
|type="{}"} | |type="{}"} | ||
| − | |H(f=0)|2 = { 2.25 } | + | $|H(f = 0)|^2 \ = \ $ { 2.25 } |
| − | { | + | {Let α=0.5, $\tau_1 = 1\hspace{0.08cm}\rm ms$ and $\tau_2 = 5\hspace{0.08cm}\rm ms$ still hold. Which values result for the function parameters of h(t)⋆h(−t) according to the diagram? |
|type="{}"} | |type="{}"} | ||
| − | C0 ={ 1.25 3% } | + | $C_0 \ = \ ${ 1.25 3% } |
| − | C3 = { 0.5 3% } | + | $C_3 \ = \ $ { 0.5 3% } |
| − | τ3 = { 4 3% } ms | + | $\tau_3 \ = \ { 4 3% }\ \rm ms$ |
| − | { | + | {What is the power of the output signal $y(t)$? |
|type="{}"} | |type="{}"} | ||
| − | Py = { 12.5 3% } mW | + | $P_y \ = \ { 12.5 3% }\ \rm mW$ |
| Line 60: | Line 66: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' H(f) | + | '''(1)''' H(f) is the Fourier transform to h(t). |
| + | *Using the shifting theorem, this is with τ1=0: | ||
:H(f)=1+α⋅e−j2πfτ2=1+α⋅cos(2πfτ2)−j⋅α⋅sin(2πfτ2). | :H(f)=1+α⋅e−j2πfτ2=1+α⋅cos(2πfτ2)−j⋅α⋅sin(2πfτ2). | ||
| − | + | *If H(f) is periodic with f0, then for all integer values of i must hold: | |
| + | :$H(f+i⋅f0)=H(f).$ | ||
| + | *With f0=1/τ2=0.25kHz_ this condition is satisfied. | ||
:H(f+i⋅f0)=1+α⋅cos(2πfτ2+i2πf0τ2)−j⋅α⋅sin(2πfτ2+i2πf0τ2)=1+α⋅cos(2πfτ2)−j⋅α⋅sin(2πfτ2). | :H(f+i⋅f0)=1+α⋅cos(2πfτ2+i2πf0τ2)−j⋅α⋅sin(2πfτ2+i2πf0τ2)=1+α⋅cos(2πfτ2)−j⋅α⋅sin(2πfτ2). | ||
| − | '''(2)''' | + | |
| + | '''(2)''' The magnitude square is the sum of squared real part and squared imaginary part: | ||
:|H(f)|2=(1+α⋅cos(A))2+(α⋅sin(A))2. | :|H(f)|2=(1+α⋅cos(A))2+(α⋅sin(A))2. | ||
| − | + | *Here the angle argument is abbreviated as A=2πfτ. After multiplying, we get because of cos2(A)+sin2(A)=1: | |
:|H(f)|2=1+α2+2α⋅cos(A). | :|H(f)|2=1+α2+2α⋅cos(A). | ||
| − | + | *At the frequency $f = 0$ $($and thus $A = 0)$, the result with α=0.5 is: | |
:|H(f=0)|2=(1+α)2=1.52=2.25_. | :|H(f=0)|2=(1+α)2=1.52=2.25_. | ||
| − | |||
| − | |||
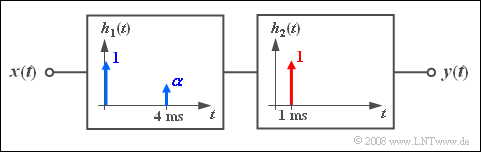
| − | + | '''(3)''' Now the transmission system can be composed of two subsystems (see diagram): | |
| + | [[File:P_ID551__Sto_Z_5_2_c.png|frame|Splitting the impulse response into two subsystems]] | ||
| + | |||
| + | *The transfer function H1(f) is calculated as in subtask '''(2)'''. | ||
| + | *For H2(f) it holds with τ1=1ms: | ||
:H2(f)=e−j2πfτ1⇒|H2(f)|=1⇒|H2(f)|2=1. | :H2(f)=e−j2πfτ1⇒|H2(f)|=1⇒|H2(f)|2=1. | ||
| − | + | *This means: Due to the additional delay time, |H(f)|2 is not changed compared to subtask '''(2)'''. | |
| + | * At the frequency f=0: $\left| {H(f = 0)} \right|^2\hspace{0.15cm} \underline{ = 2.25}$ is still valid. | ||
| + | |||
| + | |||
| − | '''(4)''' | + | '''(4)''' By comparing the drawn function h(t)⋆h(−t) with the result of subtask '''(2)''': |
:$$C_0 = 1 + \alpha ^2 \hspace{0.15cm} \underline{= 1.25}, | :$$C_0 = 1 + \alpha ^2 \hspace{0.15cm} \underline{= 1.25}, | ||
\hspace{0.5cm}C_3 = \alpha \hspace{0.15cm} \underline{= 0.5}, | \hspace{0.5cm}C_3 = \alpha \hspace{0.15cm} \underline{= 0.5}, | ||
\hspace{0.5cm}\tau _3 = \tau _2 - \tau _1 \hspace{0.15cm} \underline{= 4\;{\rm{ms}}}.$$ | \hspace{0.5cm}\tau _3 = \tau _2 - \tau _1 \hspace{0.15cm} \underline{= 4\;{\rm{ms}}}.$$ | ||
| − | '''(5)''' | + | |
| + | '''(5)''' The power-spectral density of the output signal y(t) is limited to the range of ±B and results in | ||
:Φy(f)=N0/2⋅|H(f)|2=N0/2⋅(1+α2+2α⋅cos(2πfτ3)). | :Φy(f)=N0/2⋅|H(f)|2=N0/2⋅(1+α2+2α⋅cos(2πfτ3)). | ||
| − | + | *Taking advantage of symmetry properties, we thus obtain for the power: | |
:Py=N0⋅∫B0(1+α2+2α⋅cos(2πfτ3))df. | :Py=N0⋅∫B0(1+α2+2α⋅cos(2πfτ3))df. | ||
| − | + | *$B = 10 \hspace{0.08cm} \rm kHz$ is an integer multiple of the frequency period $f_0 = 1/\tau_2= 250 \hspace{0.08cm}\rm Hz$ $($cf. solution to subtask '''1'''$)$. Therefore, the cosine function does not contribute to the integral, and we obtain: | |
:Py=N0⋅B⋅(1+α2)=1.25⋅Px=12.5mW_. | :Py=N0⋅B⋅(1+α2)=1.25⋅Px=12.5mW_. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 102: | Line 118: | ||
| − | [[Category: | + | [[Category:Theory of Stochastic Signals: Exercises|^5.1 Stochastic Systems Theory^]] |
Latest revision as of 17:51, 10 February 2022
From a transmission system it is known that following relationship exists between the input signal x(t) and the output signal y(t) :
- y(t)=x(t−τ1)+α⋅x(t−τ2).
The corresponding impulse response h(t) is sketched above.
In the sketch below, the function
- h(t)∗h(−t)∘−−−∙|H(f)|2
is shown, where the parameters C0, C3 and τ3 depend on α, τ1 and τ2 ⇒ see subtask (4).
Let the input signal x(t) be band-limited white noise
- with power density N0=10−6W/Hz
- and bandwidth B=10kHz,
from which the power Px=10mW can be calculated.
Notes:
- The exercise belongs to the chapter Stochastic System Theory.
- Use always the value α=0.5 for numerical calculations.
- For the subtasks (1) and (2), let τ1=0 and τ2=4ms be valid.
- For later tasks, assume τ1=1ms and τ2=5ms.
Questions
Solution
- Using the shifting theorem, this is with τ1=0:
- H(f)=1+α⋅e−j2πfτ2=1+α⋅cos(2πfτ2)−j⋅α⋅sin(2πfτ2).
- If H(f) is periodic with f0, then for all integer values of i must hold:
- H(f+i⋅f0)=H(f).
- With f0=1/τ2=0.25kHz_ this condition is satisfied.
- H(f+i⋅f0)=1+α⋅cos(2πfτ2+i2πf0τ2)−j⋅α⋅sin(2πfτ2+i2πf0τ2)=1+α⋅cos(2πfτ2)−j⋅α⋅sin(2πfτ2).
(2) The magnitude square is the sum of squared real part and squared imaginary part:
- |H(f)|2=(1+α⋅cos(A))2+(α⋅sin(A))2.
- Here the angle argument is abbreviated as A=2πfτ. After multiplying, we get because of cos2(A)+sin2(A)=1:
- |H(f)|2=1+α2+2α⋅cos(A).
- At the frequency f=0 (and thus A=0), the result with α=0.5 is:
- |H(f=0)|2=(1+α)2=1.52=2.25_.
(3) Now the transmission system can be composed of two subsystems (see diagram):
- The transfer function H1(f) is calculated as in subtask (2).
- For H2(f) it holds with τ1=1ms:
- H2(f)=e−j2πfτ1⇒|H2(f)|=1⇒|H2(f)|2=1.
- This means: Due to the additional delay time, |H(f)|2 is not changed compared to subtask (2).
- At the frequency f=0: |H(f=0)|2=2.25_ is still valid.
(4) By comparing the drawn function h(t)⋆h(−t) with the result of subtask (2):
- C0=1+α2=1.25_,C3=α=0.5_,τ3=τ2−τ1=4ms_.
(5) The power-spectral density of the output signal y(t) is limited to the range of ±B and results in
- Φy(f)=N0/2⋅|H(f)|2=N0/2⋅(1+α2+2α⋅cos(2πfτ3)).
- Taking advantage of symmetry properties, we thus obtain for the power:
- Py=N0⋅∫B0(1+α2+2α⋅cos(2πfτ3))df.
- B=10kHz is an integer multiple of the frequency period f0=1/τ2=250Hz (cf. solution to subtask 1). Therefore, the cosine function does not contribute to the integral, and we obtain:
- Py=N0⋅B⋅(1+α2)=1.25⋅Px=12.5mW_.