Difference between revisions of "Aufgaben:Exercise 3.7: Angular Modulation of a Harmonic Oscillation"
m (→Solution) |
|||
| (19 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Frequency_Modulation_(FM) |
}} | }} | ||
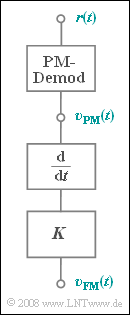
| − | [[File:P_ID1103__Mod_Z_3_6.png|right|frame| | + | [[File:P_ID1103__Mod_Z_3_6.png|right|frame|Demodulator <br>for FM]] |
| − | + | The signal arriving at a receiver is: | |
| − | :$$ r(t) = 3\,{\rm V} \cdot \cos\ | + | :$$ r(t) = 3\,{\rm V} \cdot \cos \hspace{-0.05cm} \big[2 \pi \cdot 1\,{\rm MHz} \cdot t + 3 \cdot \cos(2 \pi \cdot 10\,{\rm kHz} \cdot t)\big]\hspace{0.05cm}.$$ |
| − | + | r(t) is an angle-modulated signal that was neither distorted nor influenced by noise during transmission. | |
| − | * | + | |
| + | The signals vPM(t) and vFM(t) result after ideal demodulation by means of | ||
| + | * a phase demodulator, given by the equation | ||
:vPM(t)=1KPM⋅ϕr(t),KPM=2V−1, | :vPM(t)=1KPM⋅ϕr(t),KPM=2V−1, | ||
| − | * | + | * a frequency demodulator, consisting of a PM demodulator, a differentiator and a constant K. |
| + | |||
| + | |||
| + | In order for all signals to have equal units, this constant K is dimensionally constrained. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Modulation_Methods/Frequency_Modulation_(FM)|Frequency Modulation]]. |
| − | * | + | *Reference is also made to the chapter [[Modulation_Methods/Phase_Modulation_(PM)|Phase Modulation]] and particularly to the section [[Modulation_Methods/Frequency_Modulation_(FM)#Signal_characteristics_with_frequency_modulation|Signal characteristics with frequency modulation]]. |
| − | + | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which statements are definitely true? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + There could be a PM modulation. |
| − | + | + | + There could be a FM modulation. |
| − | - | + | - The message phase is definitely ϕ_{\rm N} = 0. |
| − | + | + | + The message phase is definitely f_{\rm N} = 10 \ \rm kHz. |
| − | { | + | {Calculate the signal v_{\rm PM}(t) after the phase demodulator. What is the signal value at time t = 0? |
|type="{}"} | |type="{}"} | ||
v_{\rm PM}(t = 0) \ = \ { 1.5 3% } \ \rm V | v_{\rm PM}(t = 0) \ = \ { 1.5 3% } \ \rm V | ||
| − | { | + | {Calculate the signal v_{\rm FM}(t). What is the message phase ϕ_{\rm N}? |
|type="{}"} | |type="{}"} | ||
ϕ_{\rm N} \ = \ { 90 3% } \ \rm Grad | ϕ_{\rm N} \ = \ { 90 3% } \ \rm Grad | ||
| − | { | + | {How should K be chosen so that the amplitude of v_{\rm FM}(t) is equal to 1.5 \ \rm V ? |
|type="{}"} | |type="{}"} | ||
| − | K\ = \ { 6.28 3% } \rm \cdot 10^4 \ 1/s | + | K\ = \ { 6.28 3% } $\ \rm \cdot 10^4 \ 1/s$ |
| − | { | + | {Which of the following statements is true for the FM-modulated signal? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The phase deviation is ϕ_{\rm max} = 3. |
| − | + | + | + The frequency deviation is Δf_{\rm A} = 30 \ \rm kHz. |
| − | + | + | + The instantaneous frequencies are between 0.97\ \rm MHz and 1.03 \ \rm MHz . |
| − | - | + | - If f_{\rm N} = 5 \ \rm kHz , the phase deviation would be unchanged. |
| − | + | + | + If f_{\rm N} = 5 \ \rm kHz the frequency deviation would be unchanged. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' <u>Answers 1, 2 and 4</u> are correct: |
| + | *From the equation for r(t) it can only be ascertained that it is an angle modulation, | ||
| + | *but not whether it is a phase modulation (PM) or a frequency modulation (FM). | ||
| + | *Based on the equation, it is clear that the message frequency is $f_{\rm N} = 10 \ \rm kHz$ . | ||
| + | *The phase $ϕ_{\rm N} = 0$ of the source signal would then only apply, if phase modulation were present. | ||
| + | |||
| + | |||
| + | |||
| + | '''(2)''' With the modulator constant K_{\rm PM} = 2 \ \rm V^{–1} this is given by: | ||
| + | :$$v_{\rm PM}(t) = \frac{1}{K_{\rm PM}} \cdot \phi_r(t) = \frac{3}{2\,{\rm V}^{-1}} \cdot \cos(2 \pi \cdot 10\,{\rm kHz} \cdot t)\hspace{0.05cm}.$$ | ||
| + | *At time t = 0 it therefore holds that: | ||
| + | :v_{\rm PM}(t = 0) = {A_{\rm N}} \hspace{0.15cm}\underline {= 1.5\,{\rm V}}\hspace{0.05cm}. | ||
| + | |||
| + | |||
| + | |||
| + | '''(3)''' The output signal v_{\rm FM}(t) of the FM demodulator – consisting of a PM–demodulator and differentiator – can be written as: | ||
| + | :v_{\rm FM}(t) = \frac{{\rm d}v_{\rm PM}(t)}{{\rm d}t} \cdot K = \frac{K \cdot A_{\rm N}}{2 \pi \cdot f_{\rm N}} \cdot (- \sin(2 \pi \cdot {f_{\rm N}} \cdot t))= \frac{K \cdot A_{\rm N}}{2 \pi \cdot f_{\rm N}} \cdot \cos(2 \pi \cdot {f_{\rm N}} \cdot t + 90^\circ)\hspace{0.05cm}. | ||
| + | *The message phase is thus ϕ_{\rm N} \hspace{0.15cm}\underline {= 90^\circ}. | ||
| + | |||
| − | ''' | + | '''(4)''' In this case, it must hold that: |
| − | $$ | + | :$$ K ={2 \pi \cdot f_{\rm N}} \hspace{0.15cm}\underline { = 6.28 \cdot 10^{4} \,\,{1}/{ s}} \hspace{0.05cm}.$$ |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | '''(5)''' <u>Answers 1, 2, 3 and 5</u> are correct: | ||
| + | *The phase deviation is identical to the modulation index, which can be discerned from the equation given: | ||
| + | :\phi_{\rm max} = \eta = 3 = \frac{\Delta f_{\rm A}}{ f_{\rm N}} \hspace{0.05cm}. | ||
| + | *This leads to the frequency deviation Δf_{\rm A} = 3 · f_{\rm N} = 30 \ \rm kHz. | ||
| + | *With a carrier frequency of f_{\rm T} = 1 \ \rm MHz , the instantaneous frequency f_{\rm T}(t) can only take values between 1±0.03 \ \rm MHz . | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | '''Thus, the following statement is also valid:''': | ||
| − | + | At half the message frequency, the phase deviation η doubles, while the frequency deviation $Δf_{\rm A}$ is unaffected: | |
| − | \eta = \frac{K_{\rm PM} \cdot A_{\rm N}}{ f_{\rm N}} = 6 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}\Delta f_{\rm A} = \eta \cdot f_{\rm N} = 6 \cdot 5\,{\rm kHz} = 30\,{\rm kHz}\hspace{0.05cm}. | + | :\eta = \frac{K_{\rm PM} \cdot A_{\rm N}}{ f_{\rm N}} = 6 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}\Delta f_{\rm A} = \eta \cdot f_{\rm N} = 6 \cdot 5\,{\rm kHz} = 30\,{\rm kHz}\hspace{0.05cm}. |
| Line 83: | Line 107: | ||
| − | [[Category: | + | [[Category:Modulation Methods: Exercises|^3.2 Frequency Modulation^]] |
Latest revision as of 17:14, 9 April 2022
The signal arriving at a receiver is:
- r(t) = 3\,{\rm V} \cdot \cos \hspace{-0.05cm} \big[2 \pi \cdot 1\,{\rm MHz} \cdot t + 3 \cdot \cos(2 \pi \cdot 10\,{\rm kHz} \cdot t)\big]\hspace{0.05cm}.
r(t) is an angle-modulated signal that was neither distorted nor influenced by noise during transmission.
The signals v_{\rm PM}(t) and v_{\rm FM}(t) result after ideal demodulation by means of
- a phase demodulator, given by the equation
- v_{\rm PM}(t) = \frac{1}{K_{\rm PM}} \cdot \phi_r(t) \hspace{0.05cm},\hspace{0.3cm} {K_{\rm PM}} = 2\,{\rm V}^{-1}\hspace{0.05cm},
- a frequency demodulator, consisting of a PM demodulator, a differentiator and a constant K.
In order for all signals to have equal units, this constant K is dimensionally constrained.
Hints:
- This exercise belongs to the chapter Frequency Modulation.
- Reference is also made to the chapter Phase Modulation and particularly to the section Signal characteristics with frequency modulation.
Questions
Solution
- From the equation for r(t) it can only be ascertained that it is an angle modulation,
- but not whether it is a phase modulation (PM) or a frequency modulation (FM).
- Based on the equation, it is clear that the message frequency is f_{\rm N} = 10 \ \rm kHz .
- The phase ϕ_{\rm N} = 0 of the source signal would then only apply, if phase modulation were present.
(2) With the modulator constant K_{\rm PM} = 2 \ \rm V^{–1} this is given by:
- v_{\rm PM}(t) = \frac{1}{K_{\rm PM}} \cdot \phi_r(t) = \frac{3}{2\,{\rm V}^{-1}} \cdot \cos(2 \pi \cdot 10\,{\rm kHz} \cdot t)\hspace{0.05cm}.
- At time t = 0 it therefore holds that:
- v_{\rm PM}(t = 0) = {A_{\rm N}} \hspace{0.15cm}\underline {= 1.5\,{\rm V}}\hspace{0.05cm}.
(3) The output signal v_{\rm FM}(t) of the FM demodulator – consisting of a PM–demodulator and differentiator – can be written as:
- v_{\rm FM}(t) = \frac{{\rm d}v_{\rm PM}(t)}{{\rm d}t} \cdot K = \frac{K \cdot A_{\rm N}}{2 \pi \cdot f_{\rm N}} \cdot (- \sin(2 \pi \cdot {f_{\rm N}} \cdot t))= \frac{K \cdot A_{\rm N}}{2 \pi \cdot f_{\rm N}} \cdot \cos(2 \pi \cdot {f_{\rm N}} \cdot t + 90^\circ)\hspace{0.05cm}.
- The message phase is thus ϕ_{\rm N} \hspace{0.15cm}\underline {= 90^\circ}.
(4) In this case, it must hold that:
- K ={2 \pi \cdot f_{\rm N}} \hspace{0.15cm}\underline { = 6.28 \cdot 10^{4} \,\,{1}/{ s}} \hspace{0.05cm}.
(5) Answers 1, 2, 3 and 5 are correct:
- The phase deviation is identical to the modulation index, which can be discerned from the equation given:
- \phi_{\rm max} = \eta = 3 = \frac{\Delta f_{\rm A}}{ f_{\rm N}} \hspace{0.05cm}.
- This leads to the frequency deviation Δf_{\rm A} = 3 · f_{\rm N} = 30 \ \rm kHz.
- With a carrier frequency of f_{\rm T} = 1 \ \rm MHz , the instantaneous frequency f_{\rm T}(t) can only take values between 1±0.03 \ \rm MHz .
Thus, the following statement is also valid::
At half the message frequency, the phase deviation η doubles, while the frequency deviation Δf_{\rm A} is unaffected:
- \eta = \frac{K_{\rm PM} \cdot A_{\rm N}}{ f_{\rm N}} = 6 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}\Delta f_{\rm A} = \eta \cdot f_{\rm N} = 6 \cdot 5\,{\rm kHz} = 30\,{\rm kHz}\hspace{0.05cm}.