Difference between revisions of "Aufgaben:Exercise 1.09: BPSK and 4-QAM"
| (25 intermediate revisions by 6 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation |
}} | }} | ||
| − | [[File:P_ID1682__Dig_A_4_2.png|right|frame| | + | [[File:P_ID1682__Dig_A_4_2.png|right|frame|Phase diagrams of BPSK and 4-QAM]] |
| − | + | The diagram shows schematically the phase diagrams of the "binary phase modulation" $($abbreviated $\rm BPSK)$ and the "quadrature amplitude modulation" $($called $\rm 4–QAM)$. | |
| − | + | *The latter can be described by two BPSK systems with cosine and minus-sine carriers, where for each of the subcomponents the transmission amplitude is reduced by a factor of $\sqrt{2}$ compared to BPSK. | |
| + | |||
| + | *The envelope of the total signal $s(t)$ is thus also constant equal to $s_{0}$. | ||
| + | |||
| + | *The error probability depending on the quotient $E_{\rm B}/N_{0}$ is the same for BPSK and 4–QAM: | ||
:$$p_{\rm B} = \ {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm B}}/{N_0 }} \hspace{0.1cm}\right | :$$p_{\rm B} = \ {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm B}}/{N_0 }} \hspace{0.1cm}\right | ||
) = \ {1}/{2}\cdot {\rm erfc}\left ( \sqrt{E_{\rm B}/{ N_0 }} \right ).$$ | ) = \ {1}/{2}\cdot {\rm erfc}\left ( \sqrt{E_{\rm B}/{ N_0 }} \right ).$$ | ||
| − | + | ||
| + | However, the error probability of the BPSK system can also be expressed in the form | ||
:$$p_{\rm B,\hspace{0.04cm}BPSK} = {\rm Q}\left ( \frac{s_0}{\sigma_d } \right | :$$p_{\rm B,\hspace{0.04cm}BPSK} = {\rm Q}\left ( \frac{s_0}{\sigma_d } \right | ||
| − | )\hspace{0.2cm}{\rm | + | )\hspace{0.2cm}{\rm with}\hspace{0.2cm}\sigma_d = \sqrt{\frac{N_0}{T_{\rm B}}}$$ |
| − | + | Correspondingly, for the 4-QAM system: | |
:$$p_{\rm B,\hspace{0.04cm}QAM} = {\rm Q}\left ( \frac{s_0/\sqrt{2}}{\sigma_d } \right | :$$p_{\rm B,\hspace{0.04cm}QAM} = {\rm Q}\left ( \frac{s_0/\sqrt{2}}{\sigma_d } \right | ||
| − | )\hspace{0.2cm}{\rm | + | )\hspace{0.2cm}{\rm with}\hspace{0.2cm}\sigma_d = \sqrt{\frac{N_0}{2 \cdot T_{\rm |
B}}}.$$ | B}}}.$$ | ||
| − | + | ||
| − | + | The equations are valid only under the condition of exact phase synchronization: | |
| + | *If there is a phase offset $\Delta\phi_{\rm T}$ between the transmitted and received carrier signals, the error probability increases significantly, with BPSK and QAM systems being degraded differently. | ||
| + | |||
| + | *In the phase diagram, the phase offset is noticeable by a rotation of the point clouds. | ||
| + | |||
| + | *In the diagram, the centers of the point clouds for $\Delta\phi_{\rm T} = 15^\circ$ are marked by yellow crosses, while the red circles indicate the centers for $\Delta\phi_{\rm T} = 0$. | ||
| + | |||
| + | |||
| + | $E_{\rm B}/N_{0} = 8$ always holds, so the error probabilities of BPSK and QAM in the best case (without phase shift) are as follows ⇒ [[Aufgaben:Exercise_1.08Z:_BPSK_Error_Probability|"Exercise 1.8Z"]]: | ||
:$$p_{\rm B} = {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm B}}/{N_0 }} \hspace{0.1cm}\right ) = {\rm Q}(4)= 0.317 \cdot 10^{-4}.$$ | :$$p_{\rm B} = {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm B}}/{N_0 }} \hspace{0.1cm}\right ) = {\rm Q}(4)= 0.317 \cdot 10^{-4}.$$ | ||
| − | + | <u>Further remarks:</u> | |
| + | *If we denote the distance of the BPSK useful samples (without noise) from the (vertical) decision threshold by $s_{0}$, we get $\sigma_{d} = s_{0}/4$ for the noise rms value. The lighter circles in the diagram mark the contour lines with radius $2\cdot \sigma_{d}$ and $3\cdot \sigma_{d}$ of the two-dimensional Gaussian PDF. | ||
| + | |||
| + | *For the 4-QAM, compared to the BPSK, the distances of the noise-free samples drawn in red from the now two decision thresholds are each smaller by a factor of $\sqrt{2}$, but it also results in a noise rms value $\sigma_{d}$ smaller by the same factor. | ||
| + | |||
| − | |||
| − | |||
| − | * | + | Notes: |
| − | + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation|"Linear Digital Modulation - Coherent Demodulation"]]. | |
| − | === | + | *Reference is made in particular to the section [[Digital_Signal_Transmission/Linear_Digital_Modulation_-_Coherent_Demodulation#Phase_offset_between_transmitter_and_receiver|"Phase offset between transmitter and receiver"]]. |
| + | |||
| + | *You can determine the values of the Q–function with the HTML5/JavaScript applet [[Applets:Komplementäre_Gaußsche_Fehlerfunktionen|"Complementary Gaussian Error Functions"]]. | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the bit error probability for BPSK with $\Delta\phi_{\rm T} = 15^\circ$? |
|type="{}"} | |type="{}"} | ||
| − | $ \ = \ $ { | + | $p_\text{B, BPSK} \ = \ $ { 0.0057 3% } $\ \% $ |
| − | + | {What is the bit error probability for BPSK with $\Delta\phi_{\rm T} = 45^\circ$? | |
|type="{}"} | |type="{}"} | ||
| − | $ \ = \ $ { | + | $p_\text{B, BPSK} \ = \ $ { 0.233 3% } $\ \%$ |
| − | { | + | {What is the bit error probability for 4-QAM with $\Delta\phi_{\rm T} = 15^\circ$? |
|type="{}"} | |type="{}"} | ||
| − | $ \ = \ $ { | + | $p_\text{B, 4-QAM} \ = \ $ { 0.117 3% } $\ \%$ |
| − | { | + | {What is the bit error probability for 4-QAM with $\Delta\phi_{\rm T} = 45^\circ$? |
|type="{}"} | |type="{}"} | ||
| − | $ \ = \ $ { | + | $p_\text{B, 4-QAM} \ = \ $ { 25 3% } $\ \%$ |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | |
| − | '''(2)''' | + | '''(1)''' Rotating the phase diagram by $\Delta\phi_{\rm T} = 15^\circ$ decreases the distance of the useful samples from the threshold by $\cos(15^\circ) \approx 0.966$. It follows that: |
| − | '''( | + | :$$p_{\rm B} = {\rm Q}(0.966 \cdot 4) \approx {\rm Q}(3.86)= 0.57 \cdot 10^{-4}\hspace{0.1cm}\underline {= 0.0057\, \%}.$$ |
| − | '''( | + | |
| − | '''( | + | |
| − | + | '''(2)''' Analogous to subtask '''(1)''', $\cos(45^\circ) \approx 0.707$ is obtained: | |
| + | :$$p_{\rm B} = {\rm Q}(0.707 \cdot 4) \approx {\rm Q}(2.83)\hspace{0.1cm}\underline {= 0.233 \, \%}.$$ | ||
| + | |||
| + | |||
| + | '''(3)''' For 4-QAM, clockwise rotation by $\Delta\phi_{\rm T}$ increases the distance | ||
| + | *from the horizontal threshold (decision of the first bit) equals $s_{0} \cdot \cos(45^\circ + \Delta\phi_{\rm T})$, i.e., smaller than without phase shift, | ||
| + | |||
| + | *from the vertical threshold (decision of the second bit) equal to $s_{0} \cdot \cos(45^\circ - \Delta\phi_{\rm T})$, thus larger than without phase shift. | ||
| + | |||
| + | |||
| + | Thus, we obtain for the average error probability: | ||
| + | :$$p_{\rm B} = {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ+{\rm \Delta} \phi_{\rm | ||
| + | T}) \cdot s_0}{0.25 \cdot s_0 / \sqrt{2}} \right | ||
| + | ) + {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ-{\rm \Delta} \phi_{\rm | ||
| + | T}) \cdot s_0}{0.25 \cdot s_0 / \sqrt{2}}\right | ||
| + | ).$$ | ||
| + | *This already takes into account the smaller noise rms value of the 4-QAM. | ||
| + | |||
| + | *As a check, we calculate the error probability for $\Delta\phi_{\rm T} = 0$: | ||
| + | :$$p_{\rm B} = {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ) \cdot 4}{1 / \sqrt{2}} \right | ||
| + | ) +{1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ) \cdot 4}{1 / \sqrt{2}} \right | ||
| + | )= {\rm Q}(4) = 0.317 \cdot 10^{-4}.$$ | ||
| + | |||
| + | *On the other hand, we obtain with $\Delta\phi_{\rm T} = 15^\circ$: | ||
| + | :$$p_{\rm B} = {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(60^\circ) \cdot 4}{1 / \sqrt{2}} \right | ||
| + | ) +{1}/{2} \cdot {\rm Q}\left ( \frac{\cos(30^\circ) \cdot 4}{1 / \sqrt{2}} \right | ||
| + | )= {1}/{2} \cdot \left [{\rm Q}(2.83)+ {\rm Q}(4.90)\right]$$ | ||
| + | :$$\Rightarrow \hspace{0.3cm} p_{\rm B} \approx | ||
| + | \frac{1}{2} \cdot \left [0.233 \cdot 10^{-2}+ 0.479 \cdot 10^{-6}\right] \hspace{0.1cm}\underline {= 0.117 \, \%}.$$ | ||
| + | |||
| + | |||
| + | '''(4)''' With a phase shift of $45^\circ$, one obtains from the equation generally derived above: | ||
| + | :$$p_{\rm B} ={1}/{2} \cdot {\rm Q}\left ( \frac{\cos(90^\circ) \cdot 4}{1 / \sqrt{2}} \right | ||
| + | ) +{1}/{2} \cdot {\rm Q}\left ( \frac{\cos(0^\circ) \cdot 4}{1 / \sqrt{2}} \right | ||
| + | )= {1}/{2} \cdot \left [{\rm Q}(0)+ {\rm Q}(5.66)\right] \approx 0.25\hspace{0.1cm}\underline {= 25 \, \%}.$$ | ||
| + | |||
| + | That is: | ||
| + | *The bit error rate for the first bit is $50\%$. | ||
| + | |||
| + | *In contrast, the second bit is decided almost error-free $(\approx 10^{–8})$. | ||
| + | |||
| + | *Overall, this results in a mean bit error probability of approx. $25\%$. | ||
| + | |||
| + | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 64: | Line 128: | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^1.5 Linear Digital Modulation^]] |
Latest revision as of 08:30, 20 May 2022
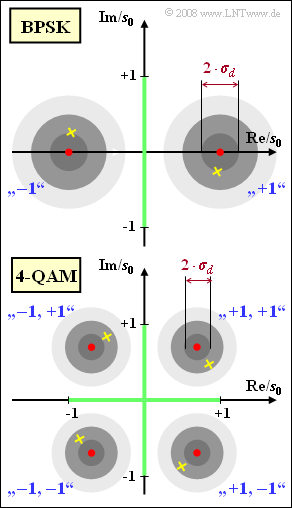
The diagram shows schematically the phase diagrams of the "binary phase modulation" $($abbreviated $\rm BPSK)$ and the "quadrature amplitude modulation" $($called $\rm 4–QAM)$.
- The latter can be described by two BPSK systems with cosine and minus-sine carriers, where for each of the subcomponents the transmission amplitude is reduced by a factor of $\sqrt{2}$ compared to BPSK.
- The envelope of the total signal $s(t)$ is thus also constant equal to $s_{0}$.
- The error probability depending on the quotient $E_{\rm B}/N_{0}$ is the same for BPSK and 4–QAM:
- $$p_{\rm B} = \ {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm B}}/{N_0 }} \hspace{0.1cm}\right ) = \ {1}/{2}\cdot {\rm erfc}\left ( \sqrt{E_{\rm B}/{ N_0 }} \right ).$$
However, the error probability of the BPSK system can also be expressed in the form
- $$p_{\rm B,\hspace{0.04cm}BPSK} = {\rm Q}\left ( \frac{s_0}{\sigma_d } \right )\hspace{0.2cm}{\rm with}\hspace{0.2cm}\sigma_d = \sqrt{\frac{N_0}{T_{\rm B}}}$$
Correspondingly, for the 4-QAM system:
- $$p_{\rm B,\hspace{0.04cm}QAM} = {\rm Q}\left ( \frac{s_0/\sqrt{2}}{\sigma_d } \right )\hspace{0.2cm}{\rm with}\hspace{0.2cm}\sigma_d = \sqrt{\frac{N_0}{2 \cdot T_{\rm B}}}.$$
The equations are valid only under the condition of exact phase synchronization:
- If there is a phase offset $\Delta\phi_{\rm T}$ between the transmitted and received carrier signals, the error probability increases significantly, with BPSK and QAM systems being degraded differently.
- In the phase diagram, the phase offset is noticeable by a rotation of the point clouds.
- In the diagram, the centers of the point clouds for $\Delta\phi_{\rm T} = 15^\circ$ are marked by yellow crosses, while the red circles indicate the centers for $\Delta\phi_{\rm T} = 0$.
$E_{\rm B}/N_{0} = 8$ always holds, so the error probabilities of BPSK and QAM in the best case (without phase shift) are as follows ⇒ "Exercise 1.8Z":
- $$p_{\rm B} = {\rm Q}\left ( \sqrt{{2 \cdot E_{\rm B}}/{N_0 }} \hspace{0.1cm}\right ) = {\rm Q}(4)= 0.317 \cdot 10^{-4}.$$
Further remarks:
- If we denote the distance of the BPSK useful samples (without noise) from the (vertical) decision threshold by $s_{0}$, we get $\sigma_{d} = s_{0}/4$ for the noise rms value. The lighter circles in the diagram mark the contour lines with radius $2\cdot \sigma_{d}$ and $3\cdot \sigma_{d}$ of the two-dimensional Gaussian PDF.
- For the 4-QAM, compared to the BPSK, the distances of the noise-free samples drawn in red from the now two decision thresholds are each smaller by a factor of $\sqrt{2}$, but it also results in a noise rms value $\sigma_{d}$ smaller by the same factor.
Notes:
- The exercise belongs to the chapter "Linear Digital Modulation - Coherent Demodulation".
- Reference is made in particular to the section "Phase offset between transmitter and receiver".
- You can determine the values of the Q–function with the HTML5/JavaScript applet "Complementary Gaussian Error Functions".
Questions
Solution
(1) Rotating the phase diagram by $\Delta\phi_{\rm T} = 15^\circ$ decreases the distance of the useful samples from the threshold by $\cos(15^\circ) \approx 0.966$. It follows that:
- $$p_{\rm B} = {\rm Q}(0.966 \cdot 4) \approx {\rm Q}(3.86)= 0.57 \cdot 10^{-4}\hspace{0.1cm}\underline {= 0.0057\, \%}.$$
(2) Analogous to subtask (1), $\cos(45^\circ) \approx 0.707$ is obtained:
- $$p_{\rm B} = {\rm Q}(0.707 \cdot 4) \approx {\rm Q}(2.83)\hspace{0.1cm}\underline {= 0.233 \, \%}.$$
(3) For 4-QAM, clockwise rotation by $\Delta\phi_{\rm T}$ increases the distance
- from the horizontal threshold (decision of the first bit) equals $s_{0} \cdot \cos(45^\circ + \Delta\phi_{\rm T})$, i.e., smaller than without phase shift,
- from the vertical threshold (decision of the second bit) equal to $s_{0} \cdot \cos(45^\circ - \Delta\phi_{\rm T})$, thus larger than without phase shift.
Thus, we obtain for the average error probability:
- $$p_{\rm B} = {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ+{\rm \Delta} \phi_{\rm T}) \cdot s_0}{0.25 \cdot s_0 / \sqrt{2}} \right ) + {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ-{\rm \Delta} \phi_{\rm T}) \cdot s_0}{0.25 \cdot s_0 / \sqrt{2}}\right ).$$
- This already takes into account the smaller noise rms value of the 4-QAM.
- As a check, we calculate the error probability for $\Delta\phi_{\rm T} = 0$:
- $$p_{\rm B} = {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ) \cdot 4}{1 / \sqrt{2}} \right ) +{1}/{2} \cdot {\rm Q}\left ( \frac{\cos(45^\circ) \cdot 4}{1 / \sqrt{2}} \right )= {\rm Q}(4) = 0.317 \cdot 10^{-4}.$$
- On the other hand, we obtain with $\Delta\phi_{\rm T} = 15^\circ$:
- $$p_{\rm B} = {1}/{2} \cdot {\rm Q}\left ( \frac{\cos(60^\circ) \cdot 4}{1 / \sqrt{2}} \right ) +{1}/{2} \cdot {\rm Q}\left ( \frac{\cos(30^\circ) \cdot 4}{1 / \sqrt{2}} \right )= {1}/{2} \cdot \left [{\rm Q}(2.83)+ {\rm Q}(4.90)\right]$$
- $$\Rightarrow \hspace{0.3cm} p_{\rm B} \approx \frac{1}{2} \cdot \left [0.233 \cdot 10^{-2}+ 0.479 \cdot 10^{-6}\right] \hspace{0.1cm}\underline {= 0.117 \, \%}.$$
(4) With a phase shift of $45^\circ$, one obtains from the equation generally derived above:
- $$p_{\rm B} ={1}/{2} \cdot {\rm Q}\left ( \frac{\cos(90^\circ) \cdot 4}{1 / \sqrt{2}} \right ) +{1}/{2} \cdot {\rm Q}\left ( \frac{\cos(0^\circ) \cdot 4}{1 / \sqrt{2}} \right )= {1}/{2} \cdot \left [{\rm Q}(0)+ {\rm Q}(5.66)\right] \approx 0.25\hspace{0.1cm}\underline {= 25 \, \%}.$$
That is:
- The bit error rate for the first bit is $50\%$.
- In contrast, the second bit is decided almost error-free $(\approx 10^{–8})$.
- Overall, this results in a mean bit error probability of approx. $25\%$.