Difference between revisions of "Aufgaben:Exercise 4.17Z: Rayleigh and Rice Distribution"
| (10 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Carrier_Frequency_Systems_with_Non-Coherent_Demodulation}} |
| − | [[File: | + | [[File:P_ID2079__Dig_Z_4_17_ret.png|right|frame|Rice (top) and Rayleigh (bottom)]] |
| − | + | For the study of transmission systems, the Rayleigh and Rice distributions are of great importance. In the following let y be a Rayleigh or a Rice distributed random variable and η in each case a realization of it. | |
| − | * | + | * The "Rayleigh distribution" results thereby for the probability density function $\rm (PDF)$ of a random variable y, which results from the two Gaussian distributed and statistically independent components u and $v$ $($both with the standard deviation $\sigma_n)$ as follows: |
:$$y = \sqrt{u^2 + v^2} \hspace{0.1cm} \Rightarrow \hspace{0.1cm} p_y (\eta) = \frac{\eta}{\sigma_n^2} | :$$y = \sqrt{u^2 + v^2} \hspace{0.1cm} \Rightarrow \hspace{0.1cm} p_y (\eta) = \frac{\eta}{\sigma_n^2} | ||
\cdot {\rm exp } \left [ - \frac{\eta^2}{2 \sigma_n^2}\right ] | \cdot {\rm exp } \left [ - \frac{\eta^2}{2 \sigma_n^2}\right ] | ||
\hspace{0.01cm}.$$ | \hspace{0.01cm}.$$ | ||
| − | * | + | * The "Rice distribution" is obtained under otherwise identical boundary conditions for the application case where a constant C is still added to one of the two components, for example: |
:$$y = \sqrt{(u+C)^2 + v^2} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_y (\eta) = \frac{\eta}{\sigma_n^2} | :$$y = \sqrt{(u+C)^2 + v^2} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_y (\eta) = \frac{\eta}{\sigma_n^2} | ||
\cdot {\rm exp } \left [ - \frac{\eta^2 + C^2}{2 \sigma_n^2}\right ] \cdot {\rm I }_0 \left [ \frac{\eta \cdot C}{ \sigma_n^2}\right ] | \cdot {\rm exp } \left [ - \frac{\eta^2 + C^2}{2 \sigma_n^2}\right ] \cdot {\rm I }_0 \left [ \frac{\eta \cdot C}{ \sigma_n^2}\right ] | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | In | + | In this equation, I0(x) denotes the [[Digital_Signal_Transmission/Carrier_Frequency_Systems_with_Non-Coherent_Demodulation#Rayleigh_and_Rice_Distribution|"modified zero-order Bessel function"]]. |
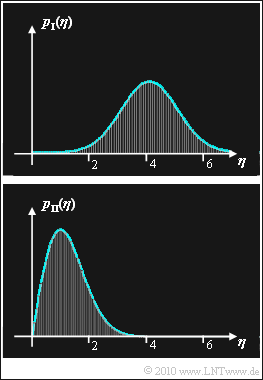
| − | In | + | In the graph, the two probability density functions are shown, but it is not indicated whether pI(η) or pII(η) belong to a Rayleigh or a Rice distribution, respectively. |
| + | * It is only known that one Rayleigh and one Rice distribution is shown. | ||
| − | + | * The parameter σn is the same for both distributions. | |
| − | |||
| − | |||
| − | + | For your decision whether to assign pI(η) or pII(η) to the Rice distribution and for the determination of the PDF parameters you can consider the following statements: | |
| − | * | + | * For large values of the quotient C/σn, the Rice distribution can be approximated by a Gaussian distribution with mean C and standard deviation σn. |
| − | * | + | |
| + | * The values of C and σn underlying the graph are integers. | ||
| + | |||
| + | |||
| + | Regarding the Rayleigh distribution, note: | ||
| + | * The same σn is used for both distributions. | ||
| + | |||
| + | * For the standard deviation (root of the variance) of the Rayleigh distribution holds: | ||
:$$\sigma_y = \sigma_n \cdot \sqrt{2 - {\pi}/{2 }} \hspace{0.2cm} \approx \hspace{0.2cm} 0.655 \cdot \sigma_n | :$$\sigma_y = \sigma_n \cdot \sqrt{2 - {\pi}/{2 }} \hspace{0.2cm} \approx \hspace{0.2cm} 0.655 \cdot \sigma_n | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | |||
| + | * For the standard deviation or for the variance of the Rice distribution in general only a complicated expression with hypergeometric functions can be given, otherwise only an approximation for C≫σn corresponding to the Gaussian distribution. | ||
| − | + | ||
| − | * | + | |
| − | + | ||
| − | * | + | |
| + | Notes: | ||
| + | * This exercise belongs to the chapter [[Digital_Signal_Transmission/Carrier_Frequency_Systems_with_Non-Coherent_Demodulation|"Carrier Frequency Systems with Non-Coherent Demodulation"]]. | ||
| + | |||
| + | * Given is also the following indefinite integral: | ||
:$$\int x \cdot {\rm e }^{-x^2} \,{\rm d} x = | :$$\int x \cdot {\rm e }^{-x^2} \,{\rm d} x = | ||
-{1}/{2} \cdot {\rm e }^{-x^2} + {\rm const. } $$ | -{1}/{2} \cdot {\rm e }^{-x^2} + {\rm const. } $$ | ||
| Line 42: | Line 52: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Assign the graphs to the Rayleigh and Rice distributions, respectively. |
|type="()"} | |type="()"} | ||
| − | - pI(η) | + | - pI(η) corresponds to the Rayleigh distribution, pII(η) to the Rice distribution. |
| − | + pI(η) | + | + pI(η) corresponds to the Rice distribution, pII(η) to the Rayleigh distribution. |
| − | { | + | {Give the parameters of the Rice distribution shown here. |
|type="{}"} | |type="{}"} | ||
C= { 4 3% } | C= { 4 3% } | ||
σn = { 1 3% } | σn = { 1 3% } | ||
| − | { | + | {Which distribution has a larger variance? |
|type="()"} | |type="()"} | ||
| − | - | + | - The Rayleigh distribution, |
| − | + | + | + the Rice distribution? |
| − | { | + | {Calculate the excess probabilities of the Rayleigh distribution. |
|type="{}"} | |type="{}"} | ||
Pr(y>σn)= { 60.7 3% } % | Pr(y>σn)= { 60.7 3% } % | ||
| Line 66: | Line 76: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The <u>second solution</u> is correct: |
| − | * | + | *The upper graph shows approximately a Gaussian distribution and belongs accordingly to the Rice distribution. |
| + | |||
| − | '''(2)''' | + | '''(2)''' You can see from the graph: The mean value of the Gaussian distribution is C=4_ and the standard deviation is σn=1_. |
| + | *It was given that C and σn were integers. Thus the two density functions are: | ||
:$$p_{\rm I} (\eta) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {\eta} | :$$p_{\rm I} (\eta) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {\eta} | ||
\cdot {\rm exp } \left [ - \frac{\eta^2 + 16}{2 }\right ] \cdot {\rm I }_0 (4\eta ) \approx | \cdot {\rm exp } \left [ - \frac{\eta^2 + 16}{2 }\right ] \cdot {\rm I }_0 (4\eta ) \approx | ||
| Line 79: | Line 91: | ||
\cdot {\rm exp } \left [ - \frac{\eta^2 }{2 }\right ] \hspace{0.05cm}.$$ | \cdot {\rm exp } \left [ - \frac{\eta^2 }{2 }\right ] \hspace{0.05cm}.$$ | ||
| − | '''(3)''' | + | |
| + | '''(3)''' <u>Solution 2</u> is correct, as can already be seen from the graph. A calculation confirms this result: | ||
:σ2Rice = σ2n=1, | :σ2Rice = σ2n=1, | ||
:$$ \sigma_{\rm Rayl}^2 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sigma_n^2 \cdot ({2 - {\pi}/{2 }}) \approx 0.429 | :$$ \sigma_{\rm Rayl}^2 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sigma_n^2 \cdot ({2 - {\pi}/{2 }}) \approx 0.429 | ||
| Line 85: | Line 98: | ||
| − | '''(4)''' | + | '''(4)''' In general, the probability that y is greater than a certain value y0 is equal to |
:$${\rm Pr}(y > y_0) = \int_{y_0}^{\infty} \frac{\eta}{\sigma_n^2} | :$${\rm Pr}(y > y_0) = \int_{y_0}^{\infty} \frac{\eta}{\sigma_n^2} | ||
\cdot {\rm exp } \left [ - \frac{\eta^2 }{2 \sigma_n^2}\right ] \,{\rm d} \eta | \cdot {\rm exp } \left [ - \frac{\eta^2 }{2 \sigma_n^2}\right ] \,{\rm d} \eta | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | *With the substitution x2=η2/(2σ2n) can be written for this: | |
:$${\rm Pr}(y > y_0) = 2 \cdot \hspace{-0.05cm}\int_{y_0/(\sqrt{2}\hspace{0.03cm} \cdot \hspace{0.03cm} \sigma_n)}^{\infty} \hspace{-0.5cm}x | :$${\rm Pr}(y > y_0) = 2 \cdot \hspace{-0.05cm}\int_{y_0/(\sqrt{2}\hspace{0.03cm} \cdot \hspace{0.03cm} \sigma_n)}^{\infty} \hspace{-0.5cm}x | ||
\cdot {\rm e }^{ - x^2} \,{\rm d} x = \left [{\rm e }^{ - x^2} \right ]_{\sqrt{2}\hspace{0.03cm} \cdot \hspace{0.03cm} \sigma_n}^{\infty} | \cdot {\rm e }^{ - x^2} \,{\rm d} x = \left [{\rm e }^{ - x^2} \right ]_{\sqrt{2}\hspace{0.03cm} \cdot \hspace{0.03cm} \sigma_n}^{\infty} | ||
= {\rm exp } \left [ -\frac{ y_0^2 }{2 \sigma_n^2 }\right ]\hspace{0.05cm}.$$ | = {\rm exp } \left [ -\frac{ y_0^2 }{2 \sigma_n^2 }\right ]\hspace{0.05cm}.$$ | ||
| − | + | *Here the indefinite integral given in the front was used. In particular: | |
:Pr(y>σn) = e−0.5≈60.7%_, | :Pr(y>σn) = e−0.5≈60.7%_, | ||
:Pr(y>2σn) = e−2.0≈13.5%_, | :Pr(y>2σn) = e−2.0≈13.5%_, | ||
| Line 104: | Line 117: | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^4.5 Non-Coherent Demodulation^]] |
Latest revision as of 13:41, 10 October 2022
For the study of transmission systems, the Rayleigh and Rice distributions are of great importance. In the following let y be a Rayleigh or a Rice distributed random variable and η in each case a realization of it.
- The "Rayleigh distribution" results thereby for the probability density function (PDF) of a random variable y, which results from the two Gaussian distributed and statistically independent components u and v (both with the standard deviation σn) as follows:
- y=√u2+v2⇒py(η)=ησ2n⋅exp[−η22σ2n].
- The "Rice distribution" is obtained under otherwise identical boundary conditions for the application case where a constant C is still added to one of the two components, for example:
- y=√(u+C)2+v2⇒py(η)=ησ2n⋅exp[−η2+C22σ2n]⋅I0[η⋅Cσ2n].
In this equation, I0(x) denotes the "modified zero-order Bessel function".
In the graph, the two probability density functions are shown, but it is not indicated whether pI(η) or pII(η) belong to a Rayleigh or a Rice distribution, respectively.
- It is only known that one Rayleigh and one Rice distribution is shown.
- The parameter σn is the same for both distributions.
For your decision whether to assign pI(η) or pII(η) to the Rice distribution and for the determination of the PDF parameters you can consider the following statements:
- For large values of the quotient C/σn, the Rice distribution can be approximated by a Gaussian distribution with mean C and standard deviation σn.
- The values of C and σn underlying the graph are integers.
Regarding the Rayleigh distribution, note:
- The same σn is used for both distributions.
- For the standard deviation (root of the variance) of the Rayleigh distribution holds:
- σy=σn⋅√2−π/2≈0.655⋅σn.
- For the standard deviation or for the variance of the Rice distribution in general only a complicated expression with hypergeometric functions can be given, otherwise only an approximation for C≫σn corresponding to the Gaussian distribution.
Notes:
- This exercise belongs to the chapter "Carrier Frequency Systems with Non-Coherent Demodulation".
- Given is also the following indefinite integral:
- ∫x⋅e−x2dx=−1/2⋅e−x2+const.
Questions
Solution
- The upper graph shows approximately a Gaussian distribution and belongs accordingly to the Rice distribution.
(2) You can see from the graph: The mean value of the Gaussian distribution is C=4_ and the standard deviation is σn=1_.
- It was given that C and σn were integers. Thus the two density functions are:
- pI(η) = η⋅exp[−η2+162]⋅I0(4η)≈1√2π⋅exp[−(η−4)22],
- pII(η) = η⋅exp[−η22].
(3) Solution 2 is correct, as can already be seen from the graph. A calculation confirms this result:
- σ2Rice = σ2n=1,
- σ2Rayl = σ2n⋅(2−π/2)≈0.429.
(4) In general, the probability that y is greater than a certain value y0 is equal to
- Pr(y>y0)=∫∞y0ησ2n⋅exp[−η22σ2n]dη.
- With the substitution x2=η2/(2σ2n) can be written for this:
- Pr(y>y0)=2⋅∫∞y0/(√2⋅σn)x⋅e−x2dx=[e−x2]∞√2⋅σn=exp[−y202σ2n].
- Here the indefinite integral given in the front was used. In particular:
- Pr(y>σn) = e−0.5≈60.7%_,
- Pr(y>2σn) = e−2.0≈13.5%_,
- Pr(y>3σn) = e−4.5≈1.1%_.