Difference between revisions of "Aufgaben:Exercise 5.6: Error Correlation Duration"
| (13 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Burst_Error_Channels}} |
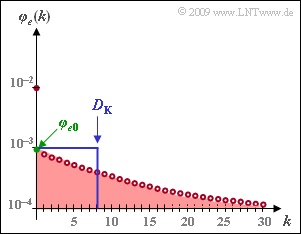
| − | [[File:P_ID1842__Dig_A_5_6.png|right|frame| | + | [[File:P_ID1842__Dig_A_5_6.png|right|frame|Error correlation function of the GE model]] |
| − | + | The graph shows the ''error correlation function'' (ECF) of the ''Gilbert–Elliott model'' with the parameters | |
:$$p_{\rm G} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.001, | :$$p_{\rm G} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0.001, | ||
\hspace{0.2cm}p_{\rm B} = 0.1,\hspace{0.2cm} | \hspace{0.2cm}p_{\rm B} = 0.1,\hspace{0.2cm} | ||
| Line 11: | Line 11: | ||
B\hspace{0.05cm}|\hspace{0.05cm} G) = 0.01\hspace{0.05cm}$$ | B\hspace{0.05cm}|\hspace{0.05cm} G) = 0.01\hspace{0.05cm}$$ | ||
| − | in | + | in logarithmic representation. |
| − | + | This model is discussed in detail in [[Aufgaben:Exercise_5.6Z:_Gilbert-Elliott_Model|"Exercise 5.6Z"]]. In particular, the error correlation function (ECF) is also calculated in this exercise. With the auxiliary quantities | |
:$$A \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (p_{\rm B}- p_{\rm M}) | :$$A \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (p_{\rm B}- p_{\rm M}) | ||
\cdot (p_{\rm M}- p_{\rm | \cdot (p_{\rm M}- p_{\rm | ||
| Line 21: | Line 21: | ||
G\hspace{0.05cm}|\hspace{0.05cm} B)$$ | G\hspace{0.05cm}|\hspace{0.05cm} B)$$ | ||
| − | + | it can be written for: | |
:$$\varphi_{e}(k) = | :$$\varphi_{e}(k) = | ||
\left\{ \begin{array}{c} p_{\rm M} \\ | \left\{ \begin{array}{c} p_{\rm M} \\ | ||
p_{\rm M}^2 + A \cdot (1-B)^k \end{array} \right.\quad | p_{\rm M}^2 + A \cdot (1-B)^k \end{array} \right.\quad | ||
| − | \begin{array}{*{1}c} f{\rm | + | \begin{array}{*{1}c} f{\rm or }\hspace{0.15cm}k = 0 \hspace{0.05cm}, |
| − | \\ f{\rm | + | \\ f{\rm or }\hspace{0.15cm} k > 0 \hspace{0.05cm}.\\ \end{array}$$ |
| − | + | This is a burst error channel. To quantitatively describe the statistical bonds, one often uses the correlation term according to the following definition: | |
:$$D_{\rm K} = \frac{1}{\varphi_{e0} - p_{\rm M}^2} \cdot \sum_{k = 1 | :$$D_{\rm K} = \frac{1}{\varphi_{e0} - p_{\rm M}^2} \cdot \sum_{k = 1 | ||
| − | }^{\infty}\hspace{0.1cm} [\varphi_{e}(k) - p_{\rm | + | }^{\infty}\hspace{0.1cm}\big [\varphi_{e}(k) - p_{\rm |
| − | M}^2]\hspace{0.05cm}.$$ | + | M}^2 \big]\hspace{0.05cm}.$$ |
| + | |||
| + | The reference value φe0 is obtained by <i>extrapolation</i> of the error correlation function to the point k=0. If, as here, the ECF curve is given analytically, φe0 can also be calculated by inserting the value k=0 into the equation which is actually only valid for k>0. | ||
| + | |||
| + | |||
| + | |||
| − | |||
| − | '' | + | ''Notes:'' |
| − | * | + | * The exercise belongs to the chapter [[Digital_Signal_Transmission/Burst_Error_Channels| "Burst Error Channels"]]. |
| − | * | + | *Reference is made in particular to the section [[Digital_Signal_Transmission/Burst_Error_Channels#Error_correlation_function_of_the_Gilbert-Elliott_model|"Error correlation function of the Gilbert-Elliott model"]]. |
| − | + | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which ECF value is exactly valid for k=0? |
|type="{}"} | |type="{}"} | ||
φe(k=0) = { 1 3% } ⋅10−2 | φe(k=0) = { 1 3% } ⋅10−2 | ||
| − | { | + | {What is the value extrapolated from the given ECF for k=0? |
|type="{}"} | |type="{}"} | ||
φe0 = { 0.091 3% } ⋅10−2 | φe0 = { 0.091 3% } ⋅10−2 | ||
| − | { | + | {What result is obtained for the error correlation duration DK with the quantities A and B defined in front? |
| − | |type=" | + | |type="()"} |
- DK=A⋅B, | - DK=A⋅B, | ||
- DK=1/A−B, | - DK=1/A−B, | ||
+ DK=1/B−1. | + DK=1/B−1. | ||
| − | { | + | {What is the correlation duration for the GE model at hand? |
|type="{}"} | |type="{}"} | ||
DK = { 8.091 3% } | DK = { 8.091 3% } | ||
| − | { | + | {Which statements are valid regarding the correlation duration DK of the GE model? Note the logarithmic ordinate for your answer. |
|type="[]"} | |type="[]"} | ||
| − | + DK | + | + DK remains the same if Pr(B|G) and Pr(G|B) are interchanged. |
| − | - DK | + | - DK depends only on the sum Pr(G|B)+Pr(B|G). |
| − | - | + | - The red area in the graph is equal to the blue rectangular area. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The ECF value φe(k=0) always indicates the mean error probability pM, while the ECF limit for k → ∞ is equal to p2M. |
| + | *From the graph on the information section, one can read pM =0.01_. | ||
| + | *In [[Aufgaben:Exercise_5.6Z:_Gilbert-Elliott_Model|"Exercise 5.6Z"]], this value is calculated in a different way. | ||
| − | '''(2)''' | + | |
| + | '''(2)''' If we insert the parameter $k = 0$ into the lower ECF equation, which is actually only valid for $k > 0$, we obtain the extrapolation value we are looking for. | ||
:$$\varphi_{e0} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} p_{\rm M}^2 + | :$$\varphi_{e0} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} p_{\rm M}^2 + | ||
(p_{\rm B}- p_{\rm M}) \cdot (p_{\rm M}- p_{\rm | (p_{\rm B}- p_{\rm M}) \cdot (p_{\rm M}- p_{\rm | ||
| Line 83: | Line 90: | ||
\hspace{0.15cm}\underline {\approx 0.091 \cdot 10^{-2}}\hspace{0.05cm}.$$ | \hspace{0.15cm}\underline {\approx 0.091 \cdot 10^{-2}}\hspace{0.05cm}.$$ | ||
| − | '''(3)''' | + | |
| + | '''(3)''' According to the general definition equation, the following holds for the error correlation period | ||
:$$D_{\rm K} = \frac{1}{\varphi_{e0} - p_{\rm M}^2} \cdot \sum_{k = 1 | :$$D_{\rm K} = \frac{1}{\varphi_{e0} - p_{\rm M}^2} \cdot \sum_{k = 1 | ||
}^{\infty}\hspace{0.1cm} [\varphi_{e}(k) - p_{\rm | }^{\infty}\hspace{0.1cm} [\varphi_{e}(k) - p_{\rm | ||
M}^2]\hspace{0.05cm}.$$ | M}^2]\hspace{0.05cm}.$$ | ||
| − | + | *With the expressions | |
:$$A \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (p_{\rm B}- p_{\rm M}) | :$$A \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (p_{\rm B}- p_{\rm M}) | ||
\cdot (p_{\rm M}- p_{\rm | \cdot (p_{\rm M}- p_{\rm | ||
| Line 96: | Line 104: | ||
G\hspace{0.05cm}|\hspace{0.05cm} B)$$ | G\hspace{0.05cm}|\hspace{0.05cm} B)$$ | ||
| − | + | :this equation can be written as follows: | |
:$$D_{\rm K} = {1}/{A} \cdot \sum_{k = 1 }^{\infty}\hspace{0.1cm} | :$$D_{\rm K} = {1}/{A} \cdot \sum_{k = 1 }^{\infty}\hspace{0.1cm} | ||
A \cdot (1 - B)^k = \sum_{k = 1 }^{\infty}\hspace{0.1cm} (1 - | A \cdot (1 - B)^k = \sum_{k = 1 }^{\infty}\hspace{0.1cm} (1 - | ||
B)^k\hspace{0.05cm}.$$ | B)^k\hspace{0.05cm}.$$ | ||
| − | + | *Using the summation formula of a geometric series, this gives the final result: | |
:$$D_{\rm K} = {1}/{B} - 1 = \frac{1}{{\rm Pr}(\rm | :$$D_{\rm K} = {1}/{B} - 1 = \frac{1}{{\rm Pr}(\rm | ||
B\hspace{0.05cm}|\hspace{0.05cm} G) + {\rm Pr}(\rm | B\hspace{0.05cm}|\hspace{0.05cm} G) + {\rm Pr}(\rm | ||
G\hspace{0.05cm}|\hspace{0.05cm} B)} - 1\hspace{0.05cm}.$$ | G\hspace{0.05cm}|\hspace{0.05cm} B)} - 1\hspace{0.05cm}.$$ | ||
| − | + | *So <u>solution 3</u> is correct. | |
| − | '''(4)''' | + | |
| + | '''(4)''' With Pr(B|G)=0.01 and Pr(G|B)=0.1 we get | ||
:DK=10.01+0.1−1≈8.091_. | :DK=10.01+0.1−1≈8.091_. | ||
| − | '''(5)''' | + | |
| − | * | + | '''(5)''' Only <u>solution 1</u> is correct, as shown in the sample solutions to the last subtasks: |
| − | * | + | *Thus the correlation term is fixed, for example: |
| − | * | + | *With Pr(B|G)=0.1 and Pr(G|B)=0.01 we get the same DK=8.091 as with Pr(B|G)=0.01 and Pr(G|B)=0.1. |
| − | * | + | *But now the mean error probability pM≈9.1% instead of 1%, respectively for pG=0.001 and pB=0.1. |
| + | *The last statement is also false. This statement would only be true if φe(k) was plotted linearly and not logarithmically as here. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^5.3 Burst Error Channels^]] |
Latest revision as of 14:22, 19 October 2022
The graph shows the error correlation function (ECF) of the Gilbert–Elliott model with the parameters
- pG = 0.001,pB=0.1,Pr(G|B) = 0.1,Pr(B|G)=0.01
in logarithmic representation.
This model is discussed in detail in "Exercise 5.6Z". In particular, the error correlation function (ECF) is also calculated in this exercise. With the auxiliary quantities
- A = (pB−pM)⋅(pM−pG),
- B = Pr(B|G)+Pr(G|B)
it can be written for:
- φe(k)={pMp2M+A⋅(1−B)kfork=0,fork>0.
This is a burst error channel. To quantitatively describe the statistical bonds, one often uses the correlation term according to the following definition:
- DK=1φe0−p2M⋅∞∑k=1[φe(k)−p2M].
The reference value φe0 is obtained by extrapolation of the error correlation function to the point k=0. If, as here, the ECF curve is given analytically, φe0 can also be calculated by inserting the value k=0 into the equation which is actually only valid for k>0.
Notes:
- The exercise belongs to the chapter "Burst Error Channels".
- Reference is made in particular to the section "Error correlation function of the Gilbert-Elliott model".
Questions
Solution
- From the graph on the information section, one can read pM =0.01_.
- In "Exercise 5.6Z", this value is calculated in a different way.
(2) If we insert the parameter k=0 into the lower ECF equation, which is actually only valid for k>0, we obtain the extrapolation value we are looking for.
- φe0 = p2M+(pB−pM)⋅(pM−pG)=10−4+(0.1−0.01)⋅(0.01−0.001)=10−4+0.09⋅0.009≈0.091⋅10−2_.
(3) According to the general definition equation, the following holds for the error correlation period
- DK=1φe0−p2M⋅∞∑k=1[φe(k)−p2M].
- With the expressions
- A = (pB−pM)⋅(pM−pG)=φe0−p2M,
- B = Pr(B|G)+Pr(G|B)
- this equation can be written as follows:
- DK=1/A⋅∞∑k=1A⋅(1−B)k=∞∑k=1(1−B)k.
- Using the summation formula of a geometric series, this gives the final result:
- DK=1/B−1=1Pr(B|G)+Pr(G|B)−1.
- So solution 3 is correct.
(4) With Pr(B|G)=0.01 and Pr(G|B)=0.1 we get
- DK=10.01+0.1−1≈8.091_.
(5) Only solution 1 is correct, as shown in the sample solutions to the last subtasks:
- Thus the correlation term is fixed, for example:
- With Pr(B|G)=0.1 and Pr(G|B)=0.01 we get the same DK=8.091 as with Pr(B|G)=0.01 and Pr(G|B)=0.1.
- But now the mean error probability pM≈9.1% instead of 1%, respectively for pG=0.001 and pB=0.1.
- The last statement is also false. This statement would only be true if φe(k) was plotted linearly and not logarithmically as here.