Difference between revisions of "Aufgaben:Exercise 2.2: Properties of Galois Fields"

Latest revision as of 14:11, 28 August 2022

Addition / multiplication for $q = 5$ and $q = 6$

Here we consider the sets of numbers

$Z_5 = \{0, \, 1, \, 2, \, 3, \, 4\} \ \Rightarrow \ q = 5$,

$Z_6 = \{0, \, 1, \, 2, \, 3, \, 4,\, 5\} \ \Rightarrow \ q = 6$.

In the adjacent graph, the (partially incomplete) addition and multiplication tables for $q = 5$ and $q = 6$ are given, where both addition ("$+$") and multiplication ("$\hspace{0.05cm}\cdot\hspace{0.05cm}$") modulo $q$ are to be understood.

To be checked is whether the number sets $Z_5$ and $Z_6$ satisfy all the conditions of a Galois field $\rm GF(5)$ and $\rm GF(6)$, respectively.

In the "theory section" a total of eight conditions are mentioned, all of which must be met. You are to check only two of these conditions:

$\rm(D)$ For all elements there is an additive inverse (Inverse for "$+$"):

$$\forall \hspace{0.15cm} z_i \in {\rm GF}(q),\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q)\text{:}\hspace{0.5cm}z_i + {\rm Inv_A}(z_i) = 0 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_A}(z_i) = -z_i \hspace{0.05cm}.$$

$\rm(E)$ All elements have a multiplicative inverse (Inverse for "$\hspace{0.05cm}\cdot\hspace{0.05cm}$"):

$$\forall \hspace{0.15cm} z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne 0, \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q)\text{:}\hspace{0.5cm}z_i \cdot {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = z_i^{-1}\hspace{0.05cm}.$$

The other conditions for a Galois field, viz.

Closure,
Existence of zero– and identity element,
validity of commutative law, associative law and distributive law

are satisfied by both, $Z_5$ and $Z_6$.

Hints: The exercise refers to the chapter "Some Basics of Algebra".

Questions

$A_{04} \ = \ $

$A_{14} \ = \ $

$A_{44} \ = \ $

$M_{04} \ = \ $

$M_{14} \ = \ $

$M_{44} \ = \ $

	Yes.
	No, there is not an additive inverse for all elements $(0, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 4)$ .
	No, the elements $1, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 4$ do not all have a multiplicative inverse.

	Yes.
	No, there is not an additive inverse for all elements $(0, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 5)$ .
	No, the elements $1, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 5$ do not all have a multiplicative inverse.

	$Z_{10} = \{0, \, 1, \, 2, \, 3, \, 4, \, 5, \, 6, \, 7, \, 8, \, 9\}$ is a Galois field?
	$Z_{11} = \{0, \, 1, \, 2, \, 3, \, 4, \,5, \, 6, \, 7, \, 8, \, 9, \, 10\}$ is a Galois field?
	$Z_{12} = \{0, \, 1, \, 2, \, 3, \, 4, \, 5, \, 6, \, 7, \, 8, \, 9, \, 10, \, 11\}$ is a Galois field?

Solution

(1) In general, for $0 ≤ \mu ≤ 4 \text{:} \hspace{0.2cm} A_{\mu 4} = (\mu + 4) \, {\rm mod} \, 5$. It follows:

$$A_{04} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (0+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 4}\hspace{0.05cm},\hspace{0.2cm}A_{14}=(1+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 0}\hspace{0.05cm},\hspace{0.2cm}A_{24}=(2+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1\hspace{0.05cm},$$

$$A_{34} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (3+4)\hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5= 2\hspace{0.05cm},\hspace{0.2cm}A_{44}=(4+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 3}\hspace{0.05cm}.$$

Due to the commutative law of addition,

$$z_i + z_j = z_j + z_i \hspace{0.5cm} {\rm for \hspace{0.2cm}all\hspace{0.2cm} } z_i, z_j \in Z_5\hspace{0.05cm},$$

the last column of the addition table is of course identical to the last row of the same table.

(2) Now $M_{\mu 4} = (\mu \cdot 4) \, {\rm mod} \, 5$ and we obtain:

$$M_{04} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (0\cdot4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 0}\hspace{0.05cm},\hspace{0.2cm}M_{14}=(1\cdot4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 4}\hspace{0.05cm},\hspace{0.2cm}M_{24}=(2\cdot4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 3\hspace{0.05cm},$$

$$M_{34} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (3\cdot4)\hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 2\hspace{0.05cm},\hspace{0.2cm}M_{44}=(4\cdot 4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 1}\hspace{0.05cm}.$$

Since multiplication is also commutative, the last column in the multiplication table again matches the last row.

Addition/multiplication tables for $q = 5$

(3) The graph shows the full addition and multiplication tables for $q = 5$. You can see:

In the addition table there is exactly one zero in each row (and also in each column).

So for every $z_i ∈ Z_5$ there is an ${\rm Inv}_{\rm A} (z_i)$ that satisfies the condition $[z_i + {\rm Inv}_{\rm A}(z_i)] \, {\rm mod} \, 5 = 0$:

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = 0 \hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-1) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 4 \hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 2\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 3 \hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 3\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-3) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 2 \hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 4\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm}.$$

In the multiplication table we leave the zero element (first row and first column) out of consideration.

In all other rows and columns of the lower table there is indeed exactly one each.

From the condition $[z_i \cdot {\rm Inv}_{\rm M}(z_i)] \, {\rm mod} \, 5 = 1$ one obtains:

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_i \cdot {\rm Inv_M}(z_i) = 1\hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 2 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 3 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_i \cdot {\rm Inv_M}(z_i) = 6 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 3 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 2 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_i \cdot {\rm Inv_M}(z_i) = 6 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 4 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 4 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_i \cdot {\rm Inv_M}(z_i) = 16 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm}.$$

Since both the required additive and multiplicative inverses exist ⇒ $Z_5$ describes a Galois field $\rm GF(5)$

Correct is the proposed solution 1.

(4) From the blue addition table on the statement page, we see that all numbers $(0, \, 1, \, 2, \, 3, \, 4, \, 5)$ of the set $Z_6$ have an additive inverse

⇒ in each row (and column) there is exactly one zero.

On the other hand, a multiplicative inverse ${\rm Inv}_{\rm M}(z_i)$ exists only for $z_i = 1$ and $z_i = 5$, viz.

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_i \cdot {\rm Inv_M}(z_i) = 1\hspace{0.05cm},$$

$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 5 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 5 \hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_i \cdot {\rm Inv_M}(z_i) = 25 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 6 = 1 \hspace{0.05cm}.$$

For $z_i = 2, \ z_i = 3$ and $z_i = 4$, we find no element $z_j$, so that $(z_i \cdot z_j) \, {\rm mod} \, 6 = 1$.

Correct is the proposed solution 3 ⇒ the blue tables for $q = 6$ do not yield a Galois field $\rm GF(6)$.

(5) Correct is the proposed solution 2:

A finite number set $Z_q = \{0, \, 1, \hspace{0.05cm} \text{...} \hspace{0.1cm} , \, q-1\}$ of natural numbers leads to a Galois field only if $q$ is a prime number.

Of the number sets mentioned above, this is true only for $Z_{11}$.

@@ Line 1: / Line 1: @@
-{{quiz-Header|Buchseite=Kanalcodierung/Einige Grundlagen der Algebra}}
+{{quiz-Header|Buchseite=Channel_Coding/Some_Basics_of_Algebra}}
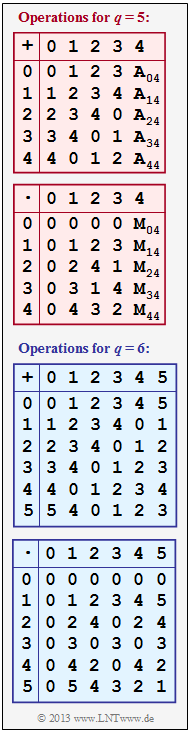
-[[File:P_ID2492__KC_A_2_2.png|right|frame|Additions– und Multiplikationstabellen für $q = 5$ und $q = 6$]]
+[[File:EN_KC_A_2_2.png|right|frame|Addition / multiplication for&nbsp; $q = 5$&nbsp; and&nbsp; $q = 6$]]
-Wir betrachten hier die Zahlenmengen
+Here we consider the sets of numbers
 * $Z_5 = \{0, \, 1, \, 2, \, 3, \, 4\} \ \Rightarrow \ q = 5$,
 * $Z_6 = \{0, \, 1, \, 2, \, 3, \, 4,\, 5\} \ \Rightarrow \ q = 6$.
-In nebenstehender Grafik sind die (teilweise unvollständigen) Additions&ndash; und Multiplikationstabellen für $q = 5$ und für $q = 6$ angegeben, wobei sowohl die Addition (&bdquo;$+$&rdquo;) als auch die Multiplikation (&bdquo;$\cdot$&rdquo;) modulo $q$ zu verstehen sind.
+In the adjacent graph,&nbsp;  the (partially incomplete)&nbsp; addition and multiplication tables for&nbsp; $q = 5$&nbsp; and&nbsp; $q = 6$&nbsp; are given,&nbsp; where both addition&nbsp; ("$+$")&nbsp; and multiplication&nbsp; ("$\hspace{0.05cm}\cdot\hspace{0.05cm}$")&nbsp; modulo&nbsp; $q$&nbsp; are to be understood.
-Zu überprüfen ist, ob die Zahlenmengen $Z_5$ und $Z_6$ alle Bedingungen eines Galoisfeldes $\rm GF(5)$ bzw. $\rm GF(6)$ erfüllen. Im [[Kanalcodierung/Einige_Grundlagen_der_Algebra#Definition_eines_Galoisfeldes|Theorieteil]] werden insgesamt acht Bedingungen genannt, die alle erfüllt sein müssen. Von Ihnen überprüft werden sollen nur zwei dieser Bedingungen:
+To be checked is whether the number sets&nbsp; $Z_5$&nbsp; and&nbsp; $Z_6$&nbsp; satisfy all the conditions of a Galois field&nbsp; $\rm GF(5)$&nbsp; and&nbsp; $\rm GF(6)$,&nbsp; respectively.
-(D) Für alle Elemente gibt es eine <span style="color: rgb(204, 0, 0);"><b>additive Inverse</b></span> (<i>Inverse for &bdquo;$+$&rdquo;</i>):
+In the&nbsp; [[Channel_Coding/Some_Basics_of_Algebra#Definition_of_a_Galois_field|"theory section"]]&nbsp; a total of eight conditions are mentioned,&nbsp; all of which must be met.&nbsp; You are to check only two of these conditions:
-:$$\forall \hspace{0.15cm}  z_i \in {\rm GF}(q),\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q):$$
-:$$\hspace{0.25cm}z_i + {\rm Inv_A}(z_i) = 0  \hspace{0.25cm} \Rightarrow \hspace{0.25cm}
+$\rm(D)$&nbsp;  For all elements there is an&nbsp; <b>additive inverse</b>&nbsp; (Inverse&nbsp; for&nbsp; "$+$"):
+:$$\forall \hspace{0.15cm}  z_i \in {\rm GF}(q),\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q)\text{:}\hspace{0.5cm}z_i + {\rm Inv_A}(z_i) = 0  \hspace{0.25cm} \Rightarrow \hspace{0.25cm}
 {\rm Inv_A}(z_i) = -z_i \hspace{0.05cm}.$$
-(E) Alle Elemente haben eine <span style="color: rgb(204, 0, 0);"><b>multiplikative Inverse</b></span> (<i>Inverse for &bdquo;$\cdot$&rdquo;</i>):
+$\rm(E)$&nbsp;  All elements have a&nbsp; <b>multiplicative inverse</b>&nbsp; (Inverse&nbsp; for&nbsp; "$\hspace{0.05cm}\cdot\hspace{0.05cm}$"):
-:$$\forall \hspace{0.15cm}  z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne 0, \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q):$$
+:$$\forall \hspace{0.15cm}  z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne 0, \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q)\text{:}\hspace{0.5cm}z_i \cdot {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}
-:$$\hspace{0.25cm}z_i \cdot {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}
 {\rm Inv_M}(z_i) = z_i^{-1}\hspace{0.05cm}.$$
-Die weiteren Bedingungen für ein Galoisfeld, nämlich
+The other conditions for a Galois field, viz.
 * Closure,
-* Existenz von Null&ndash; und Einselelement,
+* Existence of zero&ndash; and identity element,
-* Gültigkeit von Kommutativ&ndash;, Assoziativ&ndash; und Distributivgesetz
+* validity of commutative law, associative law and distributive law
+are satisfied by both,&nbsp; $Z_5$&nbsp; and&nbsp; $Z_6$.
-werden sowohl von $Z_5$ als auch von $Z_6$ erfüllt.
-''Hinweis:''
+Hints:&nbsp; The exercise refers to the chapter&nbsp; [[Channel_Coding/Some_Basics_of_Algebra| "Some Basics of Algebra"]].
-* Die Aufgabe bezieht sich auf das Kapitel [[Kanalcodierung/Einige_Grundlagen_der_Algebra| Einige Grundlagen der Algebra]].
-* Sollte die Eingabe des Zahlenwertes &bdquo;0&rdquo; erforderlich sein, so geben Sie bitte &bdquo;0.&rdquo; ein.
-===Fragebogen===
+===Questions===
 <quiz display=simple>
-{Ergänzen Sie die Additionstabelle für $q = 5$. Geben Sie folgende Werte ein:
+{Complete the addition table for&nbsp; $q = 5$.&nbsp; Enter the following values:
 |type="{}"}
-$A_{04} \ = \ ${ 4 3% }
+$A_{04} \ = \ ${ 4 }
-$A_{14} \ = \ ${ 0 3% }
+$A_{14} \ = \ ${ 0. }
-$A_{44} \ = \ ${ 3 3% }
+$A_{44} \ = \ ${ 3 }
-{Ergänzen Sie die Multiplikationstabelle für $q = 5$. Geben Sie folgende Werte ein:
+{Complete the multiplication table for&nbsp; $q = 5$.&nbsp; Enter the following values:
 |type="{}"}
-$M_{04} \ = \ ${ 0 3% }
+$M_{04} \ = \ ${ 0. }
-$M_{14} \ = \ ${ 4 3% }
+$M_{14} \ = \ ${ 4. }
-$M_{44} \ = \ ${ 1 3% }
+$M_{44} \ = \ ${ 1. }
-{Erfüllt die Menge $Z_5$ die Bedingungen eines Galoisfeldes?
+{Does the&nbsp; $Z_5$&nbsp; set satisfy the conditions of a Galois field?
 |type="[]"}
-+ Ja.
++ Yes.
-- Nein, es gibt nicht für alle Elemente $(0 \ - \ 4)$ eine additive Inverse.
+- No,&nbsp; there is not an additive inverse for all elements&nbsp; $(0, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 4)$&nbsp;.
-- Nein, die Elemente $1-4$ haben nicht alle eine multiplikative Inverse.
+- No,&nbsp; the elements&nbsp; $1, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 4$&nbsp; do not all have a multiplicative inverse.
-{Erfüllt die Menge $Z_6$ die Bedingungen eines Galoisfeldes?
+{Does the&nbsp; $Z_6$&nbsp; set satisfy the conditions of a Galois field?
 |type="[]"}
-- Ja.
+- Yes.
-- Nein, es gibt nicht für alle Elemente $(0 \ - \ 5)$ eine additive Inverse.
+- No,&nbsp; there is not an additive inverse for all elements&nbsp; $(0, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 5)$&nbsp;.
-+ Nein, die Elemente $1-5$ haben nicht alle eine multiplikative Inverse.
++ No,&nbsp; the elements&nbsp; $1, \hspace{0.05cm}\text{...} \hspace{0.1cm}, 5$&nbsp; do not all have a multiplicative inverse.
-{Die Zahlenmengen $Z_2, \ Z_3, \ Z_5$ und $Z_7$ ergeben ein Galoisfeld, die Mengen $Z_4, \ Z_6, \ Z_8, \ Z_9$ dagegen nicht. Was folgern Sie daraus?
+{The number sets&nbsp; $Z_2, \ Z_3, \ Z_5$&nbsp; and $Z_7$&nbsp; yield a Galois field,&nbsp; but the sets&nbsp; $Z_4, \ Z_6, \ Z_8, \ Z_9$&nbsp; do not.&nbsp; What do you conclude from this?
 |type="[]"}
-- $Z_{10} = \{0, \, 1, \, 2, \, 3, \, 4, \, 5, \, 6, \, 7, \, 8, \, 9\}$ ist ein Galoisfeld?
+- $Z_{10} = \{0, \, 1, \, 2, \, 3, \, 4, \, 5, \, 6, \, 7, \, 8, \, 9\}$ is a Galois field?
-+ $Z_{11} = \{0, \, 1, \, 2, \, 3, \, 4, \,5, \, 6, \, 7, \, 8, \, 9, \, 10\}$ ist ein Galoisfeld?
++ $Z_{11} = \{0, \, 1, \, 2, \, 3, \, 4, \,5, \, 6, \, 7, \, 8, \, 9, \, 10\}$ is a Galois field?
-- $Z_{12} = \{0, \, 1, \, 2, \, 3, \, 4, \, 5, \, 6, \, 7, \, 8, \, 9, \, 10, \, 11\}$ ist ein Galoisfeld?
+- $Z_{12} = \{0, \, 1, \, 2, \, 3, \, 4, \, 5, \, 6, \, 7, \, 8, \, 9, \, 10, \, 11\}$ is a Galois field?
 </quiz>
-===Musterlösung===
+===Solution===
 {{ML-Kopf}}
-'''(1)'''&nbsp;
+'''(1)'''&nbsp;  In general,&nbsp; for&nbsp; $0 &#8804; \mu &#8804; 4 \text{:} \hspace{0.2cm} A_{\mu 4} = (\mu + 4) \, {\rm mod} \, 5$.&nbsp; It follows:
+:$$A_{04} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (0+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 4}\hspace{0.05cm},\hspace{0.2cm}A_{14}=(1+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 0}\hspace{0.05cm},\hspace{0.2cm}A_{24}=(2+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1\hspace{0.05cm},$$
+:$$A_{34} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (3+4)\hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5= 2\hspace{0.05cm},\hspace{0.2cm}A_{44}=(4+4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 3}\hspace{0.05cm}.$$
+Due to the commutative law of addition,
+:$$z_i + z_j = z_j + z_i \hspace{0.5cm} {\rm for  \hspace{0.2cm}all\hspace{0.2cm} } z_i, z_j \in Z_5\hspace{0.05cm},$$
+the last column of the addition table is of course identical to the last row of the same table.
+'''(2)'''&nbsp; Now $M_{\mu 4} = (\mu \cdot 4) \, {\rm mod} \, 5$&nbsp; and we obtain:
+:$$M_{04} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (0\cdot4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 0}\hspace{0.05cm},\hspace{0.2cm}M_{14}=(1\cdot4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 4}\hspace{0.05cm},\hspace{0.2cm}M_{24}=(2\cdot4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 3\hspace{0.05cm},$$
+:$$M_{34} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (3\cdot4)\hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 2\hspace{0.05cm},\hspace{0.2cm}M_{44}=(4\cdot 4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 \hspace{0.15cm}\underline{= 1}\hspace{0.05cm}.$$
+Since multiplication is also commutative,&nbsp; the last column in the multiplication table again matches the last row.
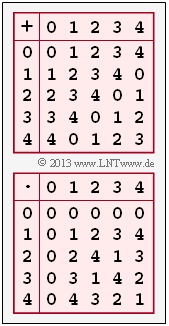
+[[File:P_ID2493__KC_A_2_2c.png|right|frame|Addition/multiplication tables for&nbsp; $q = 5$]]
+'''(3)'''&nbsp; The graph shows the full addition and multiplication tables for&nbsp; $q = 5$.&nbsp; You can see:
+* In the addition table there is exactly one zero in each row&nbsp; (and also in each column).&nbsp;
+*So for every&nbsp; $z_i &#8712; Z_5$&nbsp; there is an&nbsp; ${\rm Inv}_{\rm A} (z_i)$&nbsp; that satisfies the condition&nbsp; $[z_i + {\rm Inv}_{\rm A}(z_i)] \, {\rm mod} \, 5 = 0$:
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 0\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = 0  \hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-1) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 4 \hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 2\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 3 \hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 3\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-3) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 2 \hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 4\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm Inv_A}(z_i) = (-4) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm}.$$
+* In the multiplication table we leave the zero element&nbsp; (first row and first column)&nbsp; out of consideration.
+*In all other rows and columns of the lower table there is indeed exactly one each.&nbsp;
+*From the condition $[z_i \cdot {\rm Inv}_{\rm M}(z_i)] \, {\rm mod} \, 5 = 1$&nbsp; one obtains:
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1  \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}  z_i \cdot {\rm Inv_M}(z_i) =  1\hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 2  \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 3 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}  z_i \cdot {\rm Inv_M}(z_i) =  6 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 3  \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 2 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}  z_i \cdot {\rm Inv_M}(z_i) =  6 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 4  \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 4 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}  z_i \cdot {\rm Inv_M}(z_i) =  16 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 5 = 1 \hspace{0.05cm}.$$
+*Since both the required additive and multiplicative inverses exist &nbsp; &rArr; &nbsp; $Z_5$ describes a Galois field $\rm GF(5)$ &nbsp;
+*Correct is the <u>proposed solution 1</u>.
+'''(4)'''&nbsp; From the blue addition table on the statement page,&nbsp; we see that all numbers&nbsp; $(0, \, 1, \, 2, \, 3, \, 4, \, 5)$&nbsp; of the set $Z_6$&nbsp; have an additive inverse
-'''(2)'''&nbsp;
+&nbsp; &#8658; &nbsp; in each row&nbsp; (and column)&nbsp; there is exactly one zero.
+*On the other hand,&nbsp; a multiplicative inverse&nbsp; ${\rm Inv}_{\rm M}(z_i)$&nbsp; exists only for&nbsp; $z_i = 1$&nbsp; and&nbsp; $z_i = 5$,&nbsp; viz.
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 1  \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}  z_i \cdot {\rm Inv_M}(z_i) =  1\hspace{0.05cm},$$
+:$$z_i \hspace{-0.1cm} \ = \ \hspace{-0.1cm} 5  \hspace{0.25cm} \Rightarrow \hspace{0.25cm} {\rm Inv_M}(z_i) = 5 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}  z_i \cdot {\rm Inv_M}(z_i) =  25 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 6 = 1 \hspace{0.05cm}.$$
-'''(3)'''&nbsp;
+*For&nbsp; $z_i = 2, \ z_i = 3$ and $z_i = 4$,&nbsp; we find no element&nbsp; $z_j$,&nbsp; so that&nbsp; $(z_i \cdot z_j) \, {\rm mod} \, 6 = 1$.
+*Correct is the <u>proposed solution 3</u> &nbsp; &rArr; &nbsp; the blue tables for&nbsp; $q = 6$&nbsp; do not yield a Galois field&nbsp; $\rm GF(6)$.
-'''(4)'''&nbsp;
-'''(5)'''&nbsp;
+'''(5)'''&nbsp; Correct is the&nbsp; <u>proposed solution 2</u>:
+*A finite number set&nbsp; $Z_q = \{0, \, 1, \hspace{0.05cm} \text{...} \hspace{0.1cm} , \, q-1\}$&nbsp; of natural numbers leads to a Galois field only if&nbsp; $q$&nbsp; is a prime number.
+*Of the number sets mentioned above,&nbsp; this is true only for&nbsp; $Z_{11}$.
 {{ML-Fuß}}
-[[Category:Aufgaben zu  Kanalcodierung|^2.1 Einige Grundlagen der Algebra^]]
+[[Category:Channel Coding: Exercises|^2.1 Some Basics of Algebra^]]