Difference between revisions of "Aufgaben:Exercise 1.16: Block Error Probability Bounds for AWGN"
| (42 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Limits_for_Block_Error_Probability}} |
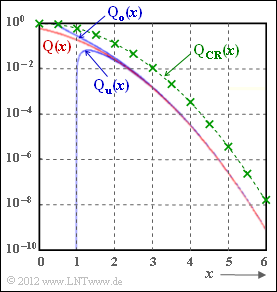
| − | [[File:P_ID2414__KC_A_1_15.png|right|frame| | + | [[File:P_ID2414__KC_A_1_15.png|right|frame|Function ${\rm Q}(x)$ and approximations;<br>it holds: ${\rm Q_u}(x)\le{\rm Q}(x)\le{\rm Q_o}(x)$ ]] |
| − | + | We assume the following constellation: | |
| − | * | + | *A linear block code with code rate $R = k/n$ and distance spectrum $\{W_i\}, \ i = 1, \ \text{...} \ , n$, |
| − | |||
| − | |||
| + | *an AWGN channel characterized by $E_{\rm B}/N_{0}$ ⇒ convertible to noise power $\sigma^2$, | ||
| − | + | *a receiver based on "soft decision" as well as the "maximum likelihood criterion". | |
| + | |||
| + | |||
| + | Under the assumption valid for the entire exercise that always the zero-word $\underline{x}_{1} = (0, 0, \text{... } \ , 0)$ is sent, the [[Channel_Coding/Limits_for_Block_Error_Probability#Union_Bound_of_the_block_error_probability|"pairwise error probability"]] with a different code word $\underline{x}_{l} (l = 2,\ \text{...} \ , 2^k)$: | ||
:$$ {\rm Pr}[\hspace{0.05cm}\underline{x}_{\hspace{0.02cm}1} \hspace{-0.02cm}\mapsto \hspace{-0.02cm}\underline{x}_{\hspace{0.02cm}l}\hspace{0.05cm}] = {\rm Q}\left ( \sqrt{w_{\rm H}(\underline{x}_{\hspace{0.02cm}l})/\sigma^2} \right ) \hspace{0.05cm}.$$ | :$$ {\rm Pr}[\hspace{0.05cm}\underline{x}_{\hspace{0.02cm}1} \hspace{-0.02cm}\mapsto \hspace{-0.02cm}\underline{x}_{\hspace{0.02cm}l}\hspace{0.05cm}] = {\rm Q}\left ( \sqrt{w_{\rm H}(\underline{x}_{\hspace{0.02cm}l})/\sigma^2} \right ) \hspace{0.05cm}.$$ | ||
| − | + | The derivation of this relation can be found in [Liv10]. Used in this equation are: | |
| − | * | + | *the [[Theory_of_Stochastic_Signals/Gaussian_Distributed_Random_Variables#Exceedance_probability|"complementary Gaussian error function"]] ${\rm Q}(x)$, |
| − | * | + | |
| − | * | + | *the [[Channel_Coding/Objective_of_Channel_Coding#Important_definitions_for_block_coding|"Hamming weight"]] $w_{\rm H}(\underline{x}_{l})$ of the code word $\underline{x}_{l}$, |
| + | |||
| + | *the [[Digital_Signal_Transmission/Optimization_of_Baseband_Transmission_Systems#System_optimization_with_power_limitation|"AWGN noise power"]] $\sigma^2 = (2 \cdot R \cdot E_{\rm B}/N_{0})^{-1}.$ | ||
| − | + | This allows various bounds to be specified for the block error probability: | |
| − | * | + | *the so called [[Channel_Coding/Limits_for_Block_Error_Probability#Union_Bound_of_the_block_error_probability|"Union Bound"]] $\rm (UB)$: |
| − | :$$p_1 = \sum_{l = 2}^{2^k}\hspace{0.05cm}{\rm Pr}[\hspace{0.05cm}\underline{x}_{\hspace{0.02cm}1} \hspace{-0.02cm}\mapsto \hspace{-0.02cm}\underline{x}_{\hspace{0.02cm}l}\hspace{0.05cm}] = \sum_{l = 2}^{2^k}\hspace{0.05cm}{\rm Q}\left ( \sqrt{w_{\rm H}(\underline{x}_{\hspace{0.02cm}l})/\sigma^2} \right ) \hspace{0.05cm},$$ | + | :$$p_1 = \sum_{l = 2}^{2^k}\hspace{0.05cm}{\rm Pr}[\hspace{0.05cm}\underline{x}_{\hspace{0.02cm}1} \hspace{-0.02cm}\mapsto \hspace{-0.02cm}\underline{x}_{\hspace{0.02cm}l}\hspace{0.05cm}] = \sum_{l \hspace{0.05cm}= \hspace{0.05cm}2}^{2^k}\hspace{0.05cm}{\rm Q}\left ( \sqrt{w_{\rm H}(\underline{x}_{\hspace{0.02cm}l})/\sigma^2} \right ) \hspace{0.05cm},$$ |
| − | * | + | *the so called [[Channel_Coding/Limits_for_Block_Error_Probability#Bounds_for_the_.287.2C_4.2C_3.29_Hamming_code_at_the_AWGN_channel|"Truncated Union Bound"]] $\rm (TUB)$: |
:$$p_2 = W_{d_{\rm min}} \cdot {\rm Q}\left ( \sqrt{d_{\rm min}/\sigma^2} \right ) \hspace{0.05cm},$$ | :$$p_2 = W_{d_{\rm min}} \cdot {\rm Q}\left ( \sqrt{d_{\rm min}/\sigma^2} \right ) \hspace{0.05cm},$$ | ||
| − | * | + | *the [[Channel_Coding/Limits_for_Block_Error_Probability#The_upper_bound_according_to_Bhattacharyya|"Bhattacharyya Bound"]]: |
| − | :$$p_3 = W(\beta) - 1\hspace{0.05cm},\hspace{0.2cm} {\rm | + | :$$p_3 = W(\beta) - 1\hspace{0.05cm},\hspace{0.2cm} {\rm with}\hspace{0.15cm} \beta = {\rm e}^{ - 1/(2\sigma^2) } \hspace{0.05cm}.$$ |
| − | In | + | :In this case, replace the distance spectrum $\{W_i\}$ with the weight enumerator function: |
:$$\left \{ \hspace{0.05cm} W_i \hspace{0.05cm} \right \} \hspace{0.3cm} \Leftrightarrow \hspace{0.3cm} W(X) = \sum_{i=0 }^{n} W_i \cdot X^{i} = W_0 + W_1 \cdot X + W_2 \cdot X^{2} + ... \hspace{0.05cm} + W_n \cdot X^{n}\hspace{0.05cm}.$$ | :$$\left \{ \hspace{0.05cm} W_i \hspace{0.05cm} \right \} \hspace{0.3cm} \Leftrightarrow \hspace{0.3cm} W(X) = \sum_{i=0 }^{n} W_i \cdot X^{i} = W_0 + W_1 \cdot X + W_2 \cdot X^{2} + ... \hspace{0.05cm} + W_n \cdot X^{n}\hspace{0.05cm}.$$ | ||
| − | + | In the transition from the "Union Bound" $p_{1}$ to the more imprecise bound $p_{3}$ among others | |
| + | *the function ${\rm Q}(x)$ is replaced by the [https://en.wikipedia.org/wiki/Chernoff_bound "Chernoff-Rubin bound"] ${\rm Q}_{\rm CR}(x)$. | ||
| + | |||
| + | *Both functions are shown in the above graph (red and green curve, resp.). | ||
| + | |||
| + | |||
| + | In the [[Aufgaben:Exercise_1.16Z:_Bounds_for_the_Gaussian_Error_Function|"Exercise 1.16Z"]] the relationship between these functions is evaluated numerically and referenced to the bounds ${\rm Q}_{\rm o}(x)$ and ${\rm Q}_{\rm u}(x)$ which are also drawn in the above graph. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | Hints: | ||
| + | * This exercise belongs to the chapter [[Channel_Coding/Bounds_for_Block_Error_Probability|"Bounds for block error probability"]]. | ||
| + | * The above cited reference "[Liv10]" refers to the lecture manuscript "Liva, G.: Channel Coding. Chair of Communications Engineering, TU Munich and DLR Oberpfaffenhofen, 2010." | ||
| − | === | + | * Further we refer to the interactive HTML5/JavaScript applet [[Applets:Komplementäre_Gaußsche_Fehlerfunktionen| "Complementary Gaussian error functions"]]. |
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which equation applies to the "Union Bound"? |
|type="[]"} | |type="[]"} | ||
| − | - $p_{1} = \sum_{l=2}^{2^k} W_{l} · {\rm Q}[(l/\sigma^2)^{0.5}],$ | + | - $p_{1} = \sum_{l\hspace{0.05cm}=\hspace{0.05cm}2}^{2^k} W_{l} · {\rm Q}\big[(l/\sigma^2)^{0.5}\big],$ |
| − | + $p_{1} = \sum_{i=1}^{n} W_{i} · {\rm Q}[(i/\sigma^2)^{0.5}] | + | + $p_{1} = \sum_{i\hspace{0.05cm}=\hspace{0.05cm}1}^{n} W_{i} · {\rm Q}\big[(i/\sigma^2)^{0.5}\big].$ |
| − | { | + | {Specify the Union Bound for the $(8, 4, 4)$ code and various $\sigma$. |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $\sigma = 1.0 \text{:} \hspace{0.4cm} p_{1} \ = \ $ { 32.15 3% } $\ \%$ |
| − | $\sigma = 0.5 \text{:} \hspace{0. | + | $\sigma = 0.5 \text{:} \hspace{0.4cm} p_{1} \ = \ $ { 0.0444 3% } $\ \%$ |
| − | { | + | {Given the same boundary conditions, what does the "Truncated Union Bound" provide? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $\sigma = 1.0 \text{:} \hspace{0.4cm} p_{2} \ = \ $ { 31.92 3% } $\ \%$ |
| − | $\sigma = 0.5 \text{:} \hspace{0. | + | $\sigma = 0.5 \text{:} \hspace{0.4cm} p_{2} \ = \ $ { 0.044 3% } $\ \%$ |
| − | { | + | {Which statement is always true (for all constellations)? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The block error probability is never greater than $p_{1}$. |
| − | - | + | - The block error probability is never greater than $p_{2}$. |
| − | { | + | {How do you get from $p_{1}$ to the "Bhattacharyya Bound" $p_{3}$? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + Replace the error function ${\rm Q}(x)$ with the function ${\rm Q}_{\rm CR}(x)$. |
| − | - | + | - Set the Bhattacharyya parameter $\beta = 1/\sigma$. |
| − | + | + | + Instead of $\{W_i\}$ uses the weight enumerator function $W(X)$. |
| − | { | + | {Specify the Bhattacharyya Bound for $\sigma = 1$ and $\sigma = 0.5$ . |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $\sigma = 1.0 \text{:} \hspace{0.4cm} p_{3} \ = \ $ { 191.3 3% } $\ \%$ |
| − | $ | + | $\sigma = 0.5 \text{:} \hspace{0.4cm} p_{3} \ = \ $ { 0.47 3% } $\ \%$ |
| + | </quiz> | ||
| + | ===Solution=== | ||
| + | {{ML-Kopf}} | ||
| + | '''(1)''' The correct solution is <u>suggestion 2</u>: | ||
| + | *The distance spectrum $\{W_i\}$ is defined for $i = 0, \ \text{...} \ , \ n$: | ||
| + | |||
| + | #$W_{1}$ indicates how often the Hamming weight $w_{\rm H}(\underline{x}_{i}) = 1$ occurs. | ||
| + | #$W_{n}$ indicates how often the Hamming weight $w_{\rm H}(\underline{x}_{i}) = n$ occurs. | ||
| − | |||
| − | + | *With that, the "Union Bound" is: | |
| − | |||
| − | |||
| − | + | :$$p_1 = {\rm Pr(Union \hspace{0.15cm}Bound)}= \sum_{i = 1}^{n}\hspace{0.05cm}W_i \cdot {\rm Q}\left ( \sqrt{i/\sigma^2} \right ) \hspace{0.05cm}.$$ | |
| − | + | ||
| − | + | '''(2)''' The distance spectrum of the $(8, 4, 4)$ code was given as $W_{0} = 1 , \ W_{4} = 14, \ W_{8} = 1$. | |
| + | *Thus, one obtains for $\sigma = 1$: | ||
| + | :$$p_1 = W_4 \cdot {\rm Q}\left ( 2 \right ) + W_8 \cdot {\rm Q}\left ( 2 \cdot \sqrt{2} \right ) | ||
| + | = 14 \cdot 2.28 \cdot 10^{-2}+ 1 \cdot 0.23 \cdot 10^{-2} \hspace{0.15cm}\underline{\approx 32.15\%}\hspace{0.05cm},$$ | ||
| − | :$$p_1 = {\rm | + | *For $\sigma = 0.5$: |
| + | :$$p_1 = 14 \cdot {\rm Q}\left ( 4 \right ) + {\rm Q}\left ( 4 \cdot \sqrt{2} \right ) | ||
| + | = 14 \cdot 3.17 \cdot 10^{-5}+ 1.1 \cdot 10^{-8} \hspace{0.15cm}\underline{\approx 0.0444 \%}\hspace{0.05cm}.$$ | ||
| − | '''( | + | '''(3)''' With the minimum distance $d_{\rm min} = 4$ we get: |
| − | + | :$$\sigma = 1.0\text{:} \hspace{0.4cm} p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} W_4 \cdot {\rm Q}\left ( 2 \right ) \hspace{0.15cm}\underline{= 31.92\%}\hspace{0.05cm},$$ | |
| + | :$$\sigma = 0.5\text{:} \hspace{0.4cm} p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm}W_4 \cdot {\rm Q}\left ( 4 \right ) \approx p_1 \hspace{0.15cm}\underline{ = 0.0444 \%}\hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | '''(4)''' The correct solution is <u>suggestion 1</u>: | ||
| + | *The "Union Bound" - denoted here by $p_{1}$ - is an upper bound on the block error probability in all cases. | ||
| − | + | *For the bound $p_{2}$ ("Truncated Union Bound") this is not always true. | |
| − | + | *For example, in the $(7, 4, 3)$ Hamming code ⇒ $W_{3} = W_{4} = 7, \ W_{7} = 1$ is obtained with standard deviation $\sigma = 1$: | |
| − | |||
| − | |||
| − | |||
:$$p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} 7 \cdot {\rm Q}\left ( \sqrt{3} \right ) = 7 \cdot 4.18 \cdot 10^{-2} \approx 0.293\hspace{0.05cm},$$ | :$$p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} 7 \cdot {\rm Q}\left ( \sqrt{3} \right ) = 7 \cdot 4.18 \cdot 10^{-2} \approx 0.293\hspace{0.05cm},$$ | ||
:$$p_1 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} p_2 + 7 \cdot {\rm Q}\left ( \sqrt{4} \right )+ 1 \cdot {\rm Q}\left ( \sqrt{7} \right ) \approx 0.455 \hspace{0.05cm}.$$ | :$$p_1 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} p_2 + 7 \cdot {\rm Q}\left ( \sqrt{4} \right )+ 1 \cdot {\rm Q}\left ( \sqrt{7} \right ) \approx 0.455 \hspace{0.05cm}.$$ | ||
| − | + | *The actual block error probability is likely to be between $p_{2} = 29.3\%$ and $p_{1} = 45.5\%$ (but this has not been verified). <br>That is, $p_{2}$ is not an upper bound. | |
| − | '''(5)''' | + | '''(5)''' Correct are <u>suggested solutions 1 and 3</u>, as the following calculation for the $(8, 4, 4)$ code shows: |
| − | * | + | *It holds ${\rm Q}(x) ≤ {\rm Q_{CR}}(x) = {\rm e}^{-x^2/2}$. Thus, for the Union Bound |
:$$p_1 = W_4 \cdot {\rm Q}\left ( \sqrt{4/\sigma^2} \right ) +W_8 \cdot {\rm Q}\left ( \sqrt{8/\sigma^2} \right )$$ | :$$p_1 = W_4 \cdot {\rm Q}\left ( \sqrt{4/\sigma^2} \right ) +W_8 \cdot {\rm Q}\left ( \sqrt{8/\sigma^2} \right )$$ | ||
| − | + | :another upper bound can be specified: | |
| − | :$$p_1 \le W_4 \cdot {\rm | + | :$$p_1 \le W_4 \cdot {\rm e}^{ - {4}/(2 \sigma^2) } +W_8 \cdot {\rm e}^{ - {8}/(2 \sigma^2) } \hspace{0.05cm}.$$ |
| − | * | + | *With $\beta = {\rm e}^{-1/(2\sigma^2)}$ can be written for this also (so the given $\beta = 1/\sigma$ is wrong): |
:$$p_1 \le W_4 \cdot \beta^4 + W_8 \cdot \beta^8 \hspace{0.05cm}.$$ | :$$p_1 \le W_4 \cdot \beta^4 + W_8 \cdot \beta^8 \hspace{0.05cm}.$$ | ||
| − | * | + | *The weight function of the $(8, 4, 4)$ code is: |
| − | :$$W(X) = 1 + W_4 \cdot X^4 + W_8 \cdot X^8 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} W(\beta) - 1 = W_4 \cdot \beta^4 + W_8 \cdot \beta^8$$ | + | :$$W(X) = 1 + W_4 \cdot X^4 + W_8 \cdot X^8 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} W(\beta) - 1 = W_4 \cdot \beta^4 + W_8 \cdot \beta^8\hspace{0.3cm} |
| + | \Rightarrow \hspace{0.3cm} p_3 = W(\beta) - 1 \ge p_1\hspace{0.05cm}.$$ | ||
| − | |||
| − | '''(6)''' | + | '''(6)''' With $\sigma = 1$, the Bhattacharyya parameter is $\beta = {\rm e}^{-0.5} = 0.6065$, and thus one obtains for the Bhattacharyya Bound: |
| − | :$$p_3 = 14 \cdot \beta^4 + \beta^8 = 14 \cdot 0.135 + 0.018 \hspace{0.15cm}\underline{= | + | :$$p_3 = 14 \cdot \beta^4 + \beta^8 = 14 \cdot 0.135 + 0.018= 1.913 \hspace{0.15cm}\underline{= 191.3%}\hspace{0.05cm}.$$ |
| + | |||
| + | *Considering that $p_{3}$ is a bound for a probability, $p_{3} = 1.913$ is only a trivial bound. | ||
| − | + | *For $\sigma = 0.5$, on the other hand, $\beta = {\rm e}^{-2} \approx 0.135.$ Then holds: | |
| − | :$$p_3 = 14 \cdot \beta^4 + \beta^8 = 14 \cdot 3.35 \cdot 10^{-4} + 1.1 \cdot 10^{-7} \hspace{0.15cm}\underline{= | + | :$$p_3 = 14 \cdot \beta^4 + \beta^8 = 14 \cdot 3.35 \cdot 10^{-4} + 1.1 \cdot 10^{-7} \hspace{0.15cm}\underline{= 0.47 \%}\hspace{0.05cm}.$$ |
| − | + | A comparison with subtask '''(2)''' shows that in the present example the Bhattacharyya Bound $p_{3}$ is above the "Union Bound" $p_{1}$ by a factor | |
| + | :$$(0.47 - 10^{-2})/(0.044 - 10^{-2}) > 10.$$ | ||
| + | |||
| + | *The reason for this large deviation is the Chernoff-Rubin bound, which is well above the ${\rm Q}$ function. | ||
| + | |||
| + | *In [[Aufgaben:Exercise_1.16Z:_Bounds_for_the_Gaussian_Error_Function|"Exercise 1.16Z"]], the deviation between ${\rm Q}_{\rm CR}$ and ${\rm Q}(x)$ is also calculated quantitatively: | ||
:$${{\rm Q_{CR}}( x )}/{{\rm Q}( x )} \approx 2.5 \cdot x \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {{\rm Q_{CR}}( x = 4 )}/{{\rm Q}( x = 4)} \approx 10 \hspace{0.05cm}.$$ | :$${{\rm Q_{CR}}( x )}/{{\rm Q}( x )} \approx 2.5 \cdot x \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {{\rm Q_{CR}}( x = 4 )}/{{\rm Q}( x = 4)} \approx 10 \hspace{0.05cm}.$$ | ||
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Channel Coding: Exercises|^1.6 Error Probability Bounds^]] |
| − | |||
| − | |||
| − | ^]] | ||
Latest revision as of 17:17, 5 August 2022
We assume the following constellation:
- A linear block code with code rate $R = k/n$ and distance spectrum $\{W_i\}, \ i = 1, \ \text{...} \ , n$,
- an AWGN channel characterized by $E_{\rm B}/N_{0}$ ⇒ convertible to noise power $\sigma^2$,
- a receiver based on "soft decision" as well as the "maximum likelihood criterion".
Under the assumption valid for the entire exercise that always the zero-word $\underline{x}_{1} = (0, 0, \text{... } \ , 0)$ is sent, the "pairwise error probability" with a different code word $\underline{x}_{l} (l = 2,\ \text{...} \ , 2^k)$:
- $$ {\rm Pr}[\hspace{0.05cm}\underline{x}_{\hspace{0.02cm}1} \hspace{-0.02cm}\mapsto \hspace{-0.02cm}\underline{x}_{\hspace{0.02cm}l}\hspace{0.05cm}] = {\rm Q}\left ( \sqrt{w_{\rm H}(\underline{x}_{\hspace{0.02cm}l})/\sigma^2} \right ) \hspace{0.05cm}.$$
The derivation of this relation can be found in [Liv10]. Used in this equation are:
- the "complementary Gaussian error function" ${\rm Q}(x)$,
- the "Hamming weight" $w_{\rm H}(\underline{x}_{l})$ of the code word $\underline{x}_{l}$,
- the "AWGN noise power" $\sigma^2 = (2 \cdot R \cdot E_{\rm B}/N_{0})^{-1}.$
This allows various bounds to be specified for the block error probability:
- the so called "Union Bound" $\rm (UB)$:
- $$p_1 = \sum_{l = 2}^{2^k}\hspace{0.05cm}{\rm Pr}[\hspace{0.05cm}\underline{x}_{\hspace{0.02cm}1} \hspace{-0.02cm}\mapsto \hspace{-0.02cm}\underline{x}_{\hspace{0.02cm}l}\hspace{0.05cm}] = \sum_{l \hspace{0.05cm}= \hspace{0.05cm}2}^{2^k}\hspace{0.05cm}{\rm Q}\left ( \sqrt{w_{\rm H}(\underline{x}_{\hspace{0.02cm}l})/\sigma^2} \right ) \hspace{0.05cm},$$
- the so called "Truncated Union Bound" $\rm (TUB)$:
- $$p_2 = W_{d_{\rm min}} \cdot {\rm Q}\left ( \sqrt{d_{\rm min}/\sigma^2} \right ) \hspace{0.05cm},$$
- $$p_3 = W(\beta) - 1\hspace{0.05cm},\hspace{0.2cm} {\rm with}\hspace{0.15cm} \beta = {\rm e}^{ - 1/(2\sigma^2) } \hspace{0.05cm}.$$
- In this case, replace the distance spectrum $\{W_i\}$ with the weight enumerator function:
- $$\left \{ \hspace{0.05cm} W_i \hspace{0.05cm} \right \} \hspace{0.3cm} \Leftrightarrow \hspace{0.3cm} W(X) = \sum_{i=0 }^{n} W_i \cdot X^{i} = W_0 + W_1 \cdot X + W_2 \cdot X^{2} + ... \hspace{0.05cm} + W_n \cdot X^{n}\hspace{0.05cm}.$$
In the transition from the "Union Bound" $p_{1}$ to the more imprecise bound $p_{3}$ among others
- the function ${\rm Q}(x)$ is replaced by the "Chernoff-Rubin bound" ${\rm Q}_{\rm CR}(x)$.
- Both functions are shown in the above graph (red and green curve, resp.).
In the "Exercise 1.16Z" the relationship between these functions is evaluated numerically and referenced to the bounds ${\rm Q}_{\rm o}(x)$ and ${\rm Q}_{\rm u}(x)$ which are also drawn in the above graph.
Hints:
- This exercise belongs to the chapter "Bounds for block error probability".
- The above cited reference "[Liv10]" refers to the lecture manuscript "Liva, G.: Channel Coding. Chair of Communications Engineering, TU Munich and DLR Oberpfaffenhofen, 2010."
- Further we refer to the interactive HTML5/JavaScript applet "Complementary Gaussian error functions".
Questions
Solution
- The distance spectrum $\{W_i\}$ is defined for $i = 0, \ \text{...} \ , \ n$:
- $W_{1}$ indicates how often the Hamming weight $w_{\rm H}(\underline{x}_{i}) = 1$ occurs.
- $W_{n}$ indicates how often the Hamming weight $w_{\rm H}(\underline{x}_{i}) = n$ occurs.
- With that, the "Union Bound" is:
- $$p_1 = {\rm Pr(Union \hspace{0.15cm}Bound)}= \sum_{i = 1}^{n}\hspace{0.05cm}W_i \cdot {\rm Q}\left ( \sqrt{i/\sigma^2} \right ) \hspace{0.05cm}.$$
(2) The distance spectrum of the $(8, 4, 4)$ code was given as $W_{0} = 1 , \ W_{4} = 14, \ W_{8} = 1$.
- Thus, one obtains for $\sigma = 1$:
- $$p_1 = W_4 \cdot {\rm Q}\left ( 2 \right ) + W_8 \cdot {\rm Q}\left ( 2 \cdot \sqrt{2} \right ) = 14 \cdot 2.28 \cdot 10^{-2}+ 1 \cdot 0.23 \cdot 10^{-2} \hspace{0.15cm}\underline{\approx 32.15\%}\hspace{0.05cm},$$
- For $\sigma = 0.5$:
- $$p_1 = 14 \cdot {\rm Q}\left ( 4 \right ) + {\rm Q}\left ( 4 \cdot \sqrt{2} \right ) = 14 \cdot 3.17 \cdot 10^{-5}+ 1.1 \cdot 10^{-8} \hspace{0.15cm}\underline{\approx 0.0444 \%}\hspace{0.05cm}.$$
(3) With the minimum distance $d_{\rm min} = 4$ we get:
- $$\sigma = 1.0\text{:} \hspace{0.4cm} p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} W_4 \cdot {\rm Q}\left ( 2 \right ) \hspace{0.15cm}\underline{= 31.92\%}\hspace{0.05cm},$$
- $$\sigma = 0.5\text{:} \hspace{0.4cm} p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm}W_4 \cdot {\rm Q}\left ( 4 \right ) \approx p_1 \hspace{0.15cm}\underline{ = 0.0444 \%}\hspace{0.05cm}.$$
(4) The correct solution is suggestion 1:
- The "Union Bound" - denoted here by $p_{1}$ - is an upper bound on the block error probability in all cases.
- For the bound $p_{2}$ ("Truncated Union Bound") this is not always true.
- For example, in the $(7, 4, 3)$ Hamming code ⇒ $W_{3} = W_{4} = 7, \ W_{7} = 1$ is obtained with standard deviation $\sigma = 1$:
- $$p_2 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} 7 \cdot {\rm Q}\left ( \sqrt{3} \right ) = 7 \cdot 4.18 \cdot 10^{-2} \approx 0.293\hspace{0.05cm},$$
- $$p_1 \hspace{-0.15cm}\ = \ \hspace{-0.15cm} p_2 + 7 \cdot {\rm Q}\left ( \sqrt{4} \right )+ 1 \cdot {\rm Q}\left ( \sqrt{7} \right ) \approx 0.455 \hspace{0.05cm}.$$
- The actual block error probability is likely to be between $p_{2} = 29.3\%$ and $p_{1} = 45.5\%$ (but this has not been verified).
That is, $p_{2}$ is not an upper bound.
(5) Correct are suggested solutions 1 and 3, as the following calculation for the $(8, 4, 4)$ code shows:
- It holds ${\rm Q}(x) ≤ {\rm Q_{CR}}(x) = {\rm e}^{-x^2/2}$. Thus, for the Union Bound
- $$p_1 = W_4 \cdot {\rm Q}\left ( \sqrt{4/\sigma^2} \right ) +W_8 \cdot {\rm Q}\left ( \sqrt{8/\sigma^2} \right )$$
- another upper bound can be specified:
- $$p_1 \le W_4 \cdot {\rm e}^{ - {4}/(2 \sigma^2) } +W_8 \cdot {\rm e}^{ - {8}/(2 \sigma^2) } \hspace{0.05cm}.$$
- With $\beta = {\rm e}^{-1/(2\sigma^2)}$ can be written for this also (so the given $\beta = 1/\sigma$ is wrong):
- $$p_1 \le W_4 \cdot \beta^4 + W_8 \cdot \beta^8 \hspace{0.05cm}.$$
- The weight function of the $(8, 4, 4)$ code is:
- $$W(X) = 1 + W_4 \cdot X^4 + W_8 \cdot X^8 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} W(\beta) - 1 = W_4 \cdot \beta^4 + W_8 \cdot \beta^8\hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_3 = W(\beta) - 1 \ge p_1\hspace{0.05cm}.$$
(6) With $\sigma = 1$, the Bhattacharyya parameter is $\beta = {\rm e}^{-0.5} = 0.6065$, and thus one obtains for the Bhattacharyya Bound:
- $$p_3 = 14 \cdot \beta^4 + \beta^8 = 14 \cdot 0.135 + 0.018= 1.913 \hspace{0.15cm}\underline{= 191.3%}\hspace{0.05cm}.$$
- Considering that $p_{3}$ is a bound for a probability, $p_{3} = 1.913$ is only a trivial bound.
- For $\sigma = 0.5$, on the other hand, $\beta = {\rm e}^{-2} \approx 0.135.$ Then holds:
- $$p_3 = 14 \cdot \beta^4 + \beta^8 = 14 \cdot 3.35 \cdot 10^{-4} + 1.1 \cdot 10^{-7} \hspace{0.15cm}\underline{= 0.47 \%}\hspace{0.05cm}.$$
A comparison with subtask (2) shows that in the present example the Bhattacharyya Bound $p_{3}$ is above the "Union Bound" $p_{1}$ by a factor
- $$(0.47 - 10^{-2})/(0.044 - 10^{-2}) > 10.$$
- The reason for this large deviation is the Chernoff-Rubin bound, which is well above the ${\rm Q}$ function.
- In "Exercise 1.16Z", the deviation between ${\rm Q}_{\rm CR}$ and ${\rm Q}(x)$ is also calculated quantitatively:
- $${{\rm Q_{CR}}( x )}/{{\rm Q}( x )} \approx 2.5 \cdot x \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {{\rm Q_{CR}}( x = 4 )}/{{\rm Q}( x = 4)} \approx 10 \hspace{0.05cm}.$$