Difference between revisions of "Aufgaben:Exercise 2.16: Bounded Distance Decoding: Decision Regions"
From LNTwww
| (20 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Error_Probability_and_Areas_of_Application}} |
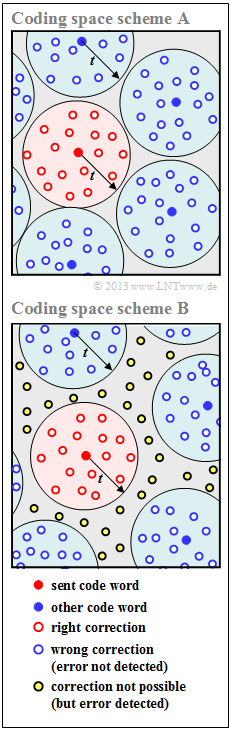
| + | [[File:EN_KC_A_2_16.png|right|frame|Two coding space schemes]] | ||
| + | We assume a block code of length $n$ with symbols $c_i ∈ {\rm GF}(2^m)$ that can correct up to $t$ symbols. | ||
| + | *Each possible received word $\underline{y}_i$ can then be viewed as a point in a high-dimensional space. | ||
| − | + | *Assuming the basis ${\rm GF}(2) = \{0, \, 1\}$ the dimension is $n \cdot m$. | |
| − | |||
| − | |||
| − | |||
| − | + | The diagram shows such spaces in schematic two-dimensional representation. The illustration is to be interpreted as follows: | |
| + | # The red dot $\underline{c}_j$ was sent. All red outlined points $\underline{y}_i$ in a hypersphere around this point $\underline{c}_j$ with the parameter $t$ as radius can be corrected. Using the nomenclature according to the [[Channel_Coding/Error_Probability_and_Areas_of_Application#Block_error_probability_for_RSC_and_BDD|$\rm graph$]] in the theory section, then $\underline{z}_i = \underline{c}_j$ <br>⇒ "Error correction is successful". | ||
| + | # For very many symbol errors, $\underline{c}_j$ may be falsified into a blue $($or white-blue$)$ dot $\underline{y}_j$ belonging to the hypersphere of another code word $\underline{c}_{k ≠ j}$. In this case the decoder makes a wrong decision <br>⇒ "The received word $\underline{y}_j$ is decoded incorrectly". | ||
| + | # Finally, as in the sketch below, there may be yellow dots that do not belong to any hypersphere <br>⇒ "The received word $\underline{y}_j$ is not decodable". | ||
| − | |||
| + | In this exercise you are to decide which of the two code space schemes is suitable for describing | ||
| + | * [[Channel_Coding/Examples_of_Binary_Block_Codes#Hamming_Codes|"Bounded Distance Decoding of Hamming codes"]], resp. | ||
| − | + | * [[Channel_Coding/Error_Probability_and_Areas_of_Application#Block_error_probability_for_RSC_and_BDD|"Bounded Distance Decoding by Reed–Solomon codes"]]. | |
| − | * [[ | ||
| − | |||
| − | |||
| − | |||
| − | === | + | <u>Hints:</u> |
| + | * The exercise complements the topic of the chapter [[Channel_Coding/Error_Probability_and_Areas_of_Application|"Error Probability and Application Areas"]]. | ||
| + | |||
| + | * It is intended to illustrate significant differences in decoding Reed–Solomon codes and Hamming codes. | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which coding space scheme applies to the Hamming codes? |
|type="()"} | |type="()"} | ||
| − | + | + | + Coding space scheme $\rm A$, |
| − | - | + | - Coding space scheme $\rm B$. |
| − | { | + | {Which statement is true for the probability that a received word $\underline{y}$ cannot be decoded with Hamming coding? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The probability ${\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} not \hspace{0.15cm} decodable)$ is exactly zero. |
| − | - ${\rm Pr}(\underline{y} \rm \ | + | - ${\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} not \hspace{0.15cm} decodable)$ is nonzero, but negligible. |
| − | - | + | - It holds ${\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} not \hspace{0.15cm} decodable) > {\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} incorrectly \hspace{0.15cm} decoded)$. |
| − | { | + | {Which coding space scheme applies to the Reed–Solomon codes? |
|type="()"} | |type="()"} | ||
| − | - | + | - Coding space scheme $\rm A$, |
| − | + | + | + Coding space scheme $\rm B$. |
| − | { | + | {Which statement applies to the probability that a received word $\underline{y}$ cannot be decoded after Reed–Solomon coding? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The probability ${\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} not \hspace{0.15cm} decodable)$ is exactly zero. |
| − | - ${\rm Pr}(\underline{y} \rm \ | + | - ${\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} not \hspace{0.15cm} decodable)$ is nonzero, but negligible. |
| − | + | + | + It holds ${\rm Pr}(\underline{y} {\rm \ is \ not \ decodable}) > {\rm Pr}(\underline{y} \rm \hspace{0.15cm} is \hspace{0.15cm} incorrectly \hspace{0.15cm} decoded)$. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct is <u>solution 1</u>, since the coding space scheme $\rm A$ describes a perfect code and each Hamming code $(n, \, k, \, 3)$ is a perfect code: |
| − | + | *For any Hamming code $(n, \, k, \, 3)$, there are a total of $2^n$ possible received words $\underline{y}_i$, which are assigned to one of $2^k$ possible code words $\underline{c}_j$ during syndrome decoding. | |
| − | + | ||
| + | *Because of the Hamming code property $d_{\rm min} = 3$, all spheres in the $n$–dimensional space have radius $t = 1$. Thus in all spheres there are $2^{n-k}$ points. | ||
| + | :* $\text{HC (7, 4, 3)}$: One point for error-free transmission and seven points for one bit error ⇒ $1 + 7 = 8 = 2^3 = 2^{7-4}$. | ||
| + | :* $\text{HC (15, 11, 3)}$: One point for error-free transmission and now fifteen points for one bit error ⇒ $1 + 15 = 16 = 2^4 = 2^{15-11}$. | ||
| − | + | <u>Note:</u> Since the Hamming code is a binary code, here the code space has the dimension $n$. | |
| − | |||
| + | '''(2)''' Correct is <u>answer 1</u>: | ||
| + | *In the gray area outside "spheres" there is not a single point in a perfect code. | ||
| − | '''( | + | *This was also shown in the calculation for subtask '''(1)'''. |
| − | + | ||
| − | :$${\rm Pr(\underline{\it y}_{\it i} \hspace{0. | + | |
| − | = {\rm Pr}(f \le t) | + | '''(3)''' The Reed–Solomon codes are described by the coding space scheme $\rm B$ ⇒ <u>Answer 2</u>. |
| − | + | *Here there are numerous yellow points in the gray area, i.e. points that cannot be assigned to any sphere in "Bounded Distance Decoding". | |
| + | |||
| + | *For example, if we consider the $\rm RSC \, (7, \, 3, \, 5)_8$ with code parameters $n = 7, \, k = 3$, and $t = 2$, there are a total of $8^7 = 2097152$ points and $8^3 = 512$ hyperspheres here. | ||
| + | |||
| + | *If this code were perfect, then there should be $8^4 = 4096$ points within each sphere. However, it holds: | ||
| + | :$${\rm Pr}(\underline{\it y}_{\it i} {\rm \hspace{0.1cm}lies\hspace{0.1cm} within\hspace{0.1cm} the\hspace{0.1cm} red\hspace{0.1cm} sphere)} | ||
| + | = {\rm Pr}(f \le t) = {\rm Pr}(f = 0)+ {\rm Pr}(f = 1)+{\rm Pr}(f = 2) =1 + {7 \choose 1} \cdot 7 + {7 \choose 2} \cdot 7^2 = 1079 | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | *For ${\rm Pr}(f = 1)$ it is considered that there can be "$7 \rm \ over \ 1$" $= 7$ error positions, and for each error position also seven different error values. The same is considered for ${\rm Pr}(f = 2)$. | |
| − | '''(4)''' | + | |
| − | :$${\rm Pr(\underline{ | + | '''(4)''' Correct is <u>answer 3</u>: |
| + | *A point in gray no-man's land is reached with fewer symbol errors than a point in another hypersphere. | ||
| + | |||
| + | *For long codes, an upper bound on the error probability is given in the literature: | ||
| + | |||
| + | :$${\rm Pr}(\underline{y} {\rm \hspace{0.15cm} is \hspace{0.15cm} incorrectly \hspace{0.15cm} decoded}) | ||
= {\rm Pr}(\underline{z} \ne \underline{c}) \le \frac{1}{t\hspace{0.05cm}!} | = {\rm Pr}(\underline{z} \ne \underline{c}) \le \frac{1}{t\hspace{0.05cm}!} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | *For the ${\rm RSC} \, (225, \, 223, \, 33)_{256} \ \Rightarrow \ t = 16$ ⇒ this upper bound yields the value $1/(16!) < 10^{-14}$. | |
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Channel Coding: Exercises|^2.6 Block Error Probability of RS Codes^]] |
Latest revision as of 17:31, 23 January 2023
We assume a block code of length $n$ with symbols $c_i ∈ {\rm GF}(2^m)$ that can correct up to $t$ symbols.
- Each possible received word $\underline{y}_i$ can then be viewed as a point in a high-dimensional space.
- Assuming the basis ${\rm GF}(2) = \{0, \, 1\}$ the dimension is $n \cdot m$.
The diagram shows such spaces in schematic two-dimensional representation. The illustration is to be interpreted as follows:
- The red dot $\underline{c}_j$ was sent. All red outlined points $\underline{y}_i$ in a hypersphere around this point $\underline{c}_j$ with the parameter $t$ as radius can be corrected. Using the nomenclature according to the $\rm graph$ in the theory section, then $\underline{z}_i = \underline{c}_j$
⇒ "Error correction is successful". - For very many symbol errors, $\underline{c}_j$ may be falsified into a blue $($or white-blue$)$ dot $\underline{y}_j$ belonging to the hypersphere of another code word $\underline{c}_{k ≠ j}$. In this case the decoder makes a wrong decision
⇒ "The received word $\underline{y}_j$ is decoded incorrectly". - Finally, as in the sketch below, there may be yellow dots that do not belong to any hypersphere
⇒ "The received word $\underline{y}_j$ is not decodable".
In this exercise you are to decide which of the two code space schemes is suitable for describing
Hints:
- The exercise complements the topic of the chapter "Error Probability and Application Areas".
- It is intended to illustrate significant differences in decoding Reed–Solomon codes and Hamming codes.
Questions
Solution
(1) Correct is solution 1, since the coding space scheme $\rm A$ describes a perfect code and each Hamming code $(n, \, k, \, 3)$ is a perfect code:
- For any Hamming code $(n, \, k, \, 3)$, there are a total of $2^n$ possible received words $\underline{y}_i$, which are assigned to one of $2^k$ possible code words $\underline{c}_j$ during syndrome decoding.
- Because of the Hamming code property $d_{\rm min} = 3$, all spheres in the $n$–dimensional space have radius $t = 1$. Thus in all spheres there are $2^{n-k}$ points.
- $\text{HC (7, 4, 3)}$: One point for error-free transmission and seven points for one bit error ⇒ $1 + 7 = 8 = 2^3 = 2^{7-4}$.
- $\text{HC (15, 11, 3)}$: One point for error-free transmission and now fifteen points for one bit error ⇒ $1 + 15 = 16 = 2^4 = 2^{15-11}$.
Note: Since the Hamming code is a binary code, here the code space has the dimension $n$.
(2) Correct is answer 1:
- In the gray area outside "spheres" there is not a single point in a perfect code.
- This was also shown in the calculation for subtask (1).
(3) The Reed–Solomon codes are described by the coding space scheme $\rm B$ ⇒ Answer 2.
- Here there are numerous yellow points in the gray area, i.e. points that cannot be assigned to any sphere in "Bounded Distance Decoding".
- For example, if we consider the $\rm RSC \, (7, \, 3, \, 5)_8$ with code parameters $n = 7, \, k = 3$, and $t = 2$, there are a total of $8^7 = 2097152$ points and $8^3 = 512$ hyperspheres here.
- If this code were perfect, then there should be $8^4 = 4096$ points within each sphere. However, it holds:
- $${\rm Pr}(\underline{\it y}_{\it i} {\rm \hspace{0.1cm}lies\hspace{0.1cm} within\hspace{0.1cm} the\hspace{0.1cm} red\hspace{0.1cm} sphere)} = {\rm Pr}(f \le t) = {\rm Pr}(f = 0)+ {\rm Pr}(f = 1)+{\rm Pr}(f = 2) =1 + {7 \choose 1} \cdot 7 + {7 \choose 2} \cdot 7^2 = 1079 \hspace{0.05cm}.$$
- For ${\rm Pr}(f = 1)$ it is considered that there can be "$7 \rm \ over \ 1$" $= 7$ error positions, and for each error position also seven different error values. The same is considered for ${\rm Pr}(f = 2)$.
(4) Correct is answer 3:
- A point in gray no-man's land is reached with fewer symbol errors than a point in another hypersphere.
- For long codes, an upper bound on the error probability is given in the literature:
- $${\rm Pr}(\underline{y} {\rm \hspace{0.15cm} is \hspace{0.15cm} incorrectly \hspace{0.15cm} decoded}) = {\rm Pr}(\underline{z} \ne \underline{c}) \le \frac{1}{t\hspace{0.05cm}!} \hspace{0.05cm}.$$
- For the ${\rm RSC} \, (225, \, 223, \, 33)_{256} \ \Rightarrow \ t = 16$ ⇒ this upper bound yields the value $1/(16!) < 10^{-14}$.