Difference between revisions of "Aufgaben:Exercise 4.1Z: Appointment to Breakfast"

Latest revision as of 16:56, 22 February 2022

Chancellor candidates – breakfast in 2002

Ms. M. and Mr. S. are known to meet often for a joint breakfast:

Both promise to come to such a meeting on a certain day between 8 am and 9 am.
Further, they agree that each of them will arrive in this period (and only in this period) on "good luck"
and wait up to fifteen minutes for the other.

Hints:

The exercise belongs to the chapter Two-Dimensional Random Variables.
Use the minute of arrival time as the time in the following questions:
"Minute = 0" stands for 8 o'clock, "Minute = 60" for 9 o'clock.
The exercise arose before the 2002 German Bundestag elections, when both Dr. Angela Merkel and Dr. Edmund Stoiber wanted to become the CDU/CSU's candidate for chancellor.
At a joint breakfast in Wolfratshausen, Ms. Merkel renounced. The later election was won by Gerhard Schröder (SPD).

Questions

$p_1 \ = \ $

$\ \%$

$p_2 \ = \ $

$\ \%$

$\rm minute \ = \ $

$p_4 \ = \ $

$\ \%$

Solution

(1) If Mr. S. arrives at 8:30, he will meet Ms. M. if she arrives between 8:15 and 8:45. Thus the probability:

$$p_1 = \text{Pr(Mr. S. meets Ms. M.)}\hspace{0.15cm}\underline{=50\%}.$$

"Favorable area" for meeting

(2) If Ms. M. arrives at 8 a.m., she meets Mr. S. only if he arrives before 8:15.

If Ms. M. arrives at 9 a.m., Mr. S. must arrive after 8:45 a.m. so that they can meet.
The probability of meeting is the same in both cases:

$$p_2 = \big[\text{Min Pr(Mr. S. meets Ms. M.)}\big]\hspace{0.15cm}\underline{=25\%}.$$

(3) Of the two arrival times calculated in (2), 9 o'clock $(\underline{\text{Minute = 60}})$ is more favorable,
since she – if Mr. S. is not there – can leave immediately.

(4) The probability $p_4$ is given as the ratio of the red area in the graph to the total area $1$.

Using the triangular areas, one obtains:

$$p_4=\rm 1-2\cdot\frac{1}{2}\cdot\frac{3}{4}\cdot\frac{3}{4}=\frac{7}{16}\hspace{0.15cm}\underline{=\rm 43.75\%}.$$

@@ Line 1: / Line 1: @@
-{{quiz-Header|Buchseite=Stochastische Signaltheorie/Zweidimensionale Zufallsgrößen
+{{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Two-Dimensional_Random_Variables
 }}
-[[File:P_ID245__Sto_Z_4_1.jpg|right|Dr. Angela Merkel und Dr. Edmund Stoiber im Jahr 2002]]
+[[File:P_ID245__Sto_Z_4_1.jpg|right|frame|Chancellor candidates &ndash; breakfast in 2002]]
-Frau M. und Herr S. treffen sich ja bekanntlich &ouml;fter einmal zu einem gemeinsamen Fr&uuml;hst&uuml;ck. Beide versprechen, an einem bestimmten Tag zwischen 8 Uhr und 9 Uhr zu einem solchen Treffen zu kommen. Weiter vereinbaren sie, dass jeder von ihnen in diesem Zeitraum (und nur in diesem) auf &bdquo;Gut Gl&uuml;ck&rdquo; eintrifft und bis zu einer Viertelstunde auf den Anderen wartet.
+Ms. M. and Mr. S. are known to meet often for a joint breakfast:
+*Both promise to come to such a meeting on a certain day between 8 am and 9 am.
+*Further,&nbsp; they agree that each of them will arrive in this period&nbsp; (and only in this period)&nbsp; on&nbsp; "good luck"&nbsp; <br>and wait up to fifteen minutes for the other.
-''Hinweise:''
-*Die Aufgabe gehört zum  Kapitel [[Stochastische_Signaltheorie/Zweidimensionale_Zufallsgrößen|Zweidimensionale Zufallsgrößen]].
-*Sollte die Eingabe des Zahlenwertes &bdquo;0&rdquo; erforderlich sein, so geben Sie bitte &bdquo;0.&rdquo; ein.
-*Verwenden Sie bei den nachfolgenden Fragen als Zeitangabe die Minute der Ankunftszeit: &bdquo;Minute = 0&rdquo; steht f&uuml;r 8 Uhr, &bdquo;Minute = 60&rdquo; f&uuml;r 9 Uhr.
-*Die Aufgabe entstand vor der Bundestagswahl 2002, als sowohl Angela Merkel als auch Edmund Stoiber Kanzlerkandidat(in) der CDU/CSU werden wollten. Bei einem gemeinsamen Frühstück in Wolfratshausen verzichtete Frau Merkel. Die spätere Wahl gewann Gerhard Schröder.
-===Fragebogen===
+Hints:
+*The exercise belongs to the chapter&nbsp; [[Theory_of_Stochastic_Signals/Two-Dimensional_Random_Variables|Two-Dimensional Random Variables]].
+*Use the minute of arrival time as the time in the following questions:&nbsp; <br>"Minute = 0"&nbsp; stands for 8 o'clock, "Minute = 60"&nbsp; for 9 o'clock.
+*The exercise arose before the 2002 German Bundestag elections,&nbsp; when both Dr. Angela Merkel and Dr. Edmund Stoiber wanted to become the CDU/CSU's candidate for chancellor.
+*At a joint breakfast in Wolfratshausen,&nbsp; Ms. Merkel renounced.&nbsp; The later election was won by Gerhard Schröder&nbsp; (SPD).
+===Questions===
 <quiz display=simple>
-{Wie gro&szlig; ist die Wahrscheinlichkeit $p_1$, dass sich die beiden treffen, wenn Herr S. um 8 Uhr 30 ankommt? Begr&uuml;nden Sie Ihre Antwort.
+{What is the probability&nbsp; $p_1$&nbsp; that the two will meet when Mr. S. arrives at 8:30?&nbsp; Give reasons for your answer.
 |type="{}"}
-$p_1 \ = $ { 50 1% } $\ \%$
+$p_1 \ = \ $ { 50 1% } $\ \%$
-{Welche Ankunftszeit sollte Frau M. w&auml;hlen, wenn sie Herrn S. eigentlich nicht treffen m&ouml;chte, sich aber trotzdem an die getroffene Vereinbarung halten will? Wie gro&szlig; ist dann die Wahrscheinlichkeit $p_2$, dass sich Frau M. und Herr S. treffen werden?
+{Which arrival time should Ms. M. choose if she does not actually want to meet Mr. S.,&nbsp; but still wants to keep to the agreement made? <br>What is the probability&nbsp; $p_2$&nbsp; that Ms. M. and Mr. S. will meet?
 |type="{}"}
-$p_2 \ = $ { 25 1% } $\ \%$
+$p_2 \ = \ $ { 25 1% } $\ \%$
-{Welche Ankunftszeit sollte Frau M. w&auml;hlen, wenn sie nicht nur ein Treffen m&ouml;glichst vermeiden, sondern die Wartezeit minimieren m&ouml;chte?
+{Which arrival time should Ms. M. choose if she not only wants to avoid a meeting as much as possible,&nbsp; but also wants to minimize the waiting time?
 |type="{}"}
-$\rm Minute$ = { 60  }
+$\rm minute \ = \ ${ 60 }
-{Wie gro&szlig; ist die Wahrscheinlichkeit $p_4$ f&uuml;r ein Zusammentreffen generell, das heißt, wenn beide tats&auml;chlich auf &bdquo;Gut Gl&uuml;ck&rdquo; erscheinen?
+{What is the probability&nbsp; $p_4$&nbsp; for a meeting in general,&nbsp; that is,&nbsp; if both actually appear on "good luck"?
 |type="{}"}
-$p_4 \ = $ { 43.75 1% } $\ \%$
+$p_4 \ = \ $ { 43.75 1% } $\ \%$
@@ Line 40: / Line 46: @@
 </quiz>
-===Musterlösung===
+===Solution===
 {{ML-Kopf}}
-'''(1)'''&nbsp; Kommt Herr S. um 8 Uhr 30, so trifft er Frau M., wenn diese zwischen 8 Uhr 15 und 8 Uhr 45 ankommt. Damit ist die Wahrscheinlichkeit
+'''(1)'''&nbsp; If Mr. S. arrives at 8:30,&nbsp; he will meet Ms. M. if she arrives between 8:15 and 8:45.&nbsp; Thus the probability:
-$$p_1 = \text{Pr(Herr S. trifft Frau M.)}\hspace{0.15cm}\underline{=50\%}.$$
+:$$p_1 = \text{Pr(Mr. S. meets Ms. M.)}\hspace{0.15cm}\underline{=50\%}.$$
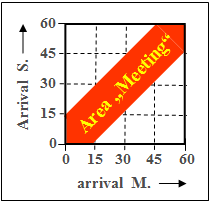
+[[File:EN_Sto_Z_4_1_d_neu.png|right|frame|"Favorable area"&nbsp; for meeting]]
+'''(2)'''&nbsp; If Ms. M. arrives at 8 a.m.,&nbsp; she meets Mr. S. only if he arrives before 8:15.
+*If Ms. M. arrives at 9 a.m.,&nbsp; Mr. S. must arrive after 8:45 a.m. so that they can meet.
+*The probability of meeting is the same in both cases:
+:$$p_2 = \big[\text{Min Pr(Mr. S. meets Ms. M.)}\big]\hspace{0.15cm}\underline{=25\%}.$$
+'''(3)'''&nbsp; Of the two arrival times calculated in&nbsp; '''(2)''',&nbsp; 9 o'clock&nbsp; $(\underline{\text{Minute = 60}})$&nbsp; is more favorable, <br>&nbsp; &nbsp; &nbsp; since she &ndash; if Mr. S. is not there &ndash; can leave immediately.
-[[File:P_ID246__Sto_Z_4_1_d.png|right|Günstiger Bereich für Zusammentreffen]]
-'''(2)'''&nbsp; Kommt Frau M. um 8 Uhr, so trifft sie Herrn S. nur dann, wenn dieser vor 8 Uhr 15 kommt. Erscheint Frau M. um 9 Uhr, dann muss Herr S. nach 8 Uhr 45 angekommen sein, damit sich beide treffen k&ouml;nnen. Die Wahrscheinlichkeit f&uuml;r ein Zusammentreffen ist in beiden F&auml;llen:
-$$p_1 = \text{Min[Pr(Herr S. trifft Frau M.)]}\hspace{0.15cm}\underline{=25\%}.$$
-'''(3)'''&nbsp; Von den beiden unter (2) berechneten Ankunftszeiten ist 9 Uhr ($\underline{\text{Minute = 60}}$) g&uuml;nstiger, da sie &ndash; falls Herr S. nicht da ist &ndash; sofort wieder gehen kann.
-'''(4)'''&nbsp; Die Wahrscheinlichkeit $p_4$ ergibt sich als das Verh&auml;ltnis der roten Fl&auml;che in der Grafik zur Gesamtfl&auml;che $1$. Mit den Dreiecksfl&auml;chen erh&auml;lt man:
+'''(4)'''&nbsp; The probability&nbsp; $p_4$&nbsp; is given as the ratio of the red area in the graph to the total area&nbsp; $1$.
-$$p_{\rm d}=\rm 1-2\cdot\frac{1}{2}\cdot\frac{3}{4}\cdot\frac{3}{4}=\frac{7}{16}\hspace{0.15cm}\underline{=\rm 0.4375}.$$
+*Using the triangular areas,&nbsp; one obtains:
+:$$p_4=\rm 1-2\cdot\frac{1}{2}\cdot\frac{3}{4}\cdot\frac{3}{4}=\frac{7}{16}\hspace{0.15cm}\underline{=\rm 43.75\%}.$$
 {{ML-Fuß}}
@@ Line 58: / Line 71: @@
-[[Category:Aufgaben zu Stochastische Signaltheorie|^4.1 Zweidimensionale Zufallsgrößen^]]
+[[Category:Theory of Stochastic Signals: Exercises|^4.1 Two-Dimensional Random Variables^]]