Difference between revisions of "Aufgaben:Exercise 4.2: Low-Pass for Signal Reconstruction"
m (Guenter verschob die Seite 4.2 Tiefpass zur Signalrekonstruktion nach Aufgabe 4.2: Tiefpass zur Signalrekonstruktion) |
|||
| (14 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Pulse_Code_Modulation |
}} | }} | ||
| − | [[File: | + | [[File:EN_Mod_A_4_2.png|right|frame|Examples of continuous and discrete spectra]] |
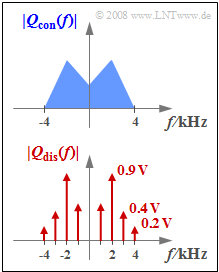
| − | + | We consider in this exercise two different source signals $q_{\rm con}(t)$ and qdis(t) whose magnitude spectra $|Q_{\rm con}(f)|$ and |Qdis(f)| are plotted. The highest frequency occurring in the signals is in each case 4kHz. | |
| − | * | + | * Nothing more is known of the spectral function $Q_{\rm con}(f)$ than that it is a continuous spectrum, where: |
| − | :$$Q_{\rm | + | :$$Q_{\rm con}(|f| \le 4\,{\rm kHz}) \ne 0 \hspace{0.05cm}.$$ |
| − | * | + | * The spectrum Qdis(f) contains spectral lines at ±1 kHz, ±2 kHz, ±3 kHz and ±4 kHz. Thus: |
| − | :qdis(t)=4∑i=1Ci⋅cos(2π⋅fi⋅t−φi) | + | :$$q_{\rm dis}(t) = \sum_{i=1}^{4}C_i \cdot \cos (2 \pi \cdot f_i \cdot t - \varphi_i),$$ |
| − | : | + | :Amplitude values: C_1 = 1.0 \ \rm V, C_2 = 1.8 \ \rm V, C_3 = 0.8 \ \rm V, C_4 = 0.4 \ \rm V. |
| + | :The phase values φ_1, φ_2 and φ_3 are respectively in the range ±180^\circ and it holds φ_4 = 90^\circ. | ||
| − | |||
| − | |||
| − | |||
| + | The signals are each sampled at frequency f_{\rm A} and immediately fed to an ideal rectangular low-pass filter with cutoff frequency f_{\rm G} This scenario applies, for example, to | ||
| + | * the interference-free pulse amplitude modulation \rm (PAM) and | ||
| + | * the interference-free pulse code modulation \rm (PCM) at infinitely large quantization stage number M. | ||
| − | |||
| + | The output signal of the (rectangular) low-pass filter is called the sink signal v(t) and for the error signal: | ||
| + | :ε(t) = v(t) - q(t). | ||
| + | This is different from zero only if the parameters of the sampling (sampling frequency f_{\rm A}) and/or the signal reconstruction (cutoff frequency f_{\rm G}) are not dimensioned in the best possible way. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | |
| + | |||
| + | Hints: | ||
| + | *The exercise belongs to the chapter [[Modulation_Methods/Pulse_Code_Modulation|"Pulse Code Modulation"]]. | ||
| + | *Reference is made in particular to the page [[Modulation_Methods/Pulse_Code_Modulation#Sampling_and_signal_reconstruction|"Sampling and Signal Reconstruction"]]. | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which statements are true for f_{\rm A} = 8\ \rm kHz and f_{\rm G} = 4\ \rm kHz ? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The signal $q_{\rm con}(t)$ can be completely reconstructed: $ε_{\rm con}(t) = 0$. |
| − | - | + | - The signal q_{\rm dis}(t) can be completely reconstructed: ε_{\rm dis}(t) = 0. |
| − | { | + | {Which statements are true for f_{\rm A} = 10\ \rm kHz and f_{\rm G} = 5\ \rm kHz ? |
| − | |type=" | + | |type="()"} |
| − | + | + | + The signal q_{\rm dis}(t) can be completely reconstructed: ε_{\rm dis}(t) = 0. |
| − | - ε_{\rm dis}(t) | + | - ε_{\rm dis}(t) is a harmonic oscillation with 4 \rm kHz. |
| − | - ε_{\rm dis}(t) | + | - ε_{\rm dis}(t) is a harmonic oscillation with 6 \rm kHz. |
| − | { | + | {Which statements are true for f_{\rm A} = 10\ \rm kHz and f_{\rm G} = 3.5\ \rm kHz ? |
| − | |type=" | + | |type="()"} |
| − | - | + | - The signal q_{\rm dis}(t) can be completely reconstructed: ε_{\rm dis}(t) = 0. |
| − | + ε_{\rm dis}(t) | + | + ε_{\rm dis}(t) is a harmonic oscillation with 4 \rm kHz. |
| − | - ε_{\rm dis}(t) | + | - ε_{\rm dis}(t) is a harmonic oscillation with 6 \rm kHz. |
| − | { | + | {Which statements are true for f_{\rm A} = 10\ \rm kHz and f_{\rm G} = 6.5\ \rm kHz? |
| − | |type=" | + | |type="()"} |
| − | - | + | - The signal q_{\rm dis}(t) can be completely reconstructed: ε_{\rm dis}(t) = 0. |
| − | - ε_{\rm dis}(t) | + | - ε_{\rm dis}(t) is a harmonic oscillation with 4 \rm kHz. |
| − | + ε_{\rm dis}(t) | + | + ε_{\rm dis}(t) is a harmonic oscillation with 6 \ \rm kHz. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Only the <u>first statement</u> is correct: |
| − | * | + | *Sampling q_{\rm dis}(t) with sampling frequency f_{\rm A} = 8 \ \rm kHz leads to an irreversible error, since Q_{\rm dis}(f) involves a discrete spectral component (Dirac delta line) at f_4 = 4\ \rm kHz and the phase value is φ_4 ≠ 0. |
| − | * | + | *With the phase value $φ_4 = 90^\circ$ $(4 \ \rm kHz sinusoidal component)$ given here holds $ε_{\rm dis}(t) = v_{\rm dis}(t) - q_{\rm dis}(t) = -0. 4 \ \rm V - \sin(2π \cdot f_4 \cdot t)$. See also solution to Exercise 4.2Z. |
| − | * | + | *On the other hand, the signal $q_{\rm con}(t)$ with the continuous spectrum $Q_{\rm con}(f)$ can also then be measured with a rectangular low-pass filter $($with cutoff frequency $f_{\rm G} = 4\ \rm kHz)$ be completely reconstructed if sampling frequency f_{\rm A} = 8\ \rm kHz was used. For all frequencies not equal to f_4 the sampling theorem is satisfied. |
| − | * | + | *The contribution of the f_4 component to the total spectrum $Q_{\rm con}(f)$ is only vanishingly small ⇒ {\rm Pr}(f_4) → 0 as long as the spectrum has no Dirac delta line at f_4. |
| + | |||
| + | |||
| + | '''(2)''' Only the <u>proposed solution 1</u> is correct: | ||
| + | *With f_{\rm A} = 10\ \rm kHz the sampling theorem is satisfied in both cases. | ||
| + | *With f_{\rm G} = f_{\rm A} /2 both error signals ε_{\rm con}(t) and ε_{\rm dis}(t) are identically zero. | ||
| + | *In addition, the signal reconstruction also works as long as f_{\rm G} > 4 \ \rm kHz and f_{\rm G} < 6 \ \rm kHz holds. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | '''(3)''' | + | '''(3)''' The correct solution here is <u>suggested solution 2</u>: |
| − | * | + | *With f_{\rm G} = 3.5 \ \rm kHz the low-pass incorrectly removes the $4\ \rm kHz$ component, that is, then holds: |
: v_{\rm dis}(t) = q_{\rm dis}(t) - 0.4\,{\rm V} \cdot \sin (2 \pi \cdot f_{\rm 4} \cdot t)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \varepsilon_{\rm dis}(t) = - 0.4\,{\rm V} \cdot \sin (2 \pi \cdot f_{\rm 4} \cdot t)\hspace{0.05cm}. | : v_{\rm dis}(t) = q_{\rm dis}(t) - 0.4\,{\rm V} \cdot \sin (2 \pi \cdot f_{\rm 4} \cdot t)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \varepsilon_{\rm dis}(t) = - 0.4\,{\rm V} \cdot \sin (2 \pi \cdot f_{\rm 4} \cdot t)\hspace{0.05cm}. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | * | + | [[File:EN_Mod_A_4_2d_neu.png|P_ID1609__Mod_A_4_2d.png|right|frame|Signal reconstruction with too large cutoff frequency]] |
| − | * | + | '''(4)''' The correct solution here is <u>suggested solution 3</u>: |
| − | * | + | *Sampling with f_{\rm A} = 10\ \rm kHz yields the periodic spectrum sketched on the right. |
| + | *The low-pass with f_{\rm G} = 6.5 \ \rm kHz removes all discrete frequency components with |f| ≥ 7\ \rm kHz, but not the 6\ \rm kHz component. | ||
| + | |||
| + | |||
| + | The error signal $ε_{\rm dis}(t) = v_{\rm dis}(t) - q_{\rm dis}(t)$ is then a harmonic oscillation with | ||
| + | * the frequency $f_6 = f_{\rm A} - f_4 = 6\ \rm kHz$, | ||
| + | * the amplitude A_4 of the f_4 component, | ||
| + | * the phase φ_{-4} = -φ_4 of the Q(f) component at f = -f_4. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 88: | Line 100: | ||
| − | [[Category: | + | [[Category:Modulation Methods: Exercises|^4.1 Pulse Code Modulation^]] |
Latest revision as of 16:31, 18 January 2023
We consider in this exercise two different source signals q_{\rm con}(t) and q_{\rm dis}(t) whose magnitude spectra |Q_{\rm con}(f)| and |Q_{\rm dis}(f)| are plotted. The highest frequency occurring in the signals is in each case 4 \rm kHz.
- Nothing more is known of the spectral function Q_{\rm con}(f) than that it is a continuous spectrum, where:
- Q_{\rm con}(|f| \le 4\,{\rm kHz}) \ne 0 \hspace{0.05cm}.
- The spectrum Q_{\rm dis}(f) contains spectral lines at ±1 \ \rm kHz, ±2 \ \rm kHz, ±3 \ \rm kHz and ±4 \ \rm kHz. Thus:
- q_{\rm dis}(t) = \sum_{i=1}^{4}C_i \cdot \cos (2 \pi \cdot f_i \cdot t - \varphi_i),
- Amplitude values: C_1 = 1.0 \ \rm V, C_2 = 1.8 \ \rm V, C_3 = 0.8 \ \rm V, C_4 = 0.4 \ \rm V.
- The phase values φ_1, φ_2 and φ_3 are respectively in the range ±180^\circ and it holds φ_4 = 90^\circ.
The signals are each sampled at frequency f_{\rm A} and immediately fed to an ideal rectangular low-pass filter with cutoff frequency f_{\rm G} This scenario applies, for example, to
- the interference-free pulse amplitude modulation \rm (PAM) and
- the interference-free pulse code modulation \rm (PCM) at infinitely large quantization stage number M.
The output signal of the (rectangular) low-pass filter is called the sink signal v(t) and for the error signal:
- ε(t) = v(t) - q(t).
This is different from zero only if the parameters of the sampling (sampling frequency f_{\rm A}) and/or the signal reconstruction (cutoff frequency f_{\rm G}) are not dimensioned in the best possible way.
Hints:
- The exercise belongs to the chapter "Pulse Code Modulation".
- Reference is made in particular to the page "Sampling and Signal Reconstruction".
Questions
Solution
- Sampling q_{\rm dis}(t) with sampling frequency f_{\rm A} = 8 \ \rm kHz leads to an irreversible error, since Q_{\rm dis}(f) involves a discrete spectral component (Dirac delta line) at f_4 = 4\ \rm kHz and the phase value is φ_4 ≠ 0.
- With the phase value φ_4 = 90^\circ (4 \ \rm kHz sinusoidal component) given here holds ε_{\rm dis}(t) = v_{\rm dis}(t) - q_{\rm dis}(t) = -0. 4 \ \rm V - \sin(2π \cdot f_4 \cdot t). See also solution to Exercise 4.2Z.
- On the other hand, the signal q_{\rm con}(t) with the continuous spectrum Q_{\rm con}(f) can also then be measured with a rectangular low-pass filter (with cutoff frequency f_{\rm G} = 4\ \rm kHz) be completely reconstructed if sampling frequency f_{\rm A} = 8\ \rm kHz was used. For all frequencies not equal to f_4 the sampling theorem is satisfied.
- The contribution of the f_4 component to the total spectrum Q_{\rm con}(f) is only vanishingly small ⇒ {\rm Pr}(f_4) → 0 as long as the spectrum has no Dirac delta line at f_4.
(2) Only the proposed solution 1 is correct:
- With f_{\rm A} = 10\ \rm kHz the sampling theorem is satisfied in both cases.
- With f_{\rm G} = f_{\rm A} /2 both error signals ε_{\rm con}(t) and ε_{\rm dis}(t) are identically zero.
- In addition, the signal reconstruction also works as long as f_{\rm G} > 4 \ \rm kHz and f_{\rm G} < 6 \ \rm kHz holds.
(3) The correct solution here is suggested solution 2:
- With f_{\rm G} = 3.5 \ \rm kHz the low-pass incorrectly removes the 4\ \rm kHz component, that is, then holds:
- v_{\rm dis}(t) = q_{\rm dis}(t) - 0.4\,{\rm V} \cdot \sin (2 \pi \cdot f_{\rm 4} \cdot t)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \varepsilon_{\rm dis}(t) = - 0.4\,{\rm V} \cdot \sin (2 \pi \cdot f_{\rm 4} \cdot t)\hspace{0.05cm}.
(4) The correct solution here is suggested solution 3:
- Sampling with f_{\rm A} = 10\ \rm kHz yields the periodic spectrum sketched on the right.
- The low-pass with f_{\rm G} = 6.5 \ \rm kHz removes all discrete frequency components with |f| ≥ 7\ \rm kHz, but not the 6\ \rm kHz component.
The error signal ε_{\rm dis}(t) = v_{\rm dis}(t) - q_{\rm dis}(t) is then a harmonic oscillation with
- the frequency f_6 = f_{\rm A} - f_4 = 6\ \rm kHz,
- the amplitude A_4 of the f_4 component,
- the phase φ_{-4} = -φ_4 of the Q(f) component at f = -f_4.