Difference between revisions of "Aufgaben:Exercise 1.13: Binary Erasure Channel Decoding"
m (Guenter verschob die Seite Aufgabe 1.13: Decodierung beim ''B'' nach Aufgabe 1.13: Decodierung beim binären Auslöschungskanal (BEC)) |
|||
| (20 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Decoding_of_Linear_Block_Codes}} |
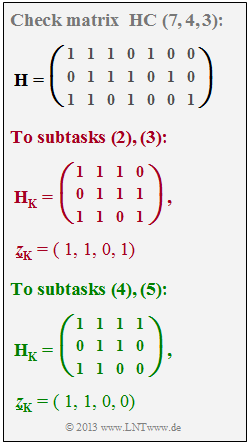
| − | [[File: | + | [[File:EN_KC_A_1_13.png|right|frame|Decoding at the BEC]] |
| − | + | We assume here the model in section [[Channel_Coding/Decoding_of_Linear_Block_Codes#Decoding_at_the_Binary_Erasure_Channel|"Decoding at the Binary Erasure Channel"]] (BEC configuration highlighted in green): | |
| − | * | + | *Each information word \underline{u} is encoded blockwise and yields the code word \underline{x}. Let the block code be linear and completely given by its parity-check matrix \boldsymbol{\rm H}. |
| − | * | + | *During transmission n_{\rm E} bits of the code word are erased ⇒ [[Channel_Coding/Channel_Models_and_Decision_Structures#Binary_Erasure_Channel_.E2.80.93_BEC|"Binary Erasure Channel"]] $\rm (BEC)$. The code word \underline{x} thus becomes the received word \underline{y}. |
| − | * | + | *If the number n_{\rm E} of erasures is less than the [[Channel_Coding/Objective_of_Channel_Coding#Important_definitions_for_block_coding|"minimum distance"]] d_{\rm min} of the code, it is possible to reconstruct from \underline{y} the code word \underline{z} = \underline{x} without error, and thus the correct information word $\underline{v} = \underline{u}$ is also obtained. |
| − | |||
| + | For exercise description, let us now consider the Hamming code word \underline{x} = (0, 1, 0, 1, 1, 0, 0) and the received word \underline{y} = (0, 1, {\rm E} , {\rm E}, 1, 0, 0). | ||
| − | + | *The third and fourth bit were thus erased by the channel. The "code word finder" thus has the task to determine the vector z_{\rm E} = (z_{3}, z_{4}) with z_{3}, \ z_{4} \in \{0, 1\} to be determined. This is done according to the equation | |
| − | :$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.05cm} | + | :$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.05cm}.$$ |
| − | + | *In the present example: | |
:\underline{z}_{\rm K} = (0, 1, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &1 &0 &0\\ 0 &1 &0 &1 &0\\ 1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &1\\ 0 &1 \end{pmatrix} \hspace{0.05cm}. | :\underline{z}_{\rm K} = (0, 1, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &1 &0 &0\\ 0 &1 &0 &1 &0\\ 1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &1\\ 0 &1 \end{pmatrix} \hspace{0.05cm}. | ||
| − | + | *This equation gives two equations for the bits to be determined, whose solution leads to the result z_{3} = 0 and z_{4} = 1. | |
| − | |||
| − | |||
| − | |||
| − | |||
| + | Hints: | ||
| + | * This exercise belongs to the chapter [[Channel_Coding/Decoding_of_Linear_Block_Codes|"Decoding of Linear Block Codes"]]. | ||
| + | * The algorithm for mapping the received word \underline{y} to the correct code word \underline{z} = \underline{x} is described in detail in the [[Channel_Coding/Decoding_of_Linear_Block_Codes#Decoding_at_the_Binary_Erasure_Channel|"theory section"]] . | ||
| + | |||
| + | * We would like to remind again that in BEC decoding we use the first decoder block \underline{y} → \underline{z} as "code word finder" since wrong decisions are excluded here. Each received word is decoded correctly, or it may not be decoded at all. | ||
| + | |||
| + | *In the BSC model, on the other hand, decoding errors cannot be avoided. Accordingly, we refer to the corresponding block there as "code word estimator". | ||
| − | === | + | |
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | { \underline{y} = (1, {\rm E}, 0, 1, 0, 0, {\rm E}) was received. Which sequence does the code word finder decide to use? |
| − | |type=" | + | |type="()"} |
- \underline{z} = (1, 0, 0, 1, 0, 0, 0), | - \underline{z} = (1, 0, 0, 1, 0, 0, 0), | ||
+ \underline{z} = (1, 1, 0, 1, 0, 0, 1), | + \underline{z} = (1, 1, 0, 1, 0, 0, 1), | ||
- \underline{z} = (1, 0, 0, 1, 0, 0, 1). | - \underline{z} = (1, 0, 0, 1, 0, 0, 1). | ||
| − | { | + | {What are the consequences of the red entries for \boldsymbol{\rm H}_{\rm K} and z_{\rm K} (see graphic in the information section)? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The erasure vector is \underline{z}_{\rm E} = (z_{5}, z_{6}, z_{7}). |
| − | + | + | + The received word is \underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}). |
| − | - \boldsymbol{\rm H}_{\rm E} | + | - \boldsymbol{\rm H}_{\rm E} is a $2 \times 3$ matrix. |
| − | + \boldsymbol{\rm H}_{\rm E} | + | + \boldsymbol{\rm H}_{\rm E} is a $3 \times 3$ matrix. |
| − | { | + | {Now apply \underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}). Which code word is selected? |
| − | |type=" | + | |type="()"} |
- \underline{z} = (1, 1, 0, 1, 1, 1, 0), | - \underline{z} = (1, 1, 0, 1, 1, 1, 0), | ||
+ \underline{z} = (1, 1, 0, 1, 0, 0, 1), | + \underline{z} = (1, 1, 0, 1, 0, 0, 1), | ||
- \underline{z} = (1, 1, 0, 0, 0, 1, 0). | - \underline{z} = (1, 1, 0, 0, 0, 1, 0). | ||
| − | - | + | - For the present \underline{y} no unique decoding is possible. |
| − | { | + | {What are the consequences of the green entries for \boldsymbol{\rm H}_{\rm K} and z_{\rm K} (see graphic in the information section)? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The received word is \underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E}). |
| − | - \boldsymbol{\rm H}_{\rm K} | + | - \boldsymbol{\rm H}_{\rm K} differs from subtask '''(2)''' in the last row. |
| − | + \boldsymbol{\rm H}_{\rm K} | + | + \boldsymbol{\rm H}_{\rm K} differs from subtask '''(2)''' in the last column. |
| − | { | + | {Now apply \underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E}). Which code word is selected? |
| − | |type=" | + | |type="()"} |
- \underline{z} = (1, 1, 0, 1, 1, 1, 0), | - \underline{z} = (1, 1, 0, 1, 1, 1, 0), | ||
- \underline{z} = (1, 1, 0, 1, 0, 0, 1), | - \underline{z} = (1, 1, 0, 1, 0, 0, 1), | ||
- \underline{z} = (1, 1, 0, 0, 0, 1, 0). | - \underline{z} = (1, 1, 0, 0, 0, 1, 0). | ||
| − | + | + | + For the present \underline{y} no unique decoding is possible. |
| − | { | + | {Which statements result for the correction capability at the BEC? n_{\rm E} indicates in the following the number of erasures. |
|type="[]"} | |type="[]"} | ||
| − | + | + | + For n_{\rm E} < d_{\rm min} unique decoding is always possible. |
| − | - | + | - For n_{\rm E} = d_{\rm min} unique decoding is always possible. |
| − | + | + | + For n_{\rm E} = d_{\rm min} sometimes a unique decoding is possible. |
| − | + | + | + For n_{\rm E} > d_{\rm min} unique decoding is never possible. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The received vector is \underline{y} = (1, {\rm E}, 0, 1, 0, 0, {\rm E}). Thus, the code symbols at positions 2 and 7 were erased. Based on the given parity-check matrix |
:{ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &0 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0 &1 \end{pmatrix} | :{ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &0 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0 &1 \end{pmatrix} | ||
| − | + | of the Hamming code is obtained for vector and matrix | |
| − | * | + | * with respect to all "correctly transmitted code symbols" $($index "$\rm K$" from German "korrekt"$)$ which are known to the code word finder: |
:\underline{z}_{\rm K} = (1, 0, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \hspace{0.05cm}, | :\underline{z}_{\rm K} = (1, 0, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \hspace{0.05cm}, | ||
| − | * | + | *with respect to the two "erased code symbols" z_{2} and $z_{7}$ $($index $\rm E)$ to be determined: |
:\underline{z}_{\rm E} = (z_2, z_7)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \hspace{0.05cm}. | :\underline{z}_{\rm E} = (z_2, z_7)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \hspace{0.05cm}. | ||
| − | + | The equation of determination is thus: | |
| + | :$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.3cm} | ||
| + | \Rightarrow \hspace{0.3cm} \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \cdot \begin{pmatrix} z_2 \\ z_7 \end{pmatrix} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 1 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | This results in three equations for the two unknowns $z_{2} and z_{7}$: | |
| − | :$$\ | + | :$${\rm (a)}\ z_{2} = 1,$$ |
| + | :$${\rm (b)}\ z_{2} = 1,$$ | ||
| + | :$${\rm (c)}\ z_{2} + z_{7} = 0 \ \Rightarrow \ z_{7}= 1.$$ | ||
| − | + | Thus, the code word finder returns $\underline{z} = (1, 1, 0, 1, 0, 0, 1)$ ⇒ <u>Suggested solution 2</u>. | |
| − | |||
| − | |||
| − | |||
| + | '''(2)''' Looking at the given matrix \boldsymbol{\rm H}_{\rm K}, we see that it coincides with the first four columns of the parity-check matrix \boldsymbol{\rm H}. | ||
| + | |||
| + | *The erasures thus affect the last three bits of the received word ⇒ \underline{z}_{\rm E} = (z_{5}, z_{6}, z_{7}) ⇒ \underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}). | ||
| + | *The erasure matrix reads: | ||
| + | |||
| + | :{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \hspace{0.05cm}. | ||
| − | + | Correct are therefore the <u>statements 1, 2 and 4</u>. | |
| − | '''( | + | '''(3)''' One obtains after some matrix multiplications: |
| + | :$${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &0\\ 0 &1 &1 &1\\ 1 &1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} | ||
| + | { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | *By equating it follows $z_{5} = 0, \ z_{6} = 0, \ z_{7} = 1$ ⇒ <u>Suggested solution 2</u>. | |
| − | |||
| − | '''( | + | '''(4)''' The matrix comparison shows that the first three columns of $\boldsymbol{\rm H} and \boldsymbol{\rm H}_{\rm K}$ are identical. |
| − | + | * The fourth column of $\boldsymbol{\rm H}_{\rm K}$ is equal to the fifth column of the parity-check matrix $\boldsymbol{\rm H}$. | |
| − | |||
| − | |||
| − | + | *From this follows for the vector $z_{\rm E} = (z_{4}, z_{6}, z_{7}) and further for the received vector \underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E})$ ⇒ <u>Suggested solutions 1 and 3</u>. | |
| − | '''( | + | '''(5)''' Analogous to subtask '''(3)''', we obtain: |
| − | + | ||
| + | :$${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &1\\ 0 &1 &1 &0\\ 1 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 0 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} | ||
| + | { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 0 &0 &0\\ 1 &1 &0\\ 1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_4 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} 0 \\ z_4 + z_6 \\ z_4 + z_7 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| + | |||
| + | *If we now set the two column vectors equal, we obtain only two equations for the three unknowns ⇒ <u>Suggested solution 4</u>. Or in other words: | ||
| − | + | *If the number of extinctions of the BEC channel is larger than the rank of the matrix $\boldsymbol{\rm H}_{\rm E}$, then no unique solution of the resulting system of equations results. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | '''(6)''' | + | '''(6)''' To solve this exercise, we again refer to the systematic Hamming code (7, 4, 3) according to the given parity-check equation and code table. <u>Note:</u> |
| + | [[File:P_ID2540__KC_A_1_13f.png|right|frame|Code table of the systematic (7, 4, 3) Hamming code<br><br><br>]] | ||
| + | *The information bits are shown in black and the parity bits in red. The minimum distance of this code is d_{\rm min} = 3. | ||
| − | + | *Further we assume that always the code word $\underline{x} = (1, 1, 0, 1, 0, 0, 1)$ with yellow background was sent. Then holds: | |
| − | + | *If the number $n_{\rm E} of erasures is smaller than d_{\rm min} = 3$, decoding by the method described here is always possible ⇒ see e.g. subtask '''(1)''' with $n_{\rm E}= 2$. | |
| − | * | + | *For $n_{\rm E} = d_{\rm min} = 3$ decoding is sometimes possible, see subtask '''(3)'''. In the code table, there is only one code word that could match $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E})$ namely the code word highlighted in yellow $\underline{x} = (1, 1, 0, 1, 0, 0, 1)$ . |
| − | * | + | *In contrast $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E})$ according to subtask '''(4)''' could not be decoded. In the code table, besides (1, 1, 0, 1, 0, 0, 1) with (1, 1, 0, 0, 0, 1, 0) one can recognize another code word (highlighted in green), which becomes the received word \underline{y} due to the n_{\rm E} = 3 erasures concerning bit 4, 6 and 7. |
| − | * | + | *This case, when the n_{\rm E} = d_{\rm min} extinctions affect exactly the d_{\rm min} different bits of two code words, leads to a matrix \mathbf{H}_{\rm E} with rank smaller d_{\rm min}. |
| − | * | + | *If $n_{\rm E} > d_{\rm min}$, the number $n - n_{\rm E}$ of bits not erased is smaller than the number k of information bits. In this case, of course, the code word cannot be decoded. |
| − | + | That is: Applicable are the <u>statements 1, 3 and 4</u>. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Channel Coding: Exercises|^1.5 Linear Block Code Decoding^]] |
Latest revision as of 18:02, 23 January 2023
We assume here the model in section "Decoding at the Binary Erasure Channel" (BEC configuration highlighted in green):
- Each information word \underline{u} is encoded blockwise and yields the code word \underline{x}. Let the block code be linear and completely given by its parity-check matrix \boldsymbol{\rm H}.
- During transmission n_{\rm E} bits of the code word are erased ⇒ "Binary Erasure Channel" \rm (BEC). The code word \underline{x} thus becomes the received word \underline{y}.
- If the number n_{\rm E} of erasures is less than the "minimum distance" d_{\rm min} of the code, it is possible to reconstruct from \underline{y} the code word \underline{z} = \underline{x} without error, and thus the correct information word \underline{v} = \underline{u} is also obtained.
For exercise description, let us now consider the Hamming code word \underline{x} = (0, 1, 0, 1, 1, 0, 0) and the received word \underline{y} = (0, 1, {\rm E} , {\rm E}, 1, 0, 0).
- The third and fourth bit were thus erased by the channel. The "code word finder" thus has the task to determine the vector z_{\rm E} = (z_{3}, z_{4}) with z_{3}, \ z_{4} \in \{0, 1\} to be determined. This is done according to the equation
- { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.05cm}.
- In the present example:
- \underline{z}_{\rm K} = (0, 1, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &1 &0 &0\\ 0 &1 &0 &1 &0\\ 1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &1\\ 0 &1 \end{pmatrix} \hspace{0.05cm}.
- This equation gives two equations for the bits to be determined, whose solution leads to the result z_{3} = 0 and z_{4} = 1.
Hints:
- This exercise belongs to the chapter "Decoding of Linear Block Codes".
- The algorithm for mapping the received word \underline{y} to the correct code word \underline{z} = \underline{x} is described in detail in the "theory section" .
- We would like to remind again that in BEC decoding we use the first decoder block \underline{y} → \underline{z} as "code word finder" since wrong decisions are excluded here. Each received word is decoded correctly, or it may not be decoded at all.
- In the BSC model, on the other hand, decoding errors cannot be avoided. Accordingly, we refer to the corresponding block there as "code word estimator".
Questions
Solution
- { \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &0 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0 &1 \end{pmatrix}
of the Hamming code is obtained for vector and matrix
- with respect to all "correctly transmitted code symbols" (index "\rm K" from German "korrekt") which are known to the code word finder:
- \underline{z}_{\rm K} = (1, 0, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \hspace{0.05cm},
- with respect to the two "erased code symbols" z_{2} and z_{7} (index \rm E) to be determined:
- \underline{z}_{\rm E} = (z_2, z_7)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \hspace{0.05cm}.
The equation of determination is thus:
- { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \cdot \begin{pmatrix} z_2 \\ z_7 \end{pmatrix} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 1 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm}.
This results in three equations for the two unknowns z_{2} and z_{7}:
- {\rm (a)}\ z_{2} = 1,
- {\rm (b)}\ z_{2} = 1,
- {\rm (c)}\ z_{2} + z_{7} = 0 \ \Rightarrow \ z_{7}= 1.
Thus, the code word finder returns \underline{z} = (1, 1, 0, 1, 0, 0, 1) ⇒ Suggested solution 2.
(2) Looking at the given matrix \boldsymbol{\rm H}_{\rm K}, we see that it coincides with the first four columns of the parity-check matrix \boldsymbol{\rm H}.
- The erasures thus affect the last three bits of the received word ⇒ \underline{z}_{\rm E} = (z_{5}, z_{6}, z_{7}) ⇒ \underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}).
- The erasure matrix reads:
- { \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \hspace{0.05cm}.
Correct are therefore the statements 1, 2 and 4.
(3) One obtains after some matrix multiplications:
- { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &0\\ 0 &1 &1 &1\\ 1 &1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} \hspace{0.05cm}.
- By equating it follows z_{5} = 0, \ z_{6} = 0, \ z_{7} = 1 ⇒ Suggested solution 2.
(4) The matrix comparison shows that the first three columns of \boldsymbol{\rm H} and \boldsymbol{\rm H}_{\rm K} are identical.
- The fourth column of \boldsymbol{\rm H}_{\rm K} is equal to the fifth column of the parity-check matrix \boldsymbol{\rm H}.
- From this follows for the vector z_{\rm E} = (z_{4}, z_{6}, z_{7}) and further for the received vector \underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E}) ⇒ Suggested solutions 1 and 3.
(5) Analogous to subtask (3), we obtain:
- { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &1\\ 0 &1 &1 &0\\ 1 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 0 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 0 &0 &0\\ 1 &1 &0\\ 1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_4 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} 0 \\ z_4 + z_6 \\ z_4 + z_7 \end{pmatrix} \hspace{0.05cm}.
- If we now set the two column vectors equal, we obtain only two equations for the three unknowns ⇒ Suggested solution 4. Or in other words:
- If the number of extinctions of the BEC channel is larger than the rank of the matrix \boldsymbol{\rm H}_{\rm E}, then no unique solution of the resulting system of equations results.
(6) To solve this exercise, we again refer to the systematic Hamming code (7, 4, 3) according to the given parity-check equation and code table. Note:
- The information bits are shown in black and the parity bits in red. The minimum distance of this code is d_{\rm min} = 3.
- Further we assume that always the code word \underline{x} = (1, 1, 0, 1, 0, 0, 1) with yellow background was sent. Then holds:
- If the number n_{\rm E} of erasures is smaller than d_{\rm min} = 3, decoding by the method described here is always possible ⇒ see e.g. subtask (1) with n_{\rm E}= 2.
- For n_{\rm E} = d_{\rm min} = 3 decoding is sometimes possible, see subtask (3). In the code table, there is only one code word that could match \underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}) namely the code word highlighted in yellow \underline{x} = (1, 1, 0, 1, 0, 0, 1) .
- In contrast \underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E}) according to subtask (4) could not be decoded. In the code table, besides (1, 1, 0, 1, 0, 0, 1) with (1, 1, 0, 0, 0, 1, 0) one can recognize another code word (highlighted in green), which becomes the received word \underline{y} due to the n_{\rm E} = 3 erasures concerning bit 4, 6 and 7.
- This case, when the n_{\rm E} = d_{\rm min} extinctions affect exactly the d_{\rm min} different bits of two code words, leads to a matrix \mathbf{H}_{\rm E} with rank smaller d_{\rm min}.
- If n_{\rm E} > d_{\rm min}, the number n - n_{\rm E} of bits not erased is smaller than the number k of information bits. In this case, of course, the code word cannot be decoded.
That is: Applicable are the statements 1, 3 and 4.