Difference between revisions of "Aufgaben:Exercise 4.2: UMTS Radio Channel Basics"
From LNTwww
| (17 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Examples_of_Communication_Systems/General_Description_of_UMTS |
}} | }} | ||
| − | [[File: | + | [[File:EN_Bei_A_4_2_v2.png|right|frame|Path loss, frequency/time–selective fading ]] |
| − | + | UMTS also has quite a few effects leading to degradation that must be taken into account during system planning: | |
| − | *${\rm | + | *${\rm Interference}$: Since all users are simultaneously served in the same frequency band, each user is interfered by other users. |
| − | |||
| − | |||
| − | |||
| + | *{\rm Path\:loss}: The received power P_{\rm E} of a radio signal decreases with distance d by a factor d^{- \gamma}. | ||
| − | + | *${\rm Multipath\:propagation}$: The signal reaches the mobile receiver not only through the direct path, but through several paths – differently attenuated and differently delayed. | |
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | : | ||
| − | |||
| − | |||
| − | |||
| + | *{\rm Doppler\:effect}: If transmitter and/or receiver move, frequency shifts can occur depending on speed and the direction (Which angle? Towards each other? Away from each other?). | ||
| + | In the book "[[Mobile Communications]]" these effects have already been discussed in detail. The diagrams convey only a few pieces of information regarding | ||
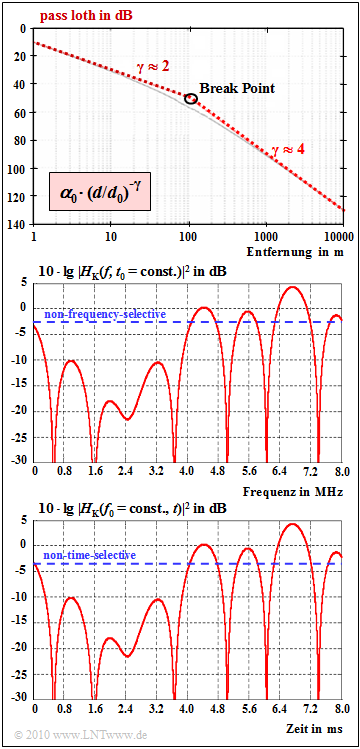
| + | *<u>Path loss:</u> Path loss indicates the decrease in the received power with distance d from the transmitter. Above the so-called "break point" applies approximately to the received power: | ||
| + | ::\frac{P(d)}{P(d_0)} = \alpha_0 \cdot \left ( {d}/{d_0}\right )^{-4}. | ||
| + | :According to the upper graph \alpha_{0} = 10^{-5} (correspondingly 50 \ \rm dB) and d_{0} = 100 \ \rm m. | ||
| + | *<u>Frequency-selective fading:</u> The power transfer function |H_{\rm K}(f)|^{2} at a given time according to the middle graph illustrates frequency-selective fading. The blue-dashed horizontal line, on the other hand, indicates non-frequency-selective fading. | ||
| + | ::Such frequency-selective fading occurs when the coherence bandwidth B_{\rm K} is much smaller than the signal bandwidth B_{\rm S}. Here, with the "delay spread" T_{\rm V} ⇒ difference between the maximum and minimum delay times: | ||
| + | ::B_{\rm K}\approx \frac{1}{T_{\rm V}}= \frac{1}{\tau_{\rm max}- \tau_{\rm min}}. | ||
| − | + | *<u>Time-selective fading:</u> The bottom graph shows the power transfer function |H_{\rm K}(t)|^{2} for a fixed frequency f_{0}. The sketch is to be understood schematically, because for the time-selective fading considered here exactly the same course was chosen as in the middle diagram for the frequency-selective fading (pure convenience of the author). | |
| + | ::Here a so-called "Doppler spread" B_{\rm D} arises, defined as the difference between the maximum and the minimum Doppler frequency. The inverse T_{\rm D} = 1/B_{\rm D} is called "coherence time" or also "correlation duration". In UMTS, time-selective fading occurs whenever T_{\rm D} \ll T_{\rm C} (chip duration). | ||
| − | |||
| − | |||
| − | |||
| − | |||
| + | <u>Hints:</u> | ||
| − | === | + | *This exercise belongs to the chapter [[Examples_of_Communication_Systems/General_Description_of_UMTS|"General Description of UMTS"]]. |
| + | |||
| + | *Reference is made in particular to the sections [[Examples_of_Communication_Systems/General_Description_of_UMTS#Properties_of_the_UMTS_radio_channel|"Properties of the UMTS radio channel"]] and [[Examples_of_Communication_Systems/General_Description_of_UMTS#Frequency.E2.80.93selective_and_time.E2.80.93selective_fading|"Frequency-selective and time-selective fading"]]. | ||
| + | |||
| + | *For UMTS, the bandwidth: $B_{\rm S} = 5 \ \rm MHz and the chip duration: T_{\rm C} \approx 0.26 \ \rm µ s$. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Starting from the top graph on the information page, calculate the path loss $($in $\rm dB)$ for d = \rm 5 \ km. |
|type="{}"} | |type="{}"} | ||
| − | ${\rm | + | ${\rm path\ loss} \ = \ { 118 3% } \ \rm dB $. |
| − | { | + | {What statements are true regarding frequency-selective fading? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + This is caused by multipath reception. |
| − | - | + | - It is caused by movement of transmitter and/or receiver. |
| − | + | + | + Different frequencies are attenuated differently. |
| − | + | + | + An echo at a distance 1\ \rm µ s results in frequency-selective fading. |
| − | { | + | {What statements are true regarding time-selective fading? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - This arises due to multipath reception. |
| − | + | + | + It results from movement of transmitter and/or receiver. |
| − | - | + | - Different frequencies are attenuated differently. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' According to the sketch, the breakpoint is at d_{0} = 100 \ \rm m. |
| − | * | + | *For d ≤ d_{0}, the path loss is equal to $\alpha_{0} \cdot (d/d_{0})^{-2}$. For d = d_{0} = 100 \ \rm m holds: |
| − | :$${\rm | + | :$${\rm path\ loss} = \alpha_0 = 10^{-5}\hspace{0.5cm}\Rightarrow\hspace{0.5cm}{50\,{\rm dB}}.$$ |
| − | * | + | |
| − | :$${\rm | + | *Above d_{0}, the path loss is equal to $\alpha_{0} \cdot (d/d_{0})^{-4}$. Thus, at 5 \ \rm km distance, one obtains: |
| + | :$${\rm path\ loss} = 10^{-5}\cdot 50^{-4} = 1.6 \cdot 10^{-12}\hspace{0.5cm}\Rightarrow\hspace{0.5cm}\underline{118\,{\rm dB}}.$$ | ||
| + | |||
| + | |||
| + | '''(2)''' Correct are the <u>statements 1, 3, and 4</u>: | ||
| + | *Frequency-selective fading is due to multipath reception. This means: | ||
| + | |||
| + | *Different frequency components are delayed and attenuated differently by the channel. | ||
| + | |||
| + | *This results in attenuation and phase distortion. | ||
| + | |||
| + | *Because \tau_{\rm max} = 1 \ \rm µ s (simplifying \tau_{\rm min} = 0 is set) further results in | ||
| + | :$$B_{\rm K} = \frac{1}{\tau_{\rm max}- \tau_{\rm min}} = 1\,{\rm MHz}\ \ll \ B_{\rm S} \hspace{0.15cm}\underline {= 5\,{\rm MHz}}.$$ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | '''(3)''' | + | '''(3)''' Correct is <u>statement 2</u>. |
| + | *Statements 1 and 3, on the other hand, are valid for frequency-selective fading – see subtask '''(2)'''. | ||
| Line 81: | Line 93: | ||
| − | [[Category: | + | [[Category:Examples of Communication Systems: Exercises|^4.1 General Description of UMTS |
^]] | ^]] | ||
Latest revision as of 17:26, 13 February 2023
UMTS also has quite a few effects leading to degradation that must be taken into account during system planning:
- {\rm Interference}: Since all users are simultaneously served in the same frequency band, each user is interfered by other users.
- {\rm Path\:loss}: The received power P_{\rm E} of a radio signal decreases with distance d by a factor d^{- \gamma}.
- {\rm Multipath\:propagation}: The signal reaches the mobile receiver not only through the direct path, but through several paths – differently attenuated and differently delayed.
- {\rm Doppler\:effect}: If transmitter and/or receiver move, frequency shifts can occur depending on speed and the direction (Which angle? Towards each other? Away from each other?).
In the book "Mobile Communications" these effects have already been discussed in detail. The diagrams convey only a few pieces of information regarding
- Path loss: Path loss indicates the decrease in the received power with distance d from the transmitter. Above the so-called "break point" applies approximately to the received power:
- \frac{P(d)}{P(d_0)} = \alpha_0 \cdot \left ( {d}/{d_0}\right )^{-4}.
- According to the upper graph \alpha_{0} = 10^{-5} (correspondingly 50 \ \rm dB) and d_{0} = 100 \ \rm m.
- Frequency-selective fading: The power transfer function |H_{\rm K}(f)|^{2} at a given time according to the middle graph illustrates frequency-selective fading. The blue-dashed horizontal line, on the other hand, indicates non-frequency-selective fading.
- Such frequency-selective fading occurs when the coherence bandwidth B_{\rm K} is much smaller than the signal bandwidth B_{\rm S}. Here, with the "delay spread" T_{\rm V} ⇒ difference between the maximum and minimum delay times:
- B_{\rm K}\approx \frac{1}{T_{\rm V}}= \frac{1}{\tau_{\rm max}- \tau_{\rm min}}.
- Time-selective fading: The bottom graph shows the power transfer function |H_{\rm K}(t)|^{2} for a fixed frequency f_{0}. The sketch is to be understood schematically, because for the time-selective fading considered here exactly the same course was chosen as in the middle diagram for the frequency-selective fading (pure convenience of the author).
- Here a so-called "Doppler spread" B_{\rm D} arises, defined as the difference between the maximum and the minimum Doppler frequency. The inverse T_{\rm D} = 1/B_{\rm D} is called "coherence time" or also "correlation duration". In UMTS, time-selective fading occurs whenever T_{\rm D} \ll T_{\rm C} (chip duration).
Hints:
- This exercise belongs to the chapter "General Description of UMTS".
- Reference is made in particular to the sections "Properties of the UMTS radio channel" and "Frequency-selective and time-selective fading".
- For UMTS, the bandwidth: B_{\rm S} = 5 \ \rm MHz and the chip duration: T_{\rm C} \approx 0.26 \ \rm µ s.
Questions
Solution
(1) According to the sketch, the breakpoint is at d_{0} = 100 \ \rm m.
- For d ≤ d_{0}, the path loss is equal to \alpha_{0} \cdot (d/d_{0})^{-2}. For d = d_{0} = 100 \ \rm m holds:
- {\rm path\ loss} = \alpha_0 = 10^{-5}\hspace{0.5cm}\Rightarrow\hspace{0.5cm}{50\,{\rm dB}}.
- Above d_{0}, the path loss is equal to \alpha_{0} \cdot (d/d_{0})^{-4}. Thus, at 5 \ \rm km distance, one obtains:
- {\rm path\ loss} = 10^{-5}\cdot 50^{-4} = 1.6 \cdot 10^{-12}\hspace{0.5cm}\Rightarrow\hspace{0.5cm}\underline{118\,{\rm dB}}.
(2) Correct are the statements 1, 3, and 4:
- Frequency-selective fading is due to multipath reception. This means:
- Different frequency components are delayed and attenuated differently by the channel.
- This results in attenuation and phase distortion.
- Because \tau_{\rm max} = 1 \ \rm µ s (simplifying \tau_{\rm min} = 0 is set) further results in
- B_{\rm K} = \frac{1}{\tau_{\rm max}- \tau_{\rm min}} = 1\,{\rm MHz}\ \ll \ B_{\rm S} \hspace{0.15cm}\underline {= 5\,{\rm MHz}}.
(3) Correct is statement 2.
- Statements 1 and 3, on the other hand, are valid for frequency-selective fading – see subtask (2).