Difference between revisions of "Exercise 2.4Z: Characteristics Measurement"
| (16 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/Nonlinear_Distortion |
}} | }} | ||
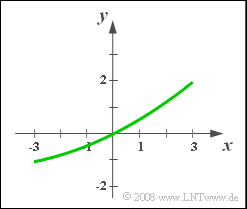
| − | [[File:P_ID898__LZI_Z_2_4.png|right|frame| | + | [[File:P_ID898__LZI_Z_2_4.png|right|frame|Given characteristic $y = g(x)$]] |
| − | + | It is known that the characteristic curve can be represented as follows for a nonlinear system: | |
:y(t)=c1⋅x(t)+c2⋅x2(t). | :y(t)=c1⋅x(t)+c2⋅x2(t). | ||
| − | + | Since the distortions are nonlinear no frequency response H(f) can be given. | |
| − | + | To determine the dimensionless coefficient c1 as well as the quadratic coefficient c2 different rectangular pulses x(t) – characterized by the amplitude Ax and the width Tx – are now applied to the input and the pulse amplitude Ay at the output is measured in each case. | |
| − | + | The first three trials generate the following values: | |
| − | * Ax=1 V,Tx=8 ms : Ay=0.55 V, | + | * Ax=1 V,Tx=8 ms : Ay=0.55 V, |
| − | * Ax=2 V,Tx=4 ms : Ay=1.20 V, | + | * Ax=2 V,Tx=4 ms : Ay=1.20 V, |
| − | * Ax=3 V,Tx=2 ms : Ay=1.95 V. | + | * Ax=3 V,Tx=2 ms : Ay=1.95 V. |
| − | |||
| − | + | For the subtasks '''(3)''' and '''(4)''' let the input signal x(t) be a harmonic oscillation because only for such an oscillation a distortion factor can be specified. | |
| − | |||
| + | In contrast, a triangular pulse with amplitude Ax=3 V and the one-sided pulse duration Tx=2 ms is considered for the subtask '''(5)''' : | ||
| + | :x(t)=Ax⋅(1−|t|/Tx) | ||
| Line 26: | Line 26: | ||
| − | '' | + | ''Please note:'' |
| − | * | + | *The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/Nonlinear_Distortion|Nonlinear Distortions]]. |
| − | + | ||
| − | * | + | *The following abbreviations are used in the formulation of the questions: |
:$$y_1(t) = c_1 \cdot x(t), \hspace{0.5cm} y_2(t) = c_2 \cdot | :$$y_1(t) = c_1 \cdot x(t), \hspace{0.5cm} y_2(t) = c_2 \cdot | ||
x^2(t).$$ | x^2(t).$$ | ||
| Line 35: | Line 35: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {A rectangular pulse x(t) with amplitude Ax and duration Tx is applied to the input. <br>Which statements hold for the output pulse y(t)? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The output pulse y(t) is triangular in shape. |
| − | - | + | - The amplitudes at the input and output are the same ⇒ Ay=Ax. |
| − | + | + | + The pulse duration is not changed by the system ⇒ Ty=Tx. |
| − | { | + | {Compute the first two coefficients of the Taylor series. |
|type="{}"} | |type="{}"} | ||
c1 = { 0.5 3% } | c1 = { 0.5 3% } | ||
| Line 51: | Line 51: | ||
| − | { | + | {Which distortion factor K is measured with the test signal $x(t) = 1 \hspace{0.08cm} {\rm V} \cdot \cos(\omega_0 \cdot t)$ ? That is: $\underline{A_x = 1\hspace{0.08cm} \rm V}$. |
|type="{}"} | |type="{}"} | ||
K = { 5 3% } % | K = { 5 3% } % | ||
| − | { | + | {Which distortion factor K is measured with the test signal $x(t) = 3 \hspace{0.08cm} {\rm V} \cdot \cos(\omega_0 \cdot t)$ ? That is: $\underline{A_x = 3\hspace{0.08cm} \rm V}$. |
|type="{}"} | |type="{}"} | ||
K = { 15 3% } % | K = { 15 3% } % | ||
| − | { | + | {Which output pulse y(t) arises as a result when the input pulse is triangular? What are the signal values at t=0 and t=Tx/2? |
|type="{}"} | |type="{}"} | ||
y(t=0) = { 1.95 3% } V | y(t=0) = { 1.95 3% } V | ||
| Line 70: | Line 70: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Proposed solution 3</u> is the only correct one: |
| − | * | + | *If the input pulse x(t) is rectangular, then x2(t) is also a rectangle with height A2x between 0 and Tx, outside zero. |
| − | * | + | *The overall output signal y(t) is thus also rectangular with the amplitude |
:Ay=c1⋅Ax+c2⋅A2x. | :Ay=c1⋅Ax+c2⋅A2x. | ||
| − | * | + | *The following holds for the pulse duration: Ty=Tx. |
| − | '''(2)''' | + | |
| + | '''(2)''' The following system of linear equations can be specified with the first two sets of parameters: | ||
:$$c_1 \cdot 1\,{\rm V} + c_2 \cdot (1\,{\rm V})^2 = 0.55\,{\rm | :$$c_1 \cdot 1\,{\rm V} + c_2 \cdot (1\,{\rm V})^2 = 0.55\,{\rm | ||
V},$$ | V},$$ | ||
| Line 85: | Line 86: | ||
V}.\hspace{0.05cm}$$ | V}.\hspace{0.05cm}$$ | ||
| − | + | *The following is obtained by multiplying the first equation by −2 and adding the two equations: | |
:$$c_2 \cdot 2\,{\rm V}^2 = 0.1\,{\rm | :$$c_2 \cdot 2\,{\rm V}^2 = 0.1\,{\rm | ||
| − | V} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} c_2 \hspace{0.15cm}\underline{= 0.05\ | + | V} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} c_2 \hspace{0.15cm}\underline{= 0.05\cdot{1/\rm |
V}}.$$ | V}}.$$ | ||
| − | + | *The linear coefficient is thus c1=0.5_. | |
| − | + | *The third set of parameters can be used to verify the result: | |
:$$c_1 \cdot 3\,{\rm V} + c_2 \cdot (3\,{\rm V})^2 = 0.5 \cdot 3\,{\rm | :$$c_1 \cdot 3\,{\rm V} + c_2 \cdot (3\,{\rm V})^2 = 0.5 \cdot 3\,{\rm | ||
V}+ 0.05 \ {1}/{\rm V}\cdot 9\,{\rm V}^2 = 1.95\,{\rm | V}+ 0.05 \ {1}/{\rm V}\cdot 9\,{\rm V}^2 = 1.95\,{\rm | ||
| Line 97: | Line 98: | ||
| − | '''(3)''' | + | '''(3)''' The specification of a distortion factor requires the use of a harmonic oscillation at the input. |
| − | + | *If X+(f)=1 V⋅δ(f−f0) holds, then the spectrum of the analytic signal at the output is: | |
:Y+(f)=c2/2⋅A2x⋅δ(f)+c1⋅Ax⋅δ(f−f0)+c2/2⋅A2x⋅δ(f−2f0). | :Y+(f)=c2/2⋅A2x⋅δ(f)+c1⋅Ax⋅δ(f−f0)+c2/2⋅A2x⋅δ(f−2f0). | ||
| − | + | *The Dirac function at f=0 follows from the trigonometric transformation cos2(α)=1/2+1/2⋅cos(α). | |
| − | + | *With A1=c1⋅Ax=0.5 V and A2=(c2/2)⋅A2x=0.025 V2 the following is thus obtained for the distortion factor: | |
| − | |||
:K=A2A1=c2/2⋅Axc1=0.0250.5=5%_. | :K=A2A1=c2/2⋅Axc1=0.0250.5=5%_. | ||
| − | '''(4)''' | + | '''(4)''' According to the solution of the last subtask K is proportional to Ax. Therefore, one now obtains K=15%_. |
| + | |||
| − | '''(5)''' | + | '''(5)''' Now the output signal is: |
:$$y(t)= c_1\cdot A_x \cdot \left( 1 - {|\hspace{0.05cm}t\hspace{0.05cm}|}/{T_x}\right) +\hspace{0.1cm} | :$$y(t)= c_1\cdot A_x \cdot \left( 1 - {|\hspace{0.05cm}t\hspace{0.05cm}|}/{T_x}\right) +\hspace{0.1cm} | ||
{c_2}\cdot A_x^2 \cdot \left( 1 - {|\hspace{0.05cm}t\hspace{0.05cm}|}/{T_x}\right)^2.$$ | {c_2}\cdot A_x^2 \cdot \left( 1 - {|\hspace{0.05cm}t\hspace{0.05cm}|}/{T_x}\right)^2.$$ | ||
| − | + | *The following values occur at times t=0 and t=Tx/2: | |
:$$y(t=0) = c_1\cdot A_x + {c_2}\cdot A_x^2 \hspace{0.15cm}\underline{= 1.95\,{\rm | :$$y(t=0) = c_1\cdot A_x + {c_2}\cdot A_x^2 \hspace{0.15cm}\underline{= 1.95\,{\rm | ||
V}},$$ | V}},$$ | ||
| Line 126: | Line 127: | ||
| − | [[Category: | + | [[Category:Linear and Time-Invariant Systems: Exercises|^2.2 Nonlinear Distortions^]] |
Latest revision as of 15:29, 1 October 2021
It is known that the characteristic curve can be represented as follows for a nonlinear system:
- y(t)=c1⋅x(t)+c2⋅x2(t).
Since the distortions are nonlinear no frequency response H(f) can be given.
To determine the dimensionless coefficient c1 as well as the quadratic coefficient c2 different rectangular pulses x(t) – characterized by the amplitude Ax and the width Tx – are now applied to the input and the pulse amplitude Ay at the output is measured in each case.
The first three trials generate the following values:
- Ax=1 V,Tx=8 ms : Ay=0.55 V,
- Ax=2 V,Tx=4 ms : Ay=1.20 V,
- Ax=3 V,Tx=2 ms : Ay=1.95 V.
For the subtasks (3) and (4) let the input signal x(t) be a harmonic oscillation because only for such an oscillation a distortion factor can be specified.
In contrast, a triangular pulse with amplitude Ax=3 V and the one-sided pulse duration Tx=2 ms is considered for the subtask (5) :
- x(t)=Ax⋅(1−|t|/Tx)
Please note:
- The exercise belongs to the chapter Nonlinear Distortions.
- The following abbreviations are used in the formulation of the questions:
- y1(t)=c1⋅x(t),y2(t)=c2⋅x2(t).
Questions
Solution
- If the input pulse x(t) is rectangular, then x2(t) is also a rectangle with height A2x between 0 and Tx, outside zero.
- The overall output signal y(t) is thus also rectangular with the amplitude
- Ay=c1⋅Ax+c2⋅A2x.
- The following holds for the pulse duration: Ty=Tx.
(2) The following system of linear equations can be specified with the first two sets of parameters:
- c1⋅1V+c2⋅(1V)2=0.55V,
- c1⋅2V+c2⋅(2V)2=1.20V.
- The following is obtained by multiplying the first equation by −2 and adding the two equations:
- c2⋅2V2=0.1V⇒c2=0.05⋅1/V_.
- The linear coefficient is thus c1=0.5_.
- The third set of parameters can be used to verify the result:
- c1⋅3V+c2⋅(3V)2=0.5⋅3V+0.05 1/V⋅9V2=1.95V.
(3) The specification of a distortion factor requires the use of a harmonic oscillation at the input.
- If X+(f)=1 V⋅δ(f−f0) holds, then the spectrum of the analytic signal at the output is:
- Y+(f)=c2/2⋅A2x⋅δ(f)+c1⋅Ax⋅δ(f−f0)+c2/2⋅A2x⋅δ(f−2f0).
- The Dirac function at f=0 follows from the trigonometric transformation cos2(α)=1/2+1/2⋅cos(α).
- With A1=c1⋅Ax=0.5 V and A2=(c2/2)⋅A2x=0.025 V2 the following is thus obtained for the distortion factor:
- K=A2A1=c2/2⋅Axc1=0.0250.5=5%_.
(4) According to the solution of the last subtask K is proportional to Ax. Therefore, one now obtains K=15%_.
(5) Now the output signal is:
- y(t)=c1⋅Ax⋅(1−|t|/Tx)+c2⋅A2x⋅(1−|t|/Tx)2.
- The following values occur at times t=0 and t=Tx/2:
- y(t=0)=c1⋅Ax+c2⋅A2x=1.95V_,
- y(t=Tx/2)=c1⋅Ax⋅1/2+c2⋅A2x⋅1/4=0.75V+0.1125V=0.8625V_.