Difference between revisions of "Aufgaben:Exercise 3.4Z: Eye Opening and Level Number"
| (8 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Intersymbol_Interference_for_Multi-Level_Transmission |
}} | }} | ||
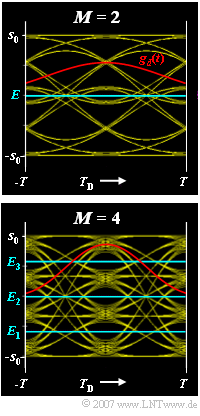
| − | [[File:P_ID1420__Dig_Z_3_4.png|right|frame| | + | [[File:P_ID1420__Dig_Z_3_4.png|right|frame|Binary and quaternary <br>eye diagrams]] |
| − | In | + | In this exercise, a redundancy-free binary system and a redundancy-free quaternary system are compared with respect to vertical eye opening. The same boundary conditions apply to the two transmission systems: |
| − | * | + | * The basic transmission pulse gs(t) is NRZ rectangular in each case and has the height s0=1V. |
| − | * | + | |
| − | * | + | * The (equivalent) bit rate is RB=100Mbit/s. |
| − | * | + | |
| + | * The AWGN noise has the (one-sided) noise power density N0. | ||
| + | |||
| + | * Let the receiver filter be a Gaussian low-pass filter with cutoff frequency fG=30MHz: | ||
:HG(f)=e−π⋅f2/(2fG)2. | :HG(f)=e−π⋅f2/(2fG)2. | ||
| − | * | + | * The decision thresholds are optimal. The detection time is TD=0. |
| − | + | For the half-eye opening of an M-level transmission system, the following holds in general: | |
:¨o(TD)/2=g0M−1−∞∑ν=1|gν|−∞∑ν=1|g−ν|. | :¨o(TD)/2=g0M−1−∞∑ν=1|gν|−∞∑ν=1|g−ν|. | ||
| − | + | *Here, g0=gd(t=0) is the "main value" of the basic detection pulse gd(t)=gs(t)∗hG(t). | |
| + | |||
| + | *The second term describes the trailers ("postcursors") gν=gd(t=νT). | ||
| + | |||
| + | *The last term describes the "precursors" g−ν=gd(t=−νT). | ||
| − | + | ||
| − | * | + | Note that in the present configuration with Gaussian low-pass |
| − | * | + | * all the basic detection pulse values ...g−1,g0,g1,... are positive, |
| − | * | + | |
| + | * the (infinite) sum ...+g−1+g0+g1... gives the constant value s0, | ||
| + | |||
| + | * the main value can be calculated with the complementary Gaussian error function Q(x): | ||
:$$g_0 = s_0 | :$$g_0 = s_0 | ||
\cdot\big [ 1- 2 \cdot {\rm Q} \left( | \cdot\big [ 1- 2 \cdot {\rm Q} \left( | ||
| Line 28: | Line 38: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The graph shows the (noiseless) eye diagrams of the binary and quaternary systems and, in red, the corresponding basic detection pulses gd(t): | |
| − | * | + | *The optimal decision thresholds E (for M=2) and E1, E2, E3 (for M=4) are also drawn. |
| − | |||
| + | *In subtask '''(7)''' these are to be determined numerically. | ||
| − | + | Notes: | |
| − | + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Intersymbol_Interference_for_Multi-Level_Transmission|"Intersymbol Interference for Multi-Level Transmission"]]. | |
| − | * | ||
| − | * | + | *For the complementary Gaussian error function applies: |
:Q(0.25)=0.4013,Q(0.50)=0.3085,Q(0.75)=0.2266,Q(1.00)=0.1587, | :Q(0.25)=0.4013,Q(0.50)=0.3085,Q(0.75)=0.2266,Q(1.00)=0.1587, | ||
:$${\rm Q}(1.25) = 0.1057,\hspace{0.2cm}{\rm Q}(1.50) = 0.0668,\hspace{0.2cm}{\rm Q}(1.75) = 0.0401,\hspace{0.2cm}{\rm Q}(2.00) = | :$${\rm Q}(1.25) = 0.1057,\hspace{0.2cm}{\rm Q}(1.50) = 0.0668,\hspace{0.2cm}{\rm Q}(1.75) = 0.0401,\hspace{0.2cm}{\rm Q}(2.00) = | ||
| Line 46: | Line 55: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the symbol duration T for the binary and the quaternary system? |
|type="{}"} | |type="{}"} | ||
M=2:T = { 10 3% } ns | M=2:T = { 10 3% } ns | ||
M=4:T = { 20 3% } ns | M=4:T = { 20 3% } ns | ||
| − | { | + | {Calculate the main value g0 for the binary system. |
|type="{}"} | |type="{}"} | ||
M=2:g0 = { 0.547 3% } V | M=2:g0 = { 0.547 3% } V | ||
| − | { | + | {Calculate the main value g0 for the quaternary system. |
|type="{}"} | |type="{}"} | ||
M=4:g0 = { 0.867 3% } V | M=4:g0 = { 0.867 3% } V | ||
| − | { | + | {Which equations are valid considering the Gaussian low-pass? |
|type="[]"} | |type="[]"} | ||
+ ¨o(TD)/2=M⋅g0/(M−1)−s0, | + ¨o(TD)/2=M⋅g0/(M−1)−s0, | ||
| Line 67: | Line 76: | ||
+ ¨o(TD)/2=s0/(M−1)⋅[1−2⋅M⋅Q(√2π⋅log2(M)⋅fG/RB)]. | + ¨o(TD)/2=s0/(M−1)⋅[1−2⋅M⋅Q(√2π⋅log2(M)⋅fG/RB)]. | ||
| − | { | + | {What is the eye opening for the binary system? |
|type="{}"} | |type="{}"} | ||
M=2:¨o(TD) = { 0.188 3% } V | M=2:¨o(TD) = { 0.188 3% } V | ||
| − | { | + | {What is the eye opening for the quaternary system? |
|type="{}"} | |type="{}"} | ||
M=4:¨o(TD) = { 0.312 3% } V | M=4:¨o(TD) = { 0.312 3% } V | ||
| − | { | + | {Determine the optimal thresholds of the quaternary system. Enter the lower threshold E1 as a control. |
|type="{}"} | |type="{}"} | ||
M=4:E1 = { -0.595--0.561 } V | M=4:E1 = { -0.595--0.561 } V | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' In the binary system, the bit duration is equal to the reciprocal of the equivalent bit rate: |
:T=1RB=1100Mbit/s=10ns_. | :T=1RB=1100Mbit/s=10ns_. | ||
| − | + | *The symbol duration of the quaternary system is twice as large: | |
:T=log24RB=20ns_. | :T=log24RB=20ns_. | ||
| − | '''(2)''' | + | |
| + | '''(2)''' According to the given equation, the following holds for the binary system: | ||
:$$g_0 \ = \ s_0 \cdot\left [ 1- 2 \cdot {\rm Q} \left( \sqrt{2\pi} \cdot f_{\rm G} \cdot | :$$g_0 \ = \ s_0 \cdot\left [ 1- 2 \cdot {\rm Q} \left( \sqrt{2\pi} \cdot f_{\rm G} \cdot | ||
T \right)\right]= 1\,{\rm V} \cdot\left [ 1- 2 \cdot {\rm Q} \left( \sqrt{2\pi} \cdot 30\,{\rm MHz} \cdot | T \right)\right]= 1\,{\rm V} \cdot\left [ 1- 2 \cdot {\rm Q} \left( \sqrt{2\pi} \cdot 30\,{\rm MHz} \cdot | ||
| Line 97: | Line 107: | ||
| − | '''(3)''' | + | '''(3)''' Due to the double symbol duration, with the same cutoff frequency for M=4: |
:$$g_0 \ = 1\,{\rm V} \cdot\left [ 1- 2 \cdot {\rm Q} \left( 1.5 \right)\right] | :$$g_0 \ = 1\,{\rm V} \cdot\left [ 1- 2 \cdot {\rm Q} \left( 1.5 \right)\right] | ||
= 1\,{\rm V} \cdot\left [ 1- 2 \cdot 0.0668 \right] \hspace{0.15cm}\underline {= 0.867\,{\rm V}} | = 1\,{\rm V} \cdot\left [ 1- 2 \cdot 0.0668 \right] \hspace{0.15cm}\underline {= 0.867\,{\rm V}} | ||
| Line 103: | Line 113: | ||
| − | '''(4)''' | + | '''(4)''' Extending the given equation by $\pm g_0$, we obtain: |
:$${\ddot{o}(T_{\rm D})}/{ 2} = \frac{g_0}{ M-1} + g_0 - g_0 - \sum_{\nu = 1}^{\infty} g_\nu - \sum_{\nu = 1}^{\infty} g_{-\nu} | :$${\ddot{o}(T_{\rm D})}/{ 2} = \frac{g_0}{ M-1} + g_0 - g_0 - \sum_{\nu = 1}^{\infty} g_\nu - \sum_{\nu = 1}^{\infty} g_{-\nu} | ||
= \frac{M}{ M-1} \cdot g_0 - s_0 \hspace{0.05cm}.$$ | = \frac{M}{ M-1} \cdot g_0 - s_0 \hspace{0.05cm}.$$ | ||
| − | + | Here is taken into account: | |
| − | * | + | *In the case of the Gaussian low-pass filter, the magnitude formation can be omitted. |
| − | * | + | *The sum over all detection pulse values is equal to s0. |
| − | + | The <u>first, but also the last solution</u> is correct: | |
:$${\ddot{o}(T_{\rm D})}/{ 2} \ = \ \frac{M}{ M-1} \cdot g_0 - s_0 | :$${\ddot{o}(T_{\rm D})}/{ 2} \ = \ \frac{M}{ M-1} \cdot g_0 - s_0 | ||
= \frac{M}{ M-1} \cdot s_0 \cdot\left [ 1- 2 \cdot {\rm Q} \left( \sqrt{2\pi} \cdot f_{\rm G} \cdot | = \frac{M}{ M-1} \cdot s_0 \cdot\left [ 1- 2 \cdot {\rm Q} \left( \sqrt{2\pi} \cdot f_{\rm G} \cdot | ||
| Line 119: | Line 129: | ||
T \right)\right] | T \right)\right] | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | Using the relation T=log2(M)/RB, we arrive at the third proposed solution, which is also applicable. | |
| − | '''(5)''' | + | '''(5)''' Using the results from '''(2)''' and '''(4)''', one obtains with M=2: |
:$${\ddot{o}(T_{\rm D})} = 2 \cdot (2 \cdot g_0 - s_0) = 2 \cdot (2 \cdot 0.547\,{\rm V} - 1\,{\rm V}) \hspace{0.15cm}\underline {= 0.188\,{\rm V}} | :$${\ddot{o}(T_{\rm D})} = 2 \cdot (2 \cdot g_0 - s_0) = 2 \cdot (2 \cdot 0.547\,{\rm V} - 1\,{\rm V}) \hspace{0.15cm}\underline {= 0.188\,{\rm V}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | '''(6)''' | + | '''(6)''' On the other hand, with g0=0.867V, s0=1V and M=4, we get: |
:$${\ddot{o}(T_{\rm D})} = 2 \cdot ({4}/{3} \cdot 0.867\,{\rm V} - 1\,{\rm V}) \hspace{0.15cm}\underline {= 0.312\,{\rm V}} | :$${\ddot{o}(T_{\rm D})} = 2 \cdot ({4}/{3} \cdot 0.867\,{\rm V} - 1\,{\rm V}) \hspace{0.15cm}\underline {= 0.312\,{\rm V}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | '''(7)''' | + | '''(7)''' According to subtask '''(3)''', g0=0.867V and correspondingly gVN=0.133V (sum of all precursors and trailers). |
| − | * | + | *The eye opening is ¨o=0.312V. |
| − | * | + | *From the sketch on the information section, we can see that the upper boundary (German: "obere Grenzlinie" ⇒ "o") of the upper eye has the following value $($for $T_{\rm D} = 0)$: |
:$$o = s_0 - 2 \cdot g_{\rm VN}= g_0 - g_{\rm VN}= 0.867\,{\rm V} - 0.133\,{\rm V} = 0.734\,{\rm V} | :$$o = s_0 - 2 \cdot g_{\rm VN}= g_0 - g_{\rm VN}= 0.867\,{\rm V} - 0.133\,{\rm V} = 0.734\,{\rm V} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *The lower limit (German: "untere Grenzlinie" ⇒ "u") is at: |
:$$u = o -{\ddot{o}} = 0.734\,{\rm V} - 0.312\,{\rm V} = 0.422\,{\rm V} | :$$u = o -{\ddot{o}} = 0.734\,{\rm V} - 0.312\,{\rm V} = 0.422\,{\rm V} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *From this follows for the optimal decision threshold of the upper eye: |
:$$E_3 = \frac{o + u}{2} = \frac{0.734\,{\rm V} + 0.422\,{\rm V}}{2} { = 0.578\,{\rm V}} | :$$E_3 = \frac{o + u}{2} = \frac{0.734\,{\rm V} + 0.422\,{\rm V}}{2} { = 0.578\,{\rm V}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *The sought threshold (for the lower eye) is E_1 \, \underline {= \, –0.578 \, V}. |
| − | * | + | |
| + | *The average decision threshold is E2=0 for symmetry reasons. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^3.4 Eye Opening with Multilevel Systems^]] |
Latest revision as of 17:09, 20 June 2022
In this exercise, a redundancy-free binary system and a redundancy-free quaternary system are compared with respect to vertical eye opening. The same boundary conditions apply to the two transmission systems:
- The basic transmission pulse gs(t) is NRZ rectangular in each case and has the height s0=1V.
- The (equivalent) bit rate is RB=100Mbit/s.
- The AWGN noise has the (one-sided) noise power density N0.
- Let the receiver filter be a Gaussian low-pass filter with cutoff frequency fG=30MHz:
- HG(f)=e−π⋅f2/(2fG)2.
- The decision thresholds are optimal. The detection time is TD=0.
For the half-eye opening of an M-level transmission system, the following holds in general:
- ¨o(TD)/2=g0M−1−∞∑ν=1|gν|−∞∑ν=1|g−ν|.
- Here, g0=gd(t=0) is the "main value" of the basic detection pulse gd(t)=gs(t)∗hG(t).
- The second term describes the trailers ("postcursors") gν=gd(t=νT).
- The last term describes the "precursors" g−ν=gd(t=−νT).
Note that in the present configuration with Gaussian low-pass
- all the basic detection pulse values ...g−1,g0,g1,... are positive,
- the (infinite) sum ...+g−1+g0+g1... gives the constant value s0,
- the main value can be calculated with the complementary Gaussian error function Q(x):
- g0=s0⋅[1−2⋅Q(√2π⋅fG⋅T)].
The graph shows the (noiseless) eye diagrams of the binary and quaternary systems and, in red, the corresponding basic detection pulses gd(t):
- The optimal decision thresholds E (for M=2) and E1, E2, E3 (for M=4) are also drawn.
- In subtask (7) these are to be determined numerically.
Notes:
- The exercise belongs to the chapter "Intersymbol Interference for Multi-Level Transmission".
- For the complementary Gaussian error function applies:
- Q(0.25)=0.4013,Q(0.50)=0.3085,Q(0.75)=0.2266,Q(1.00)=0.1587,
- Q(1.25)=0.1057,Q(1.50)=0.0668,Q(1.75)=0.0401,Q(2.00)=0.0228.
Questions
Solution
- T=1RB=1100Mbit/s=10ns_.
- The symbol duration of the quaternary system is twice as large:
- T=log24RB=20ns_.
(2) According to the given equation, the following holds for the binary system:
- g0 = s0⋅[1−2⋅Q(√2π⋅fG⋅T)]=1V⋅[1−2⋅Q(√2π⋅30MHz⋅10ns)]
- ⇒g0 ≈ 1V⋅[1−2⋅Q(0.75)]=1V⋅[1−2⋅0.2266]=0.547V_.
(3) Due to the double symbol duration, with the same cutoff frequency for M=4:
- g0 =1V⋅[1−2⋅Q(1.5)]=1V⋅[1−2⋅0.0668]=0.867V_.
(4) Extending the given equation by ±g0, we obtain:
- ¨o(TD)/2=g0M−1+g0−g0−∞∑ν=1gν−∞∑ν=1g−ν=MM−1⋅g0−s0.
Here is taken into account:
- In the case of the Gaussian low-pass filter, the magnitude formation can be omitted.
- The sum over all detection pulse values is equal to s0.
The first, but also the last solution is correct:
- ¨o(TD)/2 = MM−1⋅g0−s0=MM−1⋅s0⋅[1−2⋅Q(√2π⋅fG⋅T)]−s0
- ⇒¨o(TD)/2 = s0M−1⋅[1−2⋅M⋅Q(√2π⋅fG⋅T)].
Using the relation T=log2(M)/RB, we arrive at the third proposed solution, which is also applicable.
(5) Using the results from (2) and (4), one obtains with M=2:
- ¨o(TD)=2⋅(2⋅g0−s0)=2⋅(2⋅0.547V−1V)=0.188V_.
(6) On the other hand, with g0=0.867V, s0=1V and M=4, we get:
- ¨o(TD)=2⋅(4/3⋅0.867V−1V)=0.312V_.
(7) According to subtask (3), g0=0.867V and correspondingly gVN=0.133V (sum of all precursors and trailers).
- The eye opening is ¨o=0.312V.
- From the sketch on the information section, we can see that the upper boundary (German: "obere Grenzlinie" ⇒ "o") of the upper eye has the following value (for TD=0):
- o=s0−2⋅gVN=g0−gVN=0.867V−0.133V=0.734V.
- The lower limit (German: "untere Grenzlinie" ⇒ "u") is at:
- u=o−¨o=0.734V−0.312V=0.422V.
- From this follows for the optimal decision threshold of the upper eye:
- E3=o+u2=0.734V+0.422V2=0.578V.
- The sought threshold (for the lower eye) is E1=–0.578V_.
- The average decision threshold is E2=0 for symmetry reasons.