Difference between revisions of "Aufgaben:Exercise 2.1Z: Different Signal Courses"
| (12 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/From_Random_Experiment_to_Random_Variable |
}} | }} | ||
| − | [[File:P_ID59__Sto_Z_2_1.png|right|frame| | + | [[File:P_ID59__Sto_Z_2_1.png|right|frame|Discrete-value or continuous-value?]] |
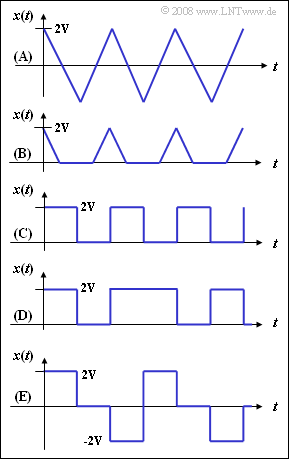
| − | + | On the right are shown five signals. The first three signals $\rm (A)$, $\rm (B)$ and $\rm (C)$ are periodic and thus also deterministic, the two lower signals have stochastic character. The current value of these signals $x(t)$ is taken as a random variable in each case. | |
| − | + | Shown in detail are: | |
| − | $\rm (A)$: | + | $\rm (A)$: A triangular-shaped periodic signal, |
| − | $\rm (B)$: | + | $\rm (B)$: the signal $\rm (A)$ after one-way rectification, |
| − | $\rm (C)$: | + | $\rm (C)$: a rectangular periodic signal, |
| − | $\rm (D)$: | + | $\rm (D)$: a rectangular random signal, |
| − | $\rm (E)$: | + | $\rm (E)$: the random signal $\rm (D)$ according to AMI coding; <br> here the "zero" is preserved, while each "one" is alternately encoded with $+2\hspace{0.03cm}\rm V$ and $-2\hspace{0.03cm} \rm V$. |
| Line 24: | Line 24: | ||
| − | + | Hints: | |
| − | * | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/From_Random_Experiment_to_Random_Variable|From Random Experiment to Random Variable]]. |
| Line 31: | Line 31: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {For which signals does the current value describe a discrete random variable? <br>Consider also the respective number of steps ⇒ $M$. |
|type="[]"} | |type="[]"} | ||
- Signal $\rm (A)$, | - Signal $\rm (A)$, | ||
| − | - | + | - signal $\rm (B)$, |
| − | + | + | + signal $\rm (C)$, |
| − | + | + | + signal $\rm (D)$, |
| − | + | + | + signal $\rm (E)$. |
| − | { | + | {For which signals is the current value (exclusively) a continuous random variable? |
|type="[]"} | |type="[]"} | ||
+ Signal $\rm (A)$, | + Signal $\rm (A)$, | ||
| − | - | + | - signal $\rm (B)$, |
| − | - | + | - signal $\rm (C)$, |
| − | - | + | - signal $\rm (D)$, |
| − | - | + | - signal $\rm (E)$. |
| − | { | + | {Which random variables have a discrete and a continuous part? |
|type="[]"} | |type="[]"} | ||
- Signal $\rm (A)$, | - Signal $\rm (A)$, | ||
| − | + | + | + signal $\rm (B)$, |
| − | - | + | - signal $\rm (C)$, |
| − | - | + | - signal $\rm (D)$, |
| − | - | + | - signal $\rm (E)$. |
| − | { | + | {For the signal $\rm (D)$ the relative frequency $h_0$ is determined empirically over $100\hspace{0.03cm}000$ binary symbols. <br>Name a lower bound for the probability that the determined value lies between $0.49$ and $0.51$ ? |
|type="{}"} | |type="{}"} | ||
| − | ${\rm Min\big[\ Pr(0.49}≤h_0≤0.51)\ \big] \ = \ $ { | + | ${\rm Min\big[\ Pr(0.49}≤h_0≤0.51)\ \big] \ = \ $ { 97.5 3% } $\%$ |
| − | { | + | {How many symbols $(N_\min)$ would you need to use for this investigation to ensure <br>that the probability for the event "The frequency so determined is between $0.499$ and $0.501$" is greater than $99\%$ ? |
|type="{}"} | |type="{}"} | ||
| − | $N_\min \ = | + | $N_\min \ = \ $ { 2.5 3% } $\ \cdot 10^9$ |
| Line 74: | Line 74: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct are <u>suggested solutions 3, 4, and 5</u>: |
| − | * | + | *The random variables $\rm (C)$ and $\rm (D)$ are binary $(M= 2)$, |
| − | * | + | *while the random variable $\rm (E)$ is trivalent $(M= 3)$. |
| − | |||
| − | |||
| − | |||
| + | '''(2)''' The <u>proposed solution 1</u> alone is correct: | ||
| + | *The random variable $\rm (A)$ is continuous in value and can take all values between $\pm 2 \hspace{0.03cm} \rm V$ with equal probability. | ||
| + | *All other random variables are discrete in value. | ||
| − | |||
| − | |||
| − | |||
| − | '''( | + | '''(3)''' The <u>proposed solution 2</u> alone is correct: |
| + | *Only the random variable $\rm (B)$ has a discrete part at $0\hspace{0.03cm}\rm V$, and | ||
| + | *also has a continuous component (between $0\hspace{0.03cm} \rm V$ and $+2\hspace{0.03cm}\rm V)$. | ||
| + | |||
| + | |||
| + | '''(4)''' According to Bernoulli's law of large numbers: | ||
:$$\rm Pr\left(|\it h_{\rm 0} - \it p_{\rm 0}|\ge\it\varepsilon\right)\le\frac{\rm 1}{\rm 4\cdot \it N\cdot\it\varepsilon^{\rm 2}} = {\it p}_{\rm \hspace{0.01cm}Bernouilli}.$$ | :$$\rm Pr\left(|\it h_{\rm 0} - \it p_{\rm 0}|\ge\it\varepsilon\right)\le\frac{\rm 1}{\rm 4\cdot \it N\cdot\it\varepsilon^{\rm 2}} = {\it p}_{\rm \hspace{0.01cm}Bernouilli}.$$ | ||
| − | + | *Thus, the probability that the relative frequency $h_0$ deviates from the probability $p_0 = 0.5$ by more than $0.01$ can be calculated as $\varepsilon = 0.01$: | |
:$${\it p}_{\rm \hspace{0.01cm}Bernoulli} = \rm\frac{1}{4\cdot 100000\cdot 0.01^2}=\rm 2.5\% \hspace{0.5cm}\Rightarrow \hspace{0.5cm} | :$${\it p}_{\rm \hspace{0.01cm}Bernoulli} = \rm\frac{1}{4\cdot 100000\cdot 0.01^2}=\rm 2.5\% \hspace{0.5cm}\Rightarrow \hspace{0.5cm} | ||
| − | {\rm Min}\big[({\rm Pr}(0.49 \le h_0 \le 0.51)\big] \hspace{0.15cm}\underline{= | + | {\rm Min}\big[({\rm Pr}(0.49 \le h_0 \le 0.51)\big] \hspace{0.15cm}\underline{= 97.5\%}.$$ |
| + | |||
| − | '''(5)''' | + | '''(5)''' With $p_{\rm Bernoulli} = 1 - 0.99 = 0.01$ and $\varepsilon = 0.001$ holds again by the law of large numbers: |
| − | :$${\it p}_{\rm \hspace{0.01cm}Bernoulli}\le\frac{\rm 1}{\rm 4\cdot | + | :$${\it p}_{\rm \hspace{0.01cm}Bernoulli}\le\frac{\rm 1}{\rm 4\cdot \it N\cdot\it \varepsilon^{\rm 2}}.$$ |
| − | + | *Solved for $N$, one gets: | |
| − | :$$N\ge\frac{\rm 1}{\rm 4\cdot\it p_{\rm \hspace{0.01cm}Bernoulli}\cdot | + | :$$N\ge\frac{\rm 1}{\rm 4\cdot\it p_{\rm \hspace{0.01cm}Bernoulli}\cdot\varepsilon^{\rm 2}}=\rm \frac{1}{4\cdot 0.01\cdot 0.001^{2}}=\rm 0.25\cdot 10^8 |
\hspace{0.5cm}\Rightarrow \hspace{0.5cm} | \hspace{0.5cm}\Rightarrow \hspace{0.5cm} | ||
{\it N}_{\rm min} \hspace{0.15cm}\underline{= 2.5\cdot 10^9}.$$ | {\it N}_{\rm min} \hspace{0.15cm}\underline{= 2.5\cdot 10^9}.$$ | ||
| Line 109: | Line 112: | ||
| − | [[Category: | + | [[Category:Theory of Stochastic Signals: Exercises|^2.1 From Experiment to Random Variable^]] |
Latest revision as of 14:02, 3 December 2021
On the right are shown five signals. The first three signals $\rm (A)$, $\rm (B)$ and $\rm (C)$ are periodic and thus also deterministic, the two lower signals have stochastic character. The current value of these signals $x(t)$ is taken as a random variable in each case.
Shown in detail are:
$\rm (A)$: A triangular-shaped periodic signal,
$\rm (B)$: the signal $\rm (A)$ after one-way rectification,
$\rm (C)$: a rectangular periodic signal,
$\rm (D)$: a rectangular random signal,
$\rm (E)$: the random signal $\rm (D)$ according to AMI coding;
here the "zero" is preserved, while each "one" is alternately encoded with $+2\hspace{0.03cm}\rm V$ and $-2\hspace{0.03cm} \rm V$.
Hints:
- The exercise belongs to the chapter From Random Experiment to Random Variable.
Questions
Solution
- The random variables $\rm (C)$ and $\rm (D)$ are binary $(M= 2)$,
- while the random variable $\rm (E)$ is trivalent $(M= 3)$.
(2) The proposed solution 1 alone is correct:
- The random variable $\rm (A)$ is continuous in value and can take all values between $\pm 2 \hspace{0.03cm} \rm V$ with equal probability.
- All other random variables are discrete in value.
(3) The proposed solution 2 alone is correct:
- Only the random variable $\rm (B)$ has a discrete part at $0\hspace{0.03cm}\rm V$, and
- also has a continuous component (between $0\hspace{0.03cm} \rm V$ and $+2\hspace{0.03cm}\rm V)$.
(4) According to Bernoulli's law of large numbers:
- $$\rm Pr\left(|\it h_{\rm 0} - \it p_{\rm 0}|\ge\it\varepsilon\right)\le\frac{\rm 1}{\rm 4\cdot \it N\cdot\it\varepsilon^{\rm 2}} = {\it p}_{\rm \hspace{0.01cm}Bernouilli}.$$
- Thus, the probability that the relative frequency $h_0$ deviates from the probability $p_0 = 0.5$ by more than $0.01$ can be calculated as $\varepsilon = 0.01$:
- $${\it p}_{\rm \hspace{0.01cm}Bernoulli} = \rm\frac{1}{4\cdot 100000\cdot 0.01^2}=\rm 2.5\% \hspace{0.5cm}\Rightarrow \hspace{0.5cm} {\rm Min}\big[({\rm Pr}(0.49 \le h_0 \le 0.51)\big] \hspace{0.15cm}\underline{= 97.5\%}.$$
(5) With $p_{\rm Bernoulli} = 1 - 0.99 = 0.01$ and $\varepsilon = 0.001$ holds again by the law of large numbers:
- $${\it p}_{\rm \hspace{0.01cm}Bernoulli}\le\frac{\rm 1}{\rm 4\cdot \it N\cdot\it \varepsilon^{\rm 2}}.$$
- Solved for $N$, one gets:
- $$N\ge\frac{\rm 1}{\rm 4\cdot\it p_{\rm \hspace{0.01cm}Bernoulli}\cdot\varepsilon^{\rm 2}}=\rm \frac{1}{4\cdot 0.01\cdot 0.001^{2}}=\rm 0.25\cdot 10^8 \hspace{0.5cm}\Rightarrow \hspace{0.5cm} {\it N}_{\rm min} \hspace{0.15cm}\underline{= 2.5\cdot 10^9}.$$