Difference between revisions of "Aufgaben:Exercise 1.7: PDF of Rice Fading"
| (12 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Mobile_Communications/Non-Frequency_Selective_Fading_With_Direct_Component}} |
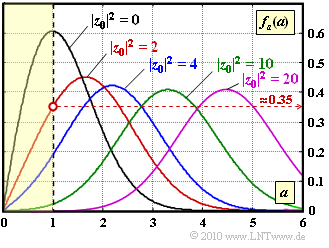
[[File:P_ID2133__Mob_A_1_7.png|right|frame| Rice fading for different values of |z0|2]] | [[File:P_ID2133__Mob_A_1_7.png|right|frame| Rice fading for different values of |z0|2]] | ||
| − | As you can see in the diagram, we consider the same scenario as in [[Aufgaben:Exercise_1.6: | + | As you can see in the diagram, we consider the same scenario as in [[Aufgaben:Exercise_1.6:_Autocorrelation_Function_and_PDS_with_Rice_Fading| Exercise 1.6]]: |
| − | * | + | * Rice fading with variance of the Gaussian processes σ2=1 and parameter |z0| for the direct path. |
* Regarding direct path, we are interested in the parameter values |z0|2=0, 2, 4, 10, 20 (see graph). | * Regarding direct path, we are interested in the parameter values |z0|2=0, 2, 4, 10, 20 (see graph). | ||
| − | * The PDF of the magnitude a(t)=|z(t)| is | + | * The PDF of the magnitude a(t)=|z(t)| is |
| − | :$$f_a(a) = | + | :$$f_a(a) ={a}/{\sigma^2} \cdot {\rm e}^{ -{(a^2+ |z_0|^2) }/({2\sigma^2})}\cdot {\rm I}_0 \left [ {a \cdot |z_0|}/{\sigma^2} \right ]\hspace{0.05cm}.$$ |
* For example, the modified zeroth order Bessel function returns the following values: | * For example, the modified zeroth order Bessel function returns the following values: | ||
| − | $${\rm I }_0 (2) = 2.28\hspace{0.05cm},\hspace{0.2cm}{\rm I }_0 (4) = 11.30\hspace{0.05cm},\hspace{0.2cm}{\rm I }_0 (3) = 67.23 | + | :$${\rm I }_0 (2) = 2.28\hspace{0.05cm},\hspace{0.2cm}{\rm I }_0 (4) = 11.30\hspace{0.05cm},\hspace{0.2cm}{\rm I }_0 (3) = 67.23 |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
* The power (noncentral second moment) of the multiplicative factor |z(t)| is | * The power (noncentral second moment) of the multiplicative factor |z(t)| is | ||
| − | $${\rm E}\left [ a^2 \right ] = {\rm E}\left [ |z(t)|^2 \right ] = 2 \cdot \sigma^2 + |z_0|^2 | + | :$${\rm E}\left [ a^2 \right ] = {\rm E}\left [ |z(t)|^2 \right ] = 2 \cdot \sigma^2 + |z_0|^2 |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * With z0=0, the | + | * With z0=0, the Rice fading becomes Rayleigh fading, which is more critical. In this case, the probability that a lies in the yellow-shaded area between 0 and 1 is |
| − | $$ {\rm Pr}(a \le 1) = 1 - {\rm e}^{-0.5/\sigma^2} \approx 0.4 | + | :$$ {\rm Pr}(a \le 1) = 1 - {\rm e}^{-0.5/\sigma^2} \approx 0.4 |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
In this task the probability {\rm Pr}(a ≤ 1) for |z_0| ≠ 0 is to be approximated. There are two ways to do this, namely: | In this task the probability {\rm Pr}(a ≤ 1) for |z_0| ≠ 0 is to be approximated. There are two ways to do this, namely: | ||
| − | * the | + | * the triangular approximation: ${\rm Pr}(a \le 1) = {1}/{2} \cdot f_a(a=1) |
| − | + | \hspace{0.05cm}.$ | |
| − | \hspace{0.05cm}. | + | * the Gaussian approximation: If |z0|≫σ, then the Rice distribution can be approximated by a Gaussian distribution with mean |z0| and standard deviation σ . |
| − | * the | ||
| Line 30: | Line 29: | ||
''Notes:'' | ''Notes:'' | ||
| − | * This task belongs to chapter [[ | + | * This task belongs to chapter [[Mobile_Communications/Non-frequency_selective_fading_with_direct_component| Non-frequency selective fading with direct component]]. |
| − | * For the numerical solutions of the last subtasks, we recommend the | + | * For the numerical solutions of the last subtasks, we recommend the applet [[Applets:Complementary_Gaussian_Error_Functions|Complementary Gaussian Error Functions]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | {Calculate some PDF values for $|z_0| = | + | {Calculate some PDF values for $|z_0| = 2 and \sigma = 1$: |
|type="{}"} | |type="{}"} | ||
fa(a=1) = { 0.187 3% } | fa(a=1) = { 0.187 3% } | ||
| Line 44: | Line 43: | ||
fa(a=3) = { 0.303 3% } | fa(a=3) = { 0.303 3% } | ||
| − | {Let |z0|=2 ⇒ |z0|2=4 | + | {Let |z0|=2 ⇒ |z0|2=4 '''(blue curve)'''. How big is {\rm Pr}(a ≤ 1)? Use the '''triangular approximation'''. |
|type="{}"} | |type="{}"} | ||
{\rm Pr}(a ≤ 1)\ = \{ 9.4 3% } % | {\rm Pr}(a ≤ 1)\ = \{ 9.4 3% } % | ||
| − | {Let |z0|2=2 | + | {Let |z0|2=2 '''(red curve)'''. How big is {\rm Pr}(a ≤ 1)? Use the '''triangular approximation'''. |
|type="{}"} | |type="{}"} | ||
{\rm Pr}(a ≤ 1) \ = \{ 17.5 3% } % | {\rm Pr}(a ≤ 1) \ = \{ 17.5 3% } % | ||
| − | {Let |z0|2=10 | + | {Let |z0|2=10 '''(green curve)'''. How big is {\rm Pr}(a ≤ 1)? Use the '''Gaussian approximation'''. |
|type="{}"} | |type="{}"} | ||
{\rm Pr}(a ≤ 1) \ = \{ 1.5 3% } % | {\rm Pr}(a ≤ 1) \ = \{ 1.5 3% } % | ||
| − | {Let |z0|2=20 | + | {Let |z0|2=20 '''(purple curve)'''. How big is {\rm Pr}(a ≤ 1)? Use the '''Gaussian approximation'''. |
|type="{}"} | |type="{}"} | ||
{\rm Pr}(a ≤ 1) \ = \{ 0.02 3% } % | {\rm Pr}(a ≤ 1) \ = \{ 0.02 3% } % | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' With |z0|=2 and $\sigma = | + | '''(1)''' With |z0|=2 and $\sigma = 1$ the Rice PDF is |
| − | fa(a)=a⋅exp[−a2+42]⋅I0(2a). | + | :fa(a)=a⋅exp[−a2+42]⋅I0(2a). |
*This gives the desired values: | *This gives the desired values: | ||
| Line 75: | Line 74: | ||
| − | '''(2)''' With the result of the subtask '''(1)''' ⇒ fa(a=1)=0.187 the triangle approximation gives | + | '''(2)''' With the result of the subtask '''(1)''' ⇒ fa(a=1)=0.187 the triangle approximation gives |
| − | Pr(a≤1)=1/2⋅0.187⋅1≈9.4%_. | + | :Pr(a≤1)=1/2⋅0.187⋅1≈9.4%_. |
| + | |||
| + | *This result will be a bit too large, because the blue curve is below the connecting line from (0,0) to (1,0.187) ⇒ convex curve. | ||
| − | |||
| − | '''(3)''' For the red curve the | + | '''(3)''' For the red curve the PDF value fa(a=1)≈0.35 can be read from the graph: |
| − | Pr(a≤1)=12⋅0.35≈17.5%_. | + | :Pr(a≤1)=12⋅0.35≈17.5%_. |
| − | *The actual probability value will be slightly larger because the red curve is concave in the range between 0 and 1. | + | *The actual probability value will be slightly larger because the red curve is concave in the range between 0 and 1. |
| − | '''(4)''' The Gaussian approximation states that one can approximate the Rice distribution by a Gaussian distribution with mean |z0|=√10=3.16 and standard deviation σ=1 if the quotient |z0|/σ is sufficiently large. Then we have | + | '''(4)''' The Gaussian approximation states that one can approximate the Rice distribution by a Gaussian distribution with mean |z0|=√10=3.16 and standard deviation σ=1 if the quotient |z0|/σ is sufficiently large. Then we have |
| − | Pr(a≤1)≈Pr(g≤−2.16)=Q(2.16)≈1.5%_. | + | :Pr(a≤1)≈Pr(g≤−2.16)=Q(2.16)≈1.5%_. |
| − | *Here, g denotes a Gaussian distributed random variable with mean 0 and standard deviation σ=1. | + | *Here, g denotes a Gaussian distributed random variable with mean 0 and standard deviation σ=1. |
| − | *The numerical value was determined with the specified interactive [[Applets: | + | *The numerical value was determined with the specified interactive applet [[Applets:Complementary_Gaussian_Error_Functions|Complementary Gaussian Error Functions]]. |
<i>Note:</i> The Gaussian approximation is certainly associated with a certain error here: | <i>Note:</i> The Gaussian approximation is certainly associated with a certain error here: | ||
| − | *From the graph you can see that the average value of the green curve is not a=3.16 , but rather 3.31. | + | *From the graph you can see that the average value of the green curve is not a=3.16, but rather 3.31. |
| − | *Then the power of the Gaussian approximation (3.312+12=12) is exactly the same as that of the Rice distribution: | + | *Then the power of the Gaussian approximation (3.312+12=12) is exactly the same as that of the Rice distribution: |
:|z0|2+2σ2=10+2=12. | :|z0|2+2σ2=10+2=12. | ||
| − | '''(5)''' Using the same calculation method, replace the Rice PDF with a Gaussian PDF with mean value $\sqrt{20} \approx | + | '''(5)''' Using the same calculation method, replace the Rice PDF with a Gaussian PDF with mean value $\sqrt{20} \approx 4.47$ and standard deviation $\sigma = 1$ and you get |
| − | Pr(a≤1)≈Pr(g≤−3.37)=Q(3.37)≈0.04%. | + | :Pr(a≤1)≈Pr(g≤−3.37)=Q(3.37)≈0.04%. |
| − | *If one assumes the equal power Gaussian distribution (see the note to the last subtask), the mean value is mg=√21≈4.58, and the probability would then be | + | *If one assumes the equal power Gaussian distribution (see the note to the last subtask), the mean value is mg=√21≈4.58, and the probability would then be |
| − | Pr(a≤1)≈Q(3.58)≈0.02%_. | + | :Pr(a≤1)≈Q(3.58)≈0.02%_. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 110: | Line 110: | ||
| − | [[Category: | + | [[Category:Mobile Communications: Exercises|^1.4 Fading with Direct Path Component^]] |
Latest revision as of 10:19, 7 July 2021
As you can see in the diagram, we consider the same scenario as in Exercise 1.6:
- Rice fading with variance of the Gaussian processes σ2=1 and parameter |z0| for the direct path.
- Regarding direct path, we are interested in the parameter values |z0|2=0, 2, 4, 10, 20 (see graph).
- The PDF of the magnitude a(t)=|z(t)| is
- fa(a)=a/σ2⋅e−(a2+|z0|2)/(2σ2)⋅I0[a⋅|z0|/σ2].
- For example, the modified zeroth order Bessel function returns the following values:
- I0(2)=2.28,I0(4)=11.30,I0(3)=67.23.
- The power (noncentral second moment) of the multiplicative factor |z(t)| is
- E[a2]=E[|z(t)|2]=2⋅σ2+|z0|2.
- With z0=0, the Rice fading becomes Rayleigh fading, which is more critical. In this case, the probability that a lies in the yellow-shaded area between 0 and 1 is
- Pr(a≤1)=1−e−0.5/σ2≈0.4.
In this task the probability Pr(a≤1) for |z0|≠0 is to be approximated. There are two ways to do this, namely:
- the triangular approximation: Pr(a≤1)=1/2⋅fa(a=1).
- the Gaussian approximation: If |z0|≫σ, then the Rice distribution can be approximated by a Gaussian distribution with mean |z0| and standard deviation σ .
Notes:
- This task belongs to chapter Non-frequency selective fading with direct component.
- For the numerical solutions of the last subtasks, we recommend the applet Complementary Gaussian Error Functions.
Questions
Solution
- fa(a)=a⋅exp[−a2+42]⋅I0(2a).
- This gives the desired values:
- fa(a=1) = 1⋅e−2.5⋅I0(2)=0.082⋅2.28=0.187_,
- fa(a=2) = 2⋅e−4⋅I0(4)=2⋅0.0183⋅11.3=0.414_,
- fa(a=3) = 3⋅e−6.5⋅I0(6)=3⋅0.0015⋅67.23=0.303_.
- The results fit well with the blue curve on the graph.
(2) With the result of the subtask (1) ⇒ fa(a=1)=0.187 the triangle approximation gives
- Pr(a≤1)=1/2⋅0.187⋅1≈9.4%_.
- This result will be a bit too large, because the blue curve is below the connecting line from (0,0) to (1,0.187) ⇒ convex curve.
(3) For the red curve the PDF value fa(a=1)≈0.35 can be read from the graph:
- Pr(a≤1)=12⋅0.35≈17.5%_.
- The actual probability value will be slightly larger because the red curve is concave in the range between 0 and 1.
(4) The Gaussian approximation states that one can approximate the Rice distribution by a Gaussian distribution with mean |z0|=√10=3.16 and standard deviation σ=1 if the quotient |z0|/σ is sufficiently large. Then we have
- Pr(a≤1)≈Pr(g≤−2.16)=Q(2.16)≈1.5%_.
- Here, g denotes a Gaussian distributed random variable with mean 0 and standard deviation σ=1.
- The numerical value was determined with the specified interactive applet Complementary Gaussian Error Functions.
Note: The Gaussian approximation is certainly associated with a certain error here:
- From the graph you can see that the average value of the green curve is not a=3.16, but rather 3.31.
- Then the power of the Gaussian approximation (3.312+12=12) is exactly the same as that of the Rice distribution:
- |z0|2+2σ2=10+2=12.
(5) Using the same calculation method, replace the Rice PDF with a Gaussian PDF with mean value √20≈4.47 and standard deviation σ=1 and you get
- Pr(a≤1)≈Pr(g≤−3.37)=Q(3.37)≈0.04%.
- If one assumes the equal power Gaussian distribution (see the note to the last subtask), the mean value is mg=√21≈4.58, and the probability would then be
- Pr(a≤1)≈Q(3.58)≈0.02%_.