Difference between revisions of "Aufgaben:Exercise 2.4: Rectified Cosine"
m (Text replacement - "Signal_Representation/Fourierreihe" to "Signal_Representation/Fourier_Series") |
|||
| (12 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal_Representation/Fourier_Series |
}} | }} | ||
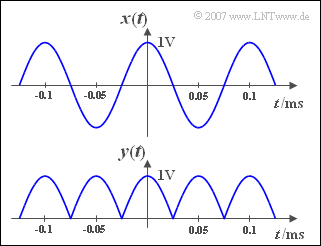
| − | [[File:P_ID300__Sig_A_2_4.png|right|frame| | + | [[File:P_ID300__Sig_A_2_4.png|right|frame|Cosine and rectified cosine]] |
| − | + | A cosine signal x(t) with amplitude 1V and frequency f0=10kHz is applied to the input of a half-wave rectifier. At its output, the signal y(t) results, which is shown in the graph below. | |
| − | + | In subtasks '''(6)''' and '''(7)''' the error signal ε3(t)=y3(t)−y(t) is also used. This describes the difference between the Fourier series ⇒ y3(t) limited to only N=3 coefficients and the actual output signal y(t). | |
| Line 15: | Line 15: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Signal_Representation/Fourier_Series|Fourier Series]]. |
| − | * | + | *To solve the problem, you can use the following definite integral $(letn$ be an integer): |
:∫π/2−π/2cos(u)⋅cos(2nu)du=(−1)n+1⋅24n2−1. | :∫π/2−π/2cos(u)⋅cos(2nu)du=(−1)n+1⋅24n2−1. | ||
| − | * | + | *You can find a compact summary of the topic in the (German language) learning video<br> [[Zur_Berechnung_der_Fourierkoeffizienten_(Lernvideo)|Zur Berechnung der Fourierkoeffizienten]] ⇒ "To calculate the Fourier coefficients". |
| − | === | + | |
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements are true for the signal x(t) ? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The period duration is T_0 = 100 \,µ{\rm s}. |
| − | + | + | + The DC signal coefficient is A0=0. |
| − | + | + | + Of all cosine coefficients An exactly one is not equal to zero. |
| − | - | + | - Of all the sine coefficients Bn exactly one is not equal to zero. |
| − | + | + | + The Fourier series x3(t) does not deviate from the actual signal x(t) . |
| − | { | + | {What is the period duration of the signal y(t)? |
|type="{}"} | |type="{}"} | ||
T0 = { 50 3% } {\rm µs} | T0 = { 50 3% } {\rm µs} | ||
| − | { | + | {Calculate the DC component of the signal y(t). |
|type="{}"} | |type="{}"} | ||
A0 = { 0.637 3% } V | A0 = { 0.637 3% } V | ||
| − | { | + | {What are the sine coefficients Bn? Justify your result. Enter the coefficient B2 as a check. |
|type="{}"} | |type="{}"} | ||
B2 = { 0. } V | B2 = { 0. } V | ||
| − | { | + | {Now calculate the cosine coefficient An. Enter the coefficient A2 as a check. |
|type="{}"} | |type="{}"} | ||
A2 = { -0.087--0.083 } V | A2 = { -0.087--0.083 } V | ||
| − | { | + | {Specify the Fourier series y3(t) analytically $($limit to N=3 sine and cosine coefficients each$)$. |
| − | <br> | + | <br>How large is the error between this finite Fourier series and the actual signal value at t=0? |
|type="{}"} | |type="{}"} | ||
ε3(t=0) = { 0.0125 3% } V | ε3(t=0) = { 0.0125 3% } V | ||
| − | { | + | {Now calculate the error \varepsilon_3(t= 25 \,µ{\rm s}). Interpret this value in comparison to the result from '''(6)'''. |
|type="{}"} | |type="{}"} | ||
\varepsilon_3(t= 25 \,µ{\rm s})\ = \ { 0.091 3% } V | \varepsilon_3(t= 25 \,µ{\rm s})\ = \ { 0.091 3% } V | ||
| Line 66: | Line 68: | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' All solutions are correct except the fourth: |
| − | * | + | *From the signal frequency f0=10kHz follows T_0 = 1/f_0 = 100\,µ\text{s}. |
| − | * | + | *The cosine signal is mean–free (A0=0) and it is completely described by a single cosine coefficient – namely A1 . |
| − | * | + | *All sine coefficients are Bn≡0, since x(t) is an even function. |
| − | * | + | *The Fourier series representation x3(t) reproduces x(t) without error. |
| − | '''(2)''' | + | '''(2)''' Due to the double path rectification, the period duration is now half the value: T_0 \hspace{0.1cm}\underline{= 50\,µ\text{s}}. |
| − | * | + | *For all subsequent points, the specification T0 refers to this value, i.e. to the period of the signal y(t). |
| − | '''(3)''' | + | '''(3)''' In the range from –T_0/2 to +T_0/2 \ (–25\,µ\text{s} \ \text{...} +25\,µ\text{s}) is y(t) = x(t). With f_x= 10\,\rm{kHz} = 1/(2T_0) therefore applies to this section: |
:y(t)={\rm 1V}\cdot\cos(2{\pi} f_0\hspace{0.05cm}t)={\rm 1V}\cdot\cos(\pi \cdot {t}/{T_0}). | :y(t)={\rm 1V}\cdot\cos(2{\pi} f_0\hspace{0.05cm}t)={\rm 1V}\cdot\cos(\pi \cdot {t}/{T_0}). | ||
| − | * | + | *This results in the following for the DC coefficient: |
:A_0=\frac{1}{T_0}\int^{T_0/2}_{-T_0/2}y(t)\,{\rm d} t=\frac{1}{T_0}\int^{T_0/2}_{-T_0/2}{\rm 1V}\cdot\cos(\pi\cdot {t}/{T_0})\,{\rm d}t. | :A_0=\frac{1}{T_0}\int^{T_0/2}_{-T_0/2}y(t)\,{\rm d} t=\frac{1}{T_0}\int^{T_0/2}_{-T_0/2}{\rm 1V}\cdot\cos(\pi\cdot {t}/{T_0})\,{\rm d}t. | ||
| − | * | + | *With the substitution u = \pi \cdot t/T_0 one obtains: |
:A_0=\left. \frac{ {\rm 1V}}{\pi}\int_{-\pi /2}^{\pi/2}\cos(u)\,{\rm d}u=\frac{ {\rm 1V}}{\pi}\sin(u)\; \right| _{-\pi/2}^{\pi/2}=\frac{ {\rm 1V}\cdot 2}{\pi} \hspace{0.15cm}\underline{\approx 0.637\;{\rm V}}. | :A_0=\left. \frac{ {\rm 1V}}{\pi}\int_{-\pi /2}^{\pi/2}\cos(u)\,{\rm d}u=\frac{ {\rm 1V}}{\pi}\sin(u)\; \right| _{-\pi/2}^{\pi/2}=\frac{ {\rm 1V}\cdot 2}{\pi} \hspace{0.15cm}\underline{\approx 0.637\;{\rm V}}. | ||
| − | '''(4)''' | + | '''(4)''' Since y(–t) = y(t) holds, all sine coefficients B_n = 0. Thus B_2 \hspace{0.1cm}\underline{= 0} also holds. |
| − | '''(5)''' | + | '''(5)''' For the coefficients A_n applies with the substitution u = \pi \cdot t/T_0 according to the given integral: |
:$$A_n = \frac{2{\rm V}}{T_0}\int_{-T_0/2}^{T_0/2}\cos(\pi\frac{t}{T_0})\cdot \cos(n\cdot 2\pi\frac{t}{T_0})\,{\rm d}t = \frac{2{\rm V}}{\pi}\int_{-\pi/2}^{\pi/2}\cos(u)\cdot \cos(2n u)\,{\rm d}u \quad | :$$A_n = \frac{2{\rm V}}{T_0}\int_{-T_0/2}^{T_0/2}\cos(\pi\frac{t}{T_0})\cdot \cos(n\cdot 2\pi\frac{t}{T_0})\,{\rm d}t = \frac{2{\rm V}}{\pi}\int_{-\pi/2}^{\pi/2}\cos(u)\cdot \cos(2n u)\,{\rm d}u \quad | ||
\Rightarrow \quad A_n = \left( { - 1} \right)^{n + 1} \frac{{4\;{\rm{V}}}}{{{\rm{\pi }}\left( {4n^2 - 1} \right)}}.$$ | \Rightarrow \quad A_n = \left( { - 1} \right)^{n + 1} \frac{{4\;{\rm{V}}}}{{{\rm{\pi }}\left( {4n^2 - 1} \right)}}.$$ | ||
| − | + | The coefficient A_2 is thus equal to -4 \,\text{V}/(15\pi) \hspace{0.1cm}\underline{\approx -\hspace{0.05cm}0.085 \, \text{V}}. | |
| + | |||
| + | '''(6)''' For the finite Fourier series with N = 3 the following applies in general: | ||
| − | |||
| − | |||
:y_3(t)=\frac{2{\rm V}}{\pi} \cdot \left [ 1+{2}/{3} \cdot \cos(\omega_0t)-{2}/{15}\cdot \cos(2\omega_0t)+{2}/{35}\cdot \cos(3\omega_0t) \right ]. | :y_3(t)=\frac{2{\rm V}}{\pi} \cdot \left [ 1+{2}/{3} \cdot \cos(\omega_0t)-{2}/{15}\cdot \cos(2\omega_0t)+{2}/{35}\cdot \cos(3\omega_0t) \right ]. | ||
| − | + | At time t = 0: y_3(0) \approx 1.0125 \ \rm V; thus the error is \varepsilon_3(t = 0) \hspace{0.15cm}\underline{= 0.0125 \,\text{V}} . | |
| − | '''(7)''' | + | '''(7)''' The time t = 25\,µ\text{s} corresponds to half the period of the signal y(t). The following applies here because of \omega_0 \cdot T_0 = 2\pi: |
:y_3(T_0/2) = \frac{2{\rm V}}{\pi} \left [1+\frac{2}{3} \cdot \cos({\pi}) -\frac{2}{15}\cdot \cos(2\pi)+\frac{2}{35}\cdot \cos(3\pi)\right ]= \frac{2{\rm V}}{\pi}\left [1-\frac{2}{3}-\frac{2}{15}-\frac{2}{35}\right ] = \frac{2{\rm V}}{7\pi}\approx 0.091{\rm V}. | :y_3(T_0/2) = \frac{2{\rm V}}{\pi} \left [1+\frac{2}{3} \cdot \cos({\pi}) -\frac{2}{15}\cdot \cos(2\pi)+\frac{2}{35}\cdot \cos(3\pi)\right ]= \frac{2{\rm V}}{\pi}\left [1-\frac{2}{3}-\frac{2}{15}-\frac{2}{35}\right ] = \frac{2{\rm V}}{7\pi}\approx 0.091{\rm V}. | ||
| − | * | + | *Since y(T_0/2) = 0 this also results in \varepsilon_3(T_0/2) \hspace{0.15cm}\underline{\approx 0.091\,{\rm V}}. |
| − | * | + | *This error is larger than the error at $t = 0 by more than a factor of 7$, since y(t) has more high-frequency components at t = T_0/2 $(peak-shaped course)$. |
| − | * | + | *If it is required that the error \varepsilon_3(T_0/2) be smaller than 0.01 then at least 32 Fourier coefficients would have to be taken into account. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^2.4 Fourier Series^]] |
Latest revision as of 14:24, 15 April 2021
A cosine signal x(t) with amplitude 1\,\rm{V} and frequency f_0= 10\,\rm{kHz} is applied to the input of a half-wave rectifier. At its output, the signal y(t) results, which is shown in the graph below.
In subtasks (6) and (7) the error signal \varepsilon_3(t) = y_3(t) - y(t) is also used. This describes the difference between the Fourier series ⇒ y_3(t) limited to only N = 3 coefficients and the actual output signal y(t).
Hints:
- This exercise belongs to the chapter Fourier Series.
- To solve the problem, you can use the following definite integral (let n be an integer):
- \int ^{\pi /2}_{-\pi /2}\cos(u)\cdot\cos(2nu)\,{\rm d}u = (-1)^{n+1}\cdot\frac{2}{4n^2-1}.
- You can find a compact summary of the topic in the (German language) learning video
Zur Berechnung der Fourierkoeffizienten ⇒ "To calculate the Fourier coefficients".
Questions
Solution
- From the signal frequency f_0= 10\,\rm{kHz} follows T_0 = 1/f_0 = 100\,µ\text{s}.
- The cosine signal is mean–free (A_0 = 0) and it is completely described by a single cosine coefficient – namely A_1 .
- All sine coefficients are B_n \equiv 0, since x(t) is an even function.
- The Fourier series representation x_3(t) reproduces x(t) without error.
(2) Due to the double path rectification, the period duration is now half the value: T_0 \hspace{0.1cm}\underline{= 50\,µ\text{s}}.
- For all subsequent points, the specification T_0 refers to this value, i.e. to the period of the signal y(t).
(3) In the range from –T_0/2 to +T_0/2 \ (–25\,µ\text{s} \ \text{...} +25\,µ\text{s}) is y(t) = x(t). With f_x= 10\,\rm{kHz} = 1/(2T_0) therefore applies to this section:
- y(t)={\rm 1V}\cdot\cos(2{\pi} f_0\hspace{0.05cm}t)={\rm 1V}\cdot\cos(\pi \cdot {t}/{T_0}).
- This results in the following for the DC coefficient:
- A_0=\frac{1}{T_0}\int^{T_0/2}_{-T_0/2}y(t)\,{\rm d} t=\frac{1}{T_0}\int^{T_0/2}_{-T_0/2}{\rm 1V}\cdot\cos(\pi\cdot {t}/{T_0})\,{\rm d}t.
- With the substitution u = \pi \cdot t/T_0 one obtains:
- A_0=\left. \frac{ {\rm 1V}}{\pi}\int_{-\pi /2}^{\pi/2}\cos(u)\,{\rm d}u=\frac{ {\rm 1V}}{\pi}\sin(u)\; \right| _{-\pi/2}^{\pi/2}=\frac{ {\rm 1V}\cdot 2}{\pi} \hspace{0.15cm}\underline{\approx 0.637\;{\rm V}}.
(4) Since y(–t) = y(t) holds, all sine coefficients B_n = 0. Thus B_2 \hspace{0.1cm}\underline{= 0} also holds.
(5) For the coefficients A_n applies with the substitution u = \pi \cdot t/T_0 according to the given integral:
- A_n = \frac{2{\rm V}}{T_0}\int_{-T_0/2}^{T_0/2}\cos(\pi\frac{t}{T_0})\cdot \cos(n\cdot 2\pi\frac{t}{T_0})\,{\rm d}t = \frac{2{\rm V}}{\pi}\int_{-\pi/2}^{\pi/2}\cos(u)\cdot \cos(2n u)\,{\rm d}u \quad \Rightarrow \quad A_n = \left( { - 1} \right)^{n + 1} \frac{{4\;{\rm{V}}}}{{{\rm{\pi }}\left( {4n^2 - 1} \right)}}.
The coefficient A_2 is thus equal to -4 \,\text{V}/(15\pi) \hspace{0.1cm}\underline{\approx -\hspace{0.05cm}0.085 \, \text{V}}.
(6) For the finite Fourier series with N = 3 the following applies in general:
- y_3(t)=\frac{2{\rm V}}{\pi} \cdot \left [ 1+{2}/{3} \cdot \cos(\omega_0t)-{2}/{15}\cdot \cos(2\omega_0t)+{2}/{35}\cdot \cos(3\omega_0t) \right ].
At time t = 0: y_3(0) \approx 1.0125 \ \rm V; thus the error is \varepsilon_3(t = 0) \hspace{0.15cm}\underline{= 0.0125 \,\text{V}} .
(7) The time t = 25\,µ\text{s} corresponds to half the period of the signal y(t). The following applies here because of \omega_0 \cdot T_0 = 2\pi:
- y_3(T_0/2) = \frac{2{\rm V}}{\pi} \left [1+\frac{2}{3} \cdot \cos({\pi}) -\frac{2}{15}\cdot \cos(2\pi)+\frac{2}{35}\cdot \cos(3\pi)\right ]= \frac{2{\rm V}}{\pi}\left [1-\frac{2}{3}-\frac{2}{15}-\frac{2}{35}\right ] = \frac{2{\rm V}}{7\pi}\approx 0.091{\rm V}.
- Since y(T_0/2) = 0 this also results in \varepsilon_3(T_0/2) \hspace{0.15cm}\underline{\approx 0.091\,{\rm V}}.
- This error is larger than the error at t = 0 by more than a factor of 7, since y(t) has more high-frequency components at t = T_0/2 (peak-shaped course).
- If it is required that the error \varepsilon_3(T_0/2) be smaller than 0.01 then at least 32 Fourier coefficients would have to be taken into account.