Difference between revisions of "Aufgaben:Exercise 3.2: From the Spectrum to the Signal"
From LNTwww
m (Text replacement - "Signaldarstellung/Fouriertransformation und -rücktransformation" to "Signal_Representation/Fourier_Transform_and_Its_Inverse") |
|||
| (8 intermediate revisions by 4 users not shown) | |||
| Line 3: | Line 3: | ||
}} | }} | ||
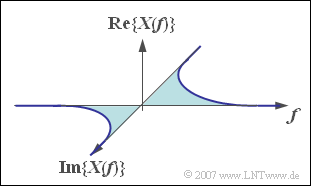
| − | [[File:P_ID495__Sig_A_3_2.png|right|frame| | + | [[File:P_ID495__Sig_A_3_2.png|right|frame|Spectral representation of the unit step function]] |
| − | + | Given the spectral function | |
:$$X(f) = \frac{{2\,{\rm V}}}{ { {\rm j}\pi f}}.$$ | :$$X(f) = \frac{{2\,{\rm V}}}{ { {\rm j}\pi f}}.$$ | ||
| − | + | The associated time function $x(t)$ can be determined with the help of [[Signal_Representation/Fourier_Transform_and_Its_Inverse#The_second_Fourier_integral|The second Fourier integral]] : | |
:$$x(t) = \int_{ - \infty }^{ + \infty } {X(f)} \cdot {\rm e}^{{\rm j}2\pi ft} {\rm d} f = x_{\rm R} (t) + {\rm j} \cdot x_{\rm I} (t),$$ | :$$x(t) = \int_{ - \infty }^{ + \infty } {X(f)} \cdot {\rm e}^{{\rm j}2\pi ft} {\rm d} f = x_{\rm R} (t) + {\rm j} \cdot x_{\rm I} (t),$$ | ||
| − | + | where holds for the real part and the imaginary part, respectively: | |
:$$x_{\rm R} (t) = 2\,{\rm V} \cdot \int_{ - \infty }^{ + \infty } {\frac{{\sin ( {2\pi ft} )}}{ {\pi f}}}\hspace{0.1cm} {\rm d}f, $$ | :$$x_{\rm R} (t) = 2\,{\rm V} \cdot \int_{ - \infty }^{ + \infty } {\frac{{\sin ( {2\pi ft} )}}{ {\pi f}}}\hspace{0.1cm} {\rm d}f, $$ | ||
| Line 22: | Line 22: | ||
| − | + | ''Hints:'' | |
| − | + | *This exercise belongs to the chapter [[Signal_Representation/Fourier_Transform_and_Its_Inverse|Fourier Transform and its Inverse]]. | |
| − | |||
| − | '' | ||
| − | * | ||
| − | * | + | *If necessary, use the following information for the solution: |
:$$x( {t = 0}) = \int_{ - \infty }^{ + \infty } {X( f )}\hspace{0.1cm} {\rm d}f,\hspace{0.5cm} X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t)}\hspace{0.1cm} {\rm d}t ,\hspace{0.5cm}\int_0^\infty {\frac{{\sin ( {ax} )}}{x}}\hspace{0.1cm} {\rm d}x = {\rm sign} ( a ) \cdot{\pi }/{2}. $$ | :$$x( {t = 0}) = \int_{ - \infty }^{ + \infty } {X( f )}\hspace{0.1cm} {\rm d}f,\hspace{0.5cm} X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t)}\hspace{0.1cm} {\rm d}t ,\hspace{0.5cm}\int_0^\infty {\frac{{\sin ( {ax} )}}{x}}\hspace{0.1cm} {\rm d}x = {\rm sign} ( a ) \cdot{\pi }/{2}. $$ | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements are true for the time signal $x(t)$ ? |
| − | |type=" | + | |type="()"} |
| − | - $x(t)$ | + | - $x(t)$ is a complex function. |
| − | + $x(t)$ | + | + $x(t)$ is purely real. |
| − | - $x(t)$ | + | - $x(t)$ is purely imaginary. |
| − | { | + | {Calculate the signal curve $x(t)$ in the entire definition area. Which values occur at the times $t = 1\, \text{ms}$ and $t = -\hspace{-0.05cm}1\, \text{ ms}$? |
|type="{}"} | |type="{}"} | ||
$x(t=+1\, \text{ms}) \ = \ $ { 2 3% } $\ \text{V}$ | $x(t=+1\, \text{ms}) \ = \ $ { 2 3% } $\ \text{V}$ | ||
$x(t=-1 \text{ms})\hspace{0.2cm} = \ $ { -2.1--1.9 } $\ \text{V}$ | $x(t=-1 \text{ms})\hspace{0.2cm} = \ $ { -2.1--1.9 } $\ \text{V}$ | ||
| − | { | + | {What is the signal value at time $t = 0$? |
|type="{}"} | |type="{}"} | ||
$x(t=0) \ = \ $ { 0. } $\ \text{V}$ | $x(t=0) \ = \ $ { 0. } $\ \text{V}$ | ||
| − | { | + | {What is the spectral value at the frequency $f = 0$? |
|type="{}"} | |type="{}"} | ||
$X(f=0) \ = \ ${ 0. } $\ \text{V/Hz}$ | $X(f=0) \ = \ ${ 0. } $\ \text{V/Hz}$ | ||
| Line 57: | Line 54: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct is the <u>proposed solution 2</u> ⇒ $x(t)$ is <u>purely real</u>: |
| − | * | + | *For the imaginary signal component ⇒ $x_{\rm I}(t)$ the integrand is an odd function (even numerator, odd denominator). |
| − | * | + | *Thus the integral from $-\infty$ bis $+\infty$ is zero. |
| − | * | + | *In contrast, for the real component $x_{\rm R}(t)$ ⇒ even integrand (odd numerator, odd denominator) yields a non-zero value. |
| − | '''(2)''' | + | '''(2)''' With the abbreviation $a = 2\pi t$ can be written for the time signal: |
:$$x(t) = x_{\rm R} \left( t \right) = \frac{{4\,{\rm V}}}{\pi }\int_0^\infty {\frac{{\sin( {af} )}}{f}}\hspace{0.1cm} {\rm d}f.$$ | :$$x(t) = x_{\rm R} \left( t \right) = \frac{{4\,{\rm V}}}{\pi }\int_0^\infty {\frac{{\sin( {af} )}}{f}}\hspace{0.1cm} {\rm d}f.$$ | ||
| − | + | This leads to the result using the given definite integral: | |
:$$x(t) = \frac{{4\,{\rm V}}}{\pi } \cdot \frac{\pi }{2} \cdot {\mathop{\rm sign}\nolimits} ( t ) = 2\;{\rm V} \cdot {\mathop{\rm sign}\nolimits} ( t ).$$ | :$$x(t) = \frac{{4\,{\rm V}}}{\pi } \cdot \frac{\pi }{2} \cdot {\mathop{\rm sign}\nolimits} ( t ) = 2\;{\rm V} \cdot {\mathop{\rm sign}\nolimits} ( t ).$$ | ||
| − | * | + | *For $t > 0$ $x(t) = +2\,\text{V}$ . |
| − | * | + | *Correspondingly, $x(t) = -\hspace{-0.1cm}2\,\text{V}$ applies for $t < 0$. |
| − | * | + | *The signal $x(t)$ thus describes a step function from $-\hspace{-0.05cm}2\,\text{V}$ auf $+2\,\text{V}$. |
| − | '''(3)''' | + | '''(3)''' $x(t)$ has a jumping point at $t = 0$. The right-hand limit value for $t \rightarrow 0$ is $x_+ = +2\,\text{V}$. |
| − | * | + | *If one approaches the jumping point of negative times as close as desired, one obtains $x_– = -\hspace{-0.05cm}2\,\text{V}$. |
| + | *The following then applies to the actual signal value at $t = 0$: | ||
:$$x( {t = 0} ) = {1}/{2}\cdot ( x_{+} + x_{-} ) \hspace{0.15 cm}\underline{= 0}.$$ | :$$x( {t = 0} ) = {1}/{2}\cdot ( x_{+} + x_{-} ) \hspace{0.15 cm}\underline{= 0}.$$ | ||
| − | * | + | *The same result is obtained by considering the relation |
:$$x( t = 0) = \int_{ - \infty }^{ + \infty } {X( f)}\hspace{0.1cm} {\rm d}f = 0.$$ | :$$x( t = 0) = \int_{ - \infty }^{ + \infty } {X( f)}\hspace{0.1cm} {\rm d}f = 0.$$ | ||
| − | '''(4)''' | + | '''(4)''' The spectral value at $f = 0$ is equal to the integral from $-\infty$ to $+\infty$ over the time function $x(t)$: |
:$$X( f = 0) = \int_{ - \infty }^{ + \infty } {x( t)}\hspace{0.1cm} {\rm d}t \hspace{0.15 cm}\underline{= 0}.$$ | :$$X( f = 0) = \int_{ - \infty }^{ + \infty } {x( t)}\hspace{0.1cm} {\rm d}t \hspace{0.15 cm}\underline{= 0}.$$ | ||
| − | + | Here is a second solution: | |
| − | * | + | *The right–hand limit for $f → 0$ is $X_+ = -\text{j} \cdot \infty$,– and the left–hand limit $X_- = \text{j} \cdot \infty$. |
| − | * | + | *So the relationship also applies with regard to the spectral value at $f = 0$: |
| − | |||
:$$X( {f = 0}) = {1}/{2}\cdot \left( {X_{ +} + X_{-} } \right) = 0.$$ | :$$X( {f = 0}) = {1}/{2}\cdot \left( {X_{ +} + X_{-} } \right) = 0.$$ | ||
| Line 103: | Line 100: | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^3.1 Fourier Transform and Its Inverse^]] |
Latest revision as of 15:25, 21 April 2021
Given the spectral function
- $$X(f) = \frac{{2\,{\rm V}}}{ { {\rm j}\pi f}}.$$
The associated time function $x(t)$ can be determined with the help of The second Fourier integral :
- $$x(t) = \int_{ - \infty }^{ + \infty } {X(f)} \cdot {\rm e}^{{\rm j}2\pi ft} {\rm d} f = x_{\rm R} (t) + {\rm j} \cdot x_{\rm I} (t),$$
where holds for the real part and the imaginary part, respectively:
- $$x_{\rm R} (t) = 2\,{\rm V} \cdot \int_{ - \infty }^{ + \infty } {\frac{{\sin ( {2\pi ft} )}}{ {\pi f}}}\hspace{0.1cm} {\rm d}f, $$
- $$x_{\rm I} (t) = -2\, {\rm V} \cdot \int_{ - \infty }^{ + \infty } {\frac{ {\cos ( {2\pi ft} )}}{ {\pi f}}} \hspace{0.1cm}{\rm d}f.$$
Hints:
- This exercise belongs to the chapter Fourier Transform and its Inverse.
- If necessary, use the following information for the solution:
- $$x( {t = 0}) = \int_{ - \infty }^{ + \infty } {X( f )}\hspace{0.1cm} {\rm d}f,\hspace{0.5cm} X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t)}\hspace{0.1cm} {\rm d}t ,\hspace{0.5cm}\int_0^\infty {\frac{{\sin ( {ax} )}}{x}}\hspace{0.1cm} {\rm d}x = {\rm sign} ( a ) \cdot{\pi }/{2}. $$
Questions
Solution
(1) Correct is the proposed solution 2 ⇒ $x(t)$ is purely real:
- For the imaginary signal component ⇒ $x_{\rm I}(t)$ the integrand is an odd function (even numerator, odd denominator).
- Thus the integral from $-\infty$ bis $+\infty$ is zero.
- In contrast, for the real component $x_{\rm R}(t)$ ⇒ even integrand (odd numerator, odd denominator) yields a non-zero value.
(2) With the abbreviation $a = 2\pi t$ can be written for the time signal:
- $$x(t) = x_{\rm R} \left( t \right) = \frac{{4\,{\rm V}}}{\pi }\int_0^\infty {\frac{{\sin( {af} )}}{f}}\hspace{0.1cm} {\rm d}f.$$
This leads to the result using the given definite integral:
- $$x(t) = \frac{{4\,{\rm V}}}{\pi } \cdot \frac{\pi }{2} \cdot {\mathop{\rm sign}\nolimits} ( t ) = 2\;{\rm V} \cdot {\mathop{\rm sign}\nolimits} ( t ).$$
- For $t > 0$ $x(t) = +2\,\text{V}$ .
- Correspondingly, $x(t) = -\hspace{-0.1cm}2\,\text{V}$ applies for $t < 0$.
- The signal $x(t)$ thus describes a step function from $-\hspace{-0.05cm}2\,\text{V}$ auf $+2\,\text{V}$.
(3) $x(t)$ has a jumping point at $t = 0$. The right-hand limit value for $t \rightarrow 0$ is $x_+ = +2\,\text{V}$.
- If one approaches the jumping point of negative times as close as desired, one obtains $x_– = -\hspace{-0.05cm}2\,\text{V}$.
- The following then applies to the actual signal value at $t = 0$:
- $$x( {t = 0} ) = {1}/{2}\cdot ( x_{+} + x_{-} ) \hspace{0.15 cm}\underline{= 0}.$$
- The same result is obtained by considering the relation
- $$x( t = 0) = \int_{ - \infty }^{ + \infty } {X( f)}\hspace{0.1cm} {\rm d}f = 0.$$
(4) The spectral value at $f = 0$ is equal to the integral from $-\infty$ to $+\infty$ over the time function $x(t)$:
- $$X( f = 0) = \int_{ - \infty }^{ + \infty } {x( t)}\hspace{0.1cm} {\rm d}t \hspace{0.15 cm}\underline{= 0}.$$
Here is a second solution:
- The right–hand limit for $f → 0$ is $X_+ = -\text{j} \cdot \infty$,– and the left–hand limit $X_- = \text{j} \cdot \infty$.
- So the relationship also applies with regard to the spectral value at $f = 0$:
- $$X( {f = 0}) = {1}/{2}\cdot \left( {X_{ +} + X_{-} } \right) = 0.$$