Difference between revisions of "Aufgaben:Exercise 4.09: Cyclo-Ergodicity"
From LNTwww
m (Text replacement - "Category:Aufgaben zu Stochastische Signaltheorie" to "Category:Theory of Stochastic Signals: Exercises") |
|||
| (6 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Auto-Correlation_Function |
}} | }} | ||
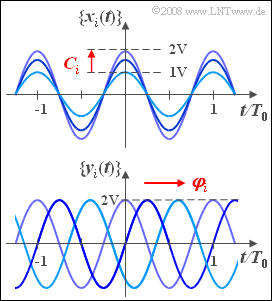
| − | [[File:P_ID379__Sto_A_4_9.png|right|frame| | + | [[File:P_ID379__Sto_A_4_9.png|right|frame|"Cyclo–ergodicity property"]] |
| − | + | We consider two different random processes whose pattern functions are harmonic oscillations, each with the same frequency f0=1/T0, where T0 denotes the period duration. | |
| − | * | + | *In the random process {xi(t)} shown above, the stochastic component is the amplitude, where the random parameter Ci can take all values between 1V and 2V with equal probability: |
| − | :$$\{ x_i(t) \} = \{ C_i \cdot \cos (2 \pi | + | :{xi(t)}={Ci⋅cos(2πf0t)}. |
| − | * | + | *In the process {yi(t)}, all pattern functions have the same amplitude: x0=2V. Here the phase φi varies, which averaged over all pattern functions is uniformly distributed between 0 and 2π |
| − | :$$\{ y_i(t) \} = \{ x_{\rm 0} \cdot \cos (2 \pi | + | :{yi(t)}={x0⋅cos(2πf0t−φi)}. |
| − | + | The properties "cyclo-stationary" and "cyclo-ergodic" state, | |
| − | * | + | *that although the processes cannot be described as stationary and ergodic in the strict sense, |
| − | * | + | *but all statistical characteristics are the same for multiples of the period duration T0 in each case. |
| − | In | + | In these cases, most of the calculation rules, which actually apply only to ergodic processes, are also applicable. |
| − | + | '''Hint''': This exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Auto-Correlation_Function|Auto-Correlation Function]]. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | '' | ||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The process {xi(t)} is stationary. |
| − | - | + | - The process {xi(t)} is ergodic. |
| − | + | + | + The process {yi(t)} is stationary. |
| − | + | + | + The process {yi(t)} is ergodic. |
| − | { | + | {Calculate the auto-correlation function φy(τ) for different τ values. |
|type="{}"} | |type="{}"} | ||
φy(τ=0) = { 2 3% } V2 | φy(τ=0) = { 2 3% } V2 | ||
| − | $\varphi_y(\tau=0.25 \cdot T_0)\ = | + | φy(τ=0.25⋅T0) = { 0. } V2 |
| − | $\varphi_y(\tau=1.50 \cdot T_0)\ = | + | φy(τ=1.50⋅T0) = { -2.06--1.94 } V2 |
| − | { | + | {Which of the following statements are true regarding {yi(t)} ? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + All pattern signals are free of DC signals. |
| − | + | + | + All pattern signals have the rms value 2V. |
| − | - | + | - The ACF has twice the period (2T0) as the pattern signals (T0). |
| − | |||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct are the <u>proposed solutions 3 and 4</u>: |
| − | * | + | *At time t=0 (and all multiples of the period T0) each pattern signal xi(t) has a value between 1V and 2V. The mean value is 1.5V. |
| − | * | + | *In contrast, for t=T0/4 the signal value of the entire ensemble is identically zero. That is: <br> Even the linear mean does not satisfy the stationarity condition: The process {xi(t)} is not stationary and therefore cannot be ergodic. |
| − | + | *In contrast, for the process {yi(t)} the same moments are expected at all times due to the uniformly distributed phase ⇒ the process is stationary. | |
| − | * | + | *Since the phase relations are lost in the ACF calculation, each individual pattern function is representative of the entire process. Therefore, ergodicity can be hypothetically assumed here. |
| − | * | + | *At the end of the exercise, check whether this assumption is justified. |
| − | * | ||
| − | + | '''(2)''' Due to ergodicity, any pattern function can be used for ACF calculation. We arbitrarily use here the phase φi=0. | |
| − | '''(2)''' | + | *Because of the periodicity, it is sufficient to report only one period T0. Then holds: |
| − | * | ||
:φy(τ)=1T0⋅∫T00y(t)⋅y(t+τ)dt=x20T0⋅∫T00cos(2πf0t)⋅cos(2πf0(t+τ))dt. | :φy(τ)=1T0⋅∫T00y(t)⋅y(t+τ)dt=x20T0⋅∫T00cos(2πf0t)⋅cos(2πf0(t+τ))dt. | ||
| − | + | *Using the trigonometric relation cos(α)⋅cos(β)=1/2⋅cos(α+β)+1/2⋅cos(α−β) it further follows: | |
| − | * | ||
:φy(τ)=x202T0⋅∫T00cos(4πf0t+2πf0τ)dt + x202T0⋅∫T00cos(−2πf0τ)dt. | :φy(τ)=x202T0⋅∫T00cos(4πf0t+2πf0τ)dt + x202T0⋅∫T00cos(−2πf0τ)dt. | ||
| − | + | *The first integral is zero (integration over two periods of the cosine function). The second integrand is independent of the integration variable t. It follows: | |
| − | * | + | :$$\varphi_y (\tau) ={{ x}_0^2}/{\rm 2} \cdot \cos (2 \pi {f_{\rm 0} \tau}). $$ |
| − | + | *For the given time points, with x0=2V: | |
| − | * | ||
:φy(0)=2V2_,φy(0.25⋅T0)=0_,φy(1.5⋅T0)=−2V2_. | :φy(0)=2V2_,φy(0.25⋅T0)=0_,φy(1.5⋅T0)=−2V2_. | ||
| − | '''(3)''' | + | '''(3)''' Correct are <u>both first proposed solutions</u>: |
| − | * | + | *The mean my can be obtained from the limit of the ACF for τ→∞ if one excludes the periodic parts. It follows my=0. |
| − | * | + | *The variance (power) is equal to the ACF value at the point τ=0 ⇒ $\sigma_y^2 =2\hspace{0.05cm}\rm V^2$. The rms value is the square root of it: σy≈1.414V. |
| − | * | + | *The period of a periodic random process is preserved in the ACF, that is, the period of the ACF is also T0. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category:Theory of Stochastic Signals: Exercises|^4.4 | + | [[Category:Theory of Stochastic Signals: Exercises|^4.4 Auto-Correlation Function^]] |
Latest revision as of 18:00, 19 March 2022
We consider two different random processes whose pattern functions are harmonic oscillations, each with the same frequency f0=1/T0, where T0 denotes the period duration.
- In the random process {xi(t)} shown above, the stochastic component is the amplitude, where the random parameter Ci can take all values between 1V and 2V with equal probability:
- {xi(t)}={Ci⋅cos(2πf0t)}.
- In the process {yi(t)}, all pattern functions have the same amplitude: x0=2V. Here the phase φi varies, which averaged over all pattern functions is uniformly distributed between 0 and 2π
- {yi(t)}={x0⋅cos(2πf0t−φi)}.
The properties "cyclo-stationary" and "cyclo-ergodic" state,
- that although the processes cannot be described as stationary and ergodic in the strict sense,
- but all statistical characteristics are the same for multiples of the period duration T0 in each case.
In these cases, most of the calculation rules, which actually apply only to ergodic processes, are also applicable.
Hint: This exercise belongs to the chapter Auto-Correlation Function.
Questions
Solution
(1) Correct are the proposed solutions 3 and 4:
- At time t=0 (and all multiples of the period T0) each pattern signal xi(t) has a value between 1V and 2V. The mean value is 1.5V.
- In contrast, for t=T0/4 the signal value of the entire ensemble is identically zero. That is:
Even the linear mean does not satisfy the stationarity condition: The process {xi(t)} is not stationary and therefore cannot be ergodic. - In contrast, for the process {yi(t)} the same moments are expected at all times due to the uniformly distributed phase ⇒ the process is stationary.
- Since the phase relations are lost in the ACF calculation, each individual pattern function is representative of the entire process. Therefore, ergodicity can be hypothetically assumed here.
- At the end of the exercise, check whether this assumption is justified.
(2) Due to ergodicity, any pattern function can be used for ACF calculation. We arbitrarily use here the phase φi=0.
- Because of the periodicity, it is sufficient to report only one period T0. Then holds:
- φy(τ)=1T0⋅∫T00y(t)⋅y(t+τ)dt=x20T0⋅∫T00cos(2πf0t)⋅cos(2πf0(t+τ))dt.
- Using the trigonometric relation cos(α)⋅cos(β)=1/2⋅cos(α+β)+1/2⋅cos(α−β) it further follows:
- φy(τ)=x202T0⋅∫T00cos(4πf0t+2πf0τ)dt + x202T0⋅∫T00cos(−2πf0τ)dt.
- The first integral is zero (integration over two periods of the cosine function). The second integrand is independent of the integration variable t. It follows:
- φy(τ)=x20/2⋅cos(2πf0τ).
- For the given time points, with x0=2V:
- φy(0)=2V2_,φy(0.25⋅T0)=0_,φy(1.5⋅T0)=−2V2_.
(3) Correct are both first proposed solutions:
- The mean my can be obtained from the limit of the ACF for τ→∞ if one excludes the periodic parts. It follows my=0.
- The variance (power) is equal to the ACF value at the point τ=0 ⇒ σ2y=2V2. The rms value is the square root of it: σy≈1.414V.
- The period of a periodic random process is preserved in the ACF, that is, the period of the ACF is also T0.