Difference between revisions of "Aufgaben:Exercise 3.12: Trellis Diagram for Two Precursors"
From LNTwww
m (Text replacement - "Category:Aufgaben zu Digitalsignalübertragung" to "Category:Digital Signal Transmission: Exercises") |
|||
| (6 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Viterbi_Receiver}} |
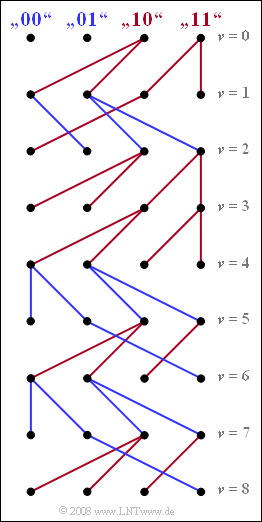
| − | [[File:P_ID1478__Dig_A_3_12.png|right|frame| | + | [[File:P_ID1478__Dig_A_3_12.png|right|frame|Trellis diagram for two precursors]] |
| − | + | We assume the basic pulse values $g_0\ne 0, g_{\rm –1}\ne 0$ and $g_{\rm –2}\ne 0$: | |
| − | * | + | *This means that the decision on the symbol aν is also influenced by the subsequent coefficients aν+1 and aν+2. |
| − | * | + | |
| − | * | + | *Thus, for each time point ν, exactly eight '''metrics''' εν have to be determined, from which the '''minimum accumulated metrics''' Γν(00), Γν(01), Γν(10) and Γν(11) can be calculated. |
| − | * | + | |
| − | :$$\big[{\it \Gamma}_{\nu-1}(00) + \varepsilon_{\nu}(001)\big] \hspace{0.15cm}{\rm | + | *For example, Γν(01) provides information about the symbol aν under the assumption that aν+1=0 and aν+2=1 will be. |
| + | |||
| + | *Here, the minimum accumulated metric Γν(01) is the smaller value obtained from the comparison of | ||
| + | :$$\big[{\it \Gamma}_{\nu-1}(00) + \varepsilon_{\nu}(001)\big] \hspace{0.15cm}{\rm and} | ||
\hspace{0.15cm}\big[{\it \Gamma}_{\nu-1}(10) + \varepsilon_{\nu}(101)\big].$$ | \hspace{0.15cm}\big[{\it \Gamma}_{\nu-1}(10) + \varepsilon_{\nu}(101)\big].$$ | ||
| − | + | To calculate the minimum accumulated metric Γ2(10) in subtasks '''(1)''' and '''(2)''', assume the following numerical values: | |
| − | * | + | * unipolar amplitude coefficients: a_{\rm \nu} ∈ \{0, 1\}, |
| − | * | + | |
| − | * | + | * basic pulse values g0=0.5, g_{\rm –1} = 0.3, g_{\rm –2} = 0.2, |
| − | * | + | |
| + | * applied noisy detection sample: d2=0.2, | ||
| + | |||
| + | * minimum accumulated metric at time ν=1: | ||
:$${\it \Gamma}_{1}(00) = 0.0,\hspace{0.2cm}{\it \Gamma}_{1}(01) = 0.2, \hspace{0.2cm} {\it \Gamma}_{1}(10) = 0.6,\hspace{0.2cm}{\it \Gamma}_{1}(11) = | :$${\it \Gamma}_{1}(00) = 0.0,\hspace{0.2cm}{\it \Gamma}_{1}(01) = 0.2, \hspace{0.2cm} {\it \Gamma}_{1}(10) = 0.6,\hspace{0.2cm}{\it \Gamma}_{1}(11) = | ||
1.2 | 1.2 | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The graph shows the simplified trellis diagram for time points ν=1 to ν=8. | |
| − | * | + | *Blue branches come from either {\it \Gamma}_{\rm \nu –1}(00) or {\it \Gamma}_{\rm \nu –1}(01) and denote a hypothetical "0". |
| − | * | + | |
| − | + | *In contrast, all red branches – starting from the {\it \Gamma}_{\rm \nu –1}(10) or {\it \Gamma}_{\rm \nu –1}(11) states – indicate the symbol "1". | |
| + | Notes: | ||
| + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Viterbi_Receiver|"Viterbi Receiver"]]. | ||
| + | |||
| + | * All quantities here are to be understood normalized. | ||
| − | + | * Also, assume unipolar and equal probability amplitude coefficients: Pr(aν=0)=Pr(aν=1)=0.5. | |
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the following metrics: |
|type="{}"} | |type="{}"} | ||
ε2(010) = { 0.01 3% } | ε2(010) = { 0.01 3% } | ||
| Line 46: | Line 51: | ||
ε2(111) = { 0.64 3% } | ε2(111) = { 0.64 3% } | ||
| − | { | + | {Calculate the following minimum accumulated metrics: |
|type="{}"} | |type="{}"} | ||
Γ2(10) = { 0.21 3% } | Γ2(10) = { 0.21 3% } | ||
Γ2(11) = { 0.29 3% } | Γ2(11) = { 0.29 3% } | ||
| − | { | + | {What are the symbols output by the Viterbi receiver? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The first seven symbols are "1011010". |
| − | - | + | - The first seven symbols are "1101101". |
| − | - | + | - The last symbol a8=1 is safe. |
| − | + | + | + No definite statement can be made about the symbol a8. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The first metric is calculated as follows: |
:$$\varepsilon_{2}(010) = [d_0 - 0 \cdot g_0 - 1 \cdot g_{-1}- 0 \cdot g_{-2}]^2= [0.2 -0.3]^2\hspace{0.15cm}\underline {=0.01} | :$$\varepsilon_{2}(010) = [d_0 - 0 \cdot g_0 - 1 \cdot g_{-1}- 0 \cdot g_{-2}]^2= [0.2 -0.3]^2\hspace{0.15cm}\underline {=0.01} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | Correspondingly, for the other metrics: | |
:ε2(011) = [0.2−0.3−0.2]2=0.09_, | :ε2(011) = [0.2−0.3−0.2]2=0.09_, | ||
:ε2(110) = [0.2−0.5−0.3]2=0.36_, | :ε2(110) = [0.2−0.5−0.3]2=0.36_, | ||
| Line 72: | Line 77: | ||
| − | '''(2)''' | + | '''(2)''' The task is to find the minimum value of each of two comparison values: |
:$${\it \Gamma}_{2}(10) \ = \ {\rm Min}\left[{\it \Gamma}_{1}(01) + \varepsilon_{2}(010), | :$${\it \Gamma}_{2}(10) \ = \ {\rm Min}\left[{\it \Gamma}_{1}(01) + \varepsilon_{2}(010), | ||
\hspace{0.2cm}{\it \Gamma}_{1}(11) + \varepsilon_{2}(110)\right] = {\rm Min}\left[0.2+ 0.01, 1.2 + 0.36\right]\hspace{0.15cm}\underline {= 0.21} | \hspace{0.2cm}{\it \Gamma}_{1}(11) + \varepsilon_{2}(110)\right] = {\rm Min}\left[0.2+ 0.01, 1.2 + 0.36\right]\hspace{0.15cm}\underline {= 0.21} | ||
| Line 81: | Line 86: | ||
| − | '''(3)''' | + | '''(3)''' The <u>first and last solutions</u> are correct: |
| − | * | + | *The sequence "1011010" can be recognized from the continuous path: "red – blue – red – red – blue – red – blue". |
| − | * | + | |
| − | * | + | *On the other hand, no final statement can be made about the symbol a8 at time ν=8: |
| + | |||
| + | *Only under the hypothesis a9=1 <u>and</u> a10=1 one would decide for a8=0, under other hypotheses for a8=1. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category:Digital Signal Transmission: Exercises|^3.8 Viterbi | + | [[Category:Digital Signal Transmission: Exercises|^3.8 Viterbi Receiver^]] |
Latest revision as of 17:34, 4 July 2022
We assume the basic pulse values g0≠0, g_{\rm –1}\ne 0 and g_{\rm –2}\ne 0:
- This means that the decision on the symbol a_{\rm \nu} is also influenced by the subsequent coefficients a_{\rm \nu +1} and a_{\rm \nu +2}.
- Thus, for each time point \nu, exactly eight metrics \varepsilon_{\rm \nu} have to be determined, from which the minimum accumulated metrics {\it \Gamma}_{\rm \nu}(00), {\it \Gamma}_{\rm \nu}(01), {\it \Gamma}_{\rm \nu}(10) and {\it \Gamma}_{\rm \nu}(11) can be calculated.
- For example, {\it \Gamma}_{\rm \nu}(01) provides information about the symbol a_{\rm \nu} under the assumption that a_{\rm \nu +1} = 0 and a_{\rm \nu +2} = 1 will be.
- Here, the minimum accumulated metric {\it \Gamma}_{\rm \nu}(01) is the smaller value obtained from the comparison of
- \big[{\it \Gamma}_{\nu-1}(00) + \varepsilon_{\nu}(001)\big] \hspace{0.15cm}{\rm and} \hspace{0.15cm}\big[{\it \Gamma}_{\nu-1}(10) + \varepsilon_{\nu}(101)\big].
To calculate the minimum accumulated metric {\it \Gamma}_2(10) in subtasks (1) and (2), assume the following numerical values:
- unipolar amplitude coefficients: a_{\rm \nu} ∈ \{0, 1\},
- basic pulse values g_0 = 0.5, g_{\rm –1} = 0.3, g_{\rm –2} = 0.2,
- applied noisy detection sample: d_2 = 0.2,
- minimum accumulated metric at time \nu = 1:
- {\it \Gamma}_{1}(00) = 0.0,\hspace{0.2cm}{\it \Gamma}_{1}(01) = 0.2, \hspace{0.2cm} {\it \Gamma}_{1}(10) = 0.6,\hspace{0.2cm}{\it \Gamma}_{1}(11) = 1.2 \hspace{0.05cm}.
The graph shows the simplified trellis diagram for time points \nu = 1 to \nu = 8.
- Blue branches come from either {\it \Gamma}_{\rm \nu –1}(00) or {\it \Gamma}_{\rm \nu –1}(01) and denote a hypothetical "0".
- In contrast, all red branches – starting from the {\it \Gamma}_{\rm \nu –1}(10) or {\it \Gamma}_{\rm \nu –1}(11) states – indicate the symbol "1".
Notes:
- The exercise belongs to the chapter "Viterbi Receiver".
- All quantities here are to be understood normalized.
- Also, assume unipolar and equal probability amplitude coefficients: {\rm Pr} (a_\nu = 0) = {\rm Pr} (a_\nu = 1)= 0.5.
Questions
Solution
(1) The first metric is calculated as follows:
- \varepsilon_{2}(010) = [d_0 - 0 \cdot g_0 - 1 \cdot g_{-1}- 0 \cdot g_{-2}]^2= [0.2 -0.3]^2\hspace{0.15cm}\underline {=0.01} \hspace{0.05cm}.
Correspondingly, for the other metrics:
- \varepsilon_{2}(011) \ = \ [0.2 -0.3- 0.2]^2\hspace{0.15cm}\underline {=0.09}\hspace{0.05cm},
- \varepsilon_{2}(110) \ = \ [0.2 -0.5- 0.3]^2\hspace{0.15cm}\underline {=0.36}\hspace{0.05cm},
- \varepsilon_{2}(111) \ = \ [0.2 -0.5- 0.3-0.2]^2\hspace{0.15cm}\underline {=0.64} \hspace{0.05cm}.
(2) The task is to find the minimum value of each of two comparison values:
- {\it \Gamma}_{2}(10) \ = \ {\rm Min}\left[{\it \Gamma}_{1}(01) + \varepsilon_{2}(010), \hspace{0.2cm}{\it \Gamma}_{1}(11) + \varepsilon_{2}(110)\right] = {\rm Min}\left[0.2+ 0.01, 1.2 + 0.36\right]\hspace{0.15cm}\underline {= 0.21} \hspace{0.05cm},
- {\it \Gamma}_{2}(11) \ = \ {\rm Min}\left[{\it \Gamma}_{1}(01) + \varepsilon_{2}(011), \hspace{0.2cm}{\it \Gamma}_{1}(11) + \varepsilon_{2}(111)\right] = {\rm Min}\left[0.2+ 0.09, 1.2 + 0.64\right]\hspace{0.15cm}\underline {= 0.29} \hspace{0.05cm}.
(3) The first and last solutions are correct:
- The sequence "1011010" can be recognized from the continuous path: "red – blue – red – red – blue – red – blue".
- On the other hand, no final statement can be made about the symbol a_8 at time \nu = 8:
- Only under the hypothesis a_9 = 1 and a_{\rm 10} = 1 one would decide for a_8 = 0, under other hypotheses for a_8 = 1.