Aufgaben:Exercise 2.3Z: Oscillation Parameters

• Return to book

Definitions of  $x_0$,  $t_1$  und  $t_2$

Every harmonic oscillation can also be written in the form

$$x(t)=C\cdot\cos\bigg(2\pi \cdot \frac{t-\tau}{T_0}\bigg).$$

The oscillation is thus completely determined by three parameters:

• the amplitude  $C$,

• the period duration  $T_0$,

• the shift  $\tau$  with respect to a cosine signal.

A second form of representation is with the basic frequency  $f_0$  and the phase  $\varphi$:

$$x(t)=C \cdot\cos(2\pi f_0t-\varphi).$$

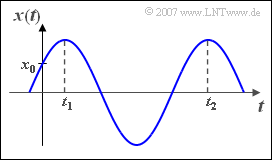
From a harmonic oscillation it is now known that

• the first signal maximum occurs at  $t_1 = 2 \,\text{ms}$,

• the second signal maximum occurs at  $t_2 = 14 \,\text{ms}$,

• the value  $x_0 ={x(t = 0)} = 3 \,\text{V}$.

Hint:

• This exercise belongs to the chapter  Harmonic Oscillation.

Questions

1

What is the period duration  $T_0$  and the base frequency  $f_0$?

$T_0\hspace{0.2cm} = \ $  $\text{ms}$

$f_0\hspace{0.2cm} = \ $  $\text{Hz}$

2

What is the value of the shift  $\tau$  and the phase  $\varphi$  $($in  $\text{degrees})$ ?

$\tau\hspace{0.25cm} = \ $  $\text{ms}$

$\varphi\hspace{0.2cm} = \ $  $\text{deg}$

3

What is the amplitude of the harmonic oscillation?

${C}\ = \ $  $\text{V}$

4

What is the spectrum  $X(f)$?  What is the weight of the spectral line at  $+f_0$ ?

$\text{Re}\big[X(f = f_0)\big]\ = \ $  $\text{V}$

$\text{Im}\big[X(f = f_0)\big] \ = \ $  $\text{V}$

Solution

Solution

(1)  It is  $T_0 = t_2 - t_1 = 12\, \text{ms}$  and  $f_0 = 1/T_0 \hspace{0.15cm} \underline{\approx 83.33\, \text{Hz}}$.

(2)  The shift is  $\tau \hspace{0.1cm} \underline{= 2\, \text{ms}}$  and the phase is  $\varphi = 2\pi \cdot \tau/T_0 = \pi/3$  corresponding to  $\varphi =\hspace{0.15cm} \underline{60^{\circ}}$.

(3)  From the value at timet  $t = 0$  it follows for the amplitude  ${C}$:

$$x_0=x(t=0)=C\cdot\cos(-60\,^\circ)={C}/{2}=\rm 3\,V \hspace{0.3 cm} \Rightarrow \hspace{0.3 cm}\hspace{0.15cm}\underline{\it C=\rm 6\,V}.$$

(4)  The corresponding spectral function is:

$$X(f)={C}/{2}\cdot{\rm e}^{-{\rm j}\varphi}\cdot\delta(f-f_0)+{C}/{2}\cdot{\rm e}^{{\rm j}\varphi}\cdot\delta(f+f_0).$$

• The weight of the Dirac delta line at   $f = f_0$  (first term) is   ${C}/2 \cdot {\rm e}^{–\text{j}\varphi} = 3 \,\text{V} \cdot \cos(60^\circ)- 3 \,\text{V} \cdot \sin(60^\circ)\hspace{0.05cm}\approx \underline{1.5 \,\text{V} - \text{j} \cdot 2.6 \,\text{V}}$.