Difference between revisions of "Aufgaben:Exercise 4.8: Diamond-shaped Joint PDF"
From LNTwww
| Line 68: | Line 68: | ||
*The area of the triangle $(1,0)\ (1,4)\ (-1,3)$ gives $0.5 · 4 · 2 = 4$. | *The area of the triangle $(1,0)\ (1,4)\ (-1,3)$ gives $0.5 · 4 · 2 = 4$. | ||
*The total area is double: $F = 8$. | *The total area is double: $F = 8$. | ||
| − | *Since the PDF | + | *Since the PDF volume is always $1$ , then $H= 1/F\hspace{0.15cm}\underline{ = 0.125}$. |
| + | |||
| + | |||
| + | |||
'''(2)''' The minimum value of $x$ is obtained for $\underline{ u=0}$ and $\underline{ v=1}$. | '''(2)''' The minimum value of $x$ is obtained for $\underline{ u=0}$ and $\underline{ v=1}$. | ||
| − | *From the above equations, the results $x= -1$ and $y= +3$ follow. | + | *From the above equations, the results $x= -1$ and $y= +3$ follow. |
| − | '''(3)''' The equation given in the theory section is valid in general, i.e., for any PDF of the two statistically independent variables $u$ and $v$, | + | |
| − | + | ||
| + | |||
| + | '''(3)''' The equation given in the theory section is valid in general, i.e., for any PDF of the two statistically independent variables $u$ and $v$, as long as they have equal standard deviations $(\sigma_u = \sigma_v)$. | ||
*With $A = 2$, $B = -2$, $D = 1$ and $E = 3$ we obtain: | *With $A = 2$, $B = -2$, $D = 1$ and $E = 3$ we obtain: | ||
:$$\rho_{xy } = \frac {\it A \cdot D + B \cdot E}{\sqrt{(\it A^{\rm 2}+\it B^{\rm 2})(\it D^{\rm 2}+\it E^{\rm 2})}} =\frac {2 \cdot 1 -2 \cdot 3}{\sqrt{(4 +4)(1+9)}} = \frac {-4}{\sqrt{80}} = \frac {-1}{\sqrt{5}}\hspace{0.15cm}\underline{ = -0.447}. $$ | :$$\rho_{xy } = \frac {\it A \cdot D + B \cdot E}{\sqrt{(\it A^{\rm 2}+\it B^{\rm 2})(\it D^{\rm 2}+\it E^{\rm 2})}} =\frac {2 \cdot 1 -2 \cdot 3}{\sqrt{(4 +4)(1+9)}} = \frac {-4}{\sqrt{80}} = \frac {-1}{\sqrt{5}}\hspace{0.15cm}\underline{ = -0.447}. $$ | ||
| − | |||
'''(4)''' The regression line is generally: | '''(4)''' The regression line is generally: | ||
| − | :$$y | + | :$$y=\frac{\sigma_y}{\sigma_x}\cdot\rho_{xy}\cdot(x-m_x)+m_y.$$ |
| − | *From the linear means $m_u = m_v = 0.5$ and the equations given in the problem statement, we obtain $m_x = 1$ and $m_y = 2$. | + | *From the linear means $m_u = m_v = 0.5$ and the equations given in the problem statement, we obtain $m_x = 1$ and $m_y = 2$. |
*The variances of $u$ and $v$ are respectively $\sigma_u^2 = \sigma_v^2 =1/12$. It follows: | *The variances of $u$ and $v$ are respectively $\sigma_u^2 = \sigma_v^2 =1/12$. It follows: | ||
| Line 88: | Line 92: | ||
:$$\sigma_y^2 = \sigma_u^2 + 9\cdot \sigma_v^2 = 5/6.$$ | :$$\sigma_y^2 = \sigma_u^2 + 9\cdot \sigma_v^2 = 5/6.$$ | ||
| − | *Substituting these values into the equation of the regression line, we get: | + | *Substituting these values into the equation of the regression line, we get: |
| − | :$$y | + | :$$y=\frac{\sqrt{5/6}}{\sqrt{2/3}}\cdot (\frac{-1}{\sqrt{5}})\cdot(x-1)+2= - x/{2} + 2.5.$$ |
| − | *From this follows the value $y_0= | + | *From this follows with $x=0$ the value $y_0=\hspace{0.15cm}\underline{ = 2.5}$ |
| − | '''(5)''' With the auxiliary quantities $q= 2u$, $r= -2v$ and $s= x-1$ | + | '''(5)''' With the auxiliary quantities $q= 2u$, $r= -2v$ and $s= x-1$: $s= q+r$. |
| − | |||
| − | |||
| − | |||
[[File:P_ID414__Sto_A_4_8_e.png|right|frame|Triangular PDF $f_x(x)$]] | [[File:P_ID414__Sto_A_4_8_e.png|right|frame|Triangular PDF $f_x(x)$]] | ||
| + | *Since $u$ and $v$ are each uniformly distributed between $0$ and $1$, $q$ has a uniform distribution in the range from $0$ to $2$ and $r$ is uniformly distributed between $-2$ and $0$. | ||
| + | *In addition, since $q$ and $r$ are not statistically dependent on each other, the PDF of the sum is: | ||
:$$f_s(s) = f_q(q) \star f_r(r).$$ | :$$f_s(s) = f_q(q) \star f_r(r).$$ | ||
*The addition $x = s+1$ leads to a shift of the triangular–PDF by $1$ to the right. | *The addition $x = s+1$ leads to a shift of the triangular–PDF by $1$ to the right. | ||
| − | *For the sought probability (highlighted in green in the | + | *For the sought probability (highlighted in green in the graphic) therefore holds: |
| − | + | :$${\rm Pr}(x < 0)\hspace{0.15cm}\underline{ = 0.125}.$$ | |
| + | |||
| + | |||
| + | |||
[[File: P_ID415__Sto_A_4_8_f.png|right|frame|Trapezoidal PDF $f_y(y)$]] | [[File: P_ID415__Sto_A_4_8_f.png|right|frame|Trapezoidal PDF $f_y(y)$]] | ||
| − | '''(6)''' Analogous to the | + | '''(6)''' Analogous to the solution for the subtask '''(5)''' holds with $t = 3v$: |
:$$f_y(y) = f_u(u) \star f_t(t).$$ | :$$f_y(y) = f_u(u) \star f_t(t).$$ | ||
| − | *The convolution between two rectangles of different widths results in a trapezoid. | + | *The convolution between two rectangles of different widths results in a trapezoid. For the probability we are looking for, we get: |
| − | + | :$${\rm Pr}(y>3) =1/6\hspace{0.15cm}\underline{ \approx 0.167}.$$ | |
*This probability is highlighted in green in the right sketch. | *This probability is highlighted in green in the right sketch. | ||
Latest revision as of 14:07, 27 February 2022
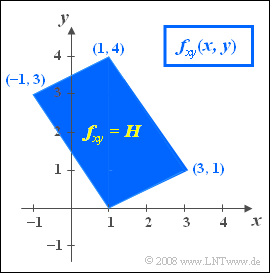
We consider a two-dimensional random variable $(x,\hspace{0.08cm} y)$ whose components arise as linear combinations of two random variables $u$ and $v$:
- $$x=2u-2v+1,$$
- $$y=u+3v.$$
Further, note:
- The two statistically independent random variables $u$ and $v$ are each uniformly distributed between $0$ and $1$.
- In the figure you can see the joint PDF. Within the parallelogram drawn in blue holds:
- $$f_{xy}(x,\hspace{0.08cm} y) = H = {\rm const.}$$
- Outside the parallelogram no values are possible: $f_{xy}(x,\hspace{0.08cm} y) = 0$.
Hints:
- The exercise belongs to the chapter Linear Combinations of Random Variables.
- Reference is also made to the page Regression line.
- We also refer here to the interactive applet Correlation coefficient and regression line
- Assume - if possible - the given equations. Use the information of the above sketch mainly only to check your results.
Questions
Solution
(1) The area of the parallelogram can be composed of two triangles of equal size.
- The area of the triangle $(1,0)\ (1,4)\ (-1,3)$ gives $0.5 · 4 · 2 = 4$.
- The total area is double: $F = 8$.
- Since the PDF volume is always $1$ , then $H= 1/F\hspace{0.15cm}\underline{ = 0.125}$.
(2) The minimum value of $x$ is obtained for $\underline{ u=0}$ and $\underline{ v=1}$.
- From the above equations, the results $x= -1$ and $y= +3$ follow.
(3) The equation given in the theory section is valid in general, i.e., for any PDF of the two statistically independent variables $u$ and $v$, as long as they have equal standard deviations $(\sigma_u = \sigma_v)$.
- With $A = 2$, $B = -2$, $D = 1$ and $E = 3$ we obtain:
- $$\rho_{xy } = \frac {\it A \cdot D + B \cdot E}{\sqrt{(\it A^{\rm 2}+\it B^{\rm 2})(\it D^{\rm 2}+\it E^{\rm 2})}} =\frac {2 \cdot 1 -2 \cdot 3}{\sqrt{(4 +4)(1+9)}} = \frac {-4}{\sqrt{80}} = \frac {-1}{\sqrt{5}}\hspace{0.15cm}\underline{ = -0.447}. $$
(4) The regression line is generally:
- $$y=\frac{\sigma_y}{\sigma_x}\cdot\rho_{xy}\cdot(x-m_x)+m_y.$$
- From the linear means $m_u = m_v = 0.5$ and the equations given in the problem statement, we obtain $m_x = 1$ and $m_y = 2$.
- The variances of $u$ and $v$ are respectively $\sigma_u^2 = \sigma_v^2 =1/12$. It follows:
- $$\sigma_x^2 = 4 \cdot \sigma_u^2 + 4 \cdot \sigma_v^2 = 2/3,$$
- $$\sigma_y^2 = \sigma_u^2 + 9\cdot \sigma_v^2 = 5/6.$$
- Substituting these values into the equation of the regression line, we get:
- $$y=\frac{\sqrt{5/6}}{\sqrt{2/3}}\cdot (\frac{-1}{\sqrt{5}})\cdot(x-1)+2= - x/{2} + 2.5.$$
- From this follows with $x=0$ the value $y_0=\hspace{0.15cm}\underline{ = 2.5}$
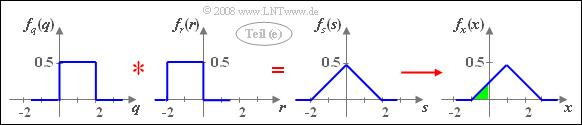
(5) With the auxiliary quantities $q= 2u$, $r= -2v$ and $s= x-1$: $s= q+r$.
- Since $u$ and $v$ are each uniformly distributed between $0$ and $1$, $q$ has a uniform distribution in the range from $0$ to $2$ and $r$ is uniformly distributed between $-2$ and $0$.
- In addition, since $q$ and $r$ are not statistically dependent on each other, the PDF of the sum is:
- $$f_s(s) = f_q(q) \star f_r(r).$$
- The addition $x = s+1$ leads to a shift of the triangular–PDF by $1$ to the right.
- For the sought probability (highlighted in green in the graphic) therefore holds:
- $${\rm Pr}(x < 0)\hspace{0.15cm}\underline{ = 0.125}.$$
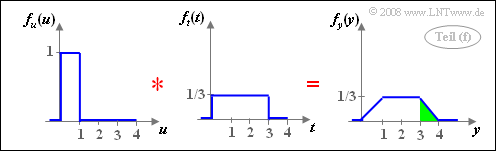
(6) Analogous to the solution for the subtask (5) holds with $t = 3v$:
- $$f_y(y) = f_u(u) \star f_t(t).$$
- The convolution between two rectangles of different widths results in a trapezoid. For the probability we are looking for, we get:
- $${\rm Pr}(y>3) =1/6\hspace{0.15cm}\underline{ \approx 0.167}.$$
- This probability is highlighted in green in the right sketch.