Difference between revisions of "Aufgaben:Exercise 3.2: Spectrum with Angle Modulation"
m (→Solution) |
|||
| (3 intermediate revisions by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Phase_Modulation_(PM) |
}} | }} | ||
| Line 7: | Line 7: | ||
* Source signal: | * Source signal: | ||
:q(t)=2V⋅sin(2π⋅3kHz⋅t), | :q(t)=2V⋅sin(2π⋅3kHz⋅t), | ||
| − | * | + | * Transmitted signal: |
:s(t)=1V⋅cos[2π⋅100kHz⋅t+KM⋅q(t)], | :s(t)=1V⋅cos[2π⋅100kHz⋅t+KM⋅q(t)], | ||
* Received signal (ideal channel): | * Received signal (ideal channel): | ||
| Line 77: | Line 77: | ||
| − | '''(2)''' An angle | + | '''(2)''' An angle modulation (PM, FM) always results in nonlinear distortion when the channel is bandlimited. |
*In contrast, double-sideband amplitude modulation (DSB-AM) here enables distortion-free transmission with BK=6 kHz ; ⇒ <u>Answer 1</u>. | *In contrast, double-sideband amplitude modulation (DSB-AM) here enables distortion-free transmission with BK=6 kHz ; ⇒ <u>Answer 1</u>. | ||
| Line 100: | Line 100: | ||
:STP(f=0)=0.765V⋅δ(f). | :STP(f=0)=0.765V⋅δ(f). | ||
*This is therefore infinite due to the Dirac function, and only the weight of the Dirac function is finite. | *This is therefore infinite due to the Dirac function, and only the weight of the Dirac function is finite. | ||
| − | *The same applies for all discrete spectral | + | *The same applies for all discrete spectral lines. |
| − | '''(5)''' S+(f) | + | '''(5)''' S+(f) is obtained from STP(f) by shifting fT to the right. Therefore |
:S+(f=97kHz)=STP(f=−3kHz)=−0.440V_. | :S+(f=97kHz)=STP(f=−3kHz)=−0.440V_. | ||
| − | * | + | *The actual spectrum differs from S+(f) by a factor of 1/2 at positive frequencies: |
:S(f=97kHz)=1/2⋅S+(f=97kHz)=−0.220V_. | :S(f=97kHz)=1/2⋅S+(f=97kHz)=−0.220V_. | ||
| − | * | + | *In general, we can write: |
:S(f)=AT2⋅+∞∑n=−∞Jn(η)⋅δ(f±(fT+n⋅fN)). | :S(f)=AT2⋅+∞∑n=−∞Jn(η)⋅δ(f±(fT+n⋅fN)). | ||
| − | '''(6)''' | + | '''(6)''' Under the suggested conditions, all the Bessel lines J|n|>3 can be disregarded. |
| − | * | + | * This gives BK=2·3·fN=18 kHz_. |
| − | '''(7)''' | + | '''(7)''' The numerical values in the table given on the exercise page show that the following channel bandwidths would now be required: |
*für η=2: BK=24 kHz_, | *für η=2: BK=24 kHz_, | ||
*für η=3: BK=36 kHz_. | *für η=3: BK=36 kHz_. | ||
Latest revision as of 17:21, 23 January 2023
The following equations are assumed here:
- Source signal:
- q(t)=2V⋅sin(2π⋅3kHz⋅t),
- Transmitted signal:
- s(t)=1V⋅cos[2π⋅100kHz⋅t+KM⋅q(t)],
- Received signal (ideal channel):
- r(t)=s(t)=1V⋅cos[2π⋅100kHz⋅t+ϕ(t)],
- ideal demodulator:
- v(t)=1KM⋅ϕ(t).
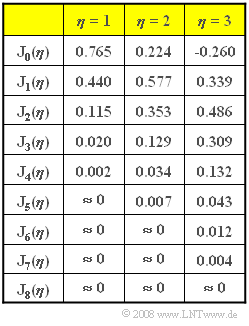
The graphs shows the n–th order Bessel functions of the first kind Jn(η) in table form.
Hints:
- This exercise belongs to the chapter Phase Modulation.
- Particular reference is made to the pages Spectral function of a phase-modulated sine signal and Interpretation of the Bessel spectrum.
Questions
Solution
(2) An angle modulation (PM, FM) always results in nonlinear distortion when the channel is bandlimited.
- In contrast, double-sideband amplitude modulation (DSB-AM) here enables distortion-free transmission with BK=6 kHz ; ⇒ Answer 1.
(3) The modulation index (or phase deviation) is equal to η=KM·AN for phase modulation.
- Thus, the modulator constant must be set to KM=1/AN=0.5⋅1/V_ to give η=1 .
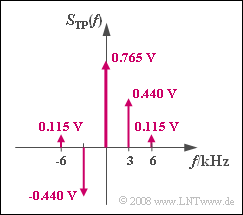
(4) A so-called Bessel spectrum is present:
- STP(f)=AT⋅+∞∑n=−∞Jn(η)⋅δ(f−n⋅fN).
- This is a discrete spectrum with components at f=n·fN, where n is an integer.
- The weights of the Dirac functions are given by the Bessel functions. When AT=1 V , one obtains:
- STP(f=0)=AT⋅J0(η=1)=0.765V_,
- STP(f=fN)=AT⋅J1(η=1)=0.440V,
- STP(f=2⋅fN)=AT⋅J2(η=1)=0.115V.
- Due to the symmetry J−n(η)=(−1)n⋅Jn(η) , the spectral line at f=−3 kHz is obtained as:
- STP(f=−fN)=−STP(f=+fN)=−0.440V_.
Note: For the spectral value at f=0 we should actually write:
- STP(f=0)=0.765V⋅δ(f).
- This is therefore infinite due to the Dirac function, and only the weight of the Dirac function is finite.
- The same applies for all discrete spectral lines.
(5) S+(f) is obtained from STP(f) by shifting fT to the right. Therefore
- S+(f=97kHz)=STP(f=−3kHz)=−0.440V_.
- The actual spectrum differs from S+(f) by a factor of 1/2 at positive frequencies:
- S(f=97kHz)=1/2⋅S+(f=97kHz)=−0.220V_.
- In general, we can write:
- S(f)=AT2⋅+∞∑n=−∞Jn(η)⋅δ(f±(fT+n⋅fN)).
(6) Under the suggested conditions, all the Bessel lines J|n|>3 can be disregarded.
- This gives BK=2·3·fN=18 kHz_.
(7) The numerical values in the table given on the exercise page show that the following channel bandwidths would now be required:
- für η=2: BK=24 kHz_,
- für η=3: BK=36 kHz_.