Difference between revisions of "Aufgaben:Exercise 4.4: About the Quantization Noise"
| (21 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Pulse_Code_Modulation |
}} | }} | ||
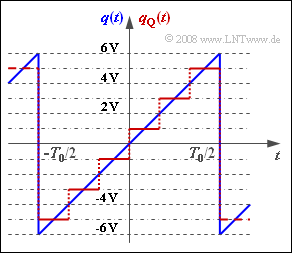
| − | [[File:P_ID1616__Mod_A_4_4.png|right|]] | + | [[File:P_ID1616__Mod_A_4_4.png|right|frame|Quantization error with sawtooth input]] |
| − | + | To calculate the quantization noise power $P_{\rm Q}$ we assume a periodic sawtooth-shaped source signal q(t) with value range $±q_{\rm max}$ and period duration T0 . | |
| + | *In the mean time domain −T0/2≤t≤T0/2 holds: $q(t) = q_{\rm max} \cdot \left ( {2 \cdot t}/{T_0} \right ).$ | ||
| + | *We refer to the power of the signal q(t) here as the transmit power $P_{\rm S}$. | ||
| − | |||
| − | |||
| − | |||
| − | + | The signal q(t) is quantized according to the graph with M = 6 steps. The quantized signal is q_{\rm Q}(t), where: | |
| + | *The linear quantizer is designed for the amplitude range $±Q_{\rm max}$ such that each quantization interval has width ${\it Δ} = 2/M \cdot Q_{\rm max}$. | ||
| + | *The diagram shows this fact for $Q_{\rm max} = q_{\rm max} = 6 \ \rm V$. These numerical values shall be assumed up to and including the subtask '''(5)'''. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
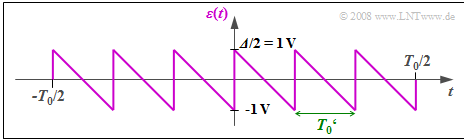
| − | + | The "quantization noise power" is defined as the second moment of the difference signal ε(t) = q_{\rm Q}(t) - q(t). It holds: | |
| + | :$$P_{\rm Q} = \frac{1}{T_0' } \cdot \int_{0}^{T_0'}\varepsilon(t)^2 \hspace{0.05cm}{\rm d}t \hspace{0.05cm},$$ | ||
| + | where the time $T_0'$ is to be chosen appropriately. The "quantization SNR" is the ratio $\rho_{\rm Q} = {P_{\rm S}}/{P_{\rm Q}}\hspace{0.05cm}$, which is usually given logarithmically (in dB). | ||
| − | === | + | |
| + | |||
| + | |||
| + | |||
| + | |||
| + | Hints: | ||
| + | *The exercise belongs to the chapter [[Modulation_Methods/Pulse_Code_Modulation|"Pulse Code Modulation"]]. | ||
| + | *Reference is made in particular to the page [[Modulation_Methods/Pulse_Code_Modulation#Quantization_and_quantization_noise|"Quantization and quantization Noise"]]. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the signal power $P_{\rm S}$ $(referred to the resistor 1 \ \rm Ω)$. |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $P_{\rm S} \ = \ { 12 3% } \ \rm V^2$ |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | {Which statements are true for the error signal ε(t)= q_{\rm Q}(t)-q(t) ? | ||
| + | |type="[]"} | ||
| + | + ε(t) has a sawtooth shape. | ||
| + | - ε(t) has a step-like progression. | ||
| + | + ε(t) is restricted to the range ±{\it Δ}/2 = ±1 \ \rm V. | ||
| + | + ε(t) has period T_0' = T_0/M. | ||
| − | { | + | {What is the quantization noise power P_{\rm Q} for M=6? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $P_{\rm Q} \ = \ { 0.333 3% } \ \rm V^2$ |
| − | { | + | {Calculate the quantization noise ratio for M = 6. |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $10 \cdot \lg \ ρ_{\rm Q} \ = \ { 15.56 3% } \ \rm dB$ |
| − | { | + | {What values result from quantization with N = 8 or N = 16 bits? |
|type="{}"} | |type="{}"} | ||
| − | $ N = 8: | + | $N = 8\text{:}\hspace{0.35cm}10 ⋅ \lg \ ρ_{\rm Q} \ = \ { 48.16 3% } \ \rm dB$ |
| − | $ N = 16: | + | $N = 16\text{:}\hspace{0.15cm}10 ⋅ \lg \ ρ_{\rm Q} \ = \ { 96.32 3% } \ \rm dB$ |
| − | { | + | {What conditions must be met for the derived equation to apply to $ρ_{\rm Q}$? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + All amplitude values are equally probable. |
| − | + | + | + A linear quantizer is present. |
| − | + | + | + The quantizer is exactly matched to the signal $(Q_{\rm max} = q_{\rm max})$. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' The signal power $P_{\rm S} $ is equal to the second moment of q(t) if the reference resistance 1 \rm Ω is used and therefore the unit $\rm V^2$ is accepted for the power. |
| − | $$P_{\rm S} | + | *Due to periodicity and symmetry, averaging over the time domain T_0/2 is sufficient: |
| − | + | :$$P_{\rm S} = \frac{1}{T_0/2} \cdot \int_{0}^{T_0/2}q^2(t) \hspace{0.05cm}{\rm d}t = \frac{2 \cdot q_{\rm max}^2}{T_0} \cdot \int_{0}^{T_0/2}\left ( { 2 \cdot t}/{T_0} \right )^2 \hspace{0.05cm}{\rm d}t= \frac{2 \cdot q_{\rm max}^2}{T_0} \cdot \frac{T_0}{2} \cdot \int_{0}^{1}x^2 \hspace{0.05cm}{\rm d}x = \frac{q_{\rm max}^2}{3} \hspace{0.05cm}.$$ | |
| + | *Here the substitution $x = 2 - t/T_0$ was used. With $q_{\rm max} = 6 \ \rm V one gets P_\rm S\hspace{0.15cm}\underline { = 12 \ V^2}$. | ||
| + | |||
| + | |||
| + | |||
| + | [[File:Mod_A_4_4b_neu.png|right|frame|Error signal for Q_{\rm max} = q_{\rm max}]] | ||
| + | '''(2)''' Correct are <u>suggested solutions 1, 3, and 4</u>: | ||
| + | *We assume here Q_{\rm max} = q_{\rm max} = 6 \ \rm V. | ||
| + | *This gives the sawtooth-shaped error signal ε(t) between ±1\ \rm V. | ||
| + | *The period duration is T_0' = T_0/6. | ||
| + | |||
| + | |||
| + | |||
| + | '''(3)''' The error signal ε(t) proceeds in the same way as q(t) sawtooth. | ||
| + | *Thus, the same equation as in subtask '''(1)''' is suitable for calculating the power. | ||
| + | *Note, however, that the amplitude is smaller by a factor M while the different period duration does not matter for the averaging: | ||
| + | :$$P_{\rm Q} = \frac{P_{\rm S}}{M^2} = \frac{12\,{\rm V}^2}{36}\hspace{0.15cm}\underline {= 0.333\,{\rm V}^2 }\hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | |||
| + | '''(4)''' The results of the subtasks '''(1)''' and '''(3)''' lead to the quantization SNR: | ||
| + | :\rho_{\rm Q} = \frac{P_{\rm S}}{P_{\rm Q}} = M^2 = 36 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q}\hspace{0.15cm}\underline { =15.56\,{\rm dB}} \hspace{0.05cm}. | ||
| + | |||
| + | |||
| + | |||
| + | '''(5)''' With M = 2^N we obtain in general: | ||
| + | : \rho_{\rm Q} = M^2 = 2^{2N} \hspace{0.3cm}\rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q} =20 \cdot {\rm lg}\hspace{0.1cm}(2)\cdot N \approx 6.02\,{\rm dB} \cdot N . | ||
| + | *This results in the special cases we are looking for: | ||
| + | :N = 8:\hspace{0.2cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q} \hspace{0.15cm}\underline {= 48.16\,{\rm dB}}\hspace{0.05cm}, | ||
| + | :N = 16:\hspace{0.2cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q} \hspace{0.15cm}\underline { = 96.32\,{\rm dB}}\hspace{0.05cm}. | ||
| + | |||
| + | |||
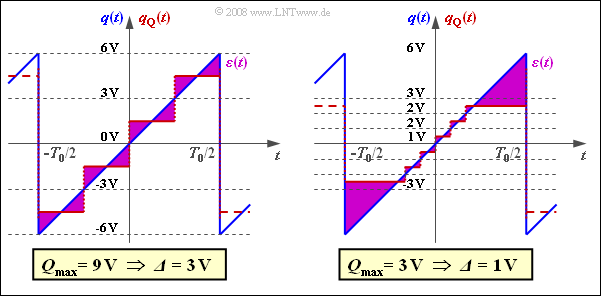
| + | [[File:P_ID1618__Mod_A_4_4f.png|right|frame|Quantization with Q_{\rm max} \ne q_{\rm max}]] | ||
| + | '''(6)''' <u>All of the above preconditions</u> must be satisfied: | ||
| + | *For non-linear quantization, the simple relation $ρ_{\rm Q} = M^2$ does not hold. | ||
| + | *For an PDF other than the uniform distribution ρ_{\rm Q} = M^2 is also only an approximation, but this is usually accepted. | ||
| + | *If Q_{\rm max} < q_{\rm max}, truncation of the peaks occurs, while with Q_{\rm max} > q_{\rm max} the quantization intervals are larger than required. | ||
| + | |||
| + | |||
| + | The graph shows the error signals ε(t) | ||
| + | #for Q_{\rm max} > q_{\rm max} (left) | ||
| + | #and Q_{\rm max} < q_{\rm max} (right): | ||
| + | |||
| + | |||
| + | :In both cases, the quantization noise power is significantly larger than calculated in sub-task '''(3)'''. | ||
| + | |||
| + | |||
| + | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Modulation Methods: Exercises|^4.1 Pulse Code Modulation^]] |
Latest revision as of 15:57, 11 April 2022
To calculate the quantization noise power P_{\rm Q} we assume a periodic sawtooth-shaped source signal q(t) with value range ±q_{\rm max} and period duration T_0 .
- In the mean time domain -T_0/2 ≤ t ≤ T_0/2 holds: q(t) = q_{\rm max} \cdot \left ( {2 \cdot t}/{T_0} \right ).
- We refer to the power of the signal q(t) here as the transmit power P_{\rm S}.
The signal q(t) is quantized according to the graph with M = 6 steps. The quantized signal is q_{\rm Q}(t), where:
- The linear quantizer is designed for the amplitude range ±Q_{\rm max} such that each quantization interval has width {\it Δ} = 2/M \cdot Q_{\rm max}.
- The diagram shows this fact for Q_{\rm max} = q_{\rm max} = 6 \ \rm V. These numerical values shall be assumed up to and including the subtask (5).
The "quantization noise power" is defined as the second moment of the difference signal ε(t) = q_{\rm Q}(t) - q(t). It holds:
- P_{\rm Q} = \frac{1}{T_0' } \cdot \int_{0}^{T_0'}\varepsilon(t)^2 \hspace{0.05cm}{\rm d}t \hspace{0.05cm},
where the time T_0' is to be chosen appropriately. The "quantization SNR" is the ratio \rho_{\rm Q} = {P_{\rm S}}/{P_{\rm Q}}\hspace{0.05cm}, which is usually given logarithmically (in dB).
Hints:
- The exercise belongs to the chapter "Pulse Code Modulation".
- Reference is made in particular to the page "Quantization and quantization Noise".
Questions
Solution
- Due to periodicity and symmetry, averaging over the time domain T_0/2 is sufficient:
- P_{\rm S} = \frac{1}{T_0/2} \cdot \int_{0}^{T_0/2}q^2(t) \hspace{0.05cm}{\rm d}t = \frac{2 \cdot q_{\rm max}^2}{T_0} \cdot \int_{0}^{T_0/2}\left ( { 2 \cdot t}/{T_0} \right )^2 \hspace{0.05cm}{\rm d}t= \frac{2 \cdot q_{\rm max}^2}{T_0} \cdot \frac{T_0}{2} \cdot \int_{0}^{1}x^2 \hspace{0.05cm}{\rm d}x = \frac{q_{\rm max}^2}{3} \hspace{0.05cm}.
- Here the substitution x = 2 - t/T_0 was used. With q_{\rm max} = 6 \ \rm V one gets P_\rm S\hspace{0.15cm}\underline { = 12 \ V^2}.
(2) Correct are suggested solutions 1, 3, and 4:
- We assume here Q_{\rm max} = q_{\rm max} = 6 \ \rm V.
- This gives the sawtooth-shaped error signal ε(t) between ±1\ \rm V.
- The period duration is T_0' = T_0/6.
(3) The error signal ε(t) proceeds in the same way as q(t) sawtooth.
- Thus, the same equation as in subtask (1) is suitable for calculating the power.
- Note, however, that the amplitude is smaller by a factor M while the different period duration does not matter for the averaging:
- P_{\rm Q} = \frac{P_{\rm S}}{M^2} = \frac{12\,{\rm V}^2}{36}\hspace{0.15cm}\underline {= 0.333\,{\rm V}^2 }\hspace{0.05cm}.
(4) The results of the subtasks (1) and (3) lead to the quantization SNR:
- \rho_{\rm Q} = \frac{P_{\rm S}}{P_{\rm Q}} = M^2 = 36 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q}\hspace{0.15cm}\underline { =15.56\,{\rm dB}} \hspace{0.05cm}.
(5) With M = 2^N we obtain in general:
- \rho_{\rm Q} = M^2 = 2^{2N} \hspace{0.3cm}\rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q} =20 \cdot {\rm lg}\hspace{0.1cm}(2)\cdot N \approx 6.02\,{\rm dB} \cdot N .
- This results in the special cases we are looking for:
- N = 8:\hspace{0.2cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q} \hspace{0.15cm}\underline {= 48.16\,{\rm dB}}\hspace{0.05cm},
- N = 16:\hspace{0.2cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm Q} \hspace{0.15cm}\underline { = 96.32\,{\rm dB}}\hspace{0.05cm}.
(6) All of the above preconditions must be satisfied:
- For non-linear quantization, the simple relation ρ_{\rm Q} = M^2 does not hold.
- For an PDF other than the uniform distribution ρ_{\rm Q} = M^2 is also only an approximation, but this is usually accepted.
- If Q_{\rm max} < q_{\rm max}, truncation of the peaks occurs, while with Q_{\rm max} > q_{\rm max} the quantization intervals are larger than required.
The graph shows the error signals ε(t)
- for Q_{\rm max} > q_{\rm max} (left)
- and Q_{\rm max} < q_{\rm max} (right):
- In both cases, the quantization noise power is significantly larger than calculated in sub-task (3).