| Line 518: | Line 518: | ||
</table> | </table> | ||
| + | == Polynome über einem endlichen Körper (1) == | ||
| + | <br> | ||
| + | Ein Polynom in einem endlichen Körper GF(<i>P</i>), wobei <i>P</i> eine Primzahl angibt, hat folgende Form: | ||
| + | :<math>a(x) = \sum_{i = 0}^{m} a_i \cdot x^{i} = a_0 + a_1 \cdot x + a_2 \cdot x^2 + \hspace{0.1cm}... \hspace{0.1cm} + a_m \cdot x^{m} | ||
| + | \hspace{0.05cm}.</math> | ||
| + | <br>Anzumerken ist: | ||
| + | *Alle Koeffizienten sind Elemente des Körpers: <i>a<sub>i</sub></i> ∈ GF(<i>P</i>).<br> | ||
| + | |||
| + | *Ist der führende Koeffizient <i>a<sub>m</sub></i> ≠ 0, so gibt <i>m</i> den Grad des Polynoms an.<br><br> | ||
| + | |||
| + | Betrachten wir ein dazu zweites Polynom mit Grad <i>M</i>, | ||
| + | |||
| + | :<math>b(x) = \sum_{i = 0}^{M} b_i \cdot x^{i} = b_0 + b_1 \cdot x + b_2 \cdot x^2 + \hspace{0.1cm}... \hspace{0.1cm} + b_M \cdot x^{M} | ||
| + | \hspace{0.05cm},</math> | ||
| + | |||
| + | so erhält man für die Summe (bzw. Differenz) und das Produkt jeweils in GF(<i>P</i>): | ||
| + | |||
| + | :<math>a(x) \pm b(x) \hspace{-0.15cm} = \hspace{-0.15cm} \sum_{i = 0}^{{\rm max}\hspace{0.05cm}(m, \hspace{0.05cm}M)} \hspace{0.15cm}(a_i \pm b_i) \cdot x^{i} \hspace{0.05cm},</math> | ||
| + | :<math>a(x) \cdot b(x) = \hspace{-0.15cm} \sum_{i = 0}^{m + M} \hspace{0.15cm}c_i \cdot x^{i}\hspace{0.05cm},\hspace{0.5cm} | ||
| + | c_i = \sum_{j = 0}^{i}\hspace{0.15cm}a_j \cdot b_{i-j} | ||
| + | \hspace{0.05cm}.</math> | ||
| + | |||
| + | {{Beispiel}}''':''' Es gelte <i>a</i>(<i>x</i>) = <i>x</i><sup>3</sup> + <i>x</i> + 1 und <i>b</i>(<i>x</i>) = <i>x</i><sup>2</sup> + <i>x</i> + 1. Im binären Galoisfeld ⇒ GF(2) ergibt sich nach den obigen Gleichungen für die Summe, die Differenz und das Produkt der beiden Polynome: | ||
| + | |||
| + | :<math>s(x) = a(x) + b(x) = x^3 + x^2 \hspace{0.05cm}, \hspace{0.5cm} | ||
| + | d(x) = a(x) - b(x) = x^3 + x^2 = s(x)\hspace{0.05cm},</math> | ||
| + | |||
| + | :<math>c(x) = a(x) \cdot b(x) =\sum_{i = 0}^{3 + 2} \hspace{0.15cm}c_i \cdot x^{i}\hspace{0.05cm},\hspace{0.5cm} | ||
| + | c_i = \sum_{j = 0}^{i}\hspace{0.15cm}a_j \cdot b_{i-j} | ||
| + | \hspace{0.05cm}.</math> | ||
| + | |||
| + | Mit <i>a</i><sub>0</sub> = <i>a</i><sub>1</sub> = <i>a</i><sub>3</sub> = <i>b</i><sub>0</sub> = <i>b</i><sub>1</sub> = <i>b</i><sub>2</sub> = 1 und <i>a</i><sub>2</sub> = <i>a</i><sub>4</sub> = <i>a</i><sub>5</sub> = <i>b</i><sub>3</sub> = <i>b</i><sub>4</sub> = <i>b</i><sub>5</sub> = 0 erhält man: | ||
| + | |||
| + | :<math>c_0 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_0 = 1 \cdot 1 = 1 \hspace{0.05cm},</math> | ||
| + | :<math>c_1 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_1 + a_1 \cdot b_0 = 1 \cdot 1 + 1 \cdot 1 = 0 \hspace{0.05cm},</math> | ||
| + | :<math>c_2 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_2 + a_1 \cdot b_1 + a_2 \cdot b_0 = 1 \cdot 1 + 1 \cdot 1 + 0 \cdot 1 = 0 \hspace{0.05cm},</math> | ||
| + | :<math>c_3 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_3 + a_1 \cdot b_2 + a_2 \cdot b_1 + a_3 \cdot b_0 | ||
| + | = 1 \cdot 0 + 1 \cdot 1 + 0 \cdot 1 + 1 \cdot 1 = 0 \hspace{0.05cm},</math> | ||
| + | :<math>c_4 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_4 + a_1 \cdot b_3 + \hspace{0.05cm}...+ \hspace{0.05cm}a_4 \cdot b_0 | ||
| + | =1 \cdot 0 + 1 \cdot 0 + 0 \cdot 1 + 1 \cdot 1 + 0 \cdot 1 = 1 \hspace{0.05cm},</math> | ||
| + | :<math>c_5 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_5 + a_1 \cdot b_4 + \hspace{0.05cm}...+ \hspace{0.05cm} a_5 \cdot b_0 | ||
| + | =1 \cdot 0 + 1 \cdot 0 + 0 \cdot 0 + 1 \cdot 1 + 0 \cdot 1 + 0 \cdot 1= 1 </math> | ||
| + | |||
| + | :<math>\Rightarrow \hspace{0.3cm} c(x) = x^5 + x^4 +1 \hspace{0.05cm}.</math> | ||
| + | |||
| + | Im Galoisfeld GF(3) erhält man aufgrund der Modulo–3–Operationen andere Ergebnisse: | ||
| + | |||
| + | :<math>s(x) \hspace{-0.15cm} = \hspace{-0.15cm} (x^3 + x + 1) + (x^2 + x + 1) = x^3 + x^2 + 2x + 2\hspace{0.05cm},</math> | ||
| + | :<math>d(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^3 + x + 1) - (x^2 + x + 1) = x^3 + 2x^2 \hspace{0.05cm},</math> | ||
| + | :<math>c(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^3 + x + 1) \cdot (x^2 + x + 1) = x^5 + x^4 + 2x^3 + 2x^2 + 2x +1\hspace{0.05cm}.</math>{{end}}<br> | ||
| + | |||
| + | == Polynome über einem endlichen Körper (2) == | ||
| + | <br> | ||
| + | {{Definition}}''':''' Ein Polynom <i>a</i>(<i>x</i>) bezeichnet man als reduzibel (englisch: <i>reducible</i>), wenn es als Produkt zweier Polynome <i>p</i>(<i>x</i>) und <i>q</i>(<i>x</i>) mit jeweils niedrigerem Grad dargestellt werden kann: | ||
| + | |||
| + | :<math>a(x) = p(x) \cdot q(x) | ||
| + | \hspace{0.05cm}.</math> | ||
| + | |||
| + | Ist diese Faktorisierung nicht möglich, das heißt, wenn | ||
| + | |||
| + | :<math>a(x) = p(x) \cdot q(x) + r(x)\hspace{0.05cm},\hspace{0.5cm} r(x) \ne 0</math> | ||
| + | |||
| + | gilt, so spricht man von einem irreduziblen (englisch: <i>irreducible</i> oder <i>prime</i>) Polynom.{{end}}<br> | ||
| + | |||
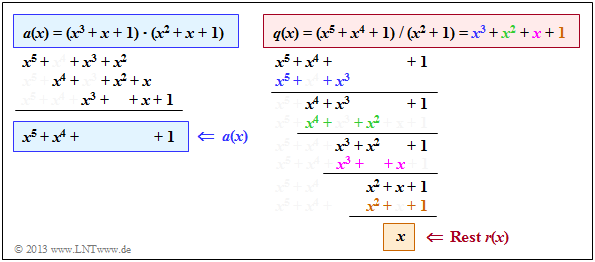
| + | {{Beispiel}}''':''' Es gelte <i>b</i>(<i>x</i>) = <i>x</i><sup>3</sup> + <i>x</i> + 1, <i>p</i><sub>1</sub>(<i>x</i>) = <i>x</i><sup>2</sup> + <i>x</i> + 1 und <i>p</i><sub>2</sub>(<i>x</i>) = <i>x</i><sup>2</sup> + 1. Die Grafik verdeutlicht links die Modulo–2–Multiplikation <i>a</i>(<i>x</i>) = <i>b</i>(<i>x</i>) · <i>p</i><sub>1</sub>(<i>x</i>). Das Ergebnis ist <i>a</i>(<i>x</i>) = <i>x</i><sup>5</sup> + <i>x</i><sup>4</sup> + 1.<br> | ||
| + | |||
| + | [[File:P ID2538 KC T 2 2 S2 v2.png|Beispiel für Polynom–Multiplikation und –Division|class=fit]]<br> | ||
| + | |||
| + | Im rechten Teil der obigen Grafik ist die Modulo–2–Division <i>q</i>(<i>x</i>) = <i>a</i>(<i>x</i>)/<i>p</i><sub>2</sub>(<i>x</i>) mit dem Ergebnis <i>q</i>(<i>x</i>) = <i>x</i><sup>3</sup> + <i>x</i><sup>2</sup> + <i>x</i> + 1 dargestellt. Es verbleibt der Rest <i>r</i>(<i>x</i>) = <i>x</i>. Allein nach dieser Rechnung könnte <i>a</i>(<i>x</i>) = <i>x</i><sup>5</sup> + <i>x</i><sup>4</sup> + 1 durchaus ein irreduzibles Polynom sein.<br> | ||
| + | |||
| + | Der Nachweis, dass das Polynom <i>a</i>(<i>x</i>) = <i>x</i><sup>5</sup> + <i>x</i><sup>4</sup> + 1 tatsächlich irreduzibel ist, wäre allerdings erst dann erbracht, wenn <i>a</i>(<i>x</i>)/<i>p</i>(<i>x</i>) für alle | ||
| + | |||
| + | :<math>p(x) = \sum_{i = 0}^{m} a_i \cdot x^{i} = a_m \cdot x^{m} + a_{m-1} \cdot x^{m-1} + \hspace{0.1cm}... \hspace{0.1cm}+ a_2 \cdot x^2 + a_1 \cdot x + a_0 \hspace{0.05cm}</math> | ||
| + | |||
| + | einen Rest <i>r</i>(<i>x</i>) ≠ 0 liefert. Dies würde im vorliegenden Beispiel (nahezu) 2<sup>5</sup> = 32 Divisionen erfordern.<br> | ||
| + | |||
| + | Aufgrund unserer linken Berechnung können wir hier sofort erkennen, dass <i>a</i>(<i>x</i>) mit Sicherheit kein irreduzibles Polynom ist, da zum Beispiel <i>a</i>(<i>x</i>) = <i>x</i><sup>5</sup> + <i>x</i><sup>4</sup> + 1 dividiert durch <i>p</i><sub>1</sub>(<i>x</i>) = <i>x</i><sup>2</sup> + <i>x</i> + 1 das Polynom <nobr><i>b</i>(<i>x</i>) = <i>x</i><sup>3</sup> + <i>x</i> + 1</nobr> ohne Rest ergibt.{{end}}<br> | ||
{{Display}} | {{Display}} | ||
Revision as of 00:05, 13 January 2017
Contents
GF(22) – Beispiel eines Erweiterungskörpers (1)
Im Kapitel 2.1 wurde bereits gezeigt, dass die endliche Zahlenmenge {0, 1, 2, 3} ⇒ q = 4 nicht die Eigenschaften eines Galoisfeldes GF(4) erfüllt. Vielmehr ergeben sich für die Addition modulo 4 und die Multiplikation modulo 4 folgende Tabellen:
\[{\rm Operationen } \hspace{0.15cm}{\rm modulo}\hspace{0.15cm}{\it q} = 4 \hspace{0.25cm} \Rightarrow\hspace{0.25cm}\]
|
|
Für zi = 2 gibt es keine multiplikative Inverse InvM(zi). Dies erkennt man daran, dass kein einziges Element zj ∈ {0, 1, 2, 3} die Bedingung 2 · zj = 1 erfüllt. Geht man dagegen vom binären Galoisfeld GF(2) = {0, 1} aus und erweitert dieses entsprechend der Gleichung
\[{\rm GF}(2^2)= \big\{k_0+k_1\cdot \alpha \ | \ k_0, k_1\in{\rm GF}(2) = \{ 0, 1\} \big \}\hspace{0.05cm}, \]
so ergibt sich die ebenfalls endliche Menge {0, 1, α, 1 + α} ⇒ die Ordnung ist weiterhin q = 4. Führt man die Rechenoperationen modulo p(α) = α2 + α + 1 durch, so erhält man das folgende Ergebnis:
\[{\rm modulo} \hspace{0.15cm} {\it p} (\alpha) \hspace{0.25cm}\Rightarrow \]
|
|
Hierzu ist anzumerken:
- Die neutralen Elemente der Addition bzw. Multiplikation sind weiterhin NA = 0 und NM = 1.
- Da bei Modulo–Ausführung kein Unterschied zwischen Addition und Subtraktion besteht, ist α + α = α – α = 0. Für alle zi gilt somit: Die additive Inverse von zi ist das Element zi selbst.
- Die Einträge in der Multiplikationstabelle ergeben sich nach folgenden Berechnungen:
- \[\big [ \alpha \cdot (1+\alpha) \big ] \hspace{0.15cm}{\rm mod} \hspace{0.15cm} p(\alpha) \hspace{-0.15cm} = \hspace{-0.15cm} (\alpha^2 + \alpha) \hspace{0.15cm}{\rm mod} \hspace{0.15cm} (\alpha^2 + \alpha + 1)= 1\hspace{0.05cm},\]
- \[\big [ \alpha \cdot \alpha \big ] \hspace{0.15cm}{\rm mod} \hspace{0.15cm} p(\alpha) \hspace{-0.15cm} = \hspace{-0.15cm} (\alpha^2 ) \hspace{0.15cm}{\rm mod} \hspace{0.15cm} (\alpha^2 + \alpha + 1)= 1+\alpha\hspace{0.05cm},\]
- \[\big [ (1+\alpha) \cdot (1+\alpha) \big ] \hspace{0.15cm}{\rm mod} \hspace{0.15cm} p(\alpha) \hspace{-0.15cm} = \hspace{-0.15cm} (\alpha^2 + 1) \hspace{0.15cm}{\rm mod} \hspace{0.15cm} (\alpha^2 + \alpha + 1)= \alpha\hspace{0.05cm}.\]
- Damit existieren für alle Elemente mit Ausnahme des Nullelements die multiplikativen Inversen:
- \[{\rm Inv_M}( 1) = 1 \hspace{0.05cm},\hspace{0.2cm}{\rm Inv_M}(\alpha) = 1+\alpha \hspace{0.05cm},\hspace{0.2cm}{\rm Inv_M}(1+\alpha) = \alpha \hspace{0.05cm}.\]
Daraus folgt: Die Menge {0, 1, α, 1 + α} stellt zusammen mit den zwei Rechenoperationen Addition und Multiplikation modulo p(α) = α2 + α + 1 ein Galoisfeld der Ordnung q = 4 dar. Dieses mit GF(22) = GF(4) bezeichnete Galoisfeld erfüllt alle in Kapitel 2.1 genannten Eigenschaften.
Im Gegensatz zum Zahlenkörper GF(3) = {0, 1, 2} mit der Eigenschaft, dass q = 3 eine Primzahl ist, nennt man GF(22) einen Erweiterungskörper (englisch: Extension Field).
GF(22) – Beispiel eines Erweiterungskörpers (2)
Das Polynom p(α) und damit die Bestimmungsgleichung p(α) = 0 darf nicht beliebig vorgegeben werden. Zum Beleg hierfür vergleichen wir die Verknüpfungstabellen für zwei verschiedene Polynome.
Polynom entsprechend der letzten Seite ⇒ p(α) = α2 + α + 1:
|
|
Neuer (allerdings ungeeigneter) Ansatz ⇒ p(α) = α2 + 1:
|
|
Die Additionstabelle ist in beiden Fällen identisch und auch die Multiplikationstabellen unterscheiden sich nur durch die vier Einträge in den beiden unteren Zeilen und den beiden hinteren Spalten:
- Aus p(α) = 0 folgt nun für das Produkt α · α = 1 und das Produkt (1+α) · (1+α) ergibt das Element 0. Das gemischte Produkt α · (1+α) ist nun 1+α.
- In der letzten Zeile der Multipliaktionstabelle und auch in der letzten Spalte steht nun keine „1” ⇒ Hinsichtlich der Bedingung p(α) = α2 + 1 = 0 existiert die multiplikative Inverse zu 1+α nicht.
- Damit erfüllt aber die endliche Menge {0, 1, α, 1+α} zusammen mit Rechenoperationen modulo p(α) = α2 + 1 auch nicht die Voraussetzungen eines Erweiterungskörpers GF(22).
Fassen wir zusammen: Aus dem binären Galoisfeld GF(2) = {0, 1} lässt sich unter Zuhilfenahme eines Polynoms vom Grad m = 2 mit binären Koeffizienten,
\[p(x) = x^2 + k_1 \cdot x + k_0 \hspace{0.05cm}, \hspace{0.45cm}k_0\hspace{0.05cm},\hspace{0.1cm}k_1 \in \{0, 1\} \hspace{0.05cm},\]
ein Erweiterungskörper GF(22) formulieren. Im vorliegenden Fall gibt es nur ein geeignetes Polynom p1(x) = x2 + x + 1. Alle anderen möglichen Polynome vom Grad m = 2, nämlich
\[p_2(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^2 + 1 \hspace{0.06cm} = (x+1) \cdot (x+1)\hspace{0.05cm},\] \[p_3(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^2 \hspace{0.76cm} = x \cdot x \hspace{0.05cm},\] \[p_4(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^2 + x = (x+1) \cdot x \]
lassen sich faktorisieren und ergeben keinen Erweiterungskörper. Man nennt die Polynome p2(x), p3(x) und p4(x) reduzibel.
Anmerkung: Die Umbenennung der Funktionsvariablen α in x hat nur formale Bedeutung im Hinblick auf die folgenden Seiten.
GF(22) – Beispiel eines Erweiterungskörpers (3)
Wir betrachten weiterhin den Körper GF(22) = {0, 1, α, 1+α} entsprechend den beiden folgenden Operationstabellen, basierend auf der Nebenbedingung p(α) = α2 + α + 1 = 0 (irreduzibles Ploynom):
|
|
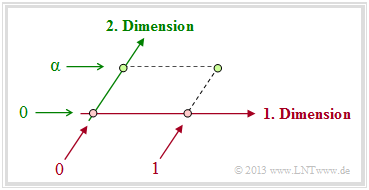
Welche Bedeutung hat aber nun das neue Element α?
- Das Polynom p(α) = α2 + α + 1 hat keine Nullstelle in GF(2) = {0, 1}. Das bedeutet weiter, dass α weder 0 noch 1 sein kann.
- Wäre α = 0 bzw. α = 1, so wären zudem zwei der vier Mengenelemente {0, 1, α, 1+α} jeweils identisch. Entweder „0” und „α” sowie „1” und „1+α” oder „1” und „α” sowie „0” und „1+α”.
- Vielmehr erhält der eindimensionale Körper GF(2) durch die Einführung des Elementes α eine zweite Dimension. Er wird also zum Galoisfeld GF(22) erweitert, wie die folgende Grafik zeigt.
- Das Element α hat ähnliche Bedeutung wie die imaginäre Einheit j, durch die man die Menge der reellen Zahlen unter der Nebenbedingung j2 + 1 = 0 zur Menge der komplexen Zahlen erweitert.
- Aufgrund der Identität α2 = 1 + α, die aus der Nebenbedingung p(α) = 0 folgt, kann man in gleicher Weise GF(22) = {0, 1, α, α2} schreiben, wobei nun folgende Operationstabellen gelten:
|
|
Polynome über einem endlichen Körper (1)
Ein Polynom in einem endlichen Körper GF(P), wobei P eine Primzahl angibt, hat folgende Form:
\[a(x) = \sum_{i = 0}^{m} a_i \cdot x^{i} = a_0 + a_1 \cdot x + a_2 \cdot x^2 + \hspace{0.1cm}... \hspace{0.1cm} + a_m \cdot x^{m} \hspace{0.05cm}.\]
Anzumerken ist:
- Alle Koeffizienten sind Elemente des Körpers: ai ∈ GF(P).
- Ist der führende Koeffizient am ≠ 0, so gibt m den Grad des Polynoms an.
Betrachten wir ein dazu zweites Polynom mit Grad M,
\[b(x) = \sum_{i = 0}^{M} b_i \cdot x^{i} = b_0 + b_1 \cdot x + b_2 \cdot x^2 + \hspace{0.1cm}... \hspace{0.1cm} + b_M \cdot x^{M} \hspace{0.05cm},\]
so erhält man für die Summe (bzw. Differenz) und das Produkt jeweils in GF(P):
\[a(x) \pm b(x) \hspace{-0.15cm} = \hspace{-0.15cm} \sum_{i = 0}^{{\rm max}\hspace{0.05cm}(m, \hspace{0.05cm}M)} \hspace{0.15cm}(a_i \pm b_i) \cdot x^{i} \hspace{0.05cm},\] \[a(x) \cdot b(x) = \hspace{-0.15cm} \sum_{i = 0}^{m + M} \hspace{0.15cm}c_i \cdot x^{i}\hspace{0.05cm},\hspace{0.5cm} c_i = \sum_{j = 0}^{i}\hspace{0.15cm}a_j \cdot b_{i-j} \hspace{0.05cm}.\]
\[s(x) = a(x) + b(x) = x^3 + x^2 \hspace{0.05cm}, \hspace{0.5cm} d(x) = a(x) - b(x) = x^3 + x^2 = s(x)\hspace{0.05cm},\]
\[c(x) = a(x) \cdot b(x) =\sum_{i = 0}^{3 + 2} \hspace{0.15cm}c_i \cdot x^{i}\hspace{0.05cm},\hspace{0.5cm} c_i = \sum_{j = 0}^{i}\hspace{0.15cm}a_j \cdot b_{i-j} \hspace{0.05cm}.\]
Mit a0 = a1 = a3 = b0 = b1 = b2 = 1 und a2 = a4 = a5 = b3 = b4 = b5 = 0 erhält man:
\[c_0 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_0 = 1 \cdot 1 = 1 \hspace{0.05cm},\] \[c_1 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_1 + a_1 \cdot b_0 = 1 \cdot 1 + 1 \cdot 1 = 0 \hspace{0.05cm},\] \[c_2 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_2 + a_1 \cdot b_1 + a_2 \cdot b_0 = 1 \cdot 1 + 1 \cdot 1 + 0 \cdot 1 = 0 \hspace{0.05cm},\] \[c_3 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_3 + a_1 \cdot b_2 + a_2 \cdot b_1 + a_3 \cdot b_0 = 1 \cdot 0 + 1 \cdot 1 + 0 \cdot 1 + 1 \cdot 1 = 0 \hspace{0.05cm},\] \[c_4 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_4 + a_1 \cdot b_3 + \hspace{0.05cm}...+ \hspace{0.05cm}a_4 \cdot b_0 =1 \cdot 0 + 1 \cdot 0 + 0 \cdot 1 + 1 \cdot 1 + 0 \cdot 1 = 1 \hspace{0.05cm},\] \[c_5 \hspace{-0.25cm} = \hspace{-0.15cm} a_0 \cdot b_5 + a_1 \cdot b_4 + \hspace{0.05cm}...+ \hspace{0.05cm} a_5 \cdot b_0 =1 \cdot 0 + 1 \cdot 0 + 0 \cdot 0 + 1 \cdot 1 + 0 \cdot 1 + 0 \cdot 1= 1 \]
\[\Rightarrow \hspace{0.3cm} c(x) = x^5 + x^4 +1 \hspace{0.05cm}.\]
Im Galoisfeld GF(3) erhält man aufgrund der Modulo–3–Operationen andere Ergebnisse:
\[s(x) \hspace{-0.15cm} = \hspace{-0.15cm} (x^3 + x + 1) + (x^2 + x + 1) = x^3 + x^2 + 2x + 2\hspace{0.05cm},\] \[d(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^3 + x + 1) - (x^2 + x + 1) = x^3 + 2x^2 \hspace{0.05cm},\]
\[c(x) \hspace{-0.15cm} = \hspace{-0.15cm} x^3 + x + 1) \cdot (x^2 + x + 1) = x^5 + x^4 + 2x^3 + 2x^2 + 2x +1\hspace{0.05cm}.\]
Polynome über einem endlichen Körper (2)
\[a(x) = p(x) \cdot q(x) \hspace{0.05cm}.\]
Ist diese Faktorisierung nicht möglich, das heißt, wenn
\[a(x) = p(x) \cdot q(x) + r(x)\hspace{0.05cm},\hspace{0.5cm} r(x) \ne 0\]
gilt, so spricht man von einem irreduziblen (englisch: irreducible oder prime) Polynom.
Im rechten Teil der obigen Grafik ist die Modulo–2–Division q(x) = a(x)/p2(x) mit dem Ergebnis q(x) = x3 + x2 + x + 1 dargestellt. Es verbleibt der Rest r(x) = x. Allein nach dieser Rechnung könnte a(x) = x5 + x4 + 1 durchaus ein irreduzibles Polynom sein.
Der Nachweis, dass das Polynom a(x) = x5 + x4 + 1 tatsächlich irreduzibel ist, wäre allerdings erst dann erbracht, wenn a(x)/p(x) für alle
\[p(x) = \sum_{i = 0}^{m} a_i \cdot x^{i} = a_m \cdot x^{m} + a_{m-1} \cdot x^{m-1} + \hspace{0.1cm}... \hspace{0.1cm}+ a_2 \cdot x^2 + a_1 \cdot x + a_0 \hspace{0.05cm}\]
einen Rest r(x) ≠ 0 liefert. Dies würde im vorliegenden Beispiel (nahezu) 25 = 32 Divisionen erfordern.