Difference between revisions of "Aufgaben:Exercise 4.6: k-parameters and alpha-parameters"
| Line 62: | Line 62: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {Berechnen Sie die Parameter der Gleichung $ \alpha_1 + C_1 \cdot \alpha_2 + C_2 = 0$, die sich aus der Ableitung ${\rm dE[...]/d}\alpha_1$ ergeben. Welche Ergebnisse sind zutreffend? | + | {Berechnen Sie die Parameter der Gleichung $\alpha_1 + C_1 \cdot \alpha_2 + C_2 = 0$, die sich aus der Ableitung ${\rm dE[...]/d}\alpha_1$ ergeben. Welche Ergebnisse sind zutreffend? |
|type="[]"} | |type="[]"} | ||
+ $C_1 = 6/5 \cdot B^{-0.5}$, | + $C_1 = 6/5 \cdot B^{-0.5}$, | ||
| Line 104: | Line 104: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | + | '''(1)''' Die Ableitung des angegebenen Erwartungswertes nach $\alpha_1$ ergibt: | |
| − | + | $$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_1}} = | |
\frac{2}{3}\cdot B^3 \cdot \alpha_1 + \frac{4}{5}\cdot B^{2.5} \cdot \alpha_2 | \frac{2}{3}\cdot B^3 \cdot \alpha_1 + \frac{4}{5}\cdot B^{2.5} \cdot \alpha_2 | ||
- \frac{2 k_2 }{k_3 | - \frac{2 k_2 }{k_3 | ||
+ 2} \cdot \frac{B^{k_3+2}}{f_0^{k_3}}= 0 | + 2} \cdot \frac{B^{k_3+2}}{f_0^{k_3}}= 0 | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | Durch Nullsetzen und Division durch $2B^2/3$ erhält man daraus: | |
| − | + | $$\alpha_1 + \frac{6}{5}\cdot B^{-0.5} \cdot \alpha_2 | |
- \frac{3 k_2 }{k_3 | - \frac{3 k_2 }{k_3 | ||
+2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | +2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | $$\Rightarrow \hspace{0.3cm} C_1 = \frac{6}{5}\cdot B^{-0.5} \hspace{0.05cm} , | |
\hspace{0.5cm} C_2 = | \hspace{0.5cm} C_2 = | ||
- \frac{3 k_2 }{k_3 | - \frac{3 k_2 }{k_3 | ||
+2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} | +2} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | Richtig sind demnach <u>die Lösungsvorschläge 1 und 6</u>. | |
| − | + | ||
| − | + | '''(2)''' Bei gleicher Vorgehensweise wie in der Teilaufgabe (1) zeigt sich, dass nun <u>die Lösungsvorschläge 2 und 5</u> richtig sind: | |
| + | $$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_2}} = | ||
\frac{4}{5}\cdot B^{2.5} \cdot \alpha_1 + B^{2} \cdot \alpha_2 | \frac{4}{5}\cdot B^{2.5} \cdot \alpha_1 + B^{2} \cdot \alpha_2 | ||
- \frac{2 k_2 }{k_3 | - \frac{2 k_2 }{k_3 | ||
+ 1.5} \cdot \frac{B^{k_3+1.5}}{f_0^{k_3}}= 0$$ | + 1.5} \cdot \frac{B^{k_3+1.5}}{f_0^{k_3}}= 0$$ | ||
| − | + | $$\Rightarrow \hspace{0.3cm} \alpha_1 + \frac{5}{4}\cdot B^{-0.5} \cdot \alpha_2 | |
- \frac{2.5 \cdot k_2 }{k_3 | - \frac{2.5 \cdot k_2 }{k_3 | ||
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | +1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0 | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | $$\Rightarrow \hspace{0.3cm}D_1 = \frac{5}{4}\cdot B^{-0.5} \hspace{0.05cm} , | |
\hspace{0.3cm}D_2 = | \hspace{0.3cm}D_2 = | ||
- \frac{2.5 \cdot k_2 }{k_3 | - \frac{2.5 \cdot k_2 }{k_3 | ||
| Line 137: | Line 138: | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | ||
| − | + | '''(3)''' Aus $C_1 \cdot \alpha_2 + C_2 = D_1 \cdot \alpha_2 + D_2$ ergibt sich eine lineare Gleichung für $\alpha_2$. Mit dem Ergebnis aus (2) kann hierfür geschrieben werden: | |
| + | $$\alpha_2 = \frac{D_2 - C_2}{C_1 - D_1} = \frac{- \frac{2.5 \cdot k_2 }{k_3 | ||
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} + \frac{3 k_2 }{k_3 +2} | +1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} + \frac{3 k_2 }{k_3 +2} | ||
\cdot \frac{B^{k_3-1}}{f_0^{k_3}}}{{6}/{5}\cdot B^{-0.5} - | \cdot \frac{B^{k_3-1}}{f_0^{k_3}}}{{6}/{5}\cdot B^{-0.5} - | ||
| − | {5}/{4}\cdot B^{-0.5}} | + | {5}/{4}\cdot B^{-0.5}} = \frac{- {2.5 \cdot k_2 |
}\cdot(k_3 +2) + {3 k_2 }\cdot (k_3 +1.5) }{({6}/{5} - | }\cdot(k_3 +2) + {3 k_2 }\cdot (k_3 +1.5) }{({6}/{5} - | ||
{5}/{4})(k_3 +1.5)(k_3 +2)} \cdot | {5}/{4})(k_3 +1.5)(k_3 +2)} \cdot | ||
| − | \frac{B^{k_3-0.5}}{f_0^{k_3}} | + | \frac{B^{k_3-0.5}}{f_0^{k_3}}$$ |
| + | $$ \Rightarrow \hspace{0.3cm}\alpha_2 = 10 \cdot (B/f_0)^{k_3 | ||
-0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}} | 2)}\cdot \frac {k_2}{\sqrt{f_0}} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | + | Für den Parameter $\alpha_1$ gilt dann: | |
| − | + | $$\alpha_1 = - C_1 \cdot \alpha_2 - C_2 = \\ = | |
-\frac{6}{5}\cdot B^{-0.5} \cdot 10 \cdot (B/f_0)^{k_3 | -\frac{6}{5}\cdot B^{-0.5} \cdot 10 \cdot (B/f_0)^{k_3 | ||
-0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}} +\frac{3 k_2 }{k_3 +2} | 2)}\cdot \frac {k_2}{\sqrt{f_0}} +\frac{3 k_2 }{k_3 +2} | ||
| − | \cdot \frac{B^{k_3-1}}{f_0^{k_3}} | + | \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= (B/f_0)^{k_3 -1}\cdot |
\frac{-12 \cdot (1-k_3) + 3 \cdot (k_3 + 1.5)}{(k_3 + 1.5)(k_3 + | \frac{-12 \cdot (1-k_3) + 3 \cdot (k_3 + 1.5)}{(k_3 + 1.5)(k_3 + | ||
| − | 2)} \cdot \frac {k_2}{f_0} | + | 2)} \cdot \frac {k_2}{f_0}$$ |
| + | $$ \Rightarrow \hspace{0.3cm}\alpha_1 = 15 \cdot (B/f_0)^{k_3 | ||
-1}\cdot \frac{k_3 -0.5}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{k_3 -0.5}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0}\hspace{0.05cm} .$$ | 2)}\cdot \frac {k_2}{f_0}\hspace{0.05cm} .$$ | ||
| − | + | Die <u>beiden Lösungsvorschläge</u> sind richtig. Unabhängig von der Bandbreite erhält man für $k_3 = 1$: | |
| − | + | $$\alpha_1 = (B/f_0)^{k_3 | |
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0} = \frac{15 \cdot 0.5}{2.5 \cdot 3}\cdot \frac {k_2}{f_0} | 2)}\cdot \frac {k_2}{f_0} = \frac{15 \cdot 0.5}{2.5 \cdot 3}\cdot \frac {k_2}{f_0} | ||
\hspace{0.15cm}\underline{ = {k_2}/{f_0}}\hspace{0.05cm} | \hspace{0.15cm}\underline{ = {k_2}/{f_0}}\hspace{0.05cm} | ||
| − | , | + | ,$$ |
| − | + | $$ \alpha_2 = (B/f_0)^{k_3 | |
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}}\hspace{0.15cm}\underline{= 0} \hspace{0.05cm} | 2)}\cdot \frac {k_2}{\sqrt{f_0}}\hspace{0.15cm}\underline{= 0} \hspace{0.05cm} | ||
.$$ | .$$ | ||
| − | + | Dagegen ergibt sich für $k_3 = 0.5$: | |
| − | + | $$\alpha_1 = (B/f_0)^{k_3 | |
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{f_0} \hspace{0.15cm}\underline{= 0}\hspace{0.05cm} | 2)}\cdot \frac {k_2}{f_0} \hspace{0.15cm}\underline{= 0}\hspace{0.05cm} | ||
| − | , | + | ,$$ |
| − | + | $$ \alpha_2 = (B/f_0)^{k_3 | |
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \hspace{0.15cm}\underline{ {k_2}/{\sqrt{f_0}}} \hspace{0.05cm} | 2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \hspace{0.15cm}\underline{ {k_2}/{\sqrt{f_0}}} \hspace{0.05cm} | ||
.$$ | .$$ | ||
| − | + | ||
| − | + | '''(4)''' Für die beiden Koeffizienten gilt mit $k_2 = 10.8 \ \rm dB/km$, $k_3 = 0.6 \ \rm dB/km$ und $B/f_0 = 30$: | |
| + | $$\alpha_1 = (B/f_0)^{k_3 | ||
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | -1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 + | ||
| − | 2)}\cdot \frac {k_2}{f_0} | + | 2)}\cdot \frac {k_2}{f_0} = 30^{-0.4}\cdot \frac{15 \cdot 0.1}{2.1 \cdot 2.6}\cdot |
| − | = 30^{-0.4}\cdot \frac{15 \cdot 0.1}{2.1 \cdot 2.6}\cdot | ||
\frac {10.8 \, {\rm dB/km} }{1 \, {\rm MHz}} | \frac {10.8 \, {\rm dB/km} }{1 \, {\rm MHz}} | ||
\hspace{0.15cm}\underline{ \approx 0.761\, | \hspace{0.15cm}\underline{ \approx 0.761\, | ||
{{\rm dB} }/{({\rm km \cdot MHz})}} | {{\rm dB} }/{({\rm km \cdot MHz})}} | ||
\hspace{0.05cm} | \hspace{0.05cm} | ||
| − | , | + | ,$$ |
| − | + | $$ \alpha_2 = (B/f_0)^{k_3 | |
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | -0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 + | ||
2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \frac {k_2}{\sqrt{f_0}} | 2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \frac {k_2}{\sqrt{f_0}} | ||
| − | |||
= 30^{0.1}\cdot \frac{10 \cdot 0.4}{2.1 \cdot 2.6}\cdot \frac | = 30^{0.1}\cdot \frac{10 \cdot 0.4}{2.1 \cdot 2.6}\cdot \frac | ||
{10.8 \, {\rm dB/km} }{1 \, {\rm MHz^{0.5}}} | {10.8 \, {\rm dB/km} }{1 \, {\rm MHz^{0.5}}} | ||
| Line 198: | Line 201: | ||
.$$ | .$$ | ||
| − | + | ||
| − | + | '''(5)''' Entsprechend der angegebenen Gleichung $\alpha_{\rm II}(f$) gilt: | |
| + | $$\alpha_{\rm II}(f = 30 \, {\rm MHz}) = \alpha_0 + \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f} | ||
= \left [ \hspace{0.05cm} 4.4 + 0.761 \cdot 30 + 11.1 \cdot \sqrt {30}\hspace{0.05cm} | = \left [ \hspace{0.05cm} 4.4 + 0.761 \cdot 30 + 11.1 \cdot \sqrt {30}\hspace{0.05cm} | ||
\right ]\frac | \right ]\frac | ||
Revision as of 18:05, 16 February 2017
Für symmetrische Kupfer–Doppeladern findet man in [PW95] die folgende empirische Formel, gültig für den Frequenzbereich $0 \le f \le 30 \ \rm MHz$: $$\alpha_{\rm I} (f) = k_1 + k_2 \cdot (f/f_0)^{k_3} , \hspace{0.15cm} f_0 = 1\,{\rm MHz} .$$ Dagegen ist das Dämpfungsmaß eines Koaxialkabels meist in der folgenden Form angegeben: $$\alpha_{\rm II}(f) = \alpha_0 + \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f}\hspace{0.05cm}.$$ Insbesondere zur Berechnung von Impulsantwort und Rechteckantwort ist es von Vorteil, auch für die Kupfer–Doppeladern die zweite Darstellungsform mit den Kabelparametern $\alpha_0$, $\alpha_1$ und $\alpha_2$ anstelle der Beschreibung durch $k_1$, $k_2$ und $k_3$ zu wählen. Für die Umrechnung geht man dabei wie folgt vor:
- Aus obigen Gleichungen ist offensichtlich, dass der die Gleichsignaldämpfung charakterisierende Koeffizient $k_1 =\alpha_0$ ist.
- Zur Bestimmung von $\alpha_1$ und $\alpha_2$ wird davon ausgegangen, dass der mittlere quadratische Fehler im Bereich einer vorgegebenen Bandbreite $B$ minimal sein soll:
- $${\rm E}[\varepsilon^2(f)] = \int_{0}^{ B} \left [ \alpha_{\rm II} (f) - \alpha_{\rm I} (f)\right ]^2 \hspace{0.1cm}{\rm d}f \hspace{0.3cm}\Rightarrow \hspace{0.3cm}{\rm Minimum} \hspace{0.05cm} .$$
- Die Differenz $\varepsilon^2(f)$ und der mittlere quadratische Fehler ${\rm E}[\varepsilon^2(f)]$ ergeben sich dabei wie folgt:
- $$\varepsilon^2(f) = \left [ \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f} - k_2 \cdot (f/f_0)^{k_3}\right ]^2 =\alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} f^2 + 2 \alpha_1 \alpha_2 \hspace{0.05cm}\cdot\hspace{0.05cm} f^{1.5} + \alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} f + k_2^2\hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{2k_3}}{f_0^{2k_3}} - 2 k_2 \alpha_1 \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{k_3+1}} {f_0^{k_3}}-{2 k_2 \alpha_2} \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{f^{k_3+0.5}}{f_0^{k_3}}$$
- $$\Rightarrow \hspace{0.3cm}{\rm E}[\varepsilon^2(f)] = \alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm}\frac{B^3}{3} + \frac{4}{5} \hspace{0.05cm}\cdot\hspace{0.05cm}\alpha_1 \alpha_2 \hspace{0.05cm}\cdot\hspace{0.05cm}B^{2.5} + \alpha_1^2 \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{B^2}{2} + \frac{k_2^2}{2k_3 +1} \hspace{0.05cm}\cdot\hspace{0.05cm} \frac{B^{2k_3+1}}{f_0^{2k_3}} - \hspace{0.15cm} \frac{2 k_2 \alpha_1}{k_3 + 2} \hspace{0.05cm}\cdot\hspace{0.05cm} $$
- Diese Gleichung beinhaltet die zu verrechnenden Kabelparameter $\alpha_1$, $\alpha_2$, $k_2$ und $k_3$ sowie die Bandbreite $B$, innerhalb derer die Approximation gültig sein soll.
- Durch Nullsetzen der Ableitungen von ${\rm E}[\varepsilon^2(f)]$ nach $\alpha_1$ bzw. $\alpha_2$ erhält man zwei Gleichungen für die bestmöglichen Koeffizienten $\alpha_1$ und $\alpha_2$, die den mittleren quadratischen Fehler minimieren. Diese lassen sich in folgender Form darstellen:
- $$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_1}} = 0 \hspace{0.2cm} \Rightarrow \hspace{0.2cm} \alpha_1 + C_1 \cdot \alpha_2 + C_2 = 0 \hspace{0.05cm} ,$$
- $$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_2}} = 0 \hspace{0.2cm} \Rightarrow \hspace{0.2cm} \alpha_1 + D_1 \cdot \alpha_2 + D_2 = 0 \hspace{0.05cm} . $$
- Aus der Gleichung $C_1 \cdot \alpha_2 + C_2 = D_1 \cdot \alpha_2 + D_2$ lässt sich daraus der Koeffizient $\alpha_2$ berechnen und anschließend aus jeder der beiden oberen Gleichungen der Koeffizient $\alpha_1$.
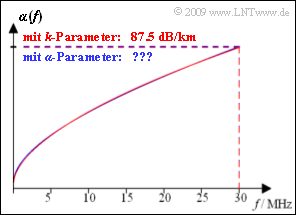
Die Grafik zeigt das Dämpfungsmaß für eine Kupferdoppelader mit 0.5 mm Durchmesser, deren $k$–Parameter lauten:
$$k_1 = 4.4\, {\rm dB}/{\rm km} \hspace{0.05cm}, \hspace{0.2cm}
k_2 = 10.8\, {\rm dB}/{\rm km}\hspace{0.05cm}, \hspace{0.2cm}k_3 = 0.60\hspace{0.05cm}
\hspace{0.05cm}.$$
- Die rote Kurve zeigt die damit berechnete Funktion $\alpha(f)$. Für $f = 30 \ \rm MHz$ ergibt sich das Dämpfungsmaß $\alpha(f) 87.5 \ \rm dB/km$.
- Die blaue Kurve gibt die Approximation mit den $\alpha$ndash;Koeffizienten an. Diese ist von der roten Kurve innerhalb der Zeichengenauigkeit fast nicht zu unterscheiden.
Hinweise:
- Die Aufgabe gehört zum Kapitel Eigenschaften von Kupfer–Doppeladern.
- Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.
- [PW95] kennzeichnet folgenden Literaturhinweis:
- Pollakowski, P.; Wellhausen, H.-W.: Eigenschaften symmetrischer Ortsanschlusskabel im Frequenzbereich bis 30 MHz. Deutsche Telekom AG, Forschungs- und Technologiezentrum Darmstadt, 1995.
Fragebogen
Musterlösung
(2) Bei gleicher Vorgehensweise wie in der Teilaufgabe (1) zeigt sich, dass nun die Lösungsvorschläge 2 und 5 richtig sind:
$$\frac{{\rm d}\,{\rm E}[\varepsilon^2(f)]}{{\rm d}\,{\alpha_2}} =
\frac{4}{5}\cdot B^{2.5} \cdot \alpha_1 + B^{2} \cdot \alpha_2
- \frac{2 k_2 }{k_3
+ 1.5} \cdot \frac{B^{k_3+1.5}}{f_0^{k_3}}= 0$$
$$\Rightarrow \hspace{0.3cm} \alpha_1 + \frac{5}{4}\cdot B^{-0.5} \cdot \alpha_2
- \frac{2.5 \cdot k_2 }{k_3
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}= 0
\hspace{0.05cm} .$$
$$\Rightarrow \hspace{0.3cm}D_1 = \frac{5}{4}\cdot B^{-0.5} \hspace{0.05cm} ,
\hspace{0.3cm}D_2 =
- \frac{2.5 \cdot k_2 }{k_3
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}}
\hspace{0.05cm} .$$
(3) Aus $C_1 \cdot \alpha_2 + C_2 = D_1 \cdot \alpha_2 + D_2$ ergibt sich eine lineare Gleichung für $\alpha_2$. Mit dem Ergebnis aus (2) kann hierfür geschrieben werden:
$$\alpha_2 = \frac{D_2 - C_2}{C_1 - D_1} = \frac{- \frac{2.5 \cdot k_2 }{k_3
+1.5} \cdot \frac{B^{k_3-1}}{f_0^{k_3}} + \frac{3 k_2 }{k_3 +2}
\cdot \frac{B^{k_3-1}}{f_0^{k_3}}}{{6}/{5}\cdot B^{-0.5} -
{5}/{4}\cdot B^{-0.5}} = \frac{- {2.5 \cdot k_2
}\cdot(k_3 +2) + {3 k_2 }\cdot (k_3 +1.5) }{({6}/{5} -
{5}/{4})(k_3 +1.5)(k_3 +2)} \cdot
\frac{B^{k_3-0.5}}{f_0^{k_3}}$$
$$ \Rightarrow \hspace{0.3cm}\alpha_2 = 10 \cdot (B/f_0)^{k_3
-0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{\sqrt{f_0}}
\hspace{0.05cm} .$$
Für den Parameter $\alpha_1$ gilt dann:
$$\alpha_1 = - C_1 \cdot \alpha_2 - C_2 = \\ =
-\frac{6}{5}\cdot B^{-0.5} \cdot 10 \cdot (B/f_0)^{k_3
-0.5}\cdot \frac{1-k_3}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{\sqrt{f_0}} +\frac{3 k_2 }{k_3 +2}
\cdot \frac{B^{k_3-1}}{f_0^{k_3}}= (B/f_0)^{k_3 -1}\cdot
\frac{-12 \cdot (1-k_3) + 3 \cdot (k_3 + 1.5)}{(k_3 + 1.5)(k_3 +
2)} \cdot \frac {k_2}{f_0}$$
$$ \Rightarrow \hspace{0.3cm}\alpha_1 = 15 \cdot (B/f_0)^{k_3
-1}\cdot \frac{k_3 -0.5}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{f_0}\hspace{0.05cm} .$$
Die beiden Lösungsvorschläge sind richtig. Unabhängig von der Bandbreite erhält man für $k_3 = 1$:
$$\alpha_1 = (B/f_0)^{k_3
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{f_0} = \frac{15 \cdot 0.5}{2.5 \cdot 3}\cdot \frac {k_2}{f_0}
\hspace{0.15cm}\underline{ = {k_2}/{f_0}}\hspace{0.05cm}
,$$
$$ \alpha_2 = (B/f_0)^{k_3
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{\sqrt{f_0}}\hspace{0.15cm}\underline{= 0} \hspace{0.05cm}
.$$
Dagegen ergibt sich für $k_3 = 0.5$:
$$\alpha_1 = (B/f_0)^{k_3
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{f_0} \hspace{0.15cm}\underline{= 0}\hspace{0.05cm}
,$$
$$ \alpha_2 = (B/f_0)^{k_3
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \hspace{0.15cm}\underline{ {k_2}/{\sqrt{f_0}}} \hspace{0.05cm}
.$$
(4) Für die beiden Koeffizienten gilt mit $k_2 = 10.8 \ \rm dB/km$, $k_3 = 0.6 \ \rm dB/km$ und $B/f_0 = 30$:
$$\alpha_1 = (B/f_0)^{k_3
-1}\cdot \frac{15 \cdot (k_3 -0.5)}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{f_0} = 30^{-0.4}\cdot \frac{15 \cdot 0.1}{2.1 \cdot 2.6}\cdot
\frac {10.8 \, {\rm dB/km} }{1 \, {\rm MHz}}
\hspace{0.15cm}\underline{ \approx 0.761\,
{{\rm dB} }/{({\rm km \cdot MHz})}}
\hspace{0.05cm}
,$$
$$ \alpha_2 = (B/f_0)^{k_3
-0.5}\cdot \frac{10 \cdot (1-k_3)}{(k_3 + 1.5)(k_3 +
2)}\cdot \frac {k_2}{\sqrt{f_0}}= \frac{10 \cdot 0.5}{2 \cdot 2.5}\cdot \frac {k_2}{\sqrt{f_0}} = \frac {k_2}{\sqrt{f_0}}
= 30^{0.1}\cdot \frac{10 \cdot 0.4}{2.1 \cdot 2.6}\cdot \frac
{10.8 \, {\rm dB/km} }{1 \, {\rm MHz^{0.5}}}
\hspace{0.15cm}\underline{ \approx 11.1\,
{{\rm dB} }/{({\rm km \cdot MHz^{0.5}}})}\hspace{0.05cm}
.$$
(5) Entsprechend der angegebenen Gleichung $\alpha_{\rm II}(f$) gilt:
$$\alpha_{\rm II}(f = 30 \, {\rm MHz}) = \alpha_0 + \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f}
= \left [ \hspace{0.05cm} 4.4 + 0.761 \cdot 30 + 11.1 \cdot \sqrt {30}\hspace{0.05cm}
\right ]\frac
{\rm dB}{\rm km }
\hspace{0.15cm}\underline{\approx 88.1\, {\rm dB}/{\rm km }}
\hspace{0.05cm}.$$