Aufgaben:Exercise 4.09Z: Laplace Distributed Noise

• Return to book

2D–Laplace–WDF

Wir betrachten zweidimensionales Rauschen $\boldsymbol{n} = (n_1, n_2)$.

Die beiden Rauschvariablen sind „independent and identically distributed”, abgekürzt i.i.d., und besitzen beide jeweils eine Laplace–Wahrscheinlichkeitsdichte:

$$p_{n_1}(x) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} K \cdot {\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm} |x|} \hspace{0.05cm},$$

$$ p_{n_2}(y) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} K \cdot {\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm} |y|} \hspace{0.05cm}. $$

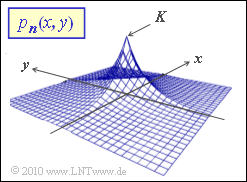
Die 2D–Wahrscheinlichkeitsdichtefunktion $p_{\it \boldsymbol{n}}(x, y)$ ist in der Grafik dargestellt. Zur Vereinfachung der Schreibweise werden hier die Realisierungen von $n_1$ und $n_2$ mit $x$ und $y$ bezeichnet.

Hinweise:

• Die Aufgabe bezieht sich auf das Kapitel Approximation der Fehlerwahrscheinlichkeit.
• Beachten Sie bitte, dass in Teilaufgabe (6) das sich ergebende Integral aufgrund der Betragsbildung in mehrere Teilintegrale aufgespalten werden muss.
• Weiterhin gilt:

$$\int_{0}^{\infty} x^2 \cdot {\rm e}^{-a \hspace{0.03cm}\cdot \hspace{0.03cm} x} \,{\rm d} x = {2}/{a^3} \hspace{0.05cm}.$$

Fragebogen

1

Wie groß ist die Konstante $K$ der 1D–WDF?

	$K = 1$.
	$K = a/2$
	$K = 1/a$.

2

Es sei $a = 1$. Wie groß sind der Mittelwert ${\rm E}[n_i]$ und die Varianz $\sigma^2 = {\rm E}[n_i^2]$ der beiden 1D–Zufallsgrößen? ($i = 1, 2$)

${\rm E}[n_i]$ =

$\sigma^2 = {\rm E}[n_i^2]$ =

3

Welche Form haben die Höhenlinien der 2D–WDF im ersten Quadranten?

	Es sind Geraden.
	Es sind Hyperbeln.
	Es sind Kreise.

4

Wie groß ist die Wahrscheinlichkeit, dass sowohl $n_1$ als auch $n_2$ negativ sind?

$a = 1 \text{:} \hspace{0.2cm} {\rm Pr}[(n_1 < 0) ∩ (n_2 < 0)]$ =

5

Wie groß ist die Wahrscheinlichkeit, dass $n_1$ und $n_2$ jeweils größer als $1$ sind?

$a = 1 \text{:} \hspace{0.2cm} {\rm Pr}[(n_1 > 1) ∩ (n_2 > 1)]$ =

6

Wie groß ist die Wahrscheinlichkeit, dass die Summe $n_1 + n_2 > 2$ ist?

$\alpha = 1 \text{:} \hspace{0.2cm} {\rm Pr}[n_1 + n_2 > 2)]$ =

Musterlösung

Solution

(1)  Die Fläche unter der WDF muss $1$ ergeben:

$$\int_{-\infty}^{+\infty} p_{n_1}(x) \,{\rm d} x = 1 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \int_{0}^{+\infty} p_{n_1}(x) \,{\rm d} x = 0.5 $$

$$\Rightarrow \hspace{0.3cm} K \cdot \int_{0}^{\infty} {\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm}x} \,{\rm d} x = - {K}/{a} \cdot \left [ {\rm e}^{- a \hspace{0.03cm} \cdot \hspace{0.03cm} x} \right ]_{0}^{\infty}= {K}/{a} = 0.5 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} K = {a}/{2}\hspace{0.05cm}.$$

Richtig ist demzufolge der Lösungsvorschlag 2.

(2)  Der lineare Mittelwert ist aufgrund der WDF–Symmetrie gleich 0. Damit ist die Varianz $\sigma^2$ tatsächlich – wie bereits in der Fragestellung angegeben – gleich dem quadratischen Mittelwert:

$$\sigma^2 = {\rm E}[n_1^2] = 2 \cdot \frac{a}{2} \cdot \int_{0}^{\infty} x^2 \cdot {\rm e}^{-a \hspace{0.03cm} \cdot \hspace{0.03cm} x} \,{\rm d} x = a \cdot {2}/{a^3}= {2}/{a^2} \hspace{0.05cm}. \hspace{0.2cm}{\rm Mit}\hspace{0.15cm}a = 1: \hspace{0.2cm}\hspace{0.1cm}\underline {\sigma^2 = 2 }\hspace{0.05cm}.$$

(3)  Im ersten Quadranten ($x ≥ 0, y ≥ 0$) kann auf die Betragsbildung verzichtet werden. Dann gilt für die 2D–WDF:

$$\boldsymbol{ p }_{\boldsymbol{ n }} (x,\hspace{0.15cm} y) = {a^2}/{4} \cdot {\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm}x} \cdot {\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm}y }= {a^2}/{4} \cdot {\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm}(x+y)}\hspace{0.05cm}.$$

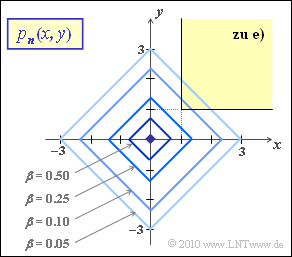
Eine Höhenlinie mit dem Faktor $\beta$ gegenüber dem Maximum hat dann den folgenden Verlauf ($0 < \beta < 1$):

Höhenlinien der 2D-Laplaceverteilung

$${\rm e}^{- a \hspace{0.03cm}\cdot \hspace{0.03cm}(x+y)} = \beta \hspace{0.3cm} \Rightarrow \hspace{0.3cm} x + y = \frac{{\rm ln}\hspace{0.15cm}1/\beta}{a} \hspace{0.05cm}.$$

Die Grafik zeigt die Höhenlinien für $a = 1$ und einige Werte von $\beta$, die jeweils ein um $45°$ gedrehtes Quadrat ergeben  ⇒  Vorschlag 1. Gleichzeitig weisen wir Sie auf das folgende Interaktionsmodul hin: Zweidimensionale Laplaceverteilung

(4)  Das hier betrachtete Wahrscheinlichkeitsereignis entspricht genau dem dritten Quadranten der oben skizzierten Verbund–WDF. Aufgrund der Symmetrie ist diese Wahrscheinlichkeit gleich 1/4.

(5)  Dafür kann mit der Verbund–WDF geschrieben werden:

$${\rm Pr} \left [ (n_1 > 1)\cap (n_2 > 1)\right ] = {1}/{4} \cdot \int_{1}^{\infty} \int_{1}^{\infty}{\rm e}^{- (x+y)} \,{\rm d} x \,{\rm d} y =$$

$$. \hspace{0.5cm} = {1}/{2} \cdot \int_{1}^{\infty} {\rm e}^{- x} \,{\rm d} x \hspace{0.15cm} \cdot \hspace{0.15cm} {1}/{2} \cdot \int_{1}^{\infty} {\rm e}^{- y} \,{\rm d} y = \left [ {\rm Pr} (n_1 > 1)\right ] \cdot \left [ {\rm Pr} (n_2 > 1)\right ]\hspace{0.05cm}. $$

Hierbei ist die statistische Unabhängigkeit zwischen $n_1$ und $n_2$ sowie die Gleichheit $p_{\it n1}(x) = p_{\it n2}(y)$ berücksichtigt. Weiter gilt für $a = 1$:

$${\rm Pr} (n_1 > 1) = {1}/{2} \cdot \int_{1}^{\infty} {\rm e}^{- x} \,{\rm d} x = {1}/({2{\rm e}})\approx 0.184$$

$$\Rightarrow \hspace{0.3cm} {\rm Pr} \left [ (n_1 > 1)\cap (n_2 > 1)\right ] = {1}/({4{\rm e}^2)}\hspace{0.1cm}\hspace{0.15cm}\underline {\approx 0.034}\hspace{0.05cm}.$$

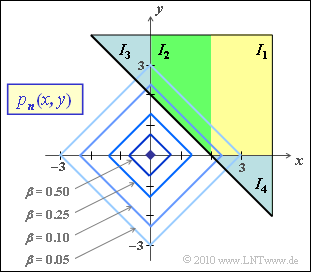
(6)  Die hier betrachtete Region ist in der folgenden Grafik farbig markiert. Die Regionen erstrecken sich aber nach rechts und oben bis ins Unendliche. Die gesuchte Wahrscheinlichkeit ergibt sich zu

Aufteilung des Integrationsbereichs

$${\rm Pr} [ n_1 \hspace{-0.2cm} \ + \ \hspace{-0.2cm} n_2 > 2 ] =$$

$$ \hspace{2cm} \ = \ \hspace{-0.2cm} \frac{1}{4} \cdot \int\limits_{-\infty}^{+\infty} {\rm e}^{-|x|} \int\limits_{2-x}^{\infty}{\rm e}^{-|y|} \,{\rm d} y \,{\rm d} x = $$

$$\hspace{2cm} \ = \ \hspace{-0.2cm} I_1 + I_2 + I_3 + I_4 \hspace{0.05cm}.$$

Aufgrund der Betragsbildung ist eine Aufspaltung in Teilintegrale vorzunehmen. Nach oben und rechts erstrecken sich alle Gebiete bis ins Unendliche. Aufgrund der Symmetrie gilt $I_4 = I_3$.

$$I_1 = {1}/{4} \cdot \int_{2}^{+\infty} {\rm e}^{-x} \int_{0}^{\infty}{\rm e}^{-y} \,{\rm d} y \,{\rm d} x = {1}/{4} \cdot \int_{2}^{+\infty} {\rm e}^{-x} \,{\rm d} x ={1}/({4{\rm e}^2})\hspace{0.05cm},$$

$$I_2 = {1}/{4} \cdot \hspace{-0.1cm} \int_{0}^{2} \hspace{-0.15cm}{\rm e}^{-x} \int_{2-x}^{\infty}\hspace{-0.15cm}{\rm e}^{-y} \,{\rm d} y \,{\rm d} x = {1}/{4} \cdot \hspace{-0.1cm} \int_{0}^{2} {\rm e}^{-x}\hspace{-0.1cm} \cdot {\rm e}^{x-2} \,{\rm d} x ={1}/{4} \cdot \hspace{-0.1cm}\int_{0}^{2} {\rm e}^{-2} \,{\rm d} x = {1}/({2{\rm e}^2})\hspace{0.05cm},$$

$$I_3 \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot \int_{-\infty}^{0} {\rm e}^{x} \int_{2-x}^{\infty}{\rm e}^{-y} \,{\rm d} y \,{\rm d} x = {1}/{4} \cdot \int_{-\infty}^{0} {\rm e}^{x} \cdot {\rm e}^{x-2} \,{\rm d} x =$$

$$ \hspace{-0.1cm} \ = \ \hspace{-0.1cm} {1}/{4} \cdot \int_{-\infty}^{0} {\rm e}^{2x-2} \,{\rm d} x = \frac{{\rm e}^{-2}}{4} \cdot \int_{0}^{\infty} {\rm e}^{-2x} \,{\rm d} x = {1}/({8{\rm e}^2})\hspace{0.05cm},$$

$$I_4 ={1}/{4} \cdot \int_{-\infty}^{0} {\rm e}^{y} \int_{2-y}^{\infty}{\rm e}^{-x} \,{\rm d} x \,{\rm d} y = ... = {1}/({8{\rm e}^2}) = I_3\hspace{0.05cm}.$$

Insgesamt ergibt sich somit:

$${\rm Pr} \left [ n_1 + n_2 > 2 \right ] = {\rm e}^{-2} \cdot ({1}/{4} +{1}/{2} +{1}/{8} +{1}/{8})= {\rm e}^{-2} \hspace{0.1cm}\hspace{0.15cm}\underline {\approx 0.135}\hspace{0.05cm}.$$