Difference between revisions of "Aufgaben:Exercise 4.08: Repetition to the Convolutional Codes"
| Line 64: | Line 64: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
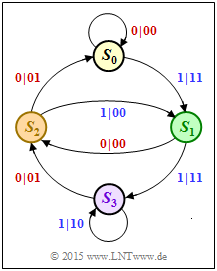
| − | '''(1)''' | + | '''(1)''' Die Impulsantwort $\underline{g}$ ist gleich der Ausgangsfolge $\underline{p}$ für die Eingangsfolge $\underline{u} = (1, \, 0, \, 0, \, 0, \, ...)$. Ausgehend vom Zustand $S_0$ ergeben sich im Zustandsübergangsdiagramm folgende Übergänge: |
| + | :$$S_0 → S_1 → S_2 → S_0 → S_0 → S_0 → \hspace{0.5cm} \Rightarrow \hspace{0.5cm}$ Impulsantwort: $\ \ \underline{g} = (1, \, 0, \, 1, \, 0, \, 0)$. | ||
| + | Richtig ist der <u>Lösungsvorschlag 2</u>. Für ein nichtrekursives Filter mit Gedächtnis $m$ gilt $g_i ≡ 0$ für $i > m$. In unserem Beispiel ist $m = 2$. Der Lösungsvorschlag 1 gilt dagegen für das rekursive Filter (RSC) entsprechend der [[Aufgaben:4.09_Wiederholung_zu_den_RSC-Codes| Aufgabe A4.9]]. | ||
| − | '''(2)''' | + | |
| + | '''(2)''' Es sei $\underline{u} = (1, \, 0, \, 0, \, 1, \, 0, \, 0, \, u_7)$ und $\underline{g} = (1, \, 0, \, 1, \, 0, \, 0, \, 0, \, ...)$. Dann gilt für die Paritysequenz aufgrund der Linearität: | ||
| + | :$$\underline{p} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} | ||
| + | (\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} 0, \hspace{0.05cm} 0,\hspace{0.05cm} u_7\hspace{0.05cm} ) | ||
| + | * (\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} 0 | ||
| + | ,\hspace{0.05cm} 0,\hspace{0.05cm} 0\hspace{0.05cm},\hspace{0.05cm} ...)= $$ | ||
| + | :$$\ = \ \hspace{-0.15cm} (\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm}0, \hspace{0.05cm} ... \hspace{0.05cm})\oplus$$ | ||
| + | :$$\ \oplus \ \hspace{-0.15cm} (\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm}0, \hspace{0.05cm} ... \hspace{0.05cm})\oplus$$ | ||
| + | :$$\ \oplus \ \hspace{-0.15cm} (\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} u_7,\hspace{0.05cm} 0,\hspace{0.05cm}u_7, \hspace{0.05cm} ... \hspace{0.05cm})= $$ | ||
| + | :$$\ = \ \hspace{-0.15cm} (\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} u_7,\hspace{0.05cm} 0,\hspace{0.05cm}u_7, \hspace{0.05cm} ... \hspace{0.05cm}) | ||
| + | \hspace{0.05cm}.$$ | ||
Revision as of 20:03, 10 December 2017
Die Turbocodes basieren auf den Faltungscodes, die im Kapitel Grundlagen der Faltungscodierung auführlich behandelt werden.
Ausgehend von dem nebenstehenden Zustandsübergangsdiagramm sollen wesentliche Eigenschaften und Kenngrößen des betrachteten Rate–1/2–Faltungscodes ermittelt werden, wobei wir ausdrücklich auf folgende Theorieseiten verweisen:
Systematische Faltungscodes (1)
Darstellung im Zustandsübergangsdiagramm (1)
Definition der freien Distanz (1)
GF(2)–Beschreibungsformen eines Digitalen Filters (2)
Anwendung der $D$–Transformation auf Rate–1/n–Faltungscodes (2)
Im Zustandsübergangsdiagramm wird grundsätzlich vom Zustand $S_0$ ausgegangen. Von jedem Zustand gehen zwei Pfeile ab. Die Beschriftung lautet „$u_i | x_i^{(1)}x_i^{(2)}$”. Bei einem systematischen Code gilt dabei:
- Das erste Codebit ist identisch mit dem Informationsbit: $\ x_i^{(1)} = u_i ∈ \{0, \, 1\}$
- Das zweite Codebit ist das Prüfbit (Paritybit): $\ x_i^{(2)} = p_i ∈ \{0, \, 1\}$.
Hinweise:
- Die Aufgabe bezieht sich auf das Kapitel Grundlegendes zu den Turbocodes.
- Ähnliche Aufgaben finden Sie in den Kapiteln 3.1 bis 3.3. In den Fragen zu dieser Aufgabe werden folgende semi–infinite Vektoren verwendet:
- Informationssequenz $\ \underline{u} = (u_1, \, u_2, \, ...)$,
- Paritysequenz $\ \underline{p} = (p_1, \, p_2, \, ...)$,
- Impulsantwort $\ \underline{g} = (g_1, \, g_2, \, ...)$; diese ist gleich der Paritysequenz $\underline{p}$ für $\underline{u} = (1, \, 0, \, 0, \, ...)$.
- Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.
Fragebogen
Musterlösung
- $$S_0 → S_1 → S_2 → S_0 → S_0 → S_0 → \hspace{0.5cm} \Rightarrow \hspace{0.5cm}$ Impulsantwort: $\ \ \underline{g} = (1, \, 0, \, 1, \, 0, \, 0)$. Richtig ist der <u>Lösungsvorschlag 2</u>. Für ein nichtrekursives Filter mit Gedächtnis $m$ gilt $g_i ≡ 0$ für $i > m$. In unserem Beispiel ist $m = 2$. Der Lösungsvorschlag 1 gilt dagegen für das rekursive Filter (RSC) entsprechend der [[Aufgaben:4.09_Wiederholung_zu_den_RSC-Codes| Aufgabe A4.9]]. '''(2)''' Es sei $\underline{u} = (1, \, 0, \, 0, \, 1, \, 0, \, 0, \, u_7)$ und $\underline{g} = (1, \, 0, \, 1, \, 0, \, 0, \, 0, \, ...)$. Dann gilt für die Paritysequenz aufgrund der Linearität: :$$\underline{p} \hspace{-0.15cm} \ = \ \hspace{-0.15cm}
(\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} 0, \hspace{0.05cm} 0,\hspace{0.05cm} u_7\hspace{0.05cm} )
- (\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} 0
,\hspace{0.05cm} 0,\hspace{0.05cm} 0\hspace{0.05cm},\hspace{0.05cm} ...)= $$ :$$\ = \ \hspace{-0.15cm} (\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm}0, \hspace{0.05cm} ... \hspace{0.05cm})\oplus$$ :$$\ \oplus \ \hspace{-0.15cm} (\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm}0, \hspace{0.05cm} ... \hspace{0.05cm})\oplus$$ :$$\ \oplus \ \hspace{-0.15cm} (\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}0,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm} 0,\hspace{0.05cm} 0,\hspace{0.05cm} u_7,\hspace{0.05cm} 0,\hspace{0.05cm}u_7, \hspace{0.05cm} ... \hspace{0.05cm})= $$ :$$\ = \ \hspace{-0.15cm} (\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}0,\hspace{0.05cm}1,\hspace{0.05cm}\hspace{0.05cm}1,\hspace{0.05cm} 0,\hspace{0.05cm} 1,\hspace{0.05cm} u_7,\hspace{0.05cm} 0,\hspace{0.05cm}u_7, \hspace{0.05cm} ... \hspace{0.05cm})
\hspace{0.05cm}.$$
(3)
(4)
(5)