Difference between revisions of "Aufgaben:Exercise 4.3: Pointer Diagram Representation"
m (Oezdemir moved page Aufgabe 4.3: Zeigerdiagrammdarstellung to Exercise 4.3: Vector Diagram Representation) |
|||
| Line 5: | Line 5: | ||
[[File:P_ID716__Sig_A_4_3.png|250px|right|frame|Zeigerdiagramm einer Harmonischen]] | [[File:P_ID716__Sig_A_4_3.png|250px|right|frame|Zeigerdiagramm einer Harmonischen]] | ||
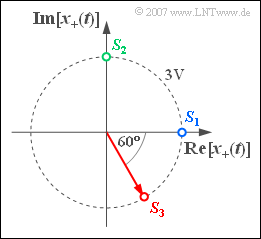
| − | + | We consider an analytical signal x+(t), which is defined by the drawn diagram in the complex plane. Depending on the choice of signal parameters, this results in three physical bandpass signals x1(t), x2(t) and x3(t), which differ by different starting points Si=xi(t=0) unterscheiden (blue, green and red point). In addition, the angular velocities of the three constellations are also different: | |
| − | * | + | *The analytical signal x1+(t) starts at S1=3 V. The angular velocity is ω1=π⋅104 1/s. |
| − | * | + | *The signal x2+(t) starts at the green starting point S2=j⋅3 V and, compared to x1+(t) , rotates with twice the angular velocity ⇒ ω2=2⋅ω1. |
| − | * | + | *The signal x_{3+}(t) starts at the red marked starting point S_3 = 3 \ \text{V} \cdot \text{e}^{–\text{j}\hspace{0.05cm}\cdot\hspace{0.05cm}\pi /3} and rotates with the same speed as the signal x_{2+}(t). |
| Line 18: | Line 18: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Signal_Representation/Analytical_Signal_and_Its_Spectral_Function|Analytical Signal and Its Spectral Function]]. |
| − | * | + | *The interactive applet [[Applets:Physikalisches_Signal_%26_Analytisches_Signal|Physikalisches Signal & Analytisches Signal]] illustrates the topic covered here. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What are the amplitudes of all signals considered?? |
|type="{}"} | |type="{}"} | ||
A\ = \ { 3 3% } \text{V} | A\ = \ { 3 3% } \text{V} | ||
| − | { | + | {What are the frequency and phase values of the signal x_1(t)? |
|type="{}"} | |type="{}"} | ||
f_1\ = \ { 5 3% } \text{kHz} | f_1\ = \ { 5 3% } \text{kHz} | ||
| − | \varphi_1\ = \ { 0. } $\text{ | + | \varphi_1\ = \ { 0. } $\text{deg}$ |
| − | { | + | {What are the frequency and phase values of the signal x_2(t)? |
|type="{}"} | |type="{}"} | ||
f_2\ = \ { 10 3% } \text{kHz} | f_2\ = \ { 10 3% } \text{kHz} | ||
| − | \varphi_2\ = \ { -91--89 } $\text{ | + | \varphi_2\ = \ { -91--89 } $\text{deg}$ |
| − | { | + | {What are the values of the frequency and phase of the signal x_3(t)? |
|type="{}"} | |type="{}"} | ||
f_3\ = \ { 10 3% } \text{kHz} | f_3\ = \ { 10 3% } \text{kHz} | ||
| − | \varphi_3\ = \ { 60 3% } $\text{ | + | \varphi_3\ = \ { 60 3% } $\text{deg}$ |
| − | { | + | {After what time t_1 is the analytical signal x_{3+}(t) equal to the initial value x_{3+}(t = 0)for the first time again? |
|type="{}"} | |type="{}"} | ||
t_1\ = \ { 0.1 3% } \text{ms} | t_1\ = \ { 0.1 3% } \text{ms} | ||
| − | { | + | {After what time t_2 is the physical signal x_3(t) for the first time again as large as at time t = 0? |
|type="{}"} | |type="{}"} | ||
t_2\ = \ { 0.033 3% } \text{ms} | t_2\ = \ { 0.033 3% } \text{ms} | ||
| Line 57: | Line 57: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The amplitude of the harmonic oscillation is equal to the pointer length. For all signals A \; \underline{= 3 \ \text{V}}. |
| − | '''(2)''' | + | '''(2)''' The sought frequency is given by f_1 = \omega_1/(2\pi ) \; \underline{= 5 \ \text{kHz}}. |
| − | * | + | *The phase can be determined from S_1 = 3 \ \text{V} \cdot \text{e}^{–\text{j} \hspace{0.05cm}\cdot \hspace{0.05cm} \varphi_1} and is \varphi_1 \; \underline{= 0}. |
| − | * | + | *In total this gives |
:$$x_1(t) = 3\hspace{0.05cm}{\rm V} | :$$x_1(t) = 3\hspace{0.05cm}{\rm V} | ||
| Line 70: | Line 70: | ||
| − | '''(3)''' | + | '''(3)''' Because of \omega_2 = 2\cdot \omega_1 , the frequency is now f_2 = 2 \cdot f_1 \; \underline{= 10 \ \text{kHz}}. |
| − | * | + | *The phase is obtained with the starting time S_2 at \text{e}^{–\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}\varphi_2} = \text{j} ⇒ \varphi_2 \; \underline{= -\pi /2 \; (-90^{\circ})}. |
| − | * | + | *Thus the time function is: |
:$$x_2(t) = 3\hspace{0.05cm}{\rm V} | :$$x_2(t) = 3\hspace{0.05cm}{\rm V} | ||
| Line 78: | Line 78: | ||
\cdot {\sin} ( 2 \pi \cdot {\rm 10 \hspace{0.05cm} kHz}\cdot t ).$$ | \cdot {\sin} ( 2 \pi \cdot {\rm 10 \hspace{0.05cm} kHz}\cdot t ).$$ | ||
| − | + | This signal is "minus-sine", which can also be read directly from the pointer diagram: | |
| − | * | + | *The real part of x_{2+}(t) at time t = 0 is zero. Since the pointer turns counterclockwise, the real part is negative at first. |
| − | * | + | *After a quarter turn, x_2(T/4) = - 3 \ \text{V}. |
| − | * | + | *If one continues to turn counterclockwise in steps of 90^\circ , the signal values 0 \ \text{V}, 3 \ \text{V} and 0 \ \text{V} result. |
| − | '''(4)''' | + | '''(4)''' This sub-task can be solved analogously to questions '''(2)''' and '''(3)''' : |
:f_3 \; \underline{= 10 \ \text{kHz}}, \ \varphi_3 \; \underline{= 60^\circ}. | :f_3 \; \underline{= 10 \ \text{kHz}}, \ \varphi_3 \; \underline{= 60^\circ}. | ||
| − | '''(5)''' | + | '''(5)''' The pointer requires exactly the period T_3 = 1/f_3 \; \underline{= 0.1 \ \text{ms}} \;(= t_1) for one revolution.. |
| − | '''(6)''' | + | '''(6)''' The analytical signal starts at S_3 = 3 \ \text{V} \cdot \text{e}^{–\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}60^{\circ}}. |
| − | * | + | *If the signal rotates further by 120^\circ , exactly the same real part results. |
| − | * | + | *The following relationship then applies with t_2 = t_1/3 \; \underline{= 0.033 \ \text{ms}} : |
:$$x_3(t = t_2) = x_3(t = 0) = 3\hspace{0.05cm}{\rm V} | :$$x_3(t = t_2) = x_3(t = 0) = 3\hspace{0.05cm}{\rm V} | ||
Revision as of 22:13, 4 February 2021
We consider an analytical signal x_+(t), which is defined by the drawn diagram in the complex plane. Depending on the choice of signal parameters, this results in three physical bandpass signals x_1(t), x_2(t) and x_3(t), which differ by different starting points S_i = x_i(t = 0) unterscheiden (blue, green and red point). In addition, the angular velocities of the three constellations are also different:
- The analytical signal x_{1+}(t) starts at S_1 = 3 \ \rm V. The angular velocity is \omega_1 = \pi \cdot 10^{4} \ 1/\text{s}.
- The signal x_{2+}(t) starts at the green starting point S_2 = {\rm j} \cdot 3 \ \text{V} and, compared to x_{1+}(t) , rotates with twice the angular velocity ⇒ \omega_2 = 2 \cdot \omega_1.
- The signal x_{3+}(t) starts at the red marked starting point S_3 = 3 \ \text{V} \cdot \text{e}^{–\text{j}\hspace{0.05cm}\cdot\hspace{0.05cm}\pi /3} and rotates with the same speed as the signal x_{2+}(t).
Hints:
- This exercise belongs to the chapter Analytical Signal and Its Spectral Function.
- The interactive applet Physikalisches Signal & Analytisches Signal illustrates the topic covered here.
Questions
Solution
(2) The sought frequency is given by f_1 = \omega_1/(2\pi ) \; \underline{= 5 \ \text{kHz}}.
- The phase can be determined from S_1 = 3 \ \text{V} \cdot \text{e}^{–\text{j} \hspace{0.05cm}\cdot \hspace{0.05cm} \varphi_1} and is \varphi_1 \; \underline{= 0}.
- In total this gives
- x_1(t) = 3\hspace{0.05cm}{\rm V} \cdot {\cos} ( 2 \pi \cdot {\rm 5 \hspace{0.05cm} kHz}\cdot t) .
(3) Because of \omega_2 = 2\cdot \omega_1 , the frequency is now f_2 = 2 \cdot f_1 \; \underline{= 10 \ \text{kHz}}.
- The phase is obtained with the starting time S_2 at \text{e}^{–\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}\varphi_2} = \text{j} ⇒ \varphi_2 \; \underline{= -\pi /2 \; (-90^{\circ})}.
- Thus the time function is:
- x_2(t) = 3\hspace{0.05cm}{\rm V} \cdot {\cos} ( 2 \pi \cdot {\rm 10 \hspace{0.05cm} kHz}\cdot t + 90^\circ) = -3\hspace{0.05cm}{\rm V} \cdot {\sin} ( 2 \pi \cdot {\rm 10 \hspace{0.05cm} kHz}\cdot t ).
This signal is "minus-sine", which can also be read directly from the pointer diagram:
- The real part of x_{2+}(t) at time t = 0 is zero. Since the pointer turns counterclockwise, the real part is negative at first.
- After a quarter turn, x_2(T/4) = - 3 \ \text{V}.
- If one continues to turn counterclockwise in steps of 90^\circ , the signal values 0 \ \text{V}, 3 \ \text{V} and 0 \ \text{V} result.
(4) This sub-task can be solved analogously to questions (2) and (3) :
- f_3 \; \underline{= 10 \ \text{kHz}}, \ \varphi_3 \; \underline{= 60^\circ}.
(5) The pointer requires exactly the period T_3 = 1/f_3 \; \underline{= 0.1 \ \text{ms}} \;(= t_1) for one revolution..
(6) The analytical signal starts at S_3 = 3 \ \text{V} \cdot \text{e}^{–\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}60^{\circ}}.
- If the signal rotates further by 120^\circ , exactly the same real part results.
- The following relationship then applies with t_2 = t_1/3 \; \underline{= 0.033 \ \text{ms}} :
- x_3(t = t_2) = x_3(t = 0) = 3\hspace{0.05cm}{\rm V} \cdot {\cos} ( 60^\circ) = 1.5\hspace{0.05cm}{\rm V} .