Difference between revisions of "Aufgaben:Exercise 1.2Z: Measurement of the Frequency Response"
| Line 51: | Line 51: | ||
| − | { | + | {Determine the damping value and the phase for filter $\rm B$ and $f_0 = 3 \ \text{kHz}$. |
|type="{}"} | |type="{}"} | ||
$a_{\rm B}(f_0 = \: \rm 3 \: kHz) \ = \ $ { 0.693 5% } $\text{Np}$ | $a_{\rm B}(f_0 = \: \rm 3 \: kHz) \ = \ $ { 0.693 5% } $\text{Np}$ | ||
| Line 58: | Line 58: | ||
| − | { | + | {What is the damping and phase value for for $f_0 = 2 \ \text{kHz}$? |
|type="{}"} | |type="{}"} | ||
$a_{\rm B}(f_0 = \: \rm 2 \: kHz) \ = \ $ { 0.916 5% } $\text{Np}$ | $a_{\rm B}(f_0 = \: \rm 2 \: kHz) \ = \ $ { 0.916 5% } $\text{Np}$ | ||
| Line 69: | Line 69: | ||
===Sample solution=== | ===Sample solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Approaches 2 und 3</u> are correct.: |
| − | *Bei einem | + | *Bei einem LTI–system gilt $Y(f) = X(f) · H(f)$. |
*Daher ist es nicht möglich, dass im Ausgangssignal ein Anteil mit $3 f_0$ vorhanden ist, wenn ein solcher im Eingangssignal fehlt. | *Daher ist es nicht möglich, dass im Ausgangssignal ein Anteil mit $3 f_0$ vorhanden ist, wenn ein solcher im Eingangssignal fehlt. | ||
*Das heißt: Es liegt hier kein LZI–System vor und dementsprechend ist auch kein Frequenzgang angebbar. | *Das heißt: Es liegt hier kein LZI–System vor und dementsprechend ist auch kein Frequenzgang angebbar. | ||
| Line 76: | Line 76: | ||
| − | '''(2)''' | + | '''(2)''' <u>Approach 3</u> is correct.: |
| − | * | + | *Based on the given numerical values for $A_y(f_0)$ filter $\rm B$ can be assumedd to be a <u>band-pass filter</u>. |
Revision as of 02:23, 29 June 2021
For the metrological determination of the filter frequency response, a sinusoidal input signal with an amplitude of $2 \hspace{0.05cm} \text{V}$ and given frequency $f_0$ is applied. The output signal $y(t)$ or its spectrum $Y(f)$ are then determined according to magnitude and phase.
- The magnitude spectrum at the output of filter $\rm A$ with frequency $f_0 = 1 \ \text{kHz}$ is:
- $$|Y_{\rm A} (f)| = 1.6\hspace{0.05cm}{\rm V} \cdot {\rm \delta } (f \pm f_0) + 0.4\hspace{0.05cm}{\rm V} \cdot {\rm \delta } (f \pm 3 f_0) .$$
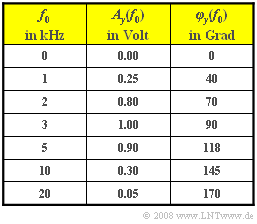
- For another filter $\rm B$ on the other hand, is always a harmonic oscillation with the (single) frequency $f_0$. For the frequencies $f_0$ given in the table the amplitudes $A_y(f_0)$ and the phases $φ_y(f_0)$ are measured. Here, the following holds:
- $$Y_{\rm B} (f) = {A_y}/{2} \cdot {\rm e}^{ {\rm j} \varphi_y} \cdot {\rm \delta } (f + f_0) + {A_y}/{2} \cdot {\rm e}^{ -{\rm j} \varphi_y} \cdot {\rm \delta } (f - f_0).$$
In the task, filter $\rm B$ should be given in the form:$$H_{\rm B}(f) = {\rm e}^{-a_{\rm B}(f)}\cdot {\rm e}^{-{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} b_{\rm B}(f)}.$$
Here,
- $a_{\rm B}(f)$ denotes the damping curve, and
- $b_{\rm B}(f)$ the phase response.
Please note:
- The task belongs to the chapter System Description in Frequency Domain.
Questions
Sample solution
- Bei einem LTI–system gilt $Y(f) = X(f) · H(f)$.
- Daher ist es nicht möglich, dass im Ausgangssignal ein Anteil mit $3 f_0$ vorhanden ist, wenn ein solcher im Eingangssignal fehlt.
- Das heißt: Es liegt hier kein LZI–System vor und dementsprechend ist auch kein Frequenzgang angebbar.
(2) Approach 3 is correct.:
- Based on the given numerical values for $A_y(f_0)$ filter $\rm B$ can be assumedd to be a band-pass filter.
(3) Mit $A_x = 2 \text{ V}$ und $\varphi_x = 90^\circ$ (Sinusfunktion) erhält man für $f_0 = f_3 =3 \text{ kHz}$:
- $$H_{\rm B} (f_3) = \frac{A_y}{A_x} \cdot {\rm e}^{ -{\rm j} (\varphi_x - \varphi_y)} = \frac{1\hspace{0.05cm}{\rm V}}{2\hspace{0.05cm}{\rm V}} \cdot {\rm e}^{ -{\rm j} (90^{\circ} - 90^{\circ})} = 0.5.$$
Somit ergeben sich für $f_0 = f_3 = 3 \text{ kHz}$ die Werte
- $a_{\rm B} (f_3)\rm \underline{\: ≈ \: 0.693 \: Np}$,
- $b_{\rm B}(f_3) \rm \underline{\: = \: 0 \: (Grad)}$.
(4) In analoger Weise kann der Frequenzgang bei $f_0 = f_2 =2 \text{ kHz}$ ermittelt werden:
- $$H_{\rm B} ( f_2) = \frac{0.8\hspace{0.05cm}{\rm V}}{2\hspace{0.05cm}{\rm V}} \cdot {\rm e}^{ -{\rm j} (90^{\circ} - 70^{\circ})} = 0.4\cdot {\rm e}^{ -{\rm j} 20^{\circ}}.$$
Damit erhält man für $f_0 = f_2 = 2 \ \text{ kHz}$:
- $a_{\rm B}(f_2) \rm \underline{\: ≈ \: 0.916 \: Np}$,
- $b_{\rm B}(f_2) \rm \underline{\: = \: 20°}$.
Bei $f_0 = -f_2 =-\hspace{-0.01cm}2 \text{ kHz}$ gilt der gleiche Dämpfungswert. Die Phase hat jedoch das umgekehrte Vorzeichen. Also ist $b_{\rm B}(–f_2) = \ –\hspace{-0.01cm}20^{\circ}.$