Aufgaben:Exercise 2.3Z: DSB-AM due to Nonlinearity

• Return to book

DSB–AM with nonlinearity

In this task, we consider the realization of a double-sideband amplitude modulation using the nonlinear characteristic curve: $$y = g(x) = c_1 \cdot x + c_2 \cdot x^2+ c_3 \cdot x^3\hspace{0.05cm}$$

$$ \Rightarrow c_1 = 2,\hspace{0.2cm}c_2 = 0.25/{\rm V},\hspace{0.2cm}c_3 = 0 \hspace{0.1cm}{\rm bzw.}\hspace{0.1cm}c_3 = 0.01/{\rm V^2}\hspace{0.05cm}.$$

The sum of the carrier signal and source signal is present at the input of this characteristic curve: $$ x(t) = z(t) + q(t) = A_{\rm T} \cdot \cos(\omega_{\rm T} t)+ q(t),\hspace{0.2cm} A_{\rm T} = 4\,{\rm V}\hspace{0.05cm}.$$

• It is known that the source signal  $q(t)$  contains spectral components between  $1 \ \rm kHz$  and  $9 \ \rm kHz$  (up to and including these limits).
• From subtask   (5)  onwards, the following source signal should be assumed:

$$q(t) = A_{\rm 1} \cdot \cos(\omega_{\rm 1} t)+A_{\rm 9} \cdot \cos(\omega_{\rm 9} t) \hspace{0.05cm}.$$

• Let the angular frequencies be  $ω_1 = 2 π · 1 \ \rm kHz$  and  $ω_9 = 2 π · 9\ \rm kHz$.  The corresponding amplitudes are given as:  $A_1 = 1\ \rm V$  and  $A_9 = 2\ \rm V$.

The following abbreviations are used in the questions for this task:

$$ y(t) = y_1(t) + y_2(t)+y_3(t),$$

$$y_1(t) = c_1 \cdot [z(t) + q(t)],$$

$$ y_2(t) = c_2 \cdot[z(t) + q(t)]^2,$$

$$y_3(t) = c_3 \cdot [z(t) + q(t)]^3 \hspace{0.05cm}.$$

Thus, the transmitted signals  $s(t)$  i.e.,  $s_1(t)$,  $s_2(t)$  and  $s_3(t)$ , result from band-limiting to the range from  $90 \ \rm kHz$  to  $110 \ \rm kHz$.

Hints:

• This exercise belongs to the chapter  Double-Sideband Amplitude Modulation.
• Particular reference is made to the page  Amplitude modulation with a quadratic characteristic curve.

• The following trigonometric transformations are given:

$$ \cos^2(\alpha) = {1}/{2} \cdot \left[ 1 + \cos(2\alpha)\right] \hspace{0.05cm}, \hspace{0.5cm} \cos^3(\alpha) = {1}/{4} \cdot \left[ 3 \cdot \cos(\alpha) + \cos(3\alpha)\right] \hspace{0.05cm}.$$

Questions

1

What is a reasonable choice for the carrier frequency?

$f_{\rm T} \ = \ $ $\ \text{kHz}$

2

What signal components does  $s_1(t)$ include?

	the term  $c_1 \cdot z(t)$,
	the term  $c_1 \cdot q(t)$.

3

What signal components does  $s_2(t)$ include?

	the term  $c_2 · z^2(t)$,
	the term  $c_2 · q^2(t)$,
	the term  $2c_2 · z(t) · q(t)$.

4

What signal components does  $s_3(t)$  (at least partially) include?

	the term  $c_3 · z^3(t)$,
	the term $3 · c_3 · z^2(t) · q(t)$,
	the term  $3 · c_3 · z(t) · q^2(t)$,
	the term  $c_3 · q^3(t)$.

5

Calculate  $s(t)$, when  $c_3 = 0$  and the source signal  $q(t)$  is composed of two cosine oscillations.
What is the modulation depth   $m$?

$m \ = \ $

6

Now calculate the transmitted signal  $s(t)$  given $c_3 = \rm 0.01/V^{2}$.  Which of the following statements is true?

	Because  $c_3 ≠ 0$  the spectral line changes at  $f_{\rm T}$ .
	Because  $c_3 ≠ 0$  linear distortions arise, which can be compensated for.
	Because  $c_3 ≠ 0$  nonlinear (i.e. irreversible) distortions arise.

Solution

Solution

(1)  Die Trägerfrequenz ist sinnvollerweise gleich der Mittenfrequenz des Bandpasses:  $f_{\rm T}\hspace{0.15cm}\underline{ = 100\ \rm kHz}$.

• Weicht  $f_{\rm T}$  um nicht mehr als  $±1 \ \rm kHz$  davon ab, ergibt sich ebenfalls eine „ZSB–AM”.

(2)  $s_1(t)$  beinhaltet nur den Träger  $z(t)$   ⇒   Antwort 1. Das Quellensignal  $q(t)$  wird durch den Bandpass entfernt.

(3)  Der quadratische Term  $z^2(t)$  besteht aus einem Gleichanteil  $($bei  $f = 0)$  sowie einem Anteil bei  $2f_{\rm T}$.

• Auch alle Spektralanteile von  $q^2(t)$  liegen außerhalb des Bandpasses.
• Richtig ist somit die letzte Antwort.

(4)  Richtig sind die Lösungsvorschläge 1 und 3:

• Der Term  $\cos^3(ω_Tt)$  hat seinen größten Signalanteil bei  $f = f_{\rm T}$.
• Der dritte Lösungsvorschlag  $(3 · c_3 · z(t) · q^2(t))$  liegt zwischen  $100\ \rm kHz ± 18 \ \rm kHz $.
• Teile davon – nämlich die Frequenzanteile zwischen  $90\ \rm kHz $ und $110 \ \rm kHz$  – werden durch den Bandpass nicht entfernt und sind somit auch in  $s(t)$  enthalten.

Erzeugtes AM–Spektrum

(5)  Das Sendesignal besteht aus insgesamt fünf Frequenzen:

$$s(t) = c_1 \cdot A_{\rm T} \cdot \cos(\omega_{\rm T} t)+ c_2 \cdot A_{\rm T} \cdot A_{\rm 1} \cdot \cos((\omega_{\rm T} \pm \omega_{\rm 1})t) + c_2 \cdot A_{\rm T} \cdot A_{\rm 2} \cdot \cos((\omega_{\rm T} \pm \omega_{\rm 2})t) \hspace{0.05cm}.$$

• Beachten Sie hierbei, dass der zweite und dritte Term jeweils zwei Signalfrequenzen beinhaltet:
  • $\text{99 kHz}$  und  $\text{101 kHz}$ bzw.
  • $\text{91 kHz}$  und  $\text{109 kHz}$.

• Mit  $A_{\rm T} = 4 \ \rm V$,  $A_1 = 1 V$,  $A_9 = 2 \ \rm V$,  $c_1 = 1$  und  $c_2 = 1/A_{\rm T} = \rm 0.25/V$  gilt auch:

$$s(t) = 4\,{\rm V} \cdot \cos(\omega_{\rm T} t) + 1\,{\rm V} \cdot \cos((\omega_{\rm T} \pm \omega_{\rm 1})t) + 2\,{\rm V}\cdot \cos((\omega_{\rm T} \pm \omega_{\rm 2})t) \hspace{0.05cm}.$$

• Daran erkennt man, dass für den Modulationsgrad gilt:

$$m =\frac{A_1 + A_9}{A_{\rm T}} = \rm \frac{1\ V + 2 \ V}{4 \ V} \hspace{0.15cm}\underline{=0.75}.$$

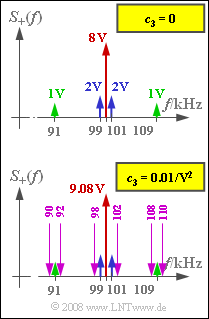
(6)  Die Grafik zeigt oben das Spektrum  $S_+(f)$  – also nur positive Frequenzen – mit  $c_3 = 0$. 

• Mit  $c_3 ≠ 0$  fallen folgende zusätzliche Spektralanteile an:

$$c_3 \cdot z^3(t)= \frac{c_3 \cdot A_{\rm T}^3}{4} \cdot \left[ 3 \cdot \cos(\omega_{\rm T} t) + \cos(3\omega_{\rm T} t)\right] \hspace{0.05cm}.$$

• Der erste Anteil fällt in den Durchlassbereich des Bandpasses.  Das Diracgewicht bei  $f_{\rm T} = 100\ \rm kHz$  wird dadurch von ursprünglich  $8 \ \rm V$  auf  $\text{8 V + 0.75 · 0.01/V}^2 · 4^3 \text{ V}^3 = 8.48 \ \rm V$  erhöht.

• Weiterhin liefert der dritte Spektralanteil von Teilaufgabe  (4)  einen unerwünschten Beitrag zu  $S_+(f)$.  Dabei gilt:

$$q^2(t) = \left[A_{\rm 1} \cdot \cos(\omega_{\rm 1} t)+A_{\rm 9} \cdot \cos(\omega_{\rm 9} t)\right]^2 = A_{\rm 1}^2 \cdot \cos^2(\omega_{\rm 1} t)+ A_{\rm 9}^2 \cdot \cos^2(\omega_{\rm 9}t) + 2 \cdot A_{\rm 1} \cdot A_{\rm 9} \cdot \cos(\omega_{\rm 1} t)\cdot \cos(\omega_{\rm 9} t)$$

$$ \Rightarrow \hspace{0.2cm} q^2(t) = \frac{A_{\rm 1}^2}{2} +\frac{A_{\rm 1}^2}{2} \cdot \cos(\omega_{\rm 2} t)+ \frac{A_{\rm 9}^2}{2} + \frac{A_{\rm 9}^2}{2} \cdot \cos(\omega_{\rm 18} t) + A_{\rm 1} \cdot A_{\rm 9} \cdot \cos(\omega_{\rm 8} t)+ A_{\rm 1} \cdot A_{\rm 9} \cdot \cos(\omega_{\rm 10} t).$$

• Nach der Multiplikation mit  $z(t)$  fallen alle diese Beiträge bis auf den vierten in den Bereich von  $\text{90 kHz}$  bis  $\text{110 kHz}$.  Das Gewicht bei  $f_{\rm T} = 100\ \rm kHz$  wird um  $3 · c_3 · A_{\rm T} · 0.5 (A_1^2 + A_9^2) = 0.6\ \rm V$  weiter erhöht und ist somit  $9.08 \ \rm V$.

Weitere Anteile ergeben sich bei:

• $98 \ \rm kHz$  und  $102 \ \rm kHz$  mit den Gewichten  $c_3 · A_{\rm T}/2 · A_1^2/2 = 0.03\ \rm V$,
• $92 \ \rm kHz$  und  $108 \ \rm kHz$  mit den Gewichten  $3c_3 · A_{\rm T}/2 · A_1 · A_9 = 0.12\ \rm V$,
• $90 \ \rm kHz$  und  $110 \ \rm kHz$  mit den Gewichten  $3c_3 · A_{\rm T}/2 · A_1 · A_9 = 0.12\ \rm V$.

Die untereSkizze in obiger Grafik zeigt das Spektrum  $S_+(f)$  unter Berücksichtigung der kubischen Anteile.  Man erkennt, dass neue Frequenzen entstanden sind, was auf nichtlineare Verzerrungen hindeutet.  Richtig sind somit die Lösungsvorschläge 1 und 3.