Difference between revisions of "Aufgaben:Exercise 3.3: Moments for Cosine-square PDF"
| Line 3: | Line 3: | ||
}} | }} | ||
| − | [[File:P_ID119__Sto_A_3_3.png|right|frame| | + | [[File:P_ID119__Sto_A_3_3.png|right|frame|Cosine–Rectangular PDF <br>and a similar PDF]] |
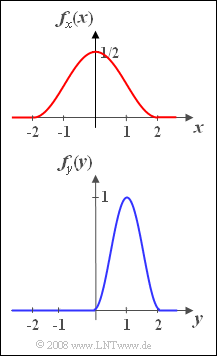
| − | + | As in [[Aufgaben:Exercise_3.1:_cos²-PDF_and_PDF_with_Dirac_Functions|Exercise 3.1]] and [[Aufgaben:Exercise_3.2:_cos²-CDF_and_CDF_with_Step_Functions|Exercise 3. 2]] we consider the random variable restricted to the range of values from $-2$ to $+2$ with the following PDF in this section: | |
:$$f_x(x)= {1}/{2}\cdot \cos^2({\pi}/{4}\cdot { x}).$$ | :$$f_x(x)= {1}/{2}\cdot \cos^2({\pi}/{4}\cdot { x}).$$ | ||
| − | + | Next to this, we consider a second random variable $y$ that returns only values between $0$ and $2$ with the following PDF: | |
| − | :$$f_y(y)=\sin^2({\pi}/{2}\cdot | + | :$$f_y(y)=\sin^2({\pi}/{2}\cdot y).$$ |
| − | * | + | *Both density functions are shown in the graph. |
| − | * | + | *Outside the ranges $-2 < x < +2$ resp. $0 < x < +2$ respectively $f_x(x) = 0$ resp. $f_y(y) = 0$ holds. |
| − | * | + | *Both random magnitudesö&ahead;can be taken as (normalized) instantaneous values of the associated random signals $x(t)$ or $y(t)$ respectively. |
| Line 22: | Line 22: | ||
| − | + | Hints: | |
| − | * | + | *This exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Expected_Values_and_Moments|expected values and moments]]. |
| − | * | + | *To solve this problem, you can use the following indefinite integral: |
:$$\int x^{2}\cdot {\cos}(ax)\,{\rm d}x=\frac{2 x}{ a^{ 2}}\cdot \cos(ax)+ \left [\frac{x^{\rm 2}}{\it a} - \frac{\rm 2}{\it a^{\rm 3}} \right ]\cdot \rm sin(\it ax \rm ) .$$ | :$$\int x^{2}\cdot {\cos}(ax)\,{\rm d}x=\frac{2 x}{ a^{ 2}}\cdot \cos(ax)+ \left [\frac{x^{\rm 2}}{\it a} - \frac{\rm 2}{\it a^{\rm 3}} \right ]\cdot \rm sin(\it ax \rm ) .$$ | ||
Revision as of 17:58, 27 December 2021
As in Exercise 3.1 and Exercise 3. 2 we consider the random variable restricted to the range of values from $-2$ to $+2$ with the following PDF in this section:
- $$f_x(x)= {1}/{2}\cdot \cos^2({\pi}/{4}\cdot { x}).$$
Next to this, we consider a second random variable $y$ that returns only values between $0$ and $2$ with the following PDF:
- $$f_y(y)=\sin^2({\pi}/{2}\cdot y).$$
- Both density functions are shown in the graph.
- Outside the ranges $-2 < x < +2$ resp. $0 < x < +2$ respectively $f_x(x) = 0$ resp. $f_y(y) = 0$ holds.
- Both random magnitudesö&ahead;can be taken as (normalized) instantaneous values of the associated random signals $x(t)$ or $y(t)$ respectively.
Hints:
- This exercise belongs to the chapter expected values and moments.
- To solve this problem, you can use the following indefinite integral:
- $$\int x^{2}\cdot {\cos}(ax)\,{\rm d}x=\frac{2 x}{ a^{ 2}}\cdot \cos(ax)+ \left [\frac{x^{\rm 2}}{\it a} - \frac{\rm 2}{\it a^{\rm 3}} \right ]\cdot \rm sin(\it ax \rm ) .$$
Fragebogen
Musterlösung
- Die erste Aussage ist nie erfüllt, wie aus dem Satz von Steiner ersichtlich ist.
- Die zweite Aussage gilt nur im (trivialen) Sonderfall $x = 0$.
Es gibt aber auch mittelwertfreie Zufallsgrößen mit unsymmetrischer WDF.
- Das bedeutet: Die Aussage 6 trifft nicht immer zu.
(2) Aufgrund der WDF-Symmetrie bezüglich $x = 0$ ergibt sich für den linearen Mittelwert $m_x \hspace{0.15cm}\underline{= 0}$.
(3) Der Effektivwert des Signals $x(t)$ ist gleich der Streuung $\sigma_x$ bzw. gleich der Wurzel aus der Varianz $\sigma_x^2$.
- Da die Zufallsgröße $x$ den Mittelwert $m_x {= 0}$ aufweist, ist die Varianz nach dem Satz von Steiner gleich dem quadratischen Mittelwert.
- Dieser wird in Zusammenhang mit Signalen auch als die Leistung $($bezogen auf $1 \ \rm \Omega)$ bezeichnet. Somit gilt:
- $$\sigma_x^{\rm 2}=\int_{-\infty}^{+\infty}x^{\rm 2}\cdot f_x(x)\hspace{0.1cm}{\rm d}x=2 \cdot \int_{\rm 0}^{\rm 2} x^2/2 \cdot \cos^2({\pi}/4\cdot\it x)\hspace{0.1cm} {\rm d}x.$$
- Mit der Beziehung $\cos^2(\alpha) = 0.5 \cdot \big[1 + \cos(2\alpha)\big]$ folgt daraus:
- $$\sigma_x^2=\int_{\rm 0}^{\rm 2}{x^{\rm 2}}/{\rm 2} \hspace{0.1cm}{\rm d}x + \int_{\rm 0}^{\rm 2}{x^{\rm 2}}/{2}\cdot \cos({\pi}/{\rm 2}\cdot\it x) \hspace{0.1cm} {\rm d}x.$$
- Diese beiden Standardintegrale findet man in Tabellen. Man erhält mit $a = \pi/2$:
- $$\sigma_x^{\rm 2}=\left[\frac{x^{\rm 3}}{\rm 6} + \frac{x}{a^2}\cdot {\cos}(a x) + \left( \frac{x^{\rm2}}{{\rm2}a} - \frac{1}{a^3} \right) \cdot \sin(a \cdot x)\right]_{x=0}^{x=2} \hspace{0.5cm} \Rightarrow \hspace{0.5cm} \sigma_{x}^{\rm 2}=\frac{\rm 4}{\rm 3}-\frac{\rm 8}{\rm \pi^2}\approx 0.524\hspace{0.5cm} \Rightarrow \hspace{0.5cm}\sigma_x \hspace{0.15cm}\underline{\approx 0.722}.$$
(4) Richtig ist der erstgenannte Vorschlag:
- Die Variante $y = 2x$ würde eine zwischen $-4$ und $+4$ verteilte Zufallsgröße liefern.
- Beim letzten Vorschlag $y = x/2-1$ wäre der Mittelwert $m_y = -1$.
(5) Aus der Grafik auf dem Angabenblatt ist bereits offensichtlich, dass $m_y \hspace{0.15cm}\underline{=+1}$ gelten muss.
(6) Der Mittelwert ändert nichts an der Varianz und an der Streuung.
- Durch die Stauchung um den Faktor $2$ wird die Streuung gegenüber Teilaufgabe (3) ebenfalls um diesen Faktor kleiner:
- $$\sigma_y=\sigma_x/\rm 2\hspace{0.15cm}\underline{\approx 0.361}.$$