Difference between revisions of "Aufgaben:Exercise 4.1: Triangular (x, y) Area"
| Line 3: | Line 3: | ||
}} | }} | ||
| − | [[File:P_ID172__Sto_A_4_1.png|right|frame| | + | [[File:P_ID172__Sto_A_4_1.png|right|frame|Triangular 2D area]] |
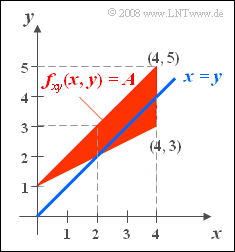
| − | + | A 2D random variable is defined by the adjacent sketch: | |
| − | *F | + | *F $(x, \ y)$ only values within the triangular-shaped region defined by the three vertices $(0,\ 1)$, $(4,\ 3)$, and $(4,\ 5)$ can occur. |
| − | * | + | *Within the triangle, all the random variables $(x,\ y)$ are equally probable. |
| − | * | + | *For the 2D–WDF, in this domain: |
:$$f_{xy}(x,y) = A .$$ | :$$f_{xy}(x,y) = A .$$ | ||
| − | + | In addition, the straight line $x = y$ ⇒ "angle bisector" is drawn in the sketch above ⇒ see subtask '''(2)'''. | |
| Line 19: | Line 19: | ||
| − | + | Hints: | |
| − | * | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Two-Dimensional_Random_Variables|Two-Dimensional Random Variables]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
Revision as of 21:01, 15 January 2022
A 2D random variable is defined by the adjacent sketch:
- F $(x, \ y)$ only values within the triangular-shaped region defined by the three vertices $(0,\ 1)$, $(4,\ 3)$, and $(4,\ 5)$ can occur.
- Within the triangle, all the random variables $(x,\ y)$ are equally probable.
- For the 2D–WDF, in this domain:
- $$f_{xy}(x,y) = A .$$
In addition, the straight line $x = y$ ⇒ "angle bisector" is drawn in the sketch above ⇒ see subtask (2).
Hints:
- The exercise belongs to the chapter Two-Dimensional Random Variables.
Questions
Musterlösung
- $$\int_{-\infty}^{+\infty}\int_{-\infty}^{+\infty}f_{xy}(x,y)\, {\rm d}x\, {\rm d}y=1.$$
- Die Dreiecksfläche ist $D = 0.5 \cdot 2 \cdot 4 = 4$.
- Da in diesem Definitionsgebiet die WDF konstant gleich $A$ ist, erhält man
- $$A= 1/D\hspace{0.15cm}\underline{= 0.25}.$$
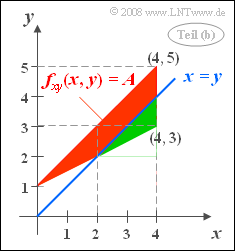
(2) Zur Lösung gehen wir von nebenstehender Skizze aus.
- Das Gebiet $x>y$ liegt rechts von der Winkelhalbierenden $x=y$ und ist grün markiert.
- Diese grüne Dreiecksfläche ist $D_{\rm (2)} = 0.5 \cdot 1 \cdot 2 = 1 $, also genau ein Viertel der Gesamtfläche $D$ des Definitionsgebietes.
- Daraus folgt ${\rm Pr}(x > y)\hspace{0.15cm}\underline{= 0.25}$.
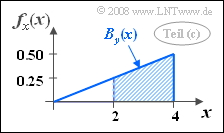
(3) Für die gesuchte Rand–WDF gilt in diesem Fall:
- $$f_x(x)=\int_{-\infty}^{+\infty}f_{xy}(x,y)\, {\rm d}y=A\cdot B_y (x).$$
- Hierbei bezeichnet $B_y(x)$ die Breite des Gebietes $f_{xy} \ne 0$ in $y$–Richtung beim betrachteten $x$–Wert.
- Es gilt: $B_y(x) = x/2$. Mit $A = 0.25$ folgt $f_{x}(x) = x/8$ für den Bereich $ 0 \le x \le 4$.
- Die gesuchte Wahrscheinlichkeit entspricht der schraffierten Fläche in nebenstehender Skizze. Man erhält:
- $$\rm Pr(\it x\ge \rm 2) = \rm 1-\rm Pr(\it x < \rm 2) = \rm 1-\frac{1}{2}\cdot2\cdot 0.25\hspace{0.15cm}\underline{ =0.75}. $$
- Zum gleichen Ergebnis gelangt man anhand der 2D–WDF: Rechts von $x = 2$ liegt $3/4$ des gesamten Definitionsgebiets.
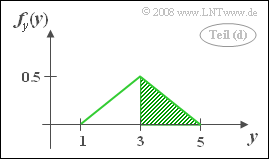
(4) Analog der Musterlösung zur Teilaufgabe (3) gilt:
- $$f_y(y)=\int_{-\infty}^{+\infty}f_{xy}(x,y)\, {\rm d}x=A\cdot B_x (y).$$
- Die Ausbreitung des WDF–Gebietes in $x$–Richtung ist für $y \le 1$ und $y \ge 5$ jeweils Null.
- Das Maximum liegt bei $y=3$ und ergibt $B_x(y=3) = 2$.
- Dazwischen ist die Zu– und Abnahme von $B_x(y)$ linear und es ergibt sich eine dreieckförmige WDF.
- Die Wahrscheinlichkeit, dass $y \ge 3$ ist, entspricht der grün schraffierten Fläche in nebenstehender Skizze.
- Aufgrund der Symmetrie erhält man:
- $${\rm Pr}(y ≥ 3)\hspace{0.15cm}\underline{ =0.5}. $$
Zum gleichen Ergebnis kommt man anhand der 2D–WDF: Oberhalb der Horizontalen $y= 3$ liegt die Hälfte des gesamten Definitionsgebietes.
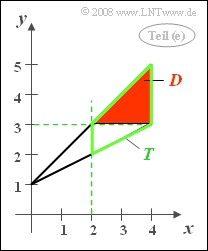
(5) Wenn $y \ge 3$ ist $($rot hinterlegtes Dreieck $D)$, gilt stets auch $x \ge 2$ $($grün umrandetes Trapez $T)$.
- Das bedeutet: In diesem Beispiel ist $D$ eine Teilmenge von $T$, und es gilt:
- $${\rm Pr}[(x ≥ 2) ∩ (y ≥ 3)] = {\rm Pr}(y ≥ 3) \hspace{0.15cm}\underline{= 0.50}.$$
(6) Entsprechend der Lösung zur Teilaufgabe (5) folgt aus $y \ge 3$ mit Sicherheit auch $x \ge 2$.
- Somit ist die gesuchte bedingte Wahrscheinlichkeit:
- $${\rm Pr}[x ≥ 2\hspace{0.05cm} | \hspace{0.05cm} y ≥ 3]\hspace{0.15cm}\underline{= 1}.$$
(7) Diese Teilaufgabe kann man mit dem Satz von Bayes und den Ergebnissen aus (2) und (5) lösen:
- $$\rm Pr(\it y \ge \rm 3\hspace{0.1cm}|\hspace{0.1cm} \it x \ge \rm 2) = \frac{ \rm Pr((\it x \ge \rm 2)\cap(\it y \ge \rm 3))} {\rm Pr(\it x \ge \rm 2)}=2/3\hspace{0.15cm}\underline{=0.667}.$$
- Oder anders ausgedrückt: Die Fläche $D$ des rot hinterlegten Dreiecks macht $2/3$ der Fläche des grün umrandeten Trapezes $T$ aus.