Difference between revisions of "Aufgaben:Exercise 1.07Z: Classification of Block Codes"
From LNTwww
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/General_Description_of_Linear_Block_Codes |
}} | }} | ||
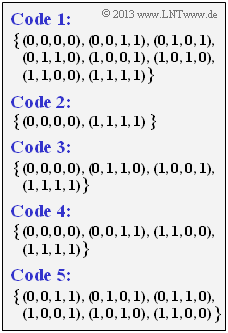
| − | [[File:P_ID2391__KC_Z_1_7_neu.png|right|frame| | + | [[File:P_ID2391__KC_Z_1_7_neu.png|right|frame|Block codes of length $n = 4$ ]] |
| − | + | We consider block codes of length $n = 4$: | |
| − | * | + | *the [[Channel_Coding/Examples_of_Binary_Block_Codes#Single_Parity-check_Codes|single parity–check]] code $\text{SPC (4, 3)}$ ⇒ "code 1" with the generator matrix |
:$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | :$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | ||
| − | * | + | *the [[Channel_Coding/Examples_of_Binary_Block_Codes#Repetition_Codes|repetition code]] $\text{RC (4, 1)}$ ⇒ "code 2" with the parity-check matrix |
:$${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | :$${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | ||
| − | * | + | *the $\text{(4, 2)}$ block code ⇒ "code 3" with the generator matrix |
:$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | :$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | ||
| − | * | + | *the $\text{(4, 2)}$ block code ⇒ "code 4" with the generator matrix |
:$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &0 &0\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | :$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &0 &0\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | ||
| − | * | + | *another "Code 5" with the code cardinality (Überstetzung von Codeumfang laut Wachter-Zeh VL) $|\hspace{0.05cm}C\hspace{0.05cm}| = 6$. |
| − | + | The individual codes are explicitly indicated in the graphic. The questions for these tasks are about the terms | |
| − | *[[Channel_Coding/ | + | *[[Channel_Coding/General_Description_of_Linear_Block_Codes#Linear_codes_and_cyclic_codes|linear codes]], |
| − | *[[Channel_Coding/ | + | *[[Channel_Coding/General_Description_of_Linear_Block_Codes#Systematic_Codes|systematic codes]], |
| − | *[[Channel_Coding/ | + | *[[Channel_Coding/General_Description_of_Linear_Block_Codes#Representation_of_SPC_and_RC_as_dual_codes|dual codes]]. |
| Line 40: | Line 40: | ||
| − | + | Hints : | |
| − | * | + | *This exercise belongs to the chapter [[Channel_Coding/General_Description_of_Linear_Block_Codes|General description of linear block codes]]. |
| − | * | + | *Reference is also made to the pages [[Channel_Coding/Examples_of_Binary_Block_Codes#Single_Parity-check_Codes|single parity–check codes]] and[[Channel_Coding/Examples_of_Binary_Block_Codes#Repetition_Codes|repetition codes]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
Revision as of 19:21, 30 June 2022
We consider block codes of length $n = 4$:
- the single parity–check code $\text{SPC (4, 3)}$ ⇒ "code 1" with the generator matrix
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$
- the repetition code $\text{RC (4, 1)}$ ⇒ "code 2" with the parity-check matrix
- $${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$
- the $\text{(4, 2)}$ block code ⇒ "code 3" with the generator matrix
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},$$
- the $\text{(4, 2)}$ block code ⇒ "code 4" with the generator matrix
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &0 &0\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$
- another "Code 5" with the code cardinality (Überstetzung von Codeumfang laut Wachter-Zeh VL) $|\hspace{0.05cm}C\hspace{0.05cm}| = 6$.
The individual codes are explicitly indicated in the graphic. The questions for these tasks are about the terms
Hints :
- This exercise belongs to the chapter General description of linear block codes.
- Reference is also made to the pages single parity–check codes andrepetition codes.
Questions
Musterlösung
(1) Richtig sind die Aussagen 1 und 2:
- Deshalb gibt es auch $\rm 4 \ über \ 2 = 6$ Codeworte.
- Aussage 3 ist falsch. Ist zum Beispiel das erste Bit $0$, so gibt es ein Codewort mit dem Beginn $00$ und zwei Codeworte, die mit $01$ beginnen.
(2) Richtig sind die Aussagen 1 bis 4:
- Alle Codes, die durch eine Generatormatrix $\boldsymbol {\rm G}$ und/oder eine Prüfmatrix $\boldsymbol {\rm H}$ beschrieben werden können, sind linear.
- Dagegen erfüllt "Code 5" keine der für lineare Codes erforderlichen Bedingungen. Beispielsweise
- fehlt das Nullwort,
- ist der Codeumfang $|\mathcal{C}|$ keine Zweierpotenz,
- ergibt $(0, 1, 0, 1) \oplus (1, 0, 1, 0) = (1, 1, 1, 1)$ kein gültiges Codewort.
(3) Richtig sind die Aussagen 1 bis 3:
- Bei einem systematischen Code müssen stets die ersten $k$ Bit eines jeden Codewortes $\underline{x}$ gleich dem Informationswort $\underline{u}$ sein.
- Dies wird erreicht, wenn der Beginn der Generatormatrix $\boldsymbol {\rm G}$ eine Einheitsmatrix $\boldsymbol{\rm I}_{k}$ darstellt.
- Dies trifft für "Code 1" (mit Dimension $k = 3$), "Code 2" (mit $k = 1$) und "Code 3" (mit $k = 2$) zu.
- Die Generatormatrix von "Code 2" ist allerdings nicht explizit angegeben. Sie lautet:
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$
(4) Richtig ist die Aussage 1:
- Von dualen Codes spricht man, wenn die Prüfmatrix $\boldsymbol {\rm H}$ des einen Codes gleich der Generatormatrix $\boldsymbol {\rm G}$ des anderen Codes ist.
- Dies trifft zum Beispiel für "Code 1" und "Code 2" zu.
- Für den SPC (4, 3) gilt:
- $${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm} { \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$
- und für den Wiederholungscode RC (4, 1):
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm} { \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$
- Aussage 2 ist mit Sicherheit falsch, schon aus Dimensionsgründen: Die Generatormatrix $\boldsymbol {\rm G}$ von "Code 3" ist eine $2×4$–Matrix und die Prüfmatrix $\boldsymbol {\rm H}$ von "Code 2" eine $3×4$–Matrix.
- "Code 3" und "Code 4" erfüllen ebenfalls nicht die Bedingungen dualer Codes. Die Prüfgleichungen von
- $${\rm Code}\hspace{0.15cm}3 = \{ (0, 0, 0, 0) \hspace{0.05cm},\hspace{0.1cm} (0, 1, 1, 0) \hspace{0.05cm},\hspace{0.1cm}(1, 0, 0, 1) \hspace{0.05cm},\hspace{0.1cm}(1, 1, 1, 1) \}$$
- lauten:
- $$x_1 \oplus x_4 = 0\hspace{0.05cm},\hspace{0.2cm}x_2 \oplus x_3 = 0 \hspace{0.3cm} \Rightarrow\hspace{0.3cm} { \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &1 &0 \end{pmatrix} \hspace{0.05cm}.$$
- Dagegen ist die Generatormatrix von "Code 4" wie folgt gegeben:
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &0 &0\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$