Difference between revisions of "Aufgaben:Exercise 1.08: Identical Codes"
From LNTwww
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/General_Description_of_Linear_Block_Codes |
}} | }} | ||
| − | [[File:P_ID2393__KC_A_1_8_neu.png|right|frame| | + | [[File:P_ID2393__KC_A_1_8_neu.png|right|frame|Assignment of the $(6, 3)$ block code]] |
| − | + | We consider a block code $\mathcal{C}$ described by the following generator matrix: | |
:$${ \boldsymbol{\rm G}} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm}.$$ | :$${ \boldsymbol{\rm G}} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | The mapping between the information words $\underline{u}$ and the code words $\underline{x}$ can be seen in the table. It can be seen that this is not a systematic code. | |
| − | + | By manipulating the generator matrix $\boldsymbol {\rm G}$ identical codes can be constructed from it. This refers to codes with the same code words but different assignments $\underline{u} \rightarrow \underline{x}$. | |
| − | |||
| − | * | + | The following operations are allowed to obtain identical code: |
| − | * | + | |
| − | * | + | *swapping or permuting the rows, |
| + | *Multiplying all rows by a constant vector not equal to $0$, | ||
| + | *Replacing a row with a linear combination between this row and another one. | ||
Revision as of 21:42, 5 July 2022
Assignment of the $(6, 3)$ block code
We consider a block code $\mathcal{C}$ described by the following generator matrix:
- $${ \boldsymbol{\rm G}} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm}.$$
The mapping between the information words $\underline{u}$ and the code words $\underline{x}$ can be seen in the table. It can be seen that this is not a systematic code.
By manipulating the generator matrix $\boldsymbol {\rm G}$ identical codes can be constructed from it. This refers to codes with the same code words but different assignments $\underline{u} \rightarrow \underline{x}$.
The following operations are allowed to obtain identical code:
- swapping or permuting the rows,
- Multiplying all rows by a constant vector not equal to $0$,
- Replacing a row with a linear combination between this row and another one.
Für den in der Teilaufgabe (3) gesuchten Code $\mathcal{C}_{\rm sys}$ mit Generatormatrix $\boldsymbol{\rm G}_{\rm sys}$ wird weiter gefordert, dass er systematisch ist.
Hinweise :
- Die Aufgabe gehört zum Kapitel Allgemeine Beschreibung linearer Blockcodes.
- Bezug genommen wird insbesondere auf die Seite Systematische Codes.
- Bezug genommen wird zudem auf die so genannte Singleton–Schranke. Diese besagt, dass die minimale Hamming–Distanz eines $(n, k)$–Blockcodes nach oben beschränkt ist: $d_{\rm min} \le n - k +1.$
Fragebogen
Musterlösung
(1) Der vorgegebene Code $\mathcal{C}$ wird durch folgende Kenngrößen charakterisiert:
- Bitanzahl der Codeworte: $\underline{n = 6}$,
- Bitanzahl der Informationsworte: $\underline{k = 3}$,
- Anzahl der Prüfbitgleichungen: $\underline{m = n - k = 3}$,
- Coderate: $R = k/n = 3/6 \Rightarrow \underline{R = 0.5}$,
- Anzahl der Codeworte (Codeumfang): $|\mathcal{C}| = 2^k \Rightarrow \underline{|C| = 8}$,
- minimale Hamming–Distanz (siehe Tabelle): $\underline{d}_{\rm min} \underline{= 3}$.
(2) Richtig ist $\underline{\rm JA}$:
- Nach der Singleton–Schranke gilt $d_{\rm min} ≤ n – k + 1$. Mit $n = 6$ und $k = 3$ erhält man hierfür $d_{\rm min} ≤ 4$.
- Es kann also durchaus ein (6, 3)–Blockcode mit größerer Minimaldistanz konstruiert werden. Wie ein solcher Code aussieht, wurde freundlicherweise nicht gefragt.
Die Minimaldistanz aller Hamming–Codes ist $d_{\rm min} = 3$, und nur der Sonderfall mit $n = 3$ und $k = 1$ erreicht den Grenzwert. Dagegen erreichen das Maximum entsprechend der Singleton–Schranke:
- alle Wiederholungscodes (Repetition Codes, RC) wegen $k = 1$und $d_{\rm min} = n$; hierzu gehört auch der (3, 1)–Hamming–Code, der ja bekannterweise identisch ist mit RC (3, 1),
- alle Single Parity–check Codes (SPC): $k = n – 1, d_{\rm min} = 2$.
(3) Richtig sind die Lösungsvorschläge 2 und 3:
- Vertauscht man Zeilen in der Generatormatrix $\boldsymbol {\rm G}$, so kommt man zu einem identischen Code $\mathcal{C}'$. Das heißt: Die Codes $\mathcal{C}$ und $\mathcal{C}'$ beinhalten die genau gleichen Codeworte.
- Beispielsweise erhält man nach zyklischem Zeilentausch $2 \rightarrow 1, 3 \rightarrow 2$ und $1 \rightarrow 3$ die neue Matrix
- $${ \boldsymbol{\rm G}}' = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$
- Die erste und die letzte Zeile der neuen Matrix entsprechen schon den Vorgaben eines systematischen Codes, nämlich, dass deren Generatormatrix ${ \boldsymbol{\rm G}_{\rm sys}}$ mit einer Diagonalmatrix beginnen muss.
- Ersetzt man die Zeile 2 durch die Modulo–2–Summe von Zeile 2 und 3, so erhält man:
- $${ \boldsymbol{\rm G}}_{\rm sys} = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &0 &1 &0 &1\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$
- Dieser systematische Code beinhaltet genau die gleichen Codeworte wie die Codes $\mathcal{C}$ und $\mathcal{C}'$.
(4) Richtig sind die Lösungsvorschläge 1 und 2:
- Wendet man die Gleichung $\underline{x}_{\rm sys} = \underline{u} \cdot \boldsymbol{\rm G}_{\rm sys}$ auf die obigen Beispiele an, so erkennt man, dass die beiden ersten Aussagen richtig sind, nicht aber die letzte.
- Ohne Rechnung kommt man zum gleichen Ergebnis, wenn man berücksichtigt, dass
- das systematische Codewort $\underline{x}_{\rm sys}$ mit $\underline{u}$ beginnen muss,
- der Code $\mathcal{C}_{\rm sys}$ die gleichen Codeworte beinhaltet wie der vorgegebene Code \mathcal{C}.
- Für $\underline{u} = (0, 1, 0)$ lautet somit das Codewort $(0, 1, 0, ?, ?, ?)$. Ein Vergleich mit der Codetabelle von $\mathcal{C}$ auf der Angabenseite führt zu $\underline{x}_{\rm sys} = (0, 1, 0, 1, 0, 1)$.
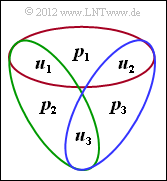
(5) Richtig ist nur die Aussage 1. Die Angaben für $p_{2}$ und $p_{3}$ sind dagegen genau vertauscht.
- Bei systematischer Codierung besteht folgender Zusammenhang zwischen Generator– und Prüfmatrix:
- $${ \boldsymbol{\rm G}} =\left({ \boldsymbol{\rm I}}_k \: ; \:{ \boldsymbol{\rm P}} \right) \hspace{0.3cm}\Leftrightarrow \hspace{0.3cm} { \boldsymbol{\rm H}} =\left({ \boldsymbol{\rm P}}^{\rm T}\: ; \:{ \boldsymbol{\rm I}}_m \right) \hspace{0.05cm}.$$
Schaubild der Prüfgleichungen
- Angewendet auf das aktuelle Beispiel erhält man so:
- $${ \boldsymbol{\rm G}}_{\rm sys} = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &0 &1 &0 &1\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.3cm} \Rightarrow\hspace{0.3cm} { \boldsymbol{\rm H}}_{\rm sys} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 1 &0 &1 &0 &1 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
Daraus ergeben sich Prüfgleichungen (siehe Grafik):
- $$u_1 \oplus u_2 \oplus p_1 \hspace{-0.15cm} \ = \ \hspace{-0.15cm}0 \hspace{0.3cm} \Rightarrow\hspace{0.3cm} p_1 = u_1 \oplus u_2 \hspace{0.05cm},$$
- $$ u_1 \oplus u_3 \oplus p_2 \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 0 \hspace{0.3cm} \Rightarrow\hspace{0.3cm} p_2 = u_1 \oplus u_3 \hspace{0.05cm},$$
- $$ u_2 \oplus u_3 \oplus p_3 \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 0 \hspace{0.3cm} \Rightarrow\hspace{0.3cm} p_3 = u_2 \oplus u_3 \hspace{0.05cm}.$$
