Difference between revisions of "Aufgaben:Exercise 4.08Z: Basics about Interleaving"
| Line 4: | Line 4: | ||
Interleaving is required, for example, for a channel with burst error characteristics in order to distribute the errors within the burst over a sufficiently large area so that they can subsequently be largely corrected (or at least detected). | Interleaving is required, for example, for a channel with burst error characteristics in order to distribute the errors within the burst over a sufficiently large area so that they can subsequently be largely corrected (or at least detected). | ||
| − | + | For turbo codes based on so-called '''RSC–Coder''' (<i>Recursive Systematic Convolutional Encoder</i>) – and only such make sense – <i>interleaving</i> is essential also with the AWGN channel, because then there are also always (some) input sequences, which deliver only zeros in the output sequence after quite a few ones, and that to infinity ⇒ there are output sequences with very small Hamming weight. | |
| − | + | If the bits of such input sequences are distributed over a wide range in the second coder, the problem can be (largely) eliminated by the interaction of both component decoders in the case of iterative symbol-wise decoding. | |
| − | + | A general distinction is made between | |
| − | * '''Block | + | * '''Block interleaver''' and |
| − | * '''Random | + | * '''Random interleaver'''. |
| − | + | In <i>block interleaving</i> one fills a matrix with $S$ columns and $Z$ rows column by column and reads the matrix row by row. This deterministically scrambles a block of information with $I_{\rm max} = S \cdot Z$ bits. | |
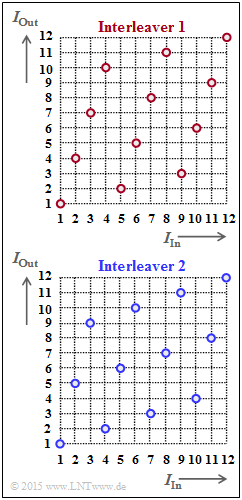
| − | + | On the right, two interleavers are indicated and in graphical form by the assignment $I_{\rm Out}(I_{\rm In})$. These quantities represent the "index of the output sequence" and the "index of the input sequence", respectively. It holds: | |
:$$1 \le I_{\rm Out} \le I_{\rm max} \hspace{0.05cm}, \hspace{0.5cm} | :$$1 \le I_{\rm Out} \le I_{\rm max} \hspace{0.05cm}, \hspace{0.5cm} | ||
1 \le I_{\rm In} \le I_{\rm max} \hspace{0.05cm}. $$ | 1 \le I_{\rm In} \le I_{\rm max} \hspace{0.05cm}. $$ | ||
| − | In | + | In the subtask '''(1)''' it is asked whether this is <i>block interleaving</i> oor <i>random interleaving</i> . Letztere werden im [[Channel_Coding/The_Basics_of_Turbo_Codes#Second_requirement_for_turbo_codes:_Interleaving|"Theorieteil"]] allerdings nur in aller Kürze besprochen. |
Revision as of 19:44, 28 November 2022
Interleaving is required, for example, for a channel with burst error characteristics in order to distribute the errors within the burst over a sufficiently large area so that they can subsequently be largely corrected (or at least detected).
For turbo codes based on so-called RSC–Coder (Recursive Systematic Convolutional Encoder) – and only such make sense – interleaving is essential also with the AWGN channel, because then there are also always (some) input sequences, which deliver only zeros in the output sequence after quite a few ones, and that to infinity ⇒ there are output sequences with very small Hamming weight.
If the bits of such input sequences are distributed over a wide range in the second coder, the problem can be (largely) eliminated by the interaction of both component decoders in the case of iterative symbol-wise decoding.
A general distinction is made between
- Block interleaver and
- Random interleaver.
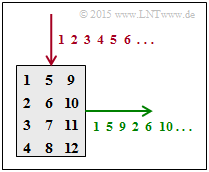
In block interleaving one fills a matrix with $S$ columns and $Z$ rows column by column and reads the matrix row by row. This deterministically scrambles a block of information with $I_{\rm max} = S \cdot Z$ bits.
On the right, two interleavers are indicated and in graphical form by the assignment $I_{\rm Out}(I_{\rm In})$. These quantities represent the "index of the output sequence" and the "index of the input sequence", respectively. It holds:
- $$1 \le I_{\rm Out} \le I_{\rm max} \hspace{0.05cm}, \hspace{0.5cm} 1 \le I_{\rm In} \le I_{\rm max} \hspace{0.05cm}. $$
In the subtask (1) it is asked whether this is block interleaving oor random interleaving . Letztere werden im "Theorieteil" allerdings nur in aller Kürze besprochen.
Hinweis:
- Die Aufgabe bezieht sich auf das Kapitel Grundlegendes zu den Turbocodes.
- Aber auch in anderen $\rm LNTwww$–Büchern wird Interleaving behandelt, unter anderem im Buch "Beispiele von Nachrichtensystemen" mit Bezug zum
- Standard Digital Subscriber Line (DSL) ⇒ Interleaving und De–Interleaving,
- 2G–Mobilfunksystem GSM ⇒ Komponenten der Sprach– und Datenübertragung,
- 3G–Mobilfunksystem UMTS ⇒ Kanalcodierung,
- 4G–Mobilfunksystem LTE ⇒ Funktionsweise von SC–FDMA (im Buch "Mobile Kommunikation").
Fragebogen
Musterlösung
(1) Aus der regelmäßigen Struktur der Funktion $I_{\rm Out}(I_{\rm In})$ erkennt man, dass es sich um einen Blockinterleaver handelt ⇒ Antwort 1.
(2) Der Index "1" wird als erstes Zeichen ausgegeben. Weiter gilt:
- Der Index 5 wird als zweites Zeichen ausgegeben ⇒ $\underline{Z = 4}$.
- Der Index 2 wird als viertes Zeichen ausgegeben ⇒ $\underline{S = 3}$.
Die obere Grafik zeigt für die 4×3–Interleaver–Matrix:
- das spaltenweise Beschreiben (rot),
- das zeilenweise Auslesen (grün).
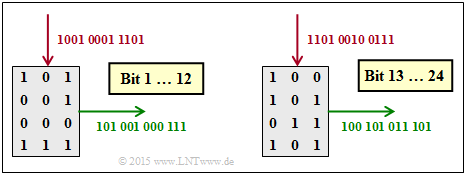
(3) Richtig ist der der Lösungsvorschlag 2:
- Die Matrix wird spaltenweise beschrieben und zeilenweise ausgelesen.
- Nach 12 Bit wird die Matrix gelöscht und die Prozedur beginnt von Neuem.
- Die Grafik zeigt, dass nun der Lösungsvorschlag 2 richtig ist.
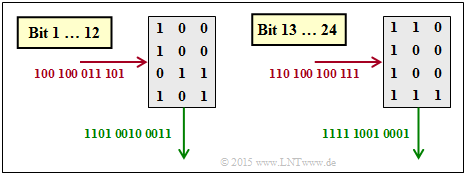
(4) Richtig ist der der Lösungsvorschlag 1:
- Beim De–Interleaving wird die Matrix zeilenweise beschrieben und spaltenweise ausgelesen.
- Die Grafik zeigt, dass hier der Lösungsvorschlag 1 richtig ist.