Difference between revisions of "Aufgaben:Exercise 4.1Z: Calculation of Moments"
| Line 62: | Line 62: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | + | <b>a)</b> Die Fläche unter der WDF muss immer 1 sein. Daraus folgt für die Exponentialverteilung: | |
| − | '''2. | + | $$A_{X} \cdot\int_{0}^{\infty} \hspace{-0.01cm} {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = A_{X} \cdot (-1/\lambda)\cdot\left [{\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\right ]_{0}^{\infty} = A_{X} \cdot (1/\lambda) \stackrel{!}{=} 1 |
| − | + | \hspace{0.3cm} \Rightarrow\hspace{0.3cm} A_{X} = \lambda \hspace{0.05cm}. $$ | |
| − | + | Richtig ist somit der <u>Lösungsvorschlag 2</u>. | |
| − | + | ||
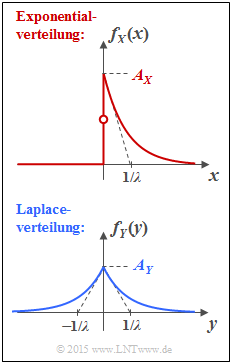
| − | + | <b>b)</b> Aus der Grafik auf der Angabenseite erkennt man, dass die Höhe <i>A<sub>Y</sub></i> der Laplaceverteilung nur halb so groß ist wie das Maximum der Exponentialverteilung ⇒<i>A<sub>Y</sub></i> = <i>λ</i>/2 ⇒ <u>Lösungsvorschlag 1</u>. | |
| − | + | ||
| + | <b>c)</b> Richtig ist <u>JA</u>, obwohl für <i>z</i> ≠ 0 stets <i>f<sub>X</sub></i>(<i>z</i>) ≠ <i>f<sub>Y</sub></i>(<i>z</i>) gilt. Betrachten wir nun den Sonderfall <i>z</i> = 0: | ||
| + | :* Für die Laplaceverteilung gilt <i>f<sub>Y</sub></i>(0) = <i>λ</i>/2. | ||
| + | :* Bei der Exponentialverteilung unterscheiden sich der links- und der rechtsseitige Grenzwert für <i>x</i> → 0. Der WDF–Wert an der Stelle <i>x</i> = 0 ist der Mittelwert dieser beiden Grenzwerte: | ||
| + | $$f_X(0) = \frac{1}{2} \cdot [ 0 + \lambda] = \lambda/2 = f_Y(0)\hspace{0.05cm}.$$ | ||
| + | |||
| + | <b>d)</b> Bei der Exponentialverteilung erhält man entsprechend [http://en.lntwww.de/Biografien_und_Bibliografien/Buchstaben_A_-_D#Buchstabe_B '''[BS01]'''] für | ||
| + | :* den linearen Mittelwert (Moment erster Ordnung): | ||
| + | $$m_1 = \lambda \cdot\int_{0}^{\infty} \hspace{-0.01cm} x \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = \lambda \cdot \left [\frac{{\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}}{(-\lambda)^2}\cdot(-\lambda \cdot x-1)\right ]_{0}^{\infty}= {1}/{\lambda} \hspace{0.05cm},$$ | ||
| + | :* den quadratischen Mittelwert (Moment zweiter Ordnung): | ||
| + | $$m_2 = \lambda \cdot\int_{0}^{\infty} \hspace{-0.01cm} x^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = \lambda \cdot\left [ {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\cdot | ||
| + | (\frac{x^2}{-\lambda} - \frac{2x}{\lambda^2} + \frac{2}{\lambda^3}) | ||
| + | \right ]_{0}^{\infty} ={2}/{\lambda^2} \hspace{0.05cm}.$$ | ||
| + | Daraus ergibt sich mit dem Satz von Steiner für die Varianz der Exponentialverteilung: | ||
| + | $$\sigma^2 = m_2 - m_1^2 = {2}/{\lambda^2} -{1}/{\lambda^2} = {1}/{\lambda^2} | ||
| + | \hspace{0.3cm} \Rightarrow\hspace{0.3cm} | ||
| + | \sigma = {1}/{\lambda}\hspace{0.05cm}.$$ | ||
| + | Richtig sind also <u>alle Lösungsvorschläge</u>. <i>Hinweis:</i> Bei der Exponentialverteilung berechnet sich das Moment <i>k</i>–ter Ordnung allgemein zu <i>m<sub>k</sub></i> = <i>k</i>!/<i>λ</i><sup><i>k</i></sup> ⇒ <i>m</i><sub>1</sub> = 1/<i>λ</i>, <i>m</i><sub>2</sub> = 2/<i>λ</i><sup>2</sup>, <i>m</i><sub>3</sub> = 6/<i>λ</i><sup>3</sup>, ... | ||
| + | |||
| + | <b>e)</b> Richtig ist nur der <u>Lösungsvorschlag 2</u>: Der quadratische Mittelwert der Laplaceverteilung ist aufgrund der symmetrischen WDF genau so groß wie bei der Exponentialverteilung: | ||
| + | $$m_2 = \frac{\lambda}{2} \cdot \int_{-\infty}^{\infty} \hspace{-0.01cm} y^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}|y|}\hspace{0.1cm}{\rm d}y = \lambda \cdot\int_{0}^{\infty} \hspace{-0.01cm} y^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}y}\hspace{0.1cm}{\rm d}y = {2}/{\lambda^2} \hspace{0.05cm}.$$ | ||
| + | Der Mittelwert der Laplaceverteilung ist <i>m</i><sub>1</sub> = 0. Damit ist die Varianz der Laplaceverteilung doppelt so groß wie bei der Exponentialverteilung: | ||
| + | $$\sigma^2 = m_2 - m_1^2 = {2}/{\lambda^2} - 0 ={2}/{\lambda^2} | ||
| + | \hspace{0.3cm} \Rightarrow\hspace{0.3cm} | ||
| + | \sigma = {\sqrt{2}}/{\lambda}\hspace{0.05cm}.$$ | ||
| + | |||
| + | [[File:P_ID2864__Inf_Z_4_1f_neu.png|right|]] | ||
| + | |||
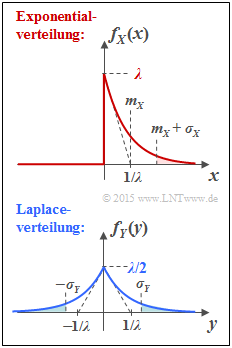
| + | <b>f)</b> Für die Exponentialverteilung ergibt sich entsprechend der oberen Grafik mit <i>m<sub>X</sub></i> = <i>σ<sub>X</sub></i> = 1/<i>λ</i>: | ||
| + | $${\rm Pr}( |X - m_X| > \sigma_X) = | ||
| + | {\rm Pr}( X > 2/\lambda) $$ $$\ | ||
| + | = \lambda \cdot\int_{2/\lambda}^{\infty} \hspace{-0.01cm} {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = | ||
| + | -\left [ {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x} | ||
| + | \right ]_{2/\lambda}^{\infty}$$ $$\ | ||
| + | = {\rm e}^{-2} \hspace{0.15cm}\underline {\approx 0.135}\hspace{0.05cm}.$$ | ||
| + | Für die Laplaceverteilung (untere Grafik) erhält man mit <i>m<sub>Y</sub></i> = 0 und <i>σ<sub>Y</sub></i> = 2<sup>0.5</sup>/<i>λ</i>: | ||
| + | $${\rm Pr}( |Y - m_Y| > \sigma_Y) = | ||
| + | 2 \cdot {\rm Pr}( Y > \sqrt{2}/\lambda) $$ $$\ | ||
| + | = 2 \cdot \frac{\lambda}{2} \cdot\int_{\sqrt{2}/\lambda}^{\infty} \hspace{-0.01cm} {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = | ||
| + | \left [ {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x} | ||
| + | \right ]_{\sqrt{2}/\lambda}^{\infty}$$ $$\ | ||
| + | = - {\rm e}^{-\sqrt{2}} \hspace{0.15cm}\underline {\approx 0.243}\hspace{0.05cm}.$$ | ||
| + | |||
| + | Ein Vergleich der schraffierten Flächen in nebenstehender Grafik bestätigt das Ergebnis qualitativ: Die blauen Flächen sind zusammen etwas größer als die rote Fläche. | ||
| + | |||
| + | |||
| + | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 17:52, 21 March 2017
Die Grafik zeigt oben die Wahrscheinlichkeitsdichtefunktion (WDF) der Exponentialverteilung: $$f_X(x) = \left\{ \begin{array}{c} A_{ X} \cdot {\rm exp}(-\lambda \cdot x) \\ A_{ X}/2 \\ 0 \\ \end{array} \right. \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \hspace{0.1cm}x>0, \\ {\rm{f\ddot{u}r}} \hspace{0.1cm}x=0, \\ {\rm{f\ddot{u}r}} \hspace{0.1cm}x<0. \\ \end{array}$$ Darunter gezeichnet ist die WDF der Laplaceverteilung, die für alle y–Werte wie folgt angegeben werden kann: $$f_Y(y) = A_{ Y} \cdot {\rm exp}(-\lambda \cdot |y|)\hspace{0.05cm}.$$ Die zwei wertkontinuierlichen Zufallsgrößen X und Y sollen hinsichtlich der folgenden Kenngrößen verglichen werden:
- dem linearen Mittelwert m1 (Moment erster Ordnung),
- dem Moment zweiter Ordnung ⇒ m2,
- der Varianz σ2 = m2 – m12 (Satz von Steiner), und
- der Streuung σ.
Hinweis: Die Aufgabe gehört zum Kapitel 4.1 des vorliegenden Buches. Sie fasst gleichzeitig die erforderlichen Vorkenntnisse von Kapitel 3 des Buches „Stochastische Signaltheorie” zusammen. Gegeben sind außerdem die beiden unbestimmten Integrale: $$\int \hspace{-0.01cm} x \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = \frac{{\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}}{(-\lambda)^2}\cdot(-\lambda \cdot x-1)\hspace{0.05cm}, $$ $$\int \hspace{-0.01cm} x^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\cdot (\frac{x^2}{-\lambda} - \frac{2x}{\lambda^2} + \frac{2}{\lambda^3}) \hspace{0.05cm}. $$
Fragebogen
Musterlösung
b) Aus der Grafik auf der Angabenseite erkennt man, dass die Höhe AY der Laplaceverteilung nur halb so groß ist wie das Maximum der Exponentialverteilung ⇒AY = λ/2 ⇒ Lösungsvorschlag 1.
c) Richtig ist JA, obwohl für z ≠ 0 stets fX(z) ≠ fY(z) gilt. Betrachten wir nun den Sonderfall z = 0:
- Für die Laplaceverteilung gilt fY(0) = λ/2.
- Bei der Exponentialverteilung unterscheiden sich der links- und der rechtsseitige Grenzwert für x → 0. Der WDF–Wert an der Stelle x = 0 ist der Mittelwert dieser beiden Grenzwerte:
$$f_X(0) = \frac{1}{2} \cdot [ 0 + \lambda] = \lambda/2 = f_Y(0)\hspace{0.05cm}.$$
d) Bei der Exponentialverteilung erhält man entsprechend [BS01] für
- den linearen Mittelwert (Moment erster Ordnung):
$$m_1 = \lambda \cdot\int_{0}^{\infty} \hspace{-0.01cm} x \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = \lambda \cdot \left [\frac{{\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}}{(-\lambda)^2}\cdot(-\lambda \cdot x-1)\right ]_{0}^{\infty}= {1}/{\lambda} \hspace{0.05cm},$$
- den quadratischen Mittelwert (Moment zweiter Ordnung):
$$m_2 = \lambda \cdot\int_{0}^{\infty} \hspace{-0.01cm} x^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = \lambda \cdot\left [ {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\cdot (\frac{x^2}{-\lambda} - \frac{2x}{\lambda^2} + \frac{2}{\lambda^3}) \right ]_{0}^{\infty} ={2}/{\lambda^2} \hspace{0.05cm}.$$ Daraus ergibt sich mit dem Satz von Steiner für die Varianz der Exponentialverteilung: $$\sigma^2 = m_2 - m_1^2 = {2}/{\lambda^2} -{1}/{\lambda^2} = {1}/{\lambda^2} \hspace{0.3cm} \Rightarrow\hspace{0.3cm} \sigma = {1}/{\lambda}\hspace{0.05cm}.$$ Richtig sind also alle Lösungsvorschläge. Hinweis: Bei der Exponentialverteilung berechnet sich das Moment k–ter Ordnung allgemein zu mk = k!/λk ⇒ m1 = 1/λ, m2 = 2/λ2, m3 = 6/λ3, ...
e) Richtig ist nur der Lösungsvorschlag 2: Der quadratische Mittelwert der Laplaceverteilung ist aufgrund der symmetrischen WDF genau so groß wie bei der Exponentialverteilung: $$m_2 = \frac{\lambda}{2} \cdot \int_{-\infty}^{\infty} \hspace{-0.01cm} y^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}|y|}\hspace{0.1cm}{\rm d}y = \lambda \cdot\int_{0}^{\infty} \hspace{-0.01cm} y^2 \cdot {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}y}\hspace{0.1cm}{\rm d}y = {2}/{\lambda^2} \hspace{0.05cm}.$$ Der Mittelwert der Laplaceverteilung ist m1 = 0. Damit ist die Varianz der Laplaceverteilung doppelt so groß wie bei der Exponentialverteilung: $$\sigma^2 = m_2 - m_1^2 = {2}/{\lambda^2} - 0 ={2}/{\lambda^2} \hspace{0.3cm} \Rightarrow\hspace{0.3cm} \sigma = {\sqrt{2}}/{\lambda}\hspace{0.05cm}.$$
f) Für die Exponentialverteilung ergibt sich entsprechend der oberen Grafik mit mX = σX = 1/λ: $${\rm Pr}( |X - m_X| > \sigma_X) = {\rm Pr}( X > 2/\lambda) $$ $$\ = \lambda \cdot\int_{2/\lambda}^{\infty} \hspace{-0.01cm} {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = -\left [ {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x} \right ]_{2/\lambda}^{\infty}$$ $$\ = {\rm e}^{-2} \hspace{0.15cm}\underline {\approx 0.135}\hspace{0.05cm}.$$ Für die Laplaceverteilung (untere Grafik) erhält man mit mY = 0 und σY = 20.5/λ: $${\rm Pr}( |Y - m_Y| > \sigma_Y) = 2 \cdot {\rm Pr}( Y > \sqrt{2}/\lambda) $$ $$\ = 2 \cdot \frac{\lambda}{2} \cdot\int_{\sqrt{2}/\lambda}^{\infty} \hspace{-0.01cm} {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x}\hspace{0.1cm}{\rm d}x = \left [ {\rm e}^{-\lambda \hspace{0.05cm}\cdot \hspace{0.05cm}x} \right ]_{\sqrt{2}/\lambda}^{\infty}$$ $$\ = - {\rm e}^{-\sqrt{2}} \hspace{0.15cm}\underline {\approx 0.243}\hspace{0.05cm}.$$
Ein Vergleich der schraffierten Flächen in nebenstehender Grafik bestätigt das Ergebnis qualitativ: Die blauen Flächen sind zusammen etwas größer als die rote Fläche.