Difference between revisions of "Aufgaben:Exercise 3.3Z: Convolution and D-Transformation"
(Die Seite wurde neu angelegt: „{{quiz-Header|Buchseite=Kanalcodierung/Algebraische und polynomische Beschreibung }} [[File:|right|]] ===Fragebogen=== <quiz display=simple> {Multipl…“) |
|||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite=Kanalcodierung/Algebraische und polynomische Beschreibung | + | {{quiz-Header|Buchseite=Kanalcodierung/Algebraische und polynomische Beschreibung}} |
| + | [[File:P_ID2628__KC_Z_3_3.png|right|frame|Vorgegebene Filter]] | ||
| + | In dieser Aufgabe beschreiben wir an einem einfachen Beispiel | ||
| + | * die endliche <span style="color: rgb(204, 0, 0);"><b>Impulsantwort</b></span> eines Filters: | ||
| + | :$$\underline{g} = \left (g_0, g_1, \hspace{0.05cm}...\hspace{0.1cm}, g_l, \hspace{0.05cm}...\hspace{0.1cm}, g_m \right ) | ||
| + | \hspace{0.05cm},\hspace{0.2cm}g_l \in {\rm GF(2) } = \{ 0, 1 \}\hspace{0.05cm}, $$ | ||
| + | * die <span style="color: rgb(204, 0, 0);"><b>Eingangssequenz</b></span> des Filters: | ||
| + | :$$\underline{u} = \left (u_0, u_1, \hspace{0.05cm}...\hspace{0.1cm}, u_i, \hspace{0.05cm}...\hspace{0.1cm} \right ) | ||
| + | \hspace{0.05cm},\hspace{0.2cm}u_i \in {\rm GF(2) } = \{ 0, 1 \}\hspace{0.05cm}, $$ | ||
| + | * die <span style="color: rgb(204, 0, 0);"><b>Ausgangssequenz</b></span> des Filters: | ||
| + | :$$\underline{x} = \left (x_0, x_1, \hspace{0.05cm}...\hspace{0.1cm}, x_i, \hspace{0.05cm}...\hspace{0.1cm} \right ) | ||
| + | \hspace{0.05cm},\hspace{0.2cm}x_i \in {\rm GF(2) } = \{ 0, 1 \}\hspace{0.05cm}. $$ | ||
| + | Die Nomenklatur für diese (digitale) Filterbeschreibung haben wir an das Buch „Einführung in die Kanalcodierung” angepasst. In anderen Büchern bezeichnet oft $\underline{x}$ den Filtereingang, $\underline{y}$ den Filterausgang, und die Impulsantwort wird $\h$ genannt. | ||
| + | Allgemein gilt für die Ausgangssequenz entsprechend der [[Signaldarstellung/Faltungssatz_und_Faltungsoperation#Faltung_im_Zeitbereich| Faltung]] (englisch: <i>Convolution</i>): | ||
| + | :$$\underline{x} = \underline{u}* \underline{g} = \left (x_0, x_1, \hspace{0.05cm}...\hspace{0.1cm}, x_i, \hspace{0.05cm}...\hspace{0.1cm} \right )\hspace{0.05cm},\hspace{0.1cm} {\rm mit} \hspace{0.2cm} x_i = \sum_{l = 0}^{m} g_l \cdot u_{i-l}\hspace{0.05cm}.$$ | ||
| − | }} | + | Wir repräsentieren nun die Zeitfunktionen $\underline{g}, \ \underline{u}$ und $\underline{x}$ durch Polynome in einer Dummy–Variablen $D$ und nennen diese die $D$–Transformierten: |
| + | :$$\underline{g} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} | ||
| + | {G}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{l = 0}^{m} g_l \cdot D\hspace{0.03cm}^l = g_0 + g_1 \cdot D + g_2 \cdot D^2 + ... + g_m \cdot D\hspace{0.03cm}^m\hspace{0.05cm},$$ | ||
| + | :$$\underline{u} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} | ||
| + | {U}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{i = 0}^{\infty} u_i \cdot D\hspace{0.03cm}^i = u_0 + u_1 \cdot D + u_2 \cdot D^2 + ... \hspace{0.05cm},$$ | ||
| + | :$$\underline{x} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} | ||
| + | {X}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{i = 0}^{\infty} x_i \cdot D\hspace{0.03cm}^i = x_0 + x_1 \cdot D + x_2 \cdot D^2 + ... \hspace{0.05cm}.$$ | ||
| + | |||
| + | Damit wird aus der (komplizierteren) Faltung eine Multiplikation: | ||
| + | :$$\underline{x} = \underline{u}* \underline{g} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} | ||
| + | {X}(D) = U(D) \cdot G(D) \hspace{0.05cm}.$$ | ||
| + | |||
| + | Formal lässt sich dieser Zusammenhang wie folgt nachweisen: | ||
| + | :$${X}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{i = 0}^{\infty} x_i \cdot D\hspace{0.03cm}^i = \sum_{i = 0}^{\infty} \sum_{l = 0}^{m}\hspace{0.1cm} | ||
| + | g_l \cdot u_{i-l} \cdot D\hspace{0.03cm}^{i} = \sum_{l = 0}^{m} \hspace{0.1cm} g_l \cdot \sum_{j = -l}^{\infty} \hspace{0.1cm} | ||
| + | u_{j} \cdot D\hspace{0.03cm}^{j+l} = $$ | ||
| + | :$$ \ = \ \hspace{-0.15cm} \sum_{l = 0}^{m} \hspace{0.1cm} g_l \cdot D\hspace{0.03cm}^l \hspace{0.1cm} \cdot \hspace{0.1cm} \sum_{j = 0}^{\infty} \hspace{0.1cm} | ||
| + | u_{j} \cdot D\hspace{0.03cm}^{j}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}{X}(D) = U(D) \cdot G(D) | ||
| + | \hspace{0.05cm}.$$ | ||
| + | |||
| + | Hierbei wurde berücksichtigt, dass alle $u_j$ für $j < 0$ nicht existieren und zu $0$ gesetzt werden können. | ||
| + | |||
| + | Beide Vorgehensweisen zur Berechnung der Ausgangssequenz $\underline{x}$, nämlich | ||
| + | * über die Faltung | ||
| + | * mit Hilfe der $D$–Transformation, | ||
| + | |||
| + | |||
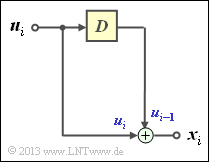
| + | sollen für das oben skizzierte Digitale Filter demonstriert werden. | ||
| + | |||
| + | ''Hinweis:'' | ||
| + | * Die Aufgabe bezieht sich auf den Lehrstoff von [[Kanalcodierung/Algebraische_und_polynomische_Beschreibung#GF.282.29.E2.80.93Beschreibungsformen_eines_Digitalen_Filters| Seite 4]] des Kapitels Algebraische und polynomische Beschreibung. | ||
| + | * Berücksichtigen Sie bei der Lösung die folgende Identität für Berechnungen in GF(2): | ||
| + | :$$1 + D + D^2 + D^3 + \hspace{0.05cm}... \hspace{0.1cm}= \frac{1}{1+D} \hspace{0.05cm}.$$ | ||
| − | |||
===Fragebogen=== | ===Fragebogen=== | ||
| − | |||
<quiz display=simple> | <quiz display=simple> | ||
| − | {Multiple-Choice | + | {Multiple-Choice |
|type="[]"} | |type="[]"} | ||
| − | + | + correct | |
| − | + | + | - false |
| − | |||
{Input-Box Frage | {Input-Box Frage | ||
|type="{}"} | |type="{}"} | ||
| − | $\ | + | $xyz \ = \ ${ 5.4 3% } $ab$ |
| − | |||
| − | |||
| − | |||
</quiz> | </quiz> | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' |
| − | '''2 | + | '''(2)''' |
| − | '''3 | + | '''(3)''' |
| − | '''4 | + | '''(4)''' |
| − | '''5 | + | '''(5)''' |
| − | |||
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | + | [[Category:Aufgaben zu Kanalcodierung|^3.2 Algebraische und polynomische Beschreibung^]] | |
| − | [[Category:Aufgaben zu Kanalcodierung|^3.2 Algebraische und polynomische Beschreibung | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ^]] | ||
Revision as of 21:45, 29 November 2017
In dieser Aufgabe beschreiben wir an einem einfachen Beispiel
- die endliche Impulsantwort eines Filters:
- $$\underline{g} = \left (g_0, g_1, \hspace{0.05cm}...\hspace{0.1cm}, g_l, \hspace{0.05cm}...\hspace{0.1cm}, g_m \right ) \hspace{0.05cm},\hspace{0.2cm}g_l \in {\rm GF(2) } = \{ 0, 1 \}\hspace{0.05cm}, $$
- die Eingangssequenz des Filters:
- $$\underline{u} = \left (u_0, u_1, \hspace{0.05cm}...\hspace{0.1cm}, u_i, \hspace{0.05cm}...\hspace{0.1cm} \right ) \hspace{0.05cm},\hspace{0.2cm}u_i \in {\rm GF(2) } = \{ 0, 1 \}\hspace{0.05cm}, $$
- die Ausgangssequenz des Filters:
- $$\underline{x} = \left (x_0, x_1, \hspace{0.05cm}...\hspace{0.1cm}, x_i, \hspace{0.05cm}...\hspace{0.1cm} \right ) \hspace{0.05cm},\hspace{0.2cm}x_i \in {\rm GF(2) } = \{ 0, 1 \}\hspace{0.05cm}. $$
Die Nomenklatur für diese (digitale) Filterbeschreibung haben wir an das Buch „Einführung in die Kanalcodierung” angepasst. In anderen Büchern bezeichnet oft $\underline{x}$ den Filtereingang, $\underline{y}$ den Filterausgang, und die Impulsantwort wird $\h$ genannt.
Allgemein gilt für die Ausgangssequenz entsprechend der Faltung (englisch: Convolution):
- $$\underline{x} = \underline{u}* \underline{g} = \left (x_0, x_1, \hspace{0.05cm}...\hspace{0.1cm}, x_i, \hspace{0.05cm}...\hspace{0.1cm} \right )\hspace{0.05cm},\hspace{0.1cm} {\rm mit} \hspace{0.2cm} x_i = \sum_{l = 0}^{m} g_l \cdot u_{i-l}\hspace{0.05cm}.$$
Wir repräsentieren nun die Zeitfunktionen $\underline{g}, \ \underline{u}$ und $\underline{x}$ durch Polynome in einer Dummy–Variablen $D$ und nennen diese die $D$–Transformierten:
- $$\underline{g} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} {G}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{l = 0}^{m} g_l \cdot D\hspace{0.03cm}^l = g_0 + g_1 \cdot D + g_2 \cdot D^2 + ... + g_m \cdot D\hspace{0.03cm}^m\hspace{0.05cm},$$
- $$\underline{u} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} {U}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{i = 0}^{\infty} u_i \cdot D\hspace{0.03cm}^i = u_0 + u_1 \cdot D + u_2 \cdot D^2 + ... \hspace{0.05cm},$$
- $$\underline{x} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} {X}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{i = 0}^{\infty} x_i \cdot D\hspace{0.03cm}^i = x_0 + x_1 \cdot D + x_2 \cdot D^2 + ... \hspace{0.05cm}.$$
Damit wird aus der (komplizierteren) Faltung eine Multiplikation:
- $$\underline{x} = \underline{u}* \underline{g} \hspace{0.25cm} \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\hspace{0.25cm} {X}(D) = U(D) \cdot G(D) \hspace{0.05cm}.$$
Formal lässt sich dieser Zusammenhang wie folgt nachweisen:
- $${X}(D) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \sum_{i = 0}^{\infty} x_i \cdot D\hspace{0.03cm}^i = \sum_{i = 0}^{\infty} \sum_{l = 0}^{m}\hspace{0.1cm} g_l \cdot u_{i-l} \cdot D\hspace{0.03cm}^{i} = \sum_{l = 0}^{m} \hspace{0.1cm} g_l \cdot \sum_{j = -l}^{\infty} \hspace{0.1cm} u_{j} \cdot D\hspace{0.03cm}^{j+l} = $$
- $$ \ = \ \hspace{-0.15cm} \sum_{l = 0}^{m} \hspace{0.1cm} g_l \cdot D\hspace{0.03cm}^l \hspace{0.1cm} \cdot \hspace{0.1cm} \sum_{j = 0}^{\infty} \hspace{0.1cm} u_{j} \cdot D\hspace{0.03cm}^{j}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}{X}(D) = U(D) \cdot G(D) \hspace{0.05cm}.$$
Hierbei wurde berücksichtigt, dass alle $u_j$ für $j < 0$ nicht existieren und zu $0$ gesetzt werden können.
Beide Vorgehensweisen zur Berechnung der Ausgangssequenz $\underline{x}$, nämlich
- über die Faltung
- mit Hilfe der $D$–Transformation,
sollen für das oben skizzierte Digitale Filter demonstriert werden.
Hinweis:
- Die Aufgabe bezieht sich auf den Lehrstoff von Seite 4 des Kapitels Algebraische und polynomische Beschreibung.
- Berücksichtigen Sie bei der Lösung die folgende Identität für Berechnungen in GF(2):
- $$1 + D + D^2 + D^3 + \hspace{0.05cm}... \hspace{0.1cm}= \frac{1}{1+D} \hspace{0.05cm}.$$
Fragebogen
Musterlösung