Aufgaben:Exercise 1.07Z: Classification of Block Codes

• Return to book

Betrachtete Blockcodes der Länge n = 4

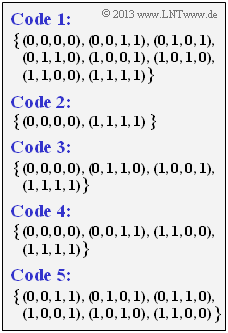
Wir betrachten Blockcodes der Länge $n = 4$:

• den Single Parity–check Code SPC (4, 3)   ⇒   „Code 1” mit

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$

• den Wiederholungscode RC (4, 1)   ⇒   „Code 2” mit der Prüfmatrix

$${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$

• den (4, 2)–Blockcode   ⇒   „Code 3” mit der Generatormatrix

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},$$

• den (4, 2)–Blockcode   ⇒   „Code 4” mit der Generatormatrix

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &0 &0\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$

• einen weiteren „Code 5” mit dem Codeumfang $|C| = 6$.

In der Grafik sind die einzelnen Codes explizit angegegeben.

Bei den Fragen zu diesen Aufgaben geht es um die Begriffe

• lineare Codes,

• systematische Codes,

• duale Codes.

Hinweise :

• Die Aufgabe gehört zum Kapitel Allgemeine Beschreibung linearer Blockcodes.
• Bezug genommen wird aber auchauf die Seiten Single Parity&nddash;Codes sowie Wiederholungscodes.

Fragebogen

1

Wie lässt sich „Code 5” beschreiben?

	In jedem Codewort sind genau zwei Nullen enthalten.
	In jedem Codewort sind genau zwei Einsen enthalten.
	Nach jeder $0$ sind die Symbole $0$ und $1$ gleichwahrscheinlich.

2

Welche der folgenden Blockcodes sind linear?

	Code 1,
	Code 2,
	Code 3,
	Code 4,
	Code 5.

3

Welche der folgenden Blockcodes sind systematisch?

	Code 1,
	Code 2,
	Code 3,
	Code 4,
	Code 5.

4

Welche Codepaare sind zueinander dual?

	Code 1 und Code 2,
	Code 2 und Code 3,
	Code 3 und Code 4.

Musterlösung

Solution

(1)  Richtig sind die Aussagen 1 und 2:

• Deshalb gibt es auch $\rm 4 \ über \ 2 = 6$ Codeworte.
• Aussage 3 ist falsch. Ist zum Beispiel das erste Bit $0$, so gibt es ein Codewort mit dem Beginn $00$ und zwei Codeworte, die mit $01$ beginnen.

(2)  Richtig sind die Aussagen 1 bis 4:

• Alle Codes, die durch eine Generatormatrix $\boldsymbol {\rm G}$ und/oder eine Prüfmatrix $\boldsymbol {\rm H}$ beschrieben werden können, sind linear.
• Dagegen erfüllt „Code 5” keine der für lineare Codes erforderlichen Bedingungen. Beispielsweise

• fehlt das Nullwort,
• ist der Codeumfang $|\mathcal{C}|$ keine Zweierpotenz,
• ergibt $(0, 1, 0, 1) \oplus (1, 0, 1, 0) = (1, 1, 1, 1)$ kein gültiges Codewort.

(3)  Richtig sind die Aussagen 1 bis 3:

• Bei einem systematischen Code müssen stets die ersten $k$ Bit eines jeden Codewortes $\underline{x}$ gleich dem Informationswort $\underline{u}$ sein.
• Dies wird erreicht, wenn der Beginn der Generatormatrix $\boldsymbol {\rm G}$ eine Einheitsmatrix $\boldsymbol{\rm I}_{k}$ darstellt.
• Dies trifft für „Code 1” (mit Dimension $k = 3$), „Code 2” (mit $k = 1$) und „Code 3” (mit $k = 2$) zu.
• Die Generatormatrix von „Code 2” ist allerdings nicht explizit angegeben. Sie lautet:

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$

(4)  Richtig ist die Aussage 1:

• Von dualen Codes spricht man, wenn die Prüfmatrix $\boldsymbol {\rm H}$ des einen Codes gleich der Generatormatrix $\boldsymbol {\rm G}$ des anderen Codes ist. Dies trifft zum Beispiel für „Code 1” und „Code 2” zu. Für den SPC (4, 3) gilt:

$${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm} { \boldsymbol{\rm G}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$

und für den Wiederholungscode RC (4, 1):

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm} { \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &0 &1\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$

• Aussage 2 ist mit Sicherheit falsch, schon aus Dimensionsgründen: Die Generatormatrix $\boldsymbol {\rm G}$ von „Code 3” ist eine $2×4$–Matrix und die Prüfmatrix $\boldsymbol {\rm H}$ von „Code 2” eine $3×4$–Matrix.

• „Code 3” und „Code 4” erfüllen ebenfalls nicht die Bedingungen dualer Codes. Die Prüfgleichungen von

$${\rm Code}\hspace{0.15cm}3 = \{ (0, 0, 0, 0) \hspace{0.05cm},\hspace{0.1cm} (0, 1, 1, 0) \hspace{0.05cm},\hspace{0.1cm}(1, 0, 0, 1) \hspace{0.05cm},\hspace{0.1cm}(1, 1, 1, 1) \}$$

lauten:

$$x_1 \oplus x_4 = 0\hspace{0.05cm},\hspace{0.2cm}x_2 \oplus x_3 = 0 \hspace{0.3cm} \Rightarrow\hspace{0.3cm} { \boldsymbol{\rm H}} = \begin{pmatrix} 1 &0 &0 &1\\ 0 &1 &1 &0 \end{pmatrix} \hspace{0.05cm}.$$

Dagegen ist die Generatormatrix von „Code 4” wie folgt gegeben:

$${ \boldsymbol{\rm G}} = \begin{pmatrix} 1 &1 &0 &0\\ 0 &0 &1 &1 \end{pmatrix} \hspace{0.05cm}.$$