Difference between revisions of "Aufgaben:Exercise 1.3Z: Rayleigh Fading Revisited"
m (Javier verschob die Seite Exercises:Exercise 1.4: Rayleigh Fading Revisited nach Exercises:Exercise 1.3Z: Rayleigh Fading Revisited) |
|||
| Line 2: | Line 2: | ||
[[File:P_ID2107__Mob_Z_1_3.png|right|frame|Zwei Kanäle, gekennzeichnet durch komplexen Faktor $z(t)$]] | [[File:P_ID2107__Mob_Z_1_3.png|right|frame|Zwei Kanäle, gekennzeichnet durch komplexen Faktor $z(t)$]] | ||
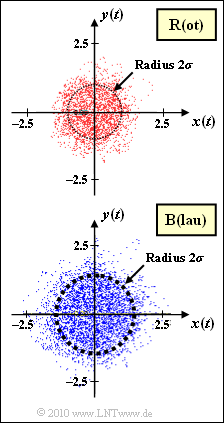
| − | + | The graph shows the multiplicative factor $z(t) = x(t) + {\rm j} \cdot y(t)$ two mobile radio channels (both without multipath propagation) in 2D–representation. The default is saved: | |
| − | * | + | * The channel $\rm R$ (the designation results from the color „Red” the point cloud) is rayleigh distributed with $\sigma_{\rm R} = 0.5$. |
| − | * | + | * For the probability density function (WDF) of amount $a(t) = |z(t)|$ or amount square $p(t) = |z(t)|^2$ the following equations $($with $\sigma = \sigma_{\rm R})$ thus apply: |
:$$f_a(a) = | :$$f_a(a) = | ||
| − | \left\{ \begin{array}{c} a/\sigma^2 \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} \\ | + | \left\{ \begin{array}{c} a/\sigma^2 \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} \\\ |
| − | 0 | + | 0 \end{array} \right.\quad |
| − | \begin{array}{*{1}c} {\rm f\ddot{u}r}\hspace{0.15cm} a \ge 0 | + | \begin{array}{*{*{1}c} {\rm f\ddot{u}r}\hspace{0.15cm} a \ge 0 |
| − | \\ | + | \\ {\rm f\ddot{u}r}\hspace{0.15cm} a < 0 \\\ \\ \end{array} |
\hspace{0.05cm},$$ | \hspace{0.05cm},$$ | ||
:$$f_p(p) = | :$$f_p(p) = | ||
| − | \left\{ \begin{array}{c} 1/(2\sigma^2) \cdot {\rm e}^{ -{p}/(2\sigma^2)} \\ | + | \left\{ \begin{array}{c} 1/(2\sigma^2) \cdot {\rm e}^{ -{p}/(2\sigma^2)} \\\ |
| − | 0 | + | 0 \end{array} \right.\quad |
| − | \begin{array}{*{1}c} {\rm f\ddot{u}r}\hspace{0.15cm} p \ge 0 | + | \begin{array}{*{*{1}c} {\rm f\ddot{u}r}\hspace{0.15cm} p \ge 0 |
| − | \\ | + | \\ {\rm f\ddot{u}r}\hspace{0.15cm} p < 0 \\\ \\ \end{array} |
.$$ | .$$ | ||
| − | * | + | * From channel $\rm B$ ("Blue") only the point cloud is given. It must be estimated whether <i>Rayleigh–Fading</i> is also present here, and if YES, how large the characteristic $\sigma = \sigma_{\rm B}$ is for this channel. |
| − | * | + | * Finally, the subtask '''(3)'' also refers to WDF $f_{\it \phi}(\phi)$ the phase function $\phi(t)$ . This is defined as follows: |
| − | + | $$\phi(t) = \arctan \hspace{0.15cm} \frac{y(t)}{x(t)} | |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| Line 28: | Line 28: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | * The task belongs to chapter [[Mobile_Communications/Probability Density_of_Rayleigh%E2%80%93Fadings|Probability Density of Rayleigh–Fadings]] of this book. |
| − | * | + | * A similar topic is treated with a different approach in chapter [[Stochastic_Signal Theory/Weitere_Verteilungen|Weitere Verteilungen]] of the book „Stochastic Signal Theory”. |
| − | * | + | * To check your results you can use the interactive applet [[Applets:WDF_VTF|WDF, VTF and Moments]] of the book „Stochastic Signal Theory”. |
| − | === | + | ===Questionnaire=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | + | Can the channel $\rm B$ also be modeled by „<i>Rayleigh</i>”? | |
|type="()"} | |type="()"} | ||
| − | + | + | + Yes. |
| − | - | + | - No. |
| − | + | Estimate the Rayleigh&ndash parameter of channel $\rm B$ . Reminder: For channel $\rm R$ this parameter has the value $\sigma_{\rm R} = 0.5$. | |
|type="{}"} | |type="{}"} | ||
$\sigma_{\rm B}\ = \ $ { 0.707 3% } | $\sigma_{\rm B}\ = \ $ { 0.707 3% } | ||
| − | { | + | {Do the phases–probability density functions $f_{\it \phi}(\phi)$ differ from channel $\rm R$ and $\rm B$ and if YES, how? |
|type="()"} | |type="()"} | ||
| − | - | + | - Yeah. |
| − | + | + | + No. |
| − | { | + | {What is the course of WDF $f_a(a)$ with $a(t) = |z(t)|$ in both cases? |
|type="()"} | |type="()"} | ||
| − | - | + | - The amount $a(t)$ is gaussian distributed. |
| − | + | + | + The amount $a(t)$ is rayleigh distributed. |
| − | - | + | - The amount $a(t)$ is positive–exponentially distributed. |
| − | { | + | {What is the course of the WDF $f_p(p)$ in both cases with $p(t) = |z(t)|^2$? |
|type="()"} | |type="()"} | ||
| − | - | + | - The amount $p(t)$ is gaussian distributed. |
| − | - | + | - The amount $p(t)$ is rayleigh distributed. |
| − | + | + | + The amount $p(t)$ is positive–exponentially distributed. |
| − | { | + | {What is the probability that the amount $a(t) = |z(t)|$ is greater than a given value $A$? |
|type="()"} | |type="()"} | ||
| − | - | + | - It applies ${\rm Pr}(|z(t)|) > A) = {\rm ln}(A/\sigma).$ |
| − | + | + | + The following applies ${\rm Pr}(|z(t)|) > A) = {\rm e}^{-A^2/2\sigma^2}.$ |
| − | - | + | - It applies ${\rm Pr}(|z(t)|) > A) = {\rm e}^{-A/\sigma}.$ |
| − | { | + | {Calculate the probability ${\rm Pr}(|z(t)| > 1)$ for both channels. |
|type="{}"} | |type="{}"} | ||
| − | + | Channel $\rm R$:$\hspace{0.4cm} {\rm Pr}(|z(t)| > 1)\ = \ $ { 0.135 3% } | |
| − | + | Channel $\rm B$:$\hspace{0.4cm} {\rm Pr}(|z(t)| > 1)\ = \ $ { 0.368 3% } | |
| − | </quiz | + | </quiz |
| − | === | + | ===Sample solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' <u> | + | '''(1)''' <u>correct is YES</u>: |
| − | * | + | *You can see the rotational symmetry here, too, if you consider that only $N = 10\hspace{0.05cm}000$ samples were displayed in the complex plane. |
| − | * | + | *Furthermore the following questions would not make sense if you answer NO. |
| − | '''(2)''' | + | '''(2)''' By measuring the two drawn circles you can see that for the „blue” channel the scattering of real– and imaginary part are larger by a factor of about 1.4 (exactly: $\sqrt{2}$) than for the „red” channel: |
| − | + | $$\sigma_{\rm B} = \sigma_{\rm R} \cdot \sqrt{2} = 0.5 \cdot \sqrt{2}= {1}/{\sqrt{2}}\hspace{0.15cm} \underline{\approx 0.707} | |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | '''(3)''' <u> | + | '''(3)''' <u>correct is NO</u>: |
| − | *In | + | *In both cases $f_{\it \phi}(\phi)$ describes an equal distribution between $-\pi$ and $+\pi$. |
| − | * | + | *The larger amplitudes of channel $\rm B$ are not important for the phase function $\phi(t)$. |
| − | '''(4)''' | + | '''(4)''' Correct is the <u>solution 2</u>: |
| − | * | + | *With Rayleigh–Fading, the real part $x(t)$ and the imaginary part $y(t)$ are each Gaussian distributed. |
| − | * | + | *The exponential distribution results for the square of the absolute value $p(t) = |z(t)|^2$. |
| − | '''(5)''' | + | '''(5)''' Correct here is the <u>solution 3</u>, as already explained in the sample solution for '''(4)'''. |
| − | '''(6)''' | + | '''(6)''' The amount $a(t)$ is rayleigh distributed. Therefore, the following applies for the searched probability: |
| − | + | $${\rm Pr}(a > A) = \int_{A}^{\infty}\frac{a}{\sigma^2} \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} \hspace{0.15cm}{\rm d}a \hspace{0.05cm}.$ | |
| − | *In | + | *In some formula collections you can find the solution for this integral, but not in all. |
| − | * | + | *But it is also valid with the one-sided–exponentially distributed random variable $p = a^2$: |
| − | + | $${\rm Pr}(a > A) = {\rm Pr}(p > A^2) = \frac{1}{2\sigma^2} \cdot\int_{A^2}^{\infty} {\rm e}^{ -{p}/(2\sigma^2)} \hspace{0.15cm}{\rm d}p \hspace{0.05cm}.$$ | |
| − | * | + | *This integral is elementary and gives the result: |
| − | + | $${\rm Pr}(a > A) = {\rm e}^{ -{A^2}/(2\sigma^2)} \hspace{0.05cm}.$$ | |
| − | + | Correct is therefore the <u>solution 2</u>. | |
| − | '''(7)''' | + | '''(7)''' For the channel $\rm R$ applies with $\sigma = 0.5$: |
| − | + | $${\rm Pr}(|z(t)| > 1) = {\rm e}^{-2} \hspace{0.15cm} \underline{\approx 0.135} | |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | *In | + | *In the upper graph this corresponds to the number of all points outside the circle drawn, based on the number $N = $10,000$ of all points. |
| − | * | + | *For the channel $\rm B$ because of the double variance $\sigma^2 = 0.5$, ${\rm Pr}(|z(t)|>1) = {\rm e}^{\rm –1} applies. \ \underline {\approx \ 0.368}$. |
| − | * | + | *The (not drawn) reference circle would also have the radius 1 in the lower graph. |
| − | * | + | *The circle drawn in the lower graphic has a larger radius than $A = $1, namely $A = \sqrt{2}\approx $1,414. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
[[Category:Exercises for Mobile Communications|^1.2 PDF of Rayleigh Fading^]] | [[Category:Exercises for Mobile Communications|^1.2 PDF of Rayleigh Fading^]] | ||
Revision as of 18:54, 25 March 2020
The graph shows the multiplicative factor $z(t) = x(t) + {\rm j} \cdot y(t)$ two mobile radio channels (both without multipath propagation) in 2D–representation. The default is saved:
- The channel $\rm R$ (the designation results from the color „Red” the point cloud) is rayleigh distributed with $\sigma_{\rm R} = 0.5$.
- For the probability density function (WDF) of amount $a(t) = |z(t)|$ or amount square $p(t) = |z(t)|^2$ the following equations $($with $\sigma = \sigma_{\rm R})$ thus apply:
- $$f_a(a) = \left\{ \begin{array}{c} a/\sigma^2 \cdot {\rm e}^{ -{a^2}/(2\sigma^2)} \\\ 0 \end{array} \right.\quad \begin{array}{*{*{1}c} {\rm f\ddot{u}r}\hspace{0.15cm} a \ge 0 \\ {\rm f\ddot{u}r}\hspace{0.15cm} a < 0 \\\ \\ \end{array} \hspace{0.05cm},$$
- $$f_p(p) = \left\{ \begin{array}{c} 1/(2\sigma^2) \cdot {\rm e}^{ -{p}/(2\sigma^2)} \\\ 0 \end{array} \right.\quad \begin{array}{*{*{1}c} {\rm f\ddot{u}r}\hspace{0.15cm} p \ge 0 \\ {\rm f\ddot{u}r}\hspace{0.15cm} p < 0 \\\ \\ \end{array} .$$
- From channel $\rm B$ ("Blue") only the point cloud is given. It must be estimated whether Rayleigh–Fading is also present here, and if YES, how large the characteristic $\sigma = \sigma_{\rm B}$ is for this channel.
- Finally, the subtask '(3) also refers to WDF $f_{\it \phi}(\phi)$ the phase function $\phi(t)$ . This is defined as follows:
$$\phi(t) = \arctan \hspace{0.15cm} \frac{y(t)}{x(t)} \hspace{0.05cm}.$$
Notes:
- The task belongs to chapter Probability Density of Rayleigh–Fadings of this book.
- A similar topic is treated with a different approach in chapter Weitere Verteilungen of the book „Stochastic Signal Theory”.
- To check your results you can use the interactive applet WDF, VTF and Moments of the book „Stochastic Signal Theory”.
Questionnaire
<quiz display=simple> Can the channel $\rm B$ also be modeled by „Rayleigh”? |type="()"} + Yes. - No.
Estimate the Rayleigh&ndash parameter of channel $\rm B$ . Reminder: For channel $\rm R$ this parameter has the value $\sigma_{\rm R} = 0.5$. |type="{}"} $\sigma_{\rm B}\ = \ $ { 0.707 3% }
{Do the phases–probability density functions $f_{\it \phi}(\phi)$ differ from channel $\rm R$ and $\rm B$ and if YES, how? |type="()"} - Yeah. + No.
{What is the course of WDF $f_a(a)$ with $a(t) = |z(t)|$ in both cases? |type="()"} - The amount $a(t)$ is gaussian distributed. + The amount $a(t)$ is rayleigh distributed. - The amount $a(t)$ is positive–exponentially distributed.
{What is the course of the WDF $f_p(p)$ in both cases with $p(t) = |z(t)|^2$? |type="()"} - The amount $p(t)$ is gaussian distributed. - The amount $p(t)$ is rayleigh distributed. + The amount $p(t)$ is positive–exponentially distributed.
{What is the probability that the amount $a(t) = |z(t)|$ is greater than a given value $A$? |type="()"} - It applies ${\rm Pr}(|z(t)|) > A) = {\rm ln}(A/\sigma).$ + The following applies ${\rm Pr}(|z(t)|) > A) = {\rm e}^{-A^2/2\sigma^2}.$ - It applies ${\rm Pr}(|z(t)|) > A) = {\rm e}^{-A/\sigma}.$
{Calculate the probability ${\rm Pr}(|z(t)| > 1)$ for both channels. |type="{}"} Channel $\rm R$:$\hspace{0.4cm} {\rm Pr}(|z(t)| > 1)\ = \ $ { 0.135 3% } Channel $\rm B$:$\hspace{0.4cm} {\rm Pr}(|z(t)| > 1)\ = \ $ { 0.368 3% } </quiz ==='"`UNIQ--h-1--QINU`"'Sample solution=== '"`UNIQ--html-00000002-QINU`"' '''(1)''' <u>correct is YES</u>: *You can see the rotational symmetry here, too, if you consider that only $N = 10\hspace{0.05cm}000$ samples were displayed in the complex plane. *Furthermore the following questions would not make sense if you answer NO. '''(2)''' By measuring the two drawn circles you can see that for the „blue” channel the scattering of real– and imaginary part are larger by a factor of about 1.4 (exactly: $\sqrt{2}$) than for the „red” channel: '"`UNIQ-MathJax4-QINU`"' '''(3)''' <u>correct is NO</u>: *In both cases $f_{\it \phi}(\phi)$ describes an equal distribution between $-\pi$ and $+\pi$. *The larger amplitudes of channel $\rm B$ are not important for the phase function $\phi(t)$. '''(4)''' Correct is the <u>solution 2</u>: *With Rayleigh–Fading, the real part $x(t)$ and the imaginary part $y(t)$ are each Gaussian distributed. *The exponential distribution results for the square of the absolute value $p(t) = |z(t)|^2$. '''(5)''' Correct here is the <u>solution 3</u>, as already explained in the sample solution for '''(4)'''. '''(6)''' The amount $a(t)$ is rayleigh distributed. Therefore, the following applies for the searched probability: '"`UNIQ-MathJax5-QINU`"'{\rm Pr}(a > A) = {\rm Pr}(p > A^2) = \frac{1}{2\sigma^2} \cdot\int_{A^2}^{\infty} {\rm e}^{ -{p}/(2\sigma^2)} \hspace{0.15cm}{\rm d}p \hspace{0.05cm}.'"`UNIQ-MathJax6-QINU`"'{\rm Pr}(a > A) = {\rm e}^{ -{A^2}/(2\sigma^2)} \hspace{0.05cm}.'"`UNIQ-MathJax7-QINU`"'{\rm Pr}(|z(t)| > 1) = {\rm e}^{-2} \hspace{0.15cm} \underline{\approx 0.135} \hspace{0.05cm}.$$ *In the upper graph this corresponds to the number of all points outside the circle drawn, based on the number $N = $10,000$ of all points.
- For the channel $\rm B$ because of the double variance $\sigma^2 = 0.5$, ${\rm Pr}(|z(t)|>1) = {\rm e}^{\rm –1} applies. \ \underline {\approx \ 0.368}$.
- The (not drawn) reference circle would also have the radius 1 in the lower graph.
- The circle drawn in the lower graphic has a larger radius than $A = $1, namely $A = \sqrt{2}\approx $1,414.