Aufgaben:Exercise 2.2: DC Component of Signals

• Return to book

Rechtecksignale mit und ohne Gleichanteil

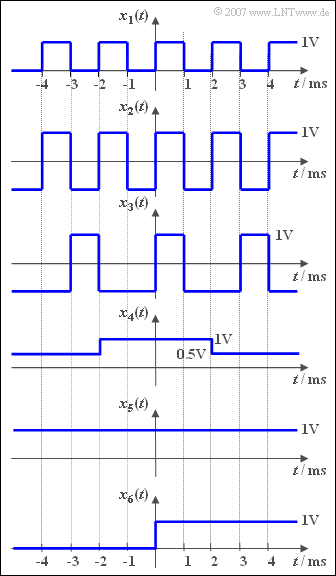
The graph shows some time signals defined for all times $($from  $-\infty$  to  $+\infty)$ . For all six sample signals  $x_i(t)$  the associated spectral function can be written as:

$$X_i(f)=A_0\cdot{\rm \delta}(f)+\Delta X_i(f).$$

Here

• $A_0$  is the DC component, and
• $\Delta X_i(f)$  is the spectrum of the residual signal reduced by the DC component  $\Delta x_i(t) = x_i(t) - A_0$.

Hint:

• This exercise belongs to the chapter  Direct Current Signal - Limit Case of a Periodic Signal.

Questions

1 Which of the signals contains a DC component, i.e. for which signals is   $A_0 \neq 0$?

	Signal  $x_1(t),$
	signal  $x_2(t),$
	signal  $x_3(t),$
	signal  $x_4(t),$
	signal  $x_5(t),$
	signal  $x_6(t).$

2 For which of the signals is the „residual spectrum”  $\Delta X_i(f) =0$?

	Signal  $x_1(t),$
	signal  $x_2(t),$
	signal  $x_3(t),$
	signal  $x_4(t),$
	signal  $x_5(t),$
	signal  $x_6(t).$

3 What is the DC component of the signal  $x_3(t)$?

$x_3(t)\hspace{-0.1cm}:\,\,A_0 \ = \ $   ${\rm V}$

4 What is the DC component of the signal  $x_4(t)$?

$x_4(t)\hspace{-0.1cm}:\,\,A_0\ = \ $   ${\rm V}$

5 What is the DC component of the signal  $x_6(t)$?

$x_6(t)\hspace{-0.1cm}:\,\,A_0\ = \ $   ${\rm V}$

Solution

Solution

(1)  The correct answers are 1, 3, 4, 5 and 6.

• All signals except  $x_2(t)$  contain a DC signal component.

(2)  Only solution 5 is correct:

• If the DC component   $1\text{V}$ is subtracted from the signal   $x_5(t)$ , the residual signal  $\Delta x_5(t) = x5(t) - 1\text{V}$  is equal to zero.
• Accordignly, the spectral function is  $\Delta X_5(f) = 0$.
• For all other time courses  $\Delta x_i(t)$  is not equal to zero and thus the associated spectral function   $\Delta X_i(f)$ is also not equal to zero.

(3)  With a periodic signal, averaging over a period duration is sufficient to calculate the DC signal component  $A_0$ .

• Beim Beispielsignal  $x_3(t)$  ist diese  $T_0 = 3\,\text{ms}$. Damit ergibt sich der gesuchte Gleichanteil zu

$$A_0=\rm \frac{1}{3\,ms}\cdot \big[1\,V\cdot 1\,ms+(-1\,V)\cdot 2\,ms \big]

\hspace{0.15cm}\underline{=-0.333\,V}.$$

(4)  Für das Signal  $x_4(t)$  kann geschrieben werden:  $x_4(t) = 0.5 \,{\rm V} + Δx_4(t)$.

• Hierbei bezeichnet  $Δx_4(t)$  einen Rechteckimpuls mit Amplitude  $0.5 \,{\rm V} $  und Dauer  $4 \,{\rm ms} $, der wegen seiner endlichen Dauer nicht zum Gleichsignalanteil beiträgt.
• Deshalb gilt hier  $A_0 \hspace{0.15cm}\underline{=0.5 \,{\rm V}}$.

(5)  Die allgemeine Gleichung zur Berechnung des Gleichsignalanteils lautet:

$$A_0=\lim_{T_{\rm M}\to \infty}\frac{1}{T_{\rm M}}\int_{-T_{\rm M}/2}^{+T_{\rm M}/2}x(t)\, {\rm d }t.$$

• Spaltet man dieses Integral in zwei Teilintegrale auf, so erhält man:

$$A_0=\lim_{T_{\rm M}\to \infty}\frac{1}{T_{\rm M}}\int _{-T_{\rm M}/2}^{0}0 {\rm V} \cdot\, {\rm d } {\it t }+\lim_{T_{\rm M}\to \infty}\frac{1}{T_{\rm M}}\int _{0}^{+T_{\rm M}/2}1 \rm V \ {\rm d }{\it t }.$$

• Nur der zweite Term liefert einen Beitrag. Daraus folgt wiederum  $A_0 \hspace{0.15cm}\underline{=0.5 \,{\rm V}}$.