Difference between revisions of "Aufgaben:Exercise 3.3Z: Rectangular Pulse and Dirac Delta"
From LNTwww
| Line 20: | Line 20: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Signal_Representation/Special_Cases_of_Impulse_Signals|Special Cases of Impulse Signals]]. |
| − | * | + | *You can check your results using the two interactive applets [[Applets:Impulse_und_Spektren|Impulse und Spektren]] sowie [[Applets:Frequenzgang_und_Impulsantwort|Frequenzgang und Impulsantwort]] . |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements are true regarding the spectrum $X_1(f)$ ? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The spectral value $X_1(f = 0)$ is equal to $10^{–3} \,\text{V/Hz}$. |
| − | + $X_1(f)$ | + | + $X_1(f)$ has zeros at a distance of $2 \,\text{kHz}$. |
| − | - $X_1(f)$ | + | - $X_1(f)$ has zeros at a distance of $4 \,\text{kHz}$. |
| − | { | + | {Which of the following statements are true regarding the spectrum $X_2(f)$ zu? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The spectral value $X_2(f = 0)$ ist gleich $10^{–3} \,\text{V/Hz}$. |
| − | - $X_2(f)$ | + | - $X_2(f)$ has zeros at a distance of $2\, \text{kHz}$. |
| − | + $X_2(f)$ | + | + $X_2(f)$ has zeros at a distance of $4 \,\text{kHz}$. |
| − | { | + | {Let $k = 10$. Calculate the frequency $f_{10}$ of the first zero and the spectral value at $f = 2 \,\text{kHz}$. |
|type="{}"} | |type="{}"} | ||
$f_{10} \ = \ ${ 20 3% } $\text{kHz}$ | $f_{10} \ = \ ${ 20 3% } $\text{kHz}$ | ||
| Line 51: | Line 51: | ||
| − | { | + | {What is the spectral value at bei $f = 2 \,\text{kHz}$ in the limiting case $k \rightarrow \infty$? Interpret the result. |
|type="{}"} | |type="{}"} | ||
$X_{\infty}(f = 2 \,\text{kHz})\ = \ $ { 1 3% } $\text{mV/Hz}$ | $X_{\infty}(f = 2 \,\text{kHz})\ = \ $ { 1 3% } $\text{mV/Hz}$ | ||
Revision as of 18:46, 23 January 2021
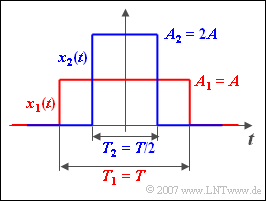
We consider here a multitude of symmetrical rectangular functions $x_k(t)$. The individual rectangles differ in amplitudes (heights)
- $$A_k = k \cdot A$$
and different pulse durations (widths)
- $$T_k = T/k.$$
Let $k$ be any positive value.
- The rectangular pulse $x_1(t)$ shown in red has the amplitude $A_1 = {A} = 2 \,\text{V}$ and the duration $T_1 = {T} = 500 \,µ\text{s}$.
- The pulse $x_2(t)$ shown in blue is half as wide ⇒ $T_2 =250 \,µ\text{s}$, but twice as high ⇒ $A_2 = 4 \text{ V}$.
Hints:
- This exercise belongs to the chapter Special Cases of Impulse Signals.
- You can check your results using the two interactive applets Impulse und Spektren sowie Frequenzgang und Impulsantwort .
Questions
Musterlösung
(1) Richtig sind die Lösungsvorschläge 1 und 2:
- Der Spektralwert bei der Frequenz $f = 0$ ist nach dem ersten Fourierintegral stets gleich der Fläche unter der Zeitfunktion:
- $$X( f ) = \int_{ - \infty }^{ + \infty } {x( t )} \cdot {\rm{e}}^{ - {\rm{j2\pi }}ft} \hspace{0.1cm} {\rm d}t \hspace{0.5cm} \Rightarrow \hspace{0.5cm} \;X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t )}\hspace{0.1cm} {\rm d}t.$$
- Im vorliegenden Fall ist die Impulsfläche stets $A \cdot T = 10^{–3} \,\text{Vs} = 1\, \text{mV/Hz}$.
- Wegen $T_1 = 500 \,µ\text{s}$ weist das Spektrum $X_1(f)$ Nulldurchgänge im Abstand $f_1 = 1/T_1 = 2 \,\text{kHz}$ auf.
(2) Richtig sind die Lösungsvorschläge 1 und 3:
- Aufgrund gleicher Impulsflächen wird der Spektralwert bei der Frequenz $f = 0$ nicht verändert.
- Die äquidistanten Nulldurchgänge treten nun im Abstand $f_2 = 1/T_2 = 4 \,\text{kHz}$ auf.
(3) Nullstellen gibt es bei Vielfachen von $f_{10} = 1/T_{10} = 20 \,\text{kHz}$, und die Spektralfunktion lautet:
- $$X_{10} ( f ) = X_0 \cdot {\mathop{\rm si}\nolimits} ( {{\rm{\pi }}f/f_{10} } ).$$
- Bei der Frequenz $f = 2 \,\text{kHz}$ ist das Argument der $\rm si$-Funktion gleich $\pi/10$ $($oder $18^{\circ})$:
- $$X_{10} ( {f = 2\;{\rm{kHz}}}) = 10^{ - 3} \;{\rm{V/Hz}} \cdot \frac{{\sin ( {18^\circ } )}}{{{\rm{\pi /10}}}} \hspace{0.15 cm}\underline{= 0.984 \;{\rm{mV/Hz}}}{\rm{.}}$$
(4) Im Grenzfall $k \rightarrow \infty$ geht der dann unendlich hohe und unendlich schmale Rechteckimpuls in den Diracimpuls über.
- Dessen Spektrum ist für alle Frequenzen konstant.
- Damit gilt auch bei der Frequenz $f = 2 \,\text{kHz}$ der Spektralwert $X_{\infty}(f = 2 \,\text{kHz})\hspace{0.15 cm}\underline{=1 \text{ mV/Hz}}$.