Aufgaben:Exercise 3.5Z: Integration of Dirac Functions

• Return to book

Integration von Diracfunktionen

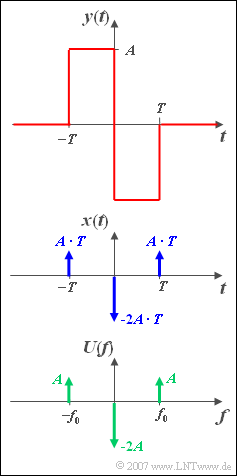
Like in  Exercise 3.5  the spectrum  ${Y(f)}$  of the signal

$$y( t ) = \left\{ \begin{array}{c} A \\ - A \\ 0 \\ \end{array} \right.\quad \begin{array}{*{20}c} {{\rm{for}}} \\ {{\rm{for}}} \\ {\rm{else.}} \\ \end{array}\;\begin{array}{*{20}c} { - T \le t < 0,} \\ {0 < t \le T,} \\ {} \\\end{array}$$

can be determined.  Again,  $A = 1 \,\text{V}$  and  $T = 0.5 \,\text{ms}$  apply.

Assume the time signal  ${x(t)}$  according to the middle sketch, which is composed of three Dirac pulses at  $–T$,  $0$  and  $+T$  with the pulse weights  ${AT}$,  $-2{AT}$  und  ${AT}$ .

The spectral function  ${X(f)}$  can be given directly by applying the  Duality Theorem  if one takes into account that the time function belonging to  ${U(f)}$  is as follows (see lower sketch):

$$u( t ) = - 2A + 2A \cdot \cos ( {2{\rm{\pi }}f_0 t} ).$$

Hints:

• This exercise belongs to the chapter  Fourier Transform Theorems.
• All the laws presented here are illustrated with examples in the (German language) learning video
       Gesetzmäßigkeiten der Fouriertransformation   ⇒   "Regularities to the Fourier transform".
• The following relationship exists between  ${x(t)}$  and  ${y(t)}$ :

$$y( t ) = \frac{1}{T} \cdot \hspace{-0.1cm} \int_{ - \infty }^{\hspace{0.05cm}t} {x( \tau )}\, {\rm d}\tau .$$

• The  Integration Theorem  reads in a correspondingly adapted form:

$$\frac{1}{T}\cdot \hspace{-0.1cm} \int_{ - \infty }^{\hspace{0.05cm}t} {x( \tau )}\,\, {\rm d}\tau\ \ \circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \ \ X( f ) \cdot \left( {\frac{1}{{{\rm{j}}\cdot 2{\rm{\pi }\cdot }fT}} + \frac{1}{2T}\cdot {\rm \delta} ( f )} \right).$$

Questions

1

Calculate the spectral function  ${X(f)}$.  What is its magnitude at the frequencies  $f = 0$  and  $f = 1\, \text{kHz}$?

$|{X(f = 0)}| \ = \ $  $\text{mV/Hz}$

$|{X(f = 1\, \text{kHz})}|\ = \ $  $\text{mV/Hz}$

2

Calculate the spectral function  ${Y(f)}$.  What values result at the frequencies  $f = 0$  and  $f = 1\, \text{kHz}$?

$|{Y(f = 0)}|\ = \ $  $\text{mV/Hz}$

$|{Y(f = 1\, \text{kHz})}| \ = \ $  $\text{mV/Hz}$

Solution

Solution

(1)  In the task description you will find the Fourier correspondence between  ${u(t)}$  and  ${U(f)}$.

• Since both the time functions  ${u(t)}$  and  ${x(t)}$  and the corresponding spectra  ${U(f)}$  and  ${X(f)}$  are even and real,  ${X(f)}$  can be easily calculated by applying the "Duality Theorem":

$$X( f ) = - 2 \cdot A \cdot T + 2 \cdot A \cdot T \cdot \cos \left( {{\rm{2\pi }}fT} \right).$$

• Because of the relation  $\sin^{2}(\alpha) = (1 – \cos(\alpha))/2$  it can also be written for this:

$$X( f ) = - 4 \cdot A \cdot T \cdot \sin ^2 ( {{\rm{\pi }}fT} ).$$

• At frequency  $f = 0$  the signal  ${x(t)}$  has no spectral components  ⇒   ${X(f = 0)} \;\underline{= 0}$.
• For  $f = 1 \,\text{kHz}$  – and also  $f \cdot T = 0.5$  –   on the other hand:

$$X( f = 1\;{\rm{kHz}} ) = - 4 \cdot A \cdot T = -2 \cdot 10^{ - 3} \;{\rm{V/Hz}}\; \Rightarrow \; |X( {f = 1\;{\rm{kHz}}} )| \hspace{0.15 cm}\underline{= 2 \;{\rm{mV/Hz}}}{\rm{.}}$$

(2)  The spectrum  ${Y(f)}$  can be determined from  ${X(f)}$  by applying the  "Integration Theorem".

• Because of  ${X(f = 0)} = 0$  the Dirac function does not have to be taken into account at the frequency  $f = 0$  and one obtains:

$$Y( f ) = \frac{X( f )}{{{\rm{j}} \cdot 2{\rm{\pi }}fT}} = \frac{{ - 4 \cdot A \cdot T \cdot \sin ^2 ( {{\rm{\pi }}fT} )}}{{{\rm{j}}\cdot 2{\rm{\pi }}fT}} = 2{\rm{j}} \cdot A \cdot T \cdot \frac{{\sin ^2 ( {{\rm{\pi }}fT} )}}{{{\rm{\pi }}fT}}.$$

• Of course, the result is the same as in  Exercise 3.5:

• At frequency  $f = 0$  The signal  ${y(t)}$  also has no spectral components  ⇒   ${Y(f = 0)} \;\underline{= 0}$.
• For  $f = 1\,\text{kHz} \ \ (f \cdot T = 0.5)$  one obtains a value smaller by a factor  $\pi$  compared to  $X(f)$:

$$|Y( {f = 1\;{\rm{kHz}}} )| = \frac{4 \cdot A \cdot T}{\rm{\pi }} \hspace{0.15 cm}\underline{= {\rm{0}}{\rm{.636}} \;{\rm{mV/Hz}}}{\rm{.}}$$