Difference between revisions of "Aufgaben:Exercise 2.3Z: Asymmetrical Characteristic Operation"
| Line 2: | Line 2: | ||
{{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/Nonlinear_Distortion}} | {{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/Nonlinear_Distortion}} | ||
| − | [[File:P_ID895__LZI_Z_2_3.png|right|frame| | + | [[File:P_ID895__LZI_Z_2_3.png|right|frame|Influence of nonlinear distortions]] |
| − | + | The cosine signal | |
:x(t)=A⋅cos(ω0t) | :x(t)=A⋅cos(ω0t) | ||
| − | + | is applied to the input of a system S where A=0.5 shall always hold for the amplitude. The system S consists of | |
| − | * | + | *the addition of a direct (DC) component C, |
| − | * | + | *a nonlinearity with the characteristic curve |
:g(x)=sin(x)≈x−x3/6=g3(x), | :g(x)=sin(x)≈x−x3/6=g3(x), | ||
| − | * | + | *as well as an ideal high-pass filter that allows all frequencies to pass unaltered except for a direct (DC) signal (f=0) . |
| − | + | The output signal of the overall system can generally be depicted as follows: | |
:$$y(t) = A_0 + A_1 \cdot \cos(\omega_0 t) + A_2 \cdot \cos(2\omega_0 t) + | :$$y(t) = A_0 + A_1 \cdot \cos(\omega_0 t) + A_2 \cdot \cos(2\omega_0 t) + | ||
A_3 \cdot \cos(3\omega_0 t) + \hspace{0.05cm}\text{...}$$ | A_3 \cdot \cos(3\omega_0 t) + \hspace{0.05cm}\text{...}$$ | ||
| − | + | The sinusoidal characteristic curve g(x) is to be approximated by the cubic approximation g3(x) throughout the whole problem according to the above equation. | |
| − | + | This would result in exactly the same constellation as in [[Aufgaben:2.3_Sinusförmige_Kennlinie|Exercise 2.3]] for C=0 in whose subtask '''(2)''' the distortion factor was calculated: | |
*K=Kg3≈1.08% für A=0.5, | *K=Kg3≈1.08% für A=0.5, | ||
*K=Kg3≈4.76% für A=1.0. | *K=Kg3≈4.76% für A=1.0. | ||
| − | + | Considering the constants A=C=0.5 the following holds for the input signal of the nonlinearity: | |
:xC(t)=C+A⋅cos(ω0t)=1/2+1/2⋅cos(ω0t). | :xC(t)=C+A⋅cos(ω0t)=1/2+1/2⋅cos(ω0t). | ||
| − | * | + | *So, the characteristic curve is operated asymmetrically with values between 0 and 1. |
| − | *In | + | *In the above graph, the signals xC(t) and yC(t) are plotted additionally directly before and after the characteristic curve g(x) . |
| Line 51: | Line 51: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Compute the output signal y(t) considering the high-pass filter. What is the direct (DC) signal component A0? |
|type="{}"} | |type="{}"} | ||
A0 = { 0. } | A0 = { 0. } | ||
| − | { | + | {State the other Fourier coefficients of the signal y(t) . |
|type="{}"} | |type="{}"} | ||
A1 = { 0.422 3% } | A1 = { 0.422 3% } | ||
| Line 64: | Line 64: | ||
| − | { | + | {Compute the distortion factor of the overall system. |
|type="{}"} | |type="{}"} | ||
K = { 7.51 3% } % | K = { 7.51 3% } % | ||
| − | { | + | {Compute the maximum and the minimum value of the signal y(t). |
|type="{}"} | |type="{}"} | ||
ymax = { 0.386 3% } | ymax = { 0.386 3% } | ||
| Line 80: | Line 80: | ||
===Solution=== | ===Solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Considering the cubic approximation g3(x) the following is obtained before the high-pass filter: |
:$$y_{\rm C}(t) = g_3\big[x_{\rm C}(t)\big] = \big[ C + A \cdot \cos(\omega_0 | :$$y_{\rm C}(t) = g_3\big[x_{\rm C}(t)\big] = \big[ C + A \cdot \cos(\omega_0 | ||
t)\big] - {1}/{6} \cdot \big[ C + A \cdot \cos(\omega_0 | t)\big] - {1}/{6} \cdot \big[ C + A \cdot \cos(\omega_0 | ||
| Line 90: | Line 90: | ||
t) + A^3 \cdot \cos^3(\omega_0 t)\big].$$ | t) + A^3 \cdot \cos^3(\omega_0 t)\big].$$ | ||
| − | * | + | *The signal yC(t) contains a direct (DC) component C−C3/6 which is no longer included in the signal y(t) due to the high-pass filter: |
:A0=0_. | :A0=0_. | ||
| − | '''(2)''' | + | '''(2)''' Applying the given trigonometric relations the following coefficients with A=C=0.5 are obtained: |
:$$A_1 = A - {1}/{6}\cdot 3 \cdot C^2 \cdot A - {1}/{6} \cdot {3}/{4}\cdot | :$$A_1 = A - {1}/{6}\cdot 3 \cdot C^2 \cdot A - {1}/{6} \cdot {3}/{4}\cdot | ||
A^3 = {1}/{2} - {1}/{16} - {1}/{64} = {27}/{64} | A^3 = {1}/{2} - {1}/{16} - {1}/{64} = {27}/{64} | ||
| Line 103: | Line 103: | ||
A^3 = - {1}/{192} \hspace{0.15cm}\underline{\approx -0.005}.$$ | A^3 = - {1}/{192} \hspace{0.15cm}\underline{\approx -0.005}.$$ | ||
| − | * | + | *Higher order terms do not occur. Thus, h A4=0_ holds. |
| − | '''(3)''' | + | '''(3)''' In this task, the higher order distortion factors are K2=2/27≈7.41% und K3=1/81≈1.23%. |
| − | * | + | *Thereby, the following is obtained for the overall distortion factor: |
:K=√K22+K23≈7.51%_. | :K=√K22+K23≈7.51%_. | ||
| − | '''(4)''' | + | '''(4)''' The maximum value occurs at time t=0 and at multiples of T : |
:$$y_{\rm max}= y(t=0) = A_1 + A_2 + A_3 = 0.422 -0.031 -0.005 \hspace{0.15cm}\underline{= | :$$y_{\rm max}= y(t=0) = A_1 + A_2 + A_3 = 0.422 -0.031 -0.005 \hspace{0.15cm}\underline{= | ||
0.386}.$$ | 0.386}.$$ | ||
| − | * | + | *The minimum values are located exactly in the middle between two maxima and it holds that: |
:$$y_{\rm min}= - A_1 + A_2 - A_3 = -0.422 -0.031 +0.005\hspace{0.15cm}\underline{ = | :$$y_{\rm min}= - A_1 + A_2 - A_3 = -0.422 -0.031 +0.005\hspace{0.15cm}\underline{ = | ||
-0.448}.$$ | -0.448}.$$ | ||
| − | * | + | *The signal y(t) is shifted downward by 0.448 compared to the signal drawn in the sketch on the information page. |
| − | * | + | *This signal value is obtained from the following equation considering A=C=1/2: |
:C−C⋅A24−C36=1/2−1/32−1/48=0.448. | :C−C⋅A24−C36=1/2−1/32−1/48=0.448. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 00:24, 15 September 2021
The cosine signal
- x(t)=A⋅cos(ω0t)
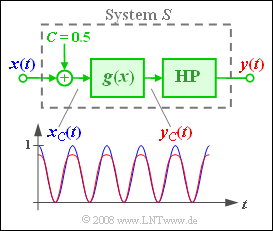
is applied to the input of a system S where A=0.5 shall always hold for the amplitude. The system S consists of
- the addition of a direct (DC) component C,
- a nonlinearity with the characteristic curve
- g(x)=sin(x)≈x−x3/6=g3(x),
- as well as an ideal high-pass filter that allows all frequencies to pass unaltered except for a direct (DC) signal (f=0) .
The output signal of the overall system can generally be depicted as follows:
- y(t)=A0+A1⋅cos(ω0t)+A2⋅cos(2ω0t)+A3⋅cos(3ω0t)+...
The sinusoidal characteristic curve g(x) is to be approximated by the cubic approximation g3(x) throughout the whole problem according to the above equation.
This would result in exactly the same constellation as in Exercise 2.3 for C=0 in whose subtask (2) the distortion factor was calculated:
- K=Kg3≈1.08% für A=0.5,
- K=Kg3≈4.76% für A=1.0.
Considering the constants A=C=0.5 the following holds for the input signal of the nonlinearity:
- xC(t)=C+A⋅cos(ω0t)=1/2+1/2⋅cos(ω0t).
- So, the characteristic curve is operated asymmetrically with values between 0 and 1.
- In the above graph, the signals xC(t) and yC(t) are plotted additionally directly before and after the characteristic curve g(x) .
Please note:
- The task belongs to the chapter Nonlinear Distortion.
- The following trigonometric relations are assumed to be known:
- cos2(α)=1/2+1/2⋅cos(2α),cos3(α)=3/4⋅cos(α)+1/4⋅cos(3α).
Questions
Solution
- yC(t)=g3[xC(t)]=[C+A⋅cos(ω0t)]−1/6⋅[C+A⋅cos(ω0t)]3
- ⇒yC(t)=C+A⋅cos(ω0t)−1/6⋅[C3+3⋅C2⋅A⋅cos(ω0t)+3⋅C⋅A2⋅cos2(ω0t)+A3⋅cos3(ω0t)].
- The signal yC(t) contains a direct (DC) component C−C3/6 which is no longer included in the signal y(t) due to the high-pass filter:
- A0=0_.
(2) Applying the given trigonometric relations the following coefficients with A=C=0.5 are obtained:
- A1=A−1/6⋅3⋅C2⋅A−1/6⋅3/4⋅A3=1/2−1/16−1/64=27/64≈0.422_,
- A2=−1/6⋅3⋅1/2⋅C⋅A2=−132≈−0.031_,
- A3=−1/6⋅14⋅A3=−1/192≈−0.005_.
- Higher order terms do not occur. Thus, h A4=0_ holds.
(3) In this task, the higher order distortion factors are K2=2/27≈7.41% und K3=1/81≈1.23%.
- Thereby, the following is obtained for the overall distortion factor:
- K=√K22+K23≈7.51%_.
(4) The maximum value occurs at time t=0 and at multiples of T :
- ymax=y(t=0)=A1+A2+A3=0.422−0.031−0.005=0.386_.
- The minimum values are located exactly in the middle between two maxima and it holds that:
- ymin=−A1+A2−A3=−0.422−0.031+0.005=−0.448_.
- The signal y(t) is shifted downward by 0.448 compared to the signal drawn in the sketch on the information page.
- This signal value is obtained from the following equation considering A=C=1/2:
- C−C⋅A24−C36=1/2−1/32−1/48=0.448.