Difference between revisions of "Aufgaben:Exercise 3.9: Circular Arc and Parabola"

Revision as of 15:53, 17 March 2022

Locus curves in FM:
Arc and Parabola

We now consider the frequency modulation of a cosine source signal

$$q(t) = A_{\rm N} \cdot \cos(2 \pi \cdot f_{\rm N} \cdot t )$$

with amplitude $A_{\rm N} = 1 \ \rm V$ and frequency $f_{\rm N} = 5 \ \rm kHz$.

The modulation index (phase deviation) is $η = 2.4$.
The corresponding low-pass signal with normalized carrier amplitude $(A_{\rm T} = 1)$ is:

$$ s_{\rm TP}(t) = \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm}\cdot \hspace{0.05cm} t}.$$

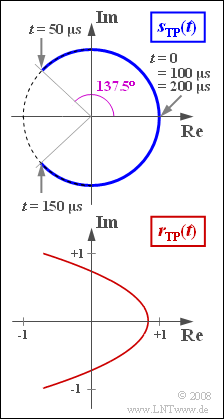
This represents an arc. Within the period $T_{\rm N} = 1/f_{\rm N} = 200 \ \rm µ s$ the following phase angles result:

$$ \phi(0) = 0, \hspace{0.2cm}\phi(0.25 \cdot T_{\rm N}) = \eta, \hspace{0.2cm}\phi(0.5 \cdot T_{\rm N})= 0,\hspace{0.2cm} \phi(0.75 \cdot T_{\rm N})= -\eta,\hspace{0.2cm}\phi(T_{\rm N})= 0.$$

Theoretically, the channel bandwidth required to transmit this signal is infinite.

However, if the bandwidth is limited to $B_{\rm K} = 25 \ \rm kHz$, for example,the equivalent low-pass signal of the received signal can be described as follows:

$$r_{\rm TP}(t) = \sum_{n = - 2}^{+2}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm}\cdot \hspace{0.05cm} t}.$$

In this case, the result is a parabolic locus curve

$$ y^2 + a \cdot x + b = 0,$$

which will be analyzed in this task.

Hints:

This exercise belongs to the chapter Frequency Modulation.
Reference is also made to the chapter Phase Modulation and particularly to the section Signal characteristics with frequency modulation.
During calculation, assume the following values of the Bessel function:

$${\rm J}_0 (2.4) \approx 0, \hspace{0.2cm}{\rm J}_1 (2.4) = -{\rm J}_{-1} (2.4)\approx 0.52, \hspace{0.2cm}{\rm J}_2 (2.4) = {\rm J}_{-2} (2.4)\approx 0.43.$$

Question

$K_{\rm FM} \ = \ $

$\ \cdot 10^4 \ \rm (Vs)^{-1}$

$x_{\rm max} \ = \ $

$x_{\rm min} \ = \ $

$y_{\rm max} \ = \ $

$y_{\rm min} \ = \ $

$ϕ(t = n · T_{\rm N}/2) \ = \ $

$\ \rm degrees$

$ϕ_{\rm max} \ = \ $

$\ \rm degrees$

$a\ = \ $

$b\ = \ $

Musterlösung

Solution

(1) Bei Frequenzmodulation eines Cosinussignals gilt für den Modulationsindex:

$$ \eta = \frac{K_{\rm FM} \cdot A_{\rm N}}{ \omega_{\rm N}} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} K_{\rm FM} = \frac{2 \pi \cdot f_{\rm N }\cdot \eta}{ A_{\rm N}} = \frac{2 \pi \cdot 5 \cdot 10^3 \,{\rm Hz}\cdot 2.4}{ 1\,{\rm V}} \hspace{0.15cm}\underline {\approx 7.54 }\cdot 10^4 \,\,{\rm V}^{-1}{\rm s}^{-1}\hspace{0.05cm}.$$

(2) Die angegebene Gleichung für das äquivalente TP–Signal lautet in ausgeschriebener Form mit $γ = ω_{\rm N} · t$ unter Berücksichtigung von ${\rm J}_{–1} = –{\rm J}_1$ und ${\rm J}_{–2} = {\rm J}_2$:

$$r_{\rm TP}(t) = {\rm J}_0 + \left[ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \gamma} - {\rm e}^{\hspace{0.05cm}{-\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \gamma} \right]\cdot {\rm J}_1 \hspace{0.27cm} +\left[ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2\gamma} + {\rm e}^{\hspace{0.05cm}{-\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2\gamma} \right]\cdot {\rm J}_2 = {\rm J}_0 + 2 \cdot {\rm j} \cdot {\rm J}_1 \cdot \sin (\gamma)+ 2 \cdot {\rm J}_2 \cdot \cos (2\gamma)\hspace{0.05cm} .$$

Somit ergibt sich für den Realteil allgemein bzw. für $η = 2.4$, das heißt ${\rm J}_0 = 0$ und ${\rm J}_2 = 0.43$:

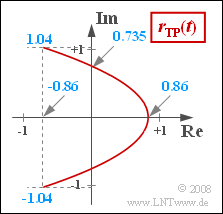
$$ x(t) = {\rm J}_0 + 2 \cdot {\rm J}_2 \cdot \cos (2 \omega_{\rm N} t ) = 2 \cdot {\rm J}_2 \cdot \cos (2 \omega_{\rm N} t ) \hspace{0.3cm} \Rightarrow \hspace{0.3cm} x_{\rm max} = 2 \cdot {\rm J}_2\hspace{0.15cm}\underline { = 0.86}, \hspace{0.3cm} x_{\rm min} = -x_{\rm max} \hspace{0.15cm}\underline {= -0.86}\hspace{0.05cm}.$$

(3) Entsprechend dem Ergebnis der Teilaufgabe (2) erhält man für den Imaginärteil $({\rm J}_1 = 0.52)$:

$$y(t) = 2 \cdot {\rm J}_1 \cdot \sin ( \omega_{\rm N} t )\hspace{0.3cm}\Rightarrow \hspace{0.3cm} y_{\rm max} =2 \cdot {\rm J}_1\hspace{0.15cm}\underline { = 1.04}\hspace{0.05cm}, \hspace{0.3cm} y_{\rm min} = -y_{\rm max}\hspace{0.15cm}\underline { = -1.04}\hspace{0.05cm}.$$

(4) Der Imaginärteil ist zu diesen Zeitpunkten jeweils Null und damit auch die Phasenfunktion:

$$ϕ(t = n · T_{\rm N}/2)\hspace{0.15cm}\underline{= 0}.$$

Diesen Sachverhalt erkennt man auch aus der Skizze auf der Angabenseite.

(5) Aus der Skizze ist bereits zu erkennen, dass der Phasenwinkel beispielsweise für $t = T_{\rm N}/4$ seinen Maximalwert erreicht.

Dieser kann mit $y_{\rm max} = 1.04$ und $x_{\rm min} = -0.86$ wie folgt berechnet werden:

$$\phi_{\rm max} = \arctan \frac{y_{\rm max}}{x_{\rm min}} = \arctan (-1.21) = 180^\circ - 50.4^\circ \hspace{0.15cm}\underline {= 129.6^\circ} \hspace{0.05cm}.$$

Ohne Bandbegrenzung würde sich hier der Phasenwinkel $ϕ(t = T_{\rm N}/4) = η = 2.4 = 137.5^\circ$ ergeben.
Die maximale Abweichung des Sinkensignals vom Quellensignal tritt somit beispielsweise zur Zeit $t = T_{\rm N}/4$ auf.

Parabelverlauf

(6) Mit $γ = ω_{\rm N} · t$ und $\cos(2γ) = 1 - 2 · \cos^2(γ)$ kann für Real– und Imaginärteil geschrieben werden:

$$x = {\rm J}_0 + 4 \cdot {\rm J}_2 \cdot \cos^2 (\gamma) - 2 \cdot {\rm J}_2\hspace{0.05cm},\hspace{0.3cm} y = 2 \cdot {\rm J}_1 \cdot \sin (\gamma) \hspace{0.05cm}.$$

Diese Gleichungen können wie folgt umgeformt werden:

$$\cos^2 (\gamma) =\frac{x-{\rm J}_0 + 2 \cdot {\rm J}_2 }{4 \cdot {\rm J}_2} \hspace{0.05cm},\hspace{0.3cm} \sin^2 (\gamma) = \frac{y^2 }{4 \cdot {\rm J}_1^2}$$

$$\Rightarrow \hspace{0.3cm} \frac{y^2 }{4 \cdot {\rm J}_1^2} + \frac{x-{\rm J}_0 + 2 \cdot {\rm J}_2 }{4 \cdot {\rm J}_2} =1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {y^2 } + \frac{{\rm J}_1^2}{ {\rm J}_2} \cdot x + {{\rm J}_1^2} \cdot \left ( 2 - \frac{{\rm J}_0}{ {\rm J}_2} \right ) =0\hspace{0.05cm}.$$

Damit lauten die Parabelparameter für $ {\rm J}_0 = 0$:

$$a = \frac{{\rm J}_1^2}{ {\rm J}_2} \hspace{0.15cm}\underline {\approx 0.629}, \hspace{0.3cm} b = 2 \cdot {\rm J}_1^2 \hspace{0.15cm}\underline {\approx 0.541} \hspace{0.05cm}.$$

Zur Kontrolle verwenden wir $y = 0$ ⇒ $ x_{\rm max} = {b}/{a} = 2 \cdot {\rm J}_2 = 0.86 \hspace{0.05cm}.$
Die Werte bei $x = 0$ sind somit: $y_0 = \pm \sqrt{2} \cdot {\rm J}_1 \approx 0.735 \hspace{0.05cm}.$

@@ Line 40: / Line 40: @@
 <quiz display=simple>
-{Wie groß ist die Modulatorkonstante &nbsp;$K_{\rm FM}$?
+{What is the modulator constant &nbsp;$K_{\rm FM}$?
 |type="{}"}
 $K_{\rm FM} \ = \ $ { 7.54 3% } $\ \cdot 10^4 \ \rm (Vs)^{-1}$
-{Berechnen Sie den Realteil &nbsp;$x(t) = {\rm Re}\big[r_{\rm TP}(t)\big]$&nbsp; des äquivalenten Tiefpass-Signals und geben Sie dessen Maximum und Minimum an.
+{Calculate the real part&nbsp;$x(t) = {\rm Re}\big[r_{\rm TP}(t)\big]$&nbsp; of the equivalent low-pass signal and give its maximum and minimum.
 |type="{}"}
 $x_{\rm max} \ = \ $ { 0.86 3% }
 $x_{\rm min} \ = \ $ { -0.89--0.83 }
-{Wie groß ist das Maximum und Minimum des Imaginärteils &nbsp;$y(t) = {\rm Im}\big[r_{\rm TP}(t)\big]$?
+{What is the maximum and minimum of the imaginary part &nbsp;$y(t) = {\rm Im}\big[r_{\rm TP}(t)\big]$?
 |type="{}"}
 $y_{\rm max} \ = \ $ { 1.04 3% }
 $y_{\rm min} \ = \ $ { -1.07--1.01 }
-{Welche Phasenwerte ergeben sich bei allen Vielfachen von &nbsp;$T_{\rm N}/2$?
+{What are the phase values for all multiples of &nbsp;$T_{\rm N}/2$?
 |type="{}"}
-$ϕ(t = n · T_{\rm N}/2) \ = \ $ { 0. } $\ \rm Grad$
+$ϕ(t = n · T_{\rm N}/2) \ = \ $ { 0. } $\ \rm degrees$
-{Wie groß ist der maximale Phasenwinkel &nbsp;$ϕ_{\rm max}$?&nbsp; Interpretieren Sie das Ergebnis.
+{What is the maximum phase angle &nbsp;$ϕ_{\rm max}$?&nbsp; Interpret the result.
 |type="{}"}
-$ϕ_{\rm max} \ = \ $ { 129.6 3% } $\ \rm Grad$
+$ϕ_{\rm max} \ = \ $ { 129.6 3% } $\ \rm degrees$
-{Zeigen Sie, dass man die Ortskurve in der Form &nbsp;$y^2 + a · x + b = 0$&nbsp; angeben kann.&nbsp; Bestimmen Sie die Parabelparameter &nbsp;$a$&nbsp; und &nbsp;$b$.
+{Show that the locus curve can be given in the form &nbsp;$y^2 + a · x + b = 0$&nbsp;.&nbsp; Determine the parabolic parameters  &nbsp;$a$&nbsp; and &nbsp;$b$.
 |type="{}"}
 $a\ = \ $ { 0.629 3%  }