Difference between revisions of "Aufgaben:Exercise 3.11: Pre-Emphase and De-Emphase"
m |
|||
| Line 4: | Line 4: | ||
[[File:P_ID1116__Mod_A_3_10.png|right|frame|Realisierung einer Preemphase]] | [[File:P_ID1116__Mod_A_3_10.png|right|frame|Realisierung einer Preemphase]] | ||
| − | + | In voice and audio signal transmission, the signal frequency band is pre-distorted before the FM modulator via an RC high-pass filter according to the diagram. This measure is called "pre-emphasis" $\rm (PE)$. | |
| − | + | The amplitude response of the preemphasis network, together with | |
| − | * | + | *the two cutoff frequencies $f_{\rm G1} = (2π · R_1 · C)^{–1}$ and $f_{\rm G2} = f_{\rm G1}/α_0$, as well as |
| − | * | + | *the DC signal transmission factor $α_0 = R_2/(R_1 + R_2)$ |
| + | is given by: | ||
:$$ |H_{\rm PE} (f)| = \alpha_0 \cdot \sqrt{\frac{1 + (f/f_{\rm G1})^2}{1 + (f/f_{\rm G2})^2}} \hspace{0.05cm}.$$ | :$$ |H_{\rm PE} (f)| = \alpha_0 \cdot \sqrt{\frac{1 + (f/f_{\rm G1})^2}{1 + (f/f_{\rm G2})^2}} \hspace{0.05cm}.$$ | ||
| − | + | For practical purposes, we can assume that the maximum message frequency $f_{\rm N}$ is much smaller than $f_{\rm G2}$ . | |
| − | + | If we further consider that the DC signal transmission factor $α_0$ can be changed by an amplification of nbsp;$α$ , we can further assume the following pre-emphasis frequency response | |
| + | where $(f_{\rm G} = f_{\rm G1} = 3 \ \rm kHz)$: | ||
:$$|H_{\rm PE} (f)| \approx \alpha \cdot \sqrt{{1 + \left({f}/{f_{\rm G}}\right)^2}} \hspace{0.05cm}.$$ | :$$|H_{\rm PE} (f)| \approx \alpha \cdot \sqrt{{1 + \left({f}/{f_{\rm G}}\right)^2}} \hspace{0.05cm}.$$ | ||
| − | + | In this network, the frequency deviation is $Δf_{\rm A}$ as a function of the message frequency $f_{\rm N}$: | |
:$$ \Delta f_{\rm A} (f_{\rm N}) = \Delta f_{\rm A, \hspace{0.08cm}min} \cdot \sqrt{{1 + \left({f_{\rm N}}/{f_{\rm G}}\right)^2}} \hspace{0.05cm}.$$ | :$$ \Delta f_{\rm A} (f_{\rm N}) = \Delta f_{\rm A, \hspace{0.08cm}min} \cdot \sqrt{{1 + \left({f_{\rm N}}/{f_{\rm G}}\right)^2}} \hspace{0.05cm}.$$ | ||
| − | * | + | *Here $Δf_\text{A, min}$ is the frequency deviation for very small frequencies $(f_{\rm N} → 0)$. |
| − | * | + | *This parameter should be chosen so that the maximum frequency deviation $Δf_\text{A, max}$ does not exceed $45 \ \rm kHz$. |
| − | + | In order not to distort the useful signal, this pre-emphasis must be rebalanced by a "de-emphasis" network at the receiver. The goal and purpose of preemphasis/deemphasis is solely to reduce the dependence of the signal-to-noise power ratio on the signal frequency. | |
| − | In | + | In this task, the following quantities are used: |
| − | * | + | * Sink SNR in double-sideband amplitude modulation (DSB-AM) $\rm (DSB–AM)$: |
:$$\rho_{{\rm AM} } = \frac{P_{\rm S}}{N_0 \cdot f_{\rm N} } = \xi\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{{\rm AM} } = 10 \cdot {\rm lg} \hspace{0.15cm}\xi \hspace{0.05cm},$$ | :$$\rho_{{\rm AM} } = \frac{P_{\rm S}}{N_0 \cdot f_{\rm N} } = \xi\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{{\rm AM} } = 10 \cdot {\rm lg} \hspace{0.15cm}\xi \hspace{0.05cm},$$ | ||
| − | * | + | * Sink SNR and sink-to-noise ratio in frequency modulation $\rm (FM)$ without pre-emphasis/de-emphasis: |
:$$ \rho_{\rm FM} = {3}/{2 } \cdot \eta^2 \cdot \rho_{\rm AM } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} | :$$ \rho_{\rm FM} = {3}/{2 } \cdot \eta^2 \cdot \rho_{\rm AM } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} | ||
G_{\rm FM} = 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{\rm FM} - | G_{\rm FM} = 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{\rm FM} - | ||
| Line 32: | Line 34: | ||
lg} \hspace{0.15cm}{3}/{2 } \cdot \eta^2 | lg} \hspace{0.15cm}{3}/{2 } \cdot \eta^2 | ||
\hspace{0.05cm},$$ | \hspace{0.05cm},$$ | ||
| − | * | + | *Sink SNR and sink-to-noise ratio in frequency modulation $\rm (FM)$ using pre-emphasis/de-emphasis: |
:$$ \rho_{\rm DE} = \frac{(f_{\rm N}/f_{\rm G})^3}{3 \cdot (f_{\rm N}/f_{\rm G} - \arctan (f_{\rm N}/f_{\rm G}) } | :$$ \rho_{\rm DE} = \frac{(f_{\rm N}/f_{\rm G})^3}{3 \cdot (f_{\rm N}/f_{\rm G} - \arctan (f_{\rm N}/f_{\rm G}) } | ||
\hspace{0.3cm}\Rightarrow \hspace{0.3cm} | \hspace{0.3cm}\Rightarrow \hspace{0.3cm} | ||
| Line 42: | Line 44: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Modulation_Methods/Influence_of_Noise_on_Systems_with_Angle_Modulation|Influence of Noise on Systems with Angle Modulation]]. |
| − | * | + | *Particular reference is made to the section [[Modulation_Methods/Influence_of_Noise_on_Systems_with_Angle_Modulation#Pre-emphasis_and_de-emphasis|Pre-emphasis and de-emphasis]]. |
| − | * | + | *Throughout the task, assume a message signal containing frequencies up to and including $B_{\rm NF}= 9 \ \rm kHz$ . |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| Line 84: | Line 86: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
'''(1)''' Richtig sind <u>der erste und der letzte Lösungsvorschlag</u>: | '''(1)''' Richtig sind <u>der erste und der letzte Lösungsvorschlag</u>: | ||
Revision as of 19:42, 17 March 2022
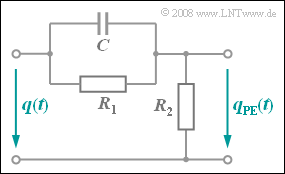
In voice and audio signal transmission, the signal frequency band is pre-distorted before the FM modulator via an RC high-pass filter according to the diagram. This measure is called "pre-emphasis" $\rm (PE)$.
The amplitude response of the preemphasis network, together with
- the two cutoff frequencies $f_{\rm G1} = (2π · R_1 · C)^{–1}$ and $f_{\rm G2} = f_{\rm G1}/α_0$, as well as
- the DC signal transmission factor $α_0 = R_2/(R_1 + R_2)$
is given by:
- $$ |H_{\rm PE} (f)| = \alpha_0 \cdot \sqrt{\frac{1 + (f/f_{\rm G1})^2}{1 + (f/f_{\rm G2})^2}} \hspace{0.05cm}.$$
For practical purposes, we can assume that the maximum message frequency $f_{\rm N}$ is much smaller than $f_{\rm G2}$ .
If we further consider that the DC signal transmission factor $α_0$ can be changed by an amplification of nbsp;$α$ , we can further assume the following pre-emphasis frequency response where $(f_{\rm G} = f_{\rm G1} = 3 \ \rm kHz)$:
- $$|H_{\rm PE} (f)| \approx \alpha \cdot \sqrt{{1 + \left({f}/{f_{\rm G}}\right)^2}} \hspace{0.05cm}.$$
In this network, the frequency deviation is $Δf_{\rm A}$ as a function of the message frequency $f_{\rm N}$:
- $$ \Delta f_{\rm A} (f_{\rm N}) = \Delta f_{\rm A, \hspace{0.08cm}min} \cdot \sqrt{{1 + \left({f_{\rm N}}/{f_{\rm G}}\right)^2}} \hspace{0.05cm}.$$
- Here $Δf_\text{A, min}$ is the frequency deviation for very small frequencies $(f_{\rm N} → 0)$.
- This parameter should be chosen so that the maximum frequency deviation $Δf_\text{A, max}$ does not exceed $45 \ \rm kHz$.
In order not to distort the useful signal, this pre-emphasis must be rebalanced by a "de-emphasis" network at the receiver. The goal and purpose of preemphasis/deemphasis is solely to reduce the dependence of the signal-to-noise power ratio on the signal frequency.
In this task, the following quantities are used:
- Sink SNR in double-sideband amplitude modulation (DSB-AM) $\rm (DSB–AM)$:
- $$\rho_{{\rm AM} } = \frac{P_{\rm S}}{N_0 \cdot f_{\rm N} } = \xi\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{{\rm AM} } = 10 \cdot {\rm lg} \hspace{0.15cm}\xi \hspace{0.05cm},$$
- Sink SNR and sink-to-noise ratio in frequency modulation $\rm (FM)$ without pre-emphasis/de-emphasis:
- $$ \rho_{\rm FM} = {3}/{2 } \cdot \eta^2 \cdot \rho_{\rm AM } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} G_{\rm FM} = 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{\rm FM} - 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{\rm AM}= 10 \cdot {\rm lg} \hspace{0.15cm}{3}/{2 } \cdot \eta^2 \hspace{0.05cm},$$
- Sink SNR and sink-to-noise ratio in frequency modulation $\rm (FM)$ using pre-emphasis/de-emphasis:
- $$ \rho_{\rm DE} = \frac{(f_{\rm N}/f_{\rm G})^3}{3 \cdot (f_{\rm N}/f_{\rm G} - \arctan (f_{\rm N}/f_{\rm G}) } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} G_{\rm DE} = 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{\rm DE} - 10 \cdot {\rm lg} \hspace{0.15cm}\rho_{\rm FM}\hspace{0.05cm}$$
Hints:
- This exercise belongs to the chapter Influence of Noise on Systems with Angle Modulation.
- Particular reference is made to the section Pre-emphasis and de-emphasis.
- Throughout the task, assume a message signal containing frequencies up to and including $B_{\rm NF}= 9 \ \rm kHz$ .
Questions
Solution
- Der Betragsfrequenzgang des Deemphase–Netzwerks ist wie folgt festgelegt:
- $$ |H_{\rm DE} (f)| = \frac{1}{|H_{\rm PE} (f)|}= \frac{1}{\alpha}\cdot \frac{1}{\sqrt{1 + (f/f_{\rm G})^2}} \hspace{0.05cm}.$$
- Der Frequenzgang eines einfachen RC–Tiefpasses – auch bekannt als Tiefpass erster Ordnung – lautet:
- $$ H_{\rm RC-TP} (f) = \frac{1}{{1 + {\rm j}\cdot f/f_{\rm G}}} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} |H_{\rm RC-TP} (f)| = \frac{1}{\sqrt{1 + (f/f_{\rm G})^2}}\hspace{0.05cm}.$$
(2) Die Frequenzmodulation ist auf die maximale Frequenz $B_{\rm NF} = f_\text{N, max}= 9 \ \rm kHz$ ausgelegt. Dann soll der (maximale) Frequenzhub $Δf_{\rm A} = 45\ \rm kHz$ betragen.
- Daraus folgt für den Modulationsindex:
- $$ \eta = \frac{\Delta f_{\rm A}}{f_{\rm N} } = 5 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} G_{\rm FM} (f_{\rm N} = 9\,{\rm kHz}) = 10 \cdot {\rm lg} \hspace{0.15cm}(1.5 \cdot 5^2) \hspace{0.15cm}\underline {\approx 15.74\,{\rm dB}} \hspace{0.05cm}.$$
- Mit der Nachrichtenfrequenz $ f_{\rm N} = 3 \ \rm kHz$ ergibt sich ein um den Faktor $3$ größerer Modulationsindex und damit ein um den Faktor $10 · \lg \ 9 = 9.54 \ \rm dB$ größerer Störabstand:
- $$G_{\rm FM} (f_{\rm N} = 3\,{\rm kHz}) = 10 \cdot {\rm lg} \hspace{0.15cm}(1.5 \cdot 15^2) \hspace{0.15cm}\underline {\approx 25.28\,{\rm dB}} \hspace{0.05cm}.$$
- Ein weiterer Zugewinn ergibt sich durch den Übergang von $3\ \rm kHz$ auf $1\ \rm kHz$:
- $$G_{\rm FM} (f_{\rm N} = 1\,{\rm kHz}) = 25.28\,{\rm dB} + 9.54\,{\rm dB}\hspace{0.15cm}\underline {= 34.82\,{\rm dB}} \hspace{0.05cm}.$$
(3) Der maximale Frequenzhub ergibt sich für $f_{\rm N} = B_{\rm NF}$.
- Daraus folgt mit $f_{\rm G} = 3 \ \rm kHz$ und $B_{\rm NF} = 9 \ \rm kHz$:
- $$\Delta f_{\rm A} (B_{\rm NF}) = \Delta f_{\rm A, \hspace{0.08cm}min} \cdot \sqrt{{1 + \left(\frac{B_{\rm NF}}{f_{\rm G}}\right)^2}} = \sqrt {10} \cdot \Delta f_{\rm A, \hspace{0.08cm}min}= \Delta f_{\rm A, \hspace{0.08cm}max} = 45\,{\rm kHz}$$
- $$\Rightarrow \hspace{0.3cm} \Delta f_{\rm A, \hspace{0.08cm}min} = \frac{45\,{\rm kHz}}{\sqrt {10}}\hspace{0.15cm}\underline {\approx 14.23\,{\rm kHz}}\hspace{0.05cm}.$$
(4) Mit der angegebenen Formel erhält man folgende "Gewinne durch Premphase/Deemphase":
- $$G_{\rm DE} (f_{\rm N} = 9\,{\rm kHz}) = 10 \cdot {\rm lg}\hspace{0.15cm} \frac{(f_{\rm N}/f_{\rm G})^3}{3 \cdot (f_{\rm N}/f_{\rm G} - \arctan (f_{\rm N}/f_{\rm G}) }= 10 \cdot {\rm lg}\hspace{0.15cm} \frac{3^3}{3 \cdot (3 - 1.249) }\hspace{0.15cm}\underline {\approx 7.1\,{\rm dB}}\hspace{0.05cm},$$
- $$ G_{\rm DE} (f_{\rm N} = 3\,{\rm kHz}) = 10 \cdot {\rm lg}\hspace{0.15cm} \frac{1^3}{3 \cdot (1 - \pi/4) }\hspace{0.15cm}\underline {\approx 1.9\,{\rm dB}}\hspace{0.05cm},$$
- $$G_{\rm DE} (f_{\rm N} = 1\,{\rm kHz}) = 10 \cdot {\rm lg}\hspace{0.15cm} \frac{(1/3)^3}{3 \cdot (1/3 - 0.322) }\hspace{0.15cm}\underline {\approx 0.28\,{\rm dB}}\hspace{0.05cm}.$$