Difference between revisions of "Aufgaben:Exercise 5.3: AWGN and BSC Model"
| Line 2: | Line 2: | ||
{{quiz-Header|Buchseite=Digital_Signal_Transmission/Binary_Symmetric_Channel_(BSC)}} | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Binary_Symmetric_Channel_(BSC)}} | ||
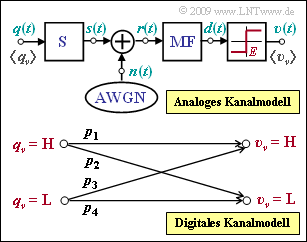
| − | [[File:P_ID1831__Dig_A_5_3.png|right|frame| | + | [[File:P_ID1831__Dig_A_5_3.png|right|frame|AWGN channel and BSC model]] |
| − | + | The graphic above shows the analog channel model of a digital transmission system, where the additive noise signal $n(t)$ with the (two-sided) noise power density $N_0/2$ is effective. This is AWGN noise. The variance of the noise component before the decision (after the matched filter) is then | |
:$$\sigma^2 = \frac{N_0}{2T} \hspace{0.05cm}.$$ | :$$\sigma^2 = \frac{N_0}{2T} \hspace{0.05cm}.$$ | ||
| − | + | Further, let hold: | |
| − | * | + | * No intersymbol interference occurs. If the symbol $q_{\nu} = \mathbf{H}$ was sent, the useful component of the detection signal is equal to $+s_0$, while for $q_{\nu} = \mathbf{L}$, it is equal to $-s_0$. |
| − | * | + | * The threshold decision takes into account a threshold drift, that is, the threshold $E$ may well deviate from the optimal value $E = 0$. The <i>decision rule</i> is: |
:$$\upsilon_\nu = | :$$\upsilon_\nu = | ||
\left\{ \begin{array}{c} \mathbf{H} \\ | \left\{ \begin{array}{c} \mathbf{H} \\ | ||
\mathbf{L} \end{array} \right.\quad | \mathbf{L} \end{array} \right.\quad | ||
| − | \begin{array}{*{1}c} {\rm | + | \begin{array}{*{1}c} {\rm if}\hspace{0.15cm}d (\nu \cdot T) > E \hspace{0.05cm}, |
| − | \\ {\rm | + | \\ {\rm if} \hspace{0.15cm} d (\nu \cdot T) \le E\hspace{0.05cm}.\\ \end{array}$$ |
| − | * | + | * With the threshold value $E = 0$, the mean error probability is given by |
:$$p_{\rm M} = {\rm Q} \left ( {s_0}/{\sigma} \right ) = 0.01\hspace{0.05cm}.$$ | :$$p_{\rm M} = {\rm Q} \left ( {s_0}/{\sigma} \right ) = 0.01\hspace{0.05cm}.$$ | ||
| − | + | The bottom graph shows a digital channel model characterized by the four transition probabilities $p_1, p_2, p_3$ and $p_4$. This is to be fitted to the analog channel model. | |
| Line 25: | Line 25: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | * The exercise belongs to the chapter [[Digital_Signal_Transmission/Binary_Symmetric_Channel_(BSC)| "Binary Symmetric Channel (BSC)"]]. |
| − | * | + | * Numerical values of the Q–function can be determined with the interactive applet [[Applets:Complementary_Gaussian_Error_Functions|"Complementary Gaussian Error Functions"]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which quotient $s_0/\sigma$ is the basis of this exercise? |
|type="{}"} | |type="{}"} | ||
$s_0/\sigma\ = \ ${ 2.32 3% } | $s_0/\sigma\ = \ ${ 2.32 3% } | ||
| − | { | + | {For the threshold, let $E = 0$. Is the digital transmission system at hand describable by the BSC model, assuming that |
|type="[]"} | |type="[]"} | ||
| − | + | + | + the source symbols $\mathbf{L}$ and $\mathbf{H}$ are equally probable, |
| − | + | + | + the source symbol $\mathbf{L}$ occurs significantly more frequently than $\mathbf{H}$? |
| − | { | + | {Calculate the transition probabilities for $E = +s_0/4$. |
|type="{}"} | |type="{}"} | ||
$p_1 \ = \ $ { 0.959 3% } | $p_1 \ = \ $ { 0.959 3% } | ||
| Line 50: | Line 50: | ||
$p_4 \ = \ $ { 0.998 3% } | $p_4 \ = \ $ { 0.998 3% } | ||
| − | { | + | {Now let $E = +s_0/4$. Is the present digital transmission system describable by the BSC model under the condition that |
|type="[]"} | |type="[]"} | ||
| − | - | + | - the source symbols $\mathbf{L}$ and $\mathbf{H}$ are equally probable, |
| − | - | + | - the source symbol $\mathbf{L}$ occurs significantly more frequently than $\mathbf{H}$? |
| − | { | + | {Let $p_{\rm L} = {\rm Pr}(q_{\nu} = \mathbf{L})$ and $p_{\rm H} = {\rm Pr}(q_{\nu} = \mathbf{H})$. Which of the following statements is then true for the mean error probability $p_{\rm M}$? |
|type="[]"} | |type="[]"} | ||
| − | + $p_{\rm M}$ | + | + $p_{\rm M}$ in the BSC model $($valid for $E = 0)$ is independent of $p_{\rm L}$ and $p_{\rm H}$. |
| − | - $p_{\rm M}$ | + | - $p_{\rm M}$ in the BSC model $($valid for $E = 0)$ is smallest for $p_{\rm L} = p_{\rm H}$. |
| − | + | + | + For $p_{\rm L} = 0.9$, $p_{\rm H} = 0.1$ and $E = +s_0/4$ is $p_{\rm M} < 1\%$. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The average error probability is $p_{\rm M} = {\rm Q}(s_0/\sigma) = 0.01$. |
| − | * | + | *From this it follows for the quotient of the detection useful sample value and the detection noise rms value: |
:$${s_0}/{\sigma}= {\rm Q}^{-1} \left ( 0.01 \right ) \hspace{0.15cm}\underline {\approx 2.32}\hspace{0.05cm}.$$ | :$${s_0}/{\sigma}= {\rm Q}^{-1} \left ( 0.01 \right ) \hspace{0.15cm}\underline {\approx 2.32}\hspace{0.05cm}.$$ | ||
| − | '''(2)''' | + | '''(2)''' With $E = 0$, the probabilities of the given digital channel model are given by: |
:$$p_2 = p_3 = p = 0.01 \hspace{0.05cm}, \hspace{0.2cm}p_1 = p_4 = 1-p = 0.99\hspace{0.05cm}.$$ | :$$p_2 = p_3 = p = 0.01 \hspace{0.05cm}, \hspace{0.2cm}p_1 = p_4 = 1-p = 0.99\hspace{0.05cm}.$$ | ||
Revision as of 13:55, 26 August 2022
The graphic above shows the analog channel model of a digital transmission system, where the additive noise signal $n(t)$ with the (two-sided) noise power density $N_0/2$ is effective. This is AWGN noise. The variance of the noise component before the decision (after the matched filter) is then
- $$\sigma^2 = \frac{N_0}{2T} \hspace{0.05cm}.$$
Further, let hold:
- No intersymbol interference occurs. If the symbol $q_{\nu} = \mathbf{H}$ was sent, the useful component of the detection signal is equal to $+s_0$, while for $q_{\nu} = \mathbf{L}$, it is equal to $-s_0$.
- The threshold decision takes into account a threshold drift, that is, the threshold $E$ may well deviate from the optimal value $E = 0$. The decision rule is:
- $$\upsilon_\nu = \left\{ \begin{array}{c} \mathbf{H} \\ \mathbf{L} \end{array} \right.\quad \begin{array}{*{1}c} {\rm if}\hspace{0.15cm}d (\nu \cdot T) > E \hspace{0.05cm}, \\ {\rm if} \hspace{0.15cm} d (\nu \cdot T) \le E\hspace{0.05cm}.\\ \end{array}$$
- With the threshold value $E = 0$, the mean error probability is given by
- $$p_{\rm M} = {\rm Q} \left ( {s_0}/{\sigma} \right ) = 0.01\hspace{0.05cm}.$$
The bottom graph shows a digital channel model characterized by the four transition probabilities $p_1, p_2, p_3$ and $p_4$. This is to be fitted to the analog channel model.
Notes:
- The exercise belongs to the chapter "Binary Symmetric Channel (BSC)".
- Numerical values of the Q–function can be determined with the interactive applet "Complementary Gaussian Error Functions".
Questions
Solution
- From this it follows for the quotient of the detection useful sample value and the detection noise rms value:
- $${s_0}/{\sigma}= {\rm Q}^{-1} \left ( 0.01 \right ) \hspace{0.15cm}\underline {\approx 2.32}\hspace{0.05cm}.$$
(2) With $E = 0$, the probabilities of the given digital channel model are given by:
- $$p_2 = p_3 = p = 0.01 \hspace{0.05cm}, \hspace{0.2cm}p_1 = p_4 = 1-p = 0.99\hspace{0.05cm}.$$
- Ein Vergleich mit dem Theorieteil zeigt, dass dieses Kanalmodell dem BSC–Modell entspricht, und zwar unabhängig von der Statistik der Quellensymbole.
- Richtig sind also beide Lösungsvorschläge.
(3) Die Übergangswahrscheinlichkeit $p_2$ beschreibt nun den Fall, dass die Enscheiderschwelle $E = 0.25 \cdot s_0$ fälschlicherweise unterschritten wurde.
- Dann ist $v_{\nu} = \mathbf{L}$, obwohl $q_{\nu} = \mathbf{H}$ gesendet wurde. Der Abstand von der Schwelle beträgt somit nur $0.75 \cdot s_0$ und es gilt:
- $$p_{\rm 2} \hspace{-0.1cm} \ = \ \hspace{-0.1cm}{\rm Q} \left ( \frac{0.75 \cdot s_0}{\sigma} \right ) = {\rm Q} \left ( 0.75 \cdot 2.32 \right ) = {\rm Q} \left ( 1.74 \right )\hspace{0.15cm}\underline {\approx 0.041}\hspace{0.05cm}, \hspace{0.5cm} p_{\rm 1} \hspace{-0.1cm} \ = \ \hspace{-0.1cm}1 - p_{\rm 2} \hspace{0.15cm}\underline {= 0.959}\hspace{0.05cm}.$$
- In ähnlicher Weise können die Übergangswahrscheinlichkeiten $p_3$ und $p_4$ berechnet werden, wobei nun vom Schwellenabstand $1.25 \cdot s_0$ auszugehen ist:
- $$p_{\rm 3} = {\rm Q} \left ( 1.25 \cdot 2.32 \right ) = {\rm Q} \left ( 2.90 \right )\hspace{0.15cm}\underline {\approx 0.002}\hspace{0.05cm}, \hspace{0.2cm} p_{\rm 4} = 1 - p_{\rm 3}\hspace{0.15cm}\underline { = 0.998}\hspace{0.05cm}.$$
(4) Keiner der beiden Lösungsvorschläge trifft zu:
- Mit der Entscheiderschwelle $E ≠ 0$ ist das BSC–Modell unabhängig von der Symbolstatistik nicht anwendbar,
- da die Symmetrieeigenschaft des Kanals (das Kennzeichen "S" in "BSC") nicht gegeben ist.
(5) Die Aussagen 1 und 3 treffen zu, nicht aber die Aussage 2:
- Beim BSC–Modell ist $p_{\rm M} = 1\%$ unabhängig von den Symbolwahrscheinlichkeiten $p_{\rm L}$ und $p_{\rm H}$.
- Dagegen gilt für $p_{\rm L} = 0.9$, $p_{\rm H} = 0.1$ sowie $E = +s_0/4$:
- $$p_{\rm M} = 0.9 \cdot p_{\rm 3} + 0.1 \cdot p_{\rm 2}= 0.9 \cdot 0.2\% + 0.1 \cdot 4.1\% \approx 0.59\% \hspace{0.05cm}.$$
- Das Minimum ergibt sich für $p_{\rm L} = 0.93$ und $p_{\rm H} = 0.07$ zu $p_{\rm M} \approx 0.45\%$.