Difference between revisions of "Aufgaben:Exercise 3.10: Metric Calculation"
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Decoding_of_Convolutional_Codes}} |
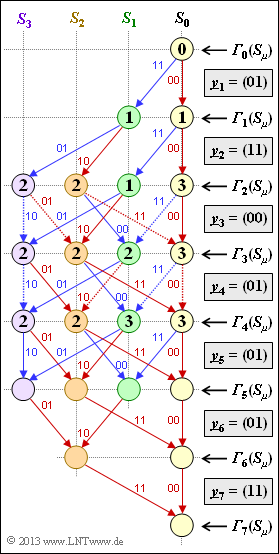
| − | [[File:P_ID2681__KC_A_3_10.png|right|frame| | + | [[File:P_ID2681__KC_A_3_10.png|right|frame|Only partially evaluated trellis]] |
| − | + | In the [[Channel_Coding/Decoding_of_Convolutional_Codes#Preliminary_remarks_on_the_following_decoding_examples| "theory section"]] of this chapter, the calculation of the branch metrics ${\it \Gamma}_i(S_{\mu})$ has been discussed in detail, based on the Hamming distance $d_{\rm H}(\underline{x}\hspace{0. 05cm}', \ \underline{y}_i)$ between the possible codewords $\underline{x}\hspace{0.05cm}' ∈ \{00, \, 01, \, 10, \, 11\}$ and the 2–bit–words $\underline{y}_i$ received at time $i$ is based. | |
| − | + | The exercise deals exactly with this topic. In the adjacent graph | |
| − | * | + | * the considered trellis is shown– valid for the code with rate $R = 1/2$, memory $m = 2$ and $\mathbf{G}(D) = (1 + D + D^2, \ 1 + D^2)$, |
| − | * | + | * are the received words $\underline{y}_1 = (01), \hspace{0.05cm}\text{ ...} \hspace{0.05cm} , \ \underline{y}_7 = (11)$ indicated in the rectangles, |
| − | * | + | * are all branch metrics ${\it \Gamma}_0(S_{\mu}), \hspace{0.05cm}\text{ ...} \hspace{0.05cm} , \ {\it \Gamma}_4(S_{\mu})$ already entered. |
| − | + | For example, the branch metric ${\it \Gamma}_4(S_0)$ with $\underline{y}_4 = (01)$ as the minimum of the two comparison values | |
| − | * ${\it \Gamma}_3(S_0) + d_{\rm H}((00), \ (01)) = 3 + 1 = 4$, | + | * ${\it \Gamma}_3(S_0) + d_{\rm H}((00), \ (01)) = 3 + 1 = 4$, and |
* ${\it \Gamma}_3(S_2) + d_{\rm H}((11), \ (01)) = 2 + 1 = 3$. | * ${\it \Gamma}_3(S_2) + d_{\rm H}((11), \ (01)) = 2 + 1 = 3$. | ||
| − | + | The surviving branch – here from ${\it \Gamma}_3(S_2)$ to ${\it \Gamma}_4(S_0)$ – is drawn solid, the eliminated branch from ${\it \Gamma}_3(S_0)$ to ${\it \Gamma}_4(S_0)$ dotted. Red arrows represent the information bit $u_i = 0$, blue arrows $u_i = 1$. | |
| − | In | + | In the subtask '''(4)''' the relationship between |
| − | * | + | *the ${\it \Gamma}_i(S_{\mu})$ minimization and |
| − | * | + | *the ${\it \Lambda}_i(S_{\mu})$ maximization. |
| − | + | shall be worked out. Here, we refer to the nodes ${\it \Lambda}_i(S_{\mu})$ as <i>metrics</i>, where the metric increment over the predecessor nodes results from the correlation value $〈\underline{x}_i\hspace{0.05cm}', \, \underline{y}_i 〉$ . For more details on this topic, see the following theory pages: | |
| − | * [[Channel_Coding/ | + | * [[Channel_Coding/Decoding_of_Convolutional_Codes#Relationship_between_Hamming_distance_and_correlation| "Relationship between Hamming distance and correlation"]] |
| − | * [[Channel_Coding/ | + | * [[Channel_Coding/Decoding_of_Convolutional_Codes#Viterbi_algorithm_based_on_correlation_and_metrics| "Viterbi algorithm based on correlation and metrics"]] |
| − | * [[Channel_Coding/ | + | * [[Channel_Coding/Decoding_of_Convolutional_Codes#Viterbi_decision_for_non-terminated_convolutional_codes| "Viterbi decision for non–terminated convolutional codes"]]. |
| Line 34: | Line 34: | ||
| − | + | Hints: | |
| − | * | + | * The exercise refers to the chapter [[Channel_Coding/Decoding_of_Convolutional_Codes| "Decoding Convolutional Codes"]]. |
| − | * | + | * For the time being, the search of surviving paths is not considered. This will be dealt with for the same example in the later [[Aufgaben:Exercise_3.11:_Viterbi_Path_Finding| "Exercise 3.11"]]. |
| Line 42: | Line 42: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
{Wie lauten die Fehlergrößen für den Zeitpunkt $i = 5$? | {Wie lauten die Fehlergrößen für den Zeitpunkt $i = 5$? | ||
Revision as of 20:18, 18 October 2022
In the "theory section" of this chapter, the calculation of the branch metrics ${\it \Gamma}_i(S_{\mu})$ has been discussed in detail, based on the Hamming distance $d_{\rm H}(\underline{x}\hspace{0. 05cm}', \ \underline{y}_i)$ between the possible codewords $\underline{x}\hspace{0.05cm}' ∈ \{00, \, 01, \, 10, \, 11\}$ and the 2–bit–words $\underline{y}_i$ received at time $i$ is based.
The exercise deals exactly with this topic. In the adjacent graph
- the considered trellis is shown– valid for the code with rate $R = 1/2$, memory $m = 2$ and $\mathbf{G}(D) = (1 + D + D^2, \ 1 + D^2)$,
- are the received words $\underline{y}_1 = (01), \hspace{0.05cm}\text{ ...} \hspace{0.05cm} , \ \underline{y}_7 = (11)$ indicated in the rectangles,
- are all branch metrics ${\it \Gamma}_0(S_{\mu}), \hspace{0.05cm}\text{ ...} \hspace{0.05cm} , \ {\it \Gamma}_4(S_{\mu})$ already entered.
For example, the branch metric ${\it \Gamma}_4(S_0)$ with $\underline{y}_4 = (01)$ as the minimum of the two comparison values
- ${\it \Gamma}_3(S_0) + d_{\rm H}((00), \ (01)) = 3 + 1 = 4$, and
- ${\it \Gamma}_3(S_2) + d_{\rm H}((11), \ (01)) = 2 + 1 = 3$.
The surviving branch – here from ${\it \Gamma}_3(S_2)$ to ${\it \Gamma}_4(S_0)$ – is drawn solid, the eliminated branch from ${\it \Gamma}_3(S_0)$ to ${\it \Gamma}_4(S_0)$ dotted. Red arrows represent the information bit $u_i = 0$, blue arrows $u_i = 1$.
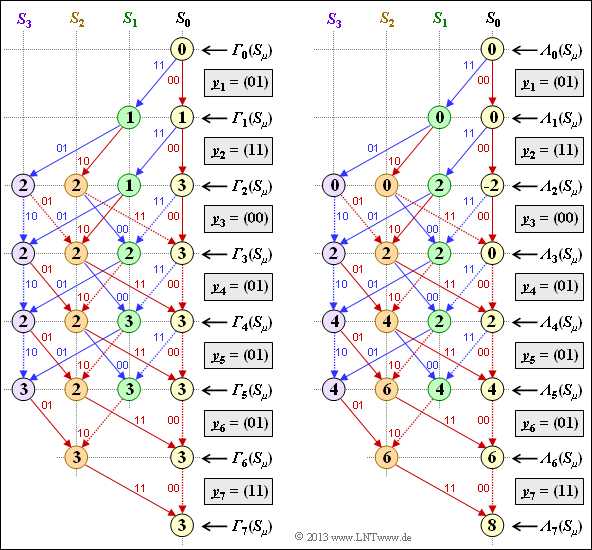
In the subtask (4) the relationship between
- the ${\it \Gamma}_i(S_{\mu})$ minimization and
- the ${\it \Lambda}_i(S_{\mu})$ maximization.
shall be worked out. Here, we refer to the nodes ${\it \Lambda}_i(S_{\mu})$ as metrics, where the metric increment over the predecessor nodes results from the correlation value $〈\underline{x}_i\hspace{0.05cm}', \, \underline{y}_i 〉$ . For more details on this topic, see the following theory pages:
- "Relationship between Hamming distance and correlation"
- "Viterbi algorithm based on correlation and metrics"
- "Viterbi decision for non–terminated convolutional codes".
Hints:
- The exercise refers to the chapter "Decoding Convolutional Codes".
- For the time being, the search of surviving paths is not considered. This will be dealt with for the same example in the later "Exercise 3.11".
Questions
Musterlösung
- $${\it \Gamma}_5(S_0) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{4}(S_0) + d_{\rm H} \big ((00)\hspace{0.05cm},\hspace{0.05cm} (01) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{4}(S_2) + d_{\rm H} \big ((11)\hspace{0.05cm},\hspace{0.05cm} (01) \big ) \right ] ={\rm min} \left [ 3+1\hspace{0.05cm},\hspace{0.05cm} 2+1 \right ] \hspace{0.15cm}\underline{= 3}\hspace{0.05cm},$$
- $${\it \Gamma}_5(S_1) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{4}(S_0) + d_{\rm H} \big ((11)\hspace{0.05cm},\hspace{0.05cm} (01) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{4}(S_2) + d_{\rm H} \big ((00)\hspace{0.05cm},\hspace{0.05cm} (01) \big ) \right ] ={\rm min} \left [ 3+1\hspace{0.05cm},\hspace{0.05cm} 2+1 \right ] \hspace{0.15cm}\underline{= 3}\hspace{0.05cm},$$
- $${\it \Gamma}_5(S_2) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{4}(S_1) + d_{\rm H} \big ((10)\hspace{0.05cm},\hspace{0.05cm} (01) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{4}(S_3) + d_{\rm H} \big ((01)\hspace{0.05cm},\hspace{0.05cm} (01) \big ) \right ] = {\rm min} \left [ 3+2\hspace{0.05cm},\hspace{0.05cm} 2+0 \right ] \hspace{0.15cm}\underline{= 2}\hspace{0.05cm},$$
- $${\it \Gamma}_5(S_3) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{4}(S_1) + d_{\rm H} \big ((01)\hspace{0.05cm},\hspace{0.05cm} (01) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{4}(S_3) + d_{\rm H} \big ((10)\hspace{0.05cm},\hspace{0.05cm} (01) \big ) \right ] ={\rm min} \left [ 3+0\hspace{0.05cm},\hspace{0.05cm} 2+2 \right ] \hspace{0.15cm}\underline{= 3}\hspace{0.05cm}.$$
Die linke Grafik zeigt das endgültig ausgewertete ${\it \Gamma}_i(S_{\mu})$–Trellis.
(2) Zum Zeitpunk $i = 6$ ist bereits die Terminierung wirksam und es gibt nur noch zwei Fehlergrößen. Für diese erhält man mit $\underline{y}_6 = (01)$:
- $${\it \Gamma}_6(S_0) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{5}(S_0) + d_{\rm H} \big ((00)\hspace{0.05cm},\hspace{0.05cm} (01) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{5}(S_2) + d_{\rm H} \big ((11)\hspace{0.05cm},\hspace{0.05cm} (01) \big ) \right ] ={\rm min} \left [ 3+1\hspace{0.05cm},\hspace{0.05cm} 2+1 \right ] \hspace{0.15cm}\underline{= 3}\hspace{0.05cm},$$
- $${\it \Gamma}_6(S_2) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{5}(S_1) + d_{\rm H} \big ((10)\hspace{0.05cm},\hspace{0.05cm} (01) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{5}(S_3) + d_{\rm H} \big ((01)\hspace{0.05cm},\hspace{0.05cm} (01) \big ) \right ] ={\rm min} \left [ 3+2\hspace{0.05cm},\hspace{0.05cm} 3+0 \right ] \hspace{0.15cm}\underline{= 3}\hspace{0.05cm}.$$
(3) Der Endwert ergibt sich zu
- $${\it \Gamma}_7(S_0) \hspace{-0.15cm} \ = \ \hspace{-0.15cm}{\rm min} \left [{\it \Gamma}_{6}(S_0) + d_{\rm H} \big ((00)\hspace{0.05cm},\hspace{0.05cm} (11) \big )\hspace{0.05cm},\hspace{0.2cm}{\it \Gamma}_{6}(S_2) + d_{\rm H} \big ((11)\hspace{0.05cm},\hspace{0.05cm} (11) \big ) \right ] ={\rm min} \left [ 3+2\hspace{0.05cm},\hspace{0.05cm} 3+0 \right ] \hspace{0.15cm}\underline{= 3}\hspace{0.05cm}.$$
Beim BSC–Modell kann man aus ${\it \Gamma}_7(S_{\mu}) = 3$ darauf schließen, dass drei Übertragungsfehler aufgetreten sind ⇒ Lösungsvorschläge 1 und 3.
(4) Richtig sind die Aussagen 1 und 2:
- Die Maximierung der Metriken ${\it \Lambda}_i(S_{\mu})$ entsprechend der rechten Skizze in obiger Grafik liefert das gleiche Ergebnis wie die links dargestellte Minimierung der Fehlergrößen ${\it \Gamma}_i(S_{\mu})$. Auch die überlebenden und gestrichenen Zweige sind in beiden Grafiken identisch.
- Die angegebene Gleichung ist ebenfalls richtig, was hier nur am Beispiel $i = 7$ gezeigt wird:
- $${\it \Lambda}_7(S_0)) = 2 \cdot \big [i - {\it \Gamma}_7(S_0) \big ] = 2 \cdot \big [7 - 3 \big ] \hspace{0.15cm}\underline{= 8}\hspace{0.05cm}.$$
- Die letzte Aussage ist falsch. Vielmehr gilt $〈x_i', \, y_i〉 ∈ \{–2, \, 0, \, +2\}$.
Hinweis: In der Aufgabe 3.11 wird für das gleiche Beispiel die Pfadsuche demonstiert, wobei von den ${\it \Lambda}_i(S_{\mu})$–Metriken gemäß der rechten Grafik ausgegangen wird.