Exercise 3.7: PN Modulation

equivalent circuit diagrams of „PN modulation” and „BPSK”

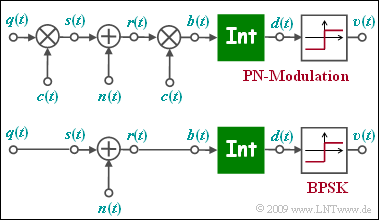
The upper figure shows the equivalent circuit diagram of PN modulation ( Direct Sequence Spread Spectrum, abbreviated DS-SS) in the equivalent low-pass range, where„ $n(t)$ stands for AWGN noise. Below, the low-pass model of binary phase modulation (BPSK) is sketched

The low-pass transmit signal $s(t)$ is equal to the rectangular source signal $q(t) ∈ \{+1, -1\}$ with rectangular duration $T$ only for reasons of uniformity.
The function of the integrator can be written as follows:

$d (\nu T) = \frac{1}{T} \cdot \int_{(\nu -1 )T }^{\nu T} \hspace{-0.03cm} b (t )\hspace{0.1cm} {\rm d}t \hspace{0.05cm}.$

The two models differ in the multiplication by the $±1$ -spreading signal $c(t)$ at the transmitter and receiver, whereas of this signal $c(t)$ only the spread degree $J$ is known.
The specification of the specific spreading sequence (M sequence or Walsh function) is not important for the solution of this task.

It has to be examined whether the lower BPSK model can also be applied with PN modulation and whether the BPSK error probability

$p_{\rm B} = {\rm Q} \left( \hspace{-0.05cm} \sqrt { {2 \cdot E_{\rm B}}/{N_{\rm 0}} } \hspace{0.05cm} \right )$

is also valid for PN modulation, or how the specified equation should be modified.

Notes:

This exercise belongs to the chapter Die Charakteristika von UMTS.

The CDMA method used for UMTS is also known as "PN modulation".
The nomenclature used in this task is also partly based on the PN–Modulation im Buch „Modulationsverfahren”.

Questionnaier

Musterlösung

Solution

(1) Richtig ist der Lösungsvorschlag 3:

Es handelt sich hier um einen optimalen Empfänger.
Ohne Rauschen ist das Signal $b(t)$ innerhalb eines jeden Bits konstant gleich $+1$ oder $-1$ .
Aus der angegebenen Gleichung für den Integrator

$d (\nu T) = \frac{1}{T} \cdot \hspace{-0.03cm} \int_{(\nu -1 )T }^{\nu T} \hspace{-0.3cm} b (t )\hspace{0.1cm} {\rm d}t$

folgt, dass

$d(\nu T)$ nur die Werte

$±1$ annehmen kann.

(2) Richtig ist wieder der Lösungsvorschlag 3:

Im rauschfreien Fall ⇒ $n(t) = 0$ kann auf die zweifache Multiplikation mit $c(t) ∈ \{+1, -1\}$ ⇒ $c(t)^{2} = 1$ verzichtet werden, so dass das obere Modell mit dem unteren Modell identisch ist.

(3) Zutreffend ist der Lösungsvorschlag 1:

Da beide Modelle im rauschfreien Fall identisch sind, muss nur das Rauschsignal angepasst werden: $n'(t) = n(t) \cdot c(t)$ .
Die Lösungsvorschläge 2 und 3 sind dagegen nicht zutreffend: Die Integration muss auch weiterhin über $T = J \cdot T_{c}$ erfolgen (nicht über $J \cdot T$ ) und die PN–Modulation verringert das AWGN–Rauschen nicht.

(4) Richtig ist der Lösungsvorschlag 3:

Die für BPSK und AWGN–Kanal gültige Gleichung

$p_{\rm B} = {\rm Q} \left( \hspace{-0.05cm} \sqrt { \frac{2 \cdot E_{\rm B}}{N_{\rm 0}} } \hspace{0.05cm} \right )$

ist somit auch bei der PN–Modulation anwendbar und zwar unabhängig vom Spreizfaktor

$J$ und von der spezifischen Spreizfolge.

Bei AWGN–Rauschen wird die Fehlerwahrscheinlichkeit durch Bandspreizung weder vergrößert noch verkleinert.

	$d(\nu T)$ is gauss distributed.
	$d(\nu T)$ can take the values $+1$ , $0$ und $-1$ .
	Only the values $d(\nu T) = +1$ und $d(\nu T) = -1$ are possible.

	The noise $n(t)$ must be replaced by $n\hspace{0.05cm}'(t) = n(t) \cdot c(t)$ .
	The integration must now be done via $J \cdot T$ .
	The noise power must be reduced by the factor $J$ .

	The larger $J$ is selected, the smaller $p_{\rm B}$ is.
	The larger $J$ is selected, the larger $p_{\rm B}$ .
	It results independently from $J$ , always the value $p_{\rm B} = 2.3 \cdot 10^{-3}$ results.