Exercise 2.7: Two-Way Channel once more

Magnitude frequency response and phase function of the two-way channel

As in Exercise 2.6, a two-way channel is considered whose impulse response is:

$$h(t) = \delta ( t - T_1) + \delta ( t - T_2).$$

In contrast to the general representation in task 2.6, the two attenuation factors are equal here: $z_1 = z_2 = 1$.

For mobile communications, this corresponds to an echo at a distance of $T_2 - T_1$ and of the same strength as the signal on the main path, for example.
For this, the transit time $T_1$ is assumed.

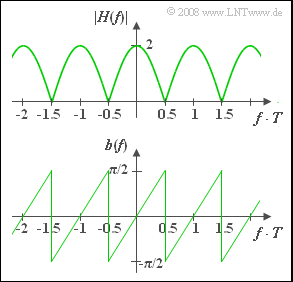
Using the transit times $T_1 = 0$ and $T_2 = T = 4 \ \rm ms$ considered in the subtasks (1) ... (4), the following is obtained for the frequency response of the two-way channel, whose magnitude is depicted in the upper graph:

$$H(f) = 1 + {\rm e}^{-{\rm j}\hspace{0.04cm}2 \pi f T} = 1 + \cos(2 \pi f T) - {\rm j} \cdot \sin(2 \pi f T)$$

$$\Rightarrow \hspace{0.4cm}|H(f)| = \sqrt{2\left(1 + \cos(2 \pi f T)\right)}= 2 \cdot |\cos(\pi f T)|.$$

The bottom graph shows the phase function:

$$b(f) = - {\rm arc} \hspace{0.1cm}H(f) = \arctan \frac{\sin(2 \pi f T)}{1 + \cos(2 \pi f T)} = \arctan \big[\tan(\pi f T)\big].$$

The following trigonometric transformation was used here:

$$ \frac{\sin(2 \alpha)}{1 + \cos(2 \alpha)} = \tan(\alpha).$$

The bottom graph shows thep hase function for $T_1 = 0$ and $T_2 = T = 4 \ \rm ms$:

In the frequency range $|f| < 1/(2T)$, $b(f)$ increases linearly: $b(f) = \pi \cdot f \cdot T.$
Also in further sections of the phase function, the phase always increases linearly from $-\pi/2$ to $+\pi/2$ .

In the questions, $y_i(t)$ denotes the signal at the output of the two-way channel if the signal $x_i(t)$ is applied to the input $( i = 1, 2, 3, 4)$.

These signals are examined as input signals:

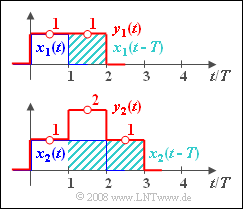
a rectangular pulse $x_1(t)$ with height $1$ between $t= 0$ and $t= T$;
$x_1(t) = 0$ holds for $t < 0$ and $t > T$ $($the value$0.5$ occurs at the two jump discontinuities, respectively$)$;
a rectangular pulse $x_2(t)$ with height $1$ in the range of $0 \ \text{...} \ 2T$;
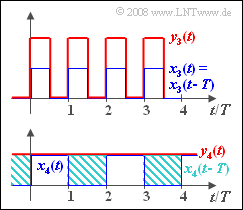
a periodic rectangular pulse $x_3(t)$ with period $T = T_0$:

$$x_3(t) = \left\{ \begin{array}{c} 1 \\ 0 \\ \end{array} \right.\quad \quad \begin{array}{c} {\rm{for}} \\ {\rm{for}} \\ \end{array}\begin{array}{*{20}c} { 0 < t < T/2,} \\ { T/2 < t < T,} \\ \end{array}$$

a periodic rectangular pulse $x_4(t)$ with period $T = 2T_0$:

$$x_4(t) = \left\{ \begin{array}{c} 1 \\ 0 \\ \end{array} \right.\quad \quad \begin{array}{c} {\rm{for}} \\ {\rm{for}} \\ \end{array}\begin{array}{*{20}c} { 0 < t < T,} \\ { T < t < 2T.} \\ \end{array}$$

Please note:

The task belongs to the chapter Linear Distortions.
For subtasks (1) to (4), $T_1 = 0$ and $T_2 = T = 4 \ \rm ms$ hold.
In subtask (5), the case $T_1 = 1 \ \rm ms$ and $T_2 = 5 \ \rm ms$ is considered.

Questions

Solution

(1) Die Lösung im Zeitbereich führt schneller zum Endergebnis:

$$y_1(t) = x_1(t) \star h(t) = x_1(t) \star \delta (t) + x_1(t) \star \delta (t - T) = x_1(t) + x_1(t-T).$$

Somit ist $y_1(t)$ ein Rechteckimpuls der Höhe $1$ und der Breite $2T$.

Zum gleichen Ergebnis – aber zeitaufwändiger – kommt man durch die Berechnung im Spektralbereich:

$$Y_1(f) = X_1(f) \cdot H(f) = T \cdot \frac {\sin(\pi f T)}{\pi f T}\cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm} \pi f T} \cdot \big[ 1 + {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T} \big].$$

Die komplexen Exponentialfunktionen können mit dem Satz von Euler wie folgt umgewandelt werden:

$${\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm} \pi f T} \big[ 1 + {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T} \big] = {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T} \cdot \big[ {\rm e}^{{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm} \pi f T} + {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm} \pi f T} \big] = {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T} \cdot 2 \cos(\pi f T) .$$

Somit kann für das Ausgangsspektrum geschrieben werden:

$$Y_1(f) = Y_{11}(f) \cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T} , \; \; {\rm mit } \; \; Y_{11}(f) = 2T \cdot \frac {\sin(\pi f T) \cdot \cos(\pi f T)}{\pi f T} = 2T \cdot \frac {\sin(2\pi f T) }{2\pi f T}.$$

Eingangs– und Ausgangssignale

Hierbei ist die Beziehung $\sin(\alpha) \cdot \cos(\alpha) = \sin(2\alpha)/2$ verwendet.

Die Fourierrücktransformation von $Y_{11}$ führt zu einem um $t = 0$ symmetrischen Rechteck der Breite $2T$.
Durch die Phasenfunktion wird dieser in den Bereich $0$ ... $2T$ verschoben und das Ergebnis der Zeitbereichsberechnung bestätigt.

Trotz der Tatsache, dass $y_1(t)$ ebenso wie $x_1(t)$ rechteckförmig ist, liegen Verzerrungen vor:

Wegen $T_y > T_x$ sind diese linear. Im interessierenden Frequenzbereich $($das sind bei einem si–förmigem Spektrum alle Frequenzen$)$ ist $|H(f)|$ nicht konstant. Also gibt es Dämpfungsverzerrungen.
Da zudem die Phase nicht im gesamten Bereich linear mit $f$ ansteigt, gibt es auch Phasenverzerrungen ⇒ Richtig sind die Lösungsvorschläge 1, 3, 4 und 5.

(2) Aufgrund der bereits in (1) angegebenen Gleichung

$$y_2(t) = x_2(t) + x_2(t-T)$$

erhält man einen stufenförmigen Verlauf entsprechend dem unteren Diagramm der obere Grafik.

Die gesuchten Zahlenwerte sind: $y_2(t = 0.5 T) \hspace{0.15cm}\underline{= 1}, \hspace{0.3cm} y_2(t = 1.5 T) \hspace{0.15cm}\underline{= 2}, \hspace{0.3cm}y_2(t = 2.5 T) \hspace{0.15cm}\underline{ = 1}.$

Lösungen (3) und (4)

(3) Die Periodendauer $T_0 = T$ des periodischen Signals $x_3(t)$ ist genau so groß wie die Verzögerung auf dem zweiten Pfad. Deshalb ist $y_3(t) = 2 \cdot x_3(t) $ und es sind keine Verzerrungen feststellbar.

Die Spektralbereichsberechnung führt zum gleichen Ergebnis.

$X_3(f)$ ist ein Linienspektrum mit Anteilen bei den Frequenzen $f = 0$, $f = \pm f_0 = \pm 1/T$, $f = \pm 3f_0$, usw..
Bei diesen diskreten Frequenzen gilt aber exakt:

$$|H(f)| = 2, \hspace{0.3cm} b(f) = 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\tau_{\rm P}(f) = 0.$$

Auch daraus folgt wieder $y_3(t) = 2 \cdot x_3(t) $.
Richtig ist somit nur der Lösungsvorschlag 1.

(4) Aus der unteren Skizze der zweiten Grafik geht hervor, dass $y_4(t) = 1$ gegenüber $x_4(t)$ verzerrt ist. Dabei handelt es sich um Dämpfungsverzerrungen ⇒ Lösungsvorschlag 2, wie die folgende Überlegung zeigt.

Wegen $T_0 = 2T$ weist das Signal $x_4(t)$ die Grundfrequenz $f_0 = 1/(2T)$ auf.
Bei allen ungeraden Vielfachen von $f_0$ hat somit der Frequenzgang Nullstellen.
Die einzige verbleibende Spektrallinie von $Y_4(f)$ liegt bei $f = 0$, wobei gilt:

$$Y_4(f) = 2 \cdot 0.5 \cdot \delta (f) = 1 \cdot \delta (f) \hspace{0.5cm}\Rightarrow \hspace{0.5cm} y_4(t) = 1.$$

(5) Der Frequenzgang lautet nun mit $T_1 = 1 \ \rm ms$, $T_2 = 5 \ \rm ms$ und $T = T_2 -T_1 = 4 \ \rm ms$:

$$H(f) = {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T_1}+ {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T_2}= \big[ 1 + {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T} \big]\cdot {\rm e}^{-{\rm j}\hspace{0.05cm} \cdot \hspace{0.05cm}2 \pi f T_1}.$$

Der Klammerausdruck beschreibt den bereits bisher betrachteten Frequenzgang.
Der zweite Term bewirkt eine zusätzliche Laufzeit um $ \tau = T_1$, und es gilt für alle Signale $(i = 1, 2, 3, 4)$:

$$y_i^{\rm (5)}(t) = y_i(t-T_1).$$

Alle Aussagen hinsichtlich der Verzerrungen sind weiter gültig. Dies entspricht dem Lösungsvorschlag 1.

	$y_1(t)$ is rectangular like $x_1(t)$ .
	$y_1(t)$ is triangular in shape.
	The absolute pulse duration is $2T$.
	$y_1(t)$ exhibits attenuation distortions with respect to $x_1(t)$ .
	$y_1(t)$ exhibits phase distortions with respect to $x_1(t)$ .

	$y_3(t)$ ist gegenüber $x_3(t)$ unverzerrt.
	$y_3(t)$ weist gegenüber $x_3(t)$ Dämpfungsverzerrungen auf.
	$y_3(t)$ weist gegenüber $x_3(t)$ Phasenverzerrungen auf.

	$y_4(t)$ ist gegenüber $x_4(t)$ unverzerrt.
	$y_4(t)$ weist gegenüber $x_4(t)$ Dämpfungsverzerrungen auf.
	$y_4(t)$ weist gegenüber $x_4(t)$ Phasenverzerrungen auf.

	Die obigen Aussagen hinsichtlich Verzerrungen sind weiterhin gültig.
	Fundierte Aussagen sind erst nach einer Neuberechnung möglich.
	Die Kombination $T_1 = 1 \ \rm ms$ und $T_2 = 5 \ \rm ms$ führt bei allen Signalen zu Verzerrungen.